一类非自治差分竞争系统的持久性及正周期解的全局渐近稳定性

付贞文， 向会立

(1.咸丰县黄金洞民族中小学，湖北 咸丰 445614；2.湖北民族学院 理学院，湖北 恩施 445000)

一类非自治差分竞争系统的持久性及正周期解的全局渐近稳定性

付贞文1， 向会立2*

(1.咸丰县黄金洞民族中小学，湖北 咸丰 445614；2.湖北民族学院 理学院，湖北 恩施 445000)

建立了一类非自治差分竞争系统, 通过运用差分方程理论、Brouwer定理和Lyapunov 函数方法, 分别获得了系统的持久性、正周期解的存在性和全局渐近稳定性的充分条件.

非自治差分竞争系统；持久性；正周期解；全局渐近稳定性

文献[1]介绍了如下连续自治系统:

(1)

在本文中,将研究系统(1)的离散形式:

(2)

其中n∈N,N是自然数集.在这个问题的研究上，主要受到了文献[2-6]启发.

在本文中,令:fU=supn∈N{f(n)},fL=infn∈N{f(n)},其中f(n)是非负的有界函数.在系统(2)中,r1(n),r2(n)为净增长率,a1(n),a2(n)为种内竞争率,b1(n),b2(n)为种间竞争率,c1(n),c2(n)为扰动系数.同时,因为生物意义的原因,只考虑x1(0)>0,x2(0)>0,bi(n)≥0,ci(n)≥0(i=1,2)时解的情况.

1 持久性

在本节,将研究系统(2)的持久性.

引理1 如果存在n0∈N使得xi(n0+1)≥xi(n0),那么对所有的n>n0，就有:

(i=1,2).

(3)

证明因为存在n0∈N,使得xi(n0+1)≥xi(n0),由系统(2)可得:

由上面的式子可得ri(n0)-ai(n0)xi(n0)≥0,经变形可得到下面的式子：

(4)

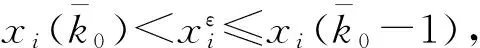
引理2 如果对任意的n∈N，都有xi(n+1)

(i=1,2).

(5)

利用minn∈R+{exp(x-1)/x}=1,R+是正实数集,可以得到:

(6)

通过引理1和引理2,得到了下面的定理.

定理1 对任意的正解xi(n)(i=1,2),系统(2)满足：

(i=1,2).

(7)

引理3 对任意的ε>0,存在n*∈N,对所有的n≥n*,xi(n)≤Mi+ε成立.

引理4 假设存在n0≥n*,使得xi(n0+1)≤xi(n0),同时，假定系统(2)满足如下条件:

(8)

证明当存在n0≥n*,使得xi(n0+1)≤xi(n0)时,由系统(2)可以得到:

因此,

(10)

通过式(9)和式(10)就有:

“中央空调主机智能节电管理系统”是通过采集末端和室外的温、湿度变化信号，经过服务器AS4N分析和运算，给出控制信号到控制器GCRE，控制器控制主机按原厂自有的逻辑调节空调负载。把空调主机和末端直接、统一管理，实现了中央空调系统的协调、即时运行和综合性能优化。智能节电管理系统的核心就是数据库和策略库。如图3所示。

引理5 如果对所有的n≥n*,有xi(n+1)>xi(n)成立,那么有:

定理2 如果式(8)成立,那么对任意的正数解xi(n),系统(2)满足:

由定理1和定理2,得到如下定理.

定理3 如果式(8)成立,那么系统(2)具有持久性.

2 正周期解的存在性和全局渐近稳定性

在这部分假设系统(2)是周期性系统,同时,将进一步研究这个系统的正周期解具有全局渐近稳定性.因此,假设系统(2)的所有系数是ω-周期的.

证明令J=[m1,M1]×[m2,M2],由定理1和定理2知J是系统(2)的不变集.定义J到F的连续映射:

定理5 若条件(8)成立，且有:

(12)

则系统(2)的正周期解是全局渐近稳定的.

(13)

那么系统(2)将改写如下:

由微分中值定理可以得到:

(14)

其中θi∈(0,1)(i=1,2,3,4,5,6).由式(12),当选取ε充分小时就有:

由定理1和定理2.存在n0∈N,当n≥n0时有：

由于θi∈(0,1)(i=1,2,3,4,5,6),以及式(13),由系统(14)的第一个式子可得:

max{|u(n+1)|,|v(n+1)|}≤λεmax{|u(n)|,|v(n)|}≤(λε)n-n0max{|u(n0)|,|v(n0)|}.

[1] Gopalsamy K. Stability and oscillations in delay differential equations of population dynamics[M].Boston：Kluwer Academic,1992.

[2] Xiang,H L. Permanence and positive periodic solutions of a difference competitive system with non-linear disturbance[C]//Proceedings of the 7th conference on biological dynamic system and stability of differential equation, Chongqing,May,2010:17-20.

[3] Qin, W J.Permanence and global stability of positive periodic solutions of discrete competitive system[J].Discrete Dyn Nat Soc，2009,Art.ID 830537,13.

[4] Chen F D.Permanence and global atractivity of a discrete multispecies Lotka-VoLterra competion predator-prey systems[J].Appl Math Comput，2008,182(1):2-12.

[5] Zhou Z.Stable periodic solutions in discrete periodic logistic equation[J].Appl Math Comput,2003,16(2):165-171.

[6] 周敏.一类与算子谱对应的方程解的性质研究[J]. 湖北民族学院学报：自然科学版,2009,27(4):391-393.

PermanenceandGlobalAsymptoticStabilityofPositivePeriodicSolutionsofaNonautonomousDifferenceCompetitiveSystem

FU Zhen-wen1,XIANG Hui-li2*

(1.Xianfeng Huangjindong Junior High and Primary School for Nationalities,Xianfeng 445614,China;2.School of Science, Hubei University for Nationalities, Enshi 445000,China)

In this paper,a nonautonomous difference competitive system is established. By using the difference equation theory,Brouwer fixed point theorem and Lyapunov function, sufficient conditions are obtained for the permanence of system, the existence and global asymptotic stability of positive periodic solutions of system.

nonautonomous difference competitive system,permanence; positive periodic solution; global asymptotic stability

2013-08-16.

教育部重点项目(212111).

付贞文(1984-)，男(土家族)，主要从事常微分方程研究;*

:向会立(1979-)，男(土家族)，博士生，讲师，主要从事随机偏微分方程、最优控制方面的研究.

0175.2

A

1008-8423(2013)03-0267-04