On Generalized PST-groups∗

WANG JUN-XIN

(Institute of Mathematics,Shanxi University of Finance and Economics,Taiyuan,030031)

On Generalized PST-groups∗

WANG JUN-XIN

(Institute of Mathematics,Shanxi University of Finance and Economics,Taiyuan,030031)

A finite groupGis called a generalizedPST-group if every subgroup contained in F(G)permutes all Sylow subgroups of G,where F(G)is the Fitting subgroup of G.The class of generalized PST-groups is not subgroup and quotient group closed,and it properly contains the class of PST-groups.In this paper,the structure of generalized PST-groups is first investigated.Then,with its help,groups whose every subgroup(or every quotient group)is a generalized PST-group are determined,and it is shown that such groups are precisely PST-groups.As applications, T-groups and PT-groups are characterized.

s-permutable subgroup,power automorphism,PST-group

1 Introduction

Let G be a finite group.A subgroup H of G is said to be s-permutable in G,if H permutes all Sylow subgroups of G.It is well-known that an s-permutable subgroup is subnormal(see [1]).In this paper,we call G a generalized PST-group if every subgroup of G contained in F(G)is s-permutable in G,where F(G)is the Fitting subgroup of G.Agrawal[2]and many other authors have studied the so-called PST-group,i.e.,group in which subnormal subgroups are s-permutable(see,e.g.,[3]–[5]).Since subgroups of G contained in F(G)are subnormal in G,it is clear that PST-groups are certainly generalized PST-groups.But the converse is not true.A counterexample is as follows:

Example 1.1Suppose that A=hai is a cyclic group of order 7 and

is the symmetric group on three letters.Let

Clearly,the mapping

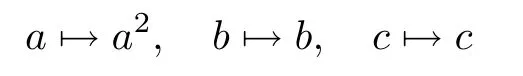
determines an automorphism α of H of order 3.Let G be the semidirect product of H and hαi.Then

(i) F(G)=hai×hbi,and every subgroup of F(G)is normal in G.Hence G is a generalized PST-group.

(ii)Let

Since hbαi cannot permute the Sylow 2-subgroup hci of K,K is not a generalized PST-group.

(iii)As G/A～=K,G/A is not a generalized PST-group.

(iv)G is not a PST-group because any quotient group of a PST-group is still a PST-group.

From the above example we see that the class of generalized PST-groups is not subgroup and quotient group closed,and it properly contains the class of PST-groups.So it is meaningful to investigate the generalized PST-groups.Especially,we are interested in determining the groups in which every subgroup(or every quotient group)is still a generalized PST-group.The result we obtain is surprising:those groups are precisely PST-groups.In our investigation,power automorphisms play an important role.A power automorphism of a group G is an automorphism that leaves every subgroup of G invariant.Such an automorphism maps each element to one of its powers.The main result is the following:Theorem 1.1LetGbe a finite group.Then the following conditions are equivalent:

(i)Every subgroup ofGis aPST-group;

(ii)Every subgroup ofGis a generalizedPST-group;

(iii)The nilpotent residueLofG,i.e.,the smallest term of the lower central series ofG,is an abelian Hall subgroup ofGof odd order,and every element ofGinduces a power automorphism inL;

(iv)Gis a solvablePST-group;

(v)Gis solvable and every quotient group ofGis a generalizedPST-group.

A direct consequence of Theorem 1.1 is the following corollary:

Corollary 1.1A non-solvablePST-group has a subgroup which is not aPST-group.

Let G be a finite group.G is called a T-group if every subnormal subgroup of G is normal in G,and G is called a PT-group if every subnormal subgroup of G permutes all subgroups of G.Clearly,T-groups and PT-groups are both PST-groups.By Theorem 1.1, Lemma 13.4.5 of[6]and Lemma 1 of[7],we can easily obtain the following results:

Corollary 1.2[8]A finite solvable groupGis aT-group if and only if it has an abelian normal Hall subgroupLof odd order such thatG/Lis a Dedekind group and every element ofGinduces a power automorphism inL.

Corollary 1.3[9]A finite solvable groupGis aPT-group if and only if it has an abelian normal Hall subgroupLof odd order such thatG/Lis a quasi-Hamiltonian group and every element ofGinduces a power automorphism inL.

A finite group G is called a quasi-Hamiltonian group if HK=KH for any two subgroups H and K of G.The structure of quasi-Hamiltonian groups has been determined in[10].

All groups considered in this paper are finite.For a group G,if H≤G,then G−H denotes the set{x|x∈G but x 6∈H},and HGdenotes the core of H in G.Other notations and terminologies without mention here agree with standard usage.

2 Proofs of Main Results

Recall that an automorphism α of a group G is said to be fixed-point-free if CG(α)=1.

Lemma 2.1([11],Lemma 3)A groupGis an abelian group of odd order if and only ifGhas a fixed-point-free power automorphism.

Lemma 2.2Letpbe a prime.If ap-groupPhas a non-trivial power automorphismαwith order prime top,thenαis fixed-point-free andPis abelian of odd order.

Proof.By Lemma 2.1,if α is fixed-point-free,then P is abelian of odd order.So we need only to prove that

Now assume the conclusion is false,and let P be a counterexample of minimal order.Since α is non-trivial,we have

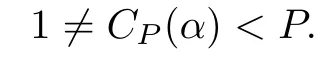
Let M be a maximal subgroup of P containing CP(α).By the minimality of P,we see that

Let x∈P−M.Then

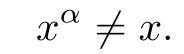
It follows from Theorem 13.4.3 of[6]that

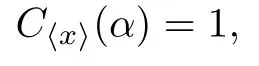
and therefore

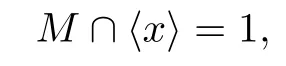
from which we deduce that

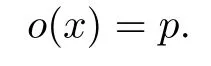
Choose y∈M∩Z(P)such that

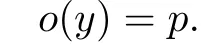
Clearly,

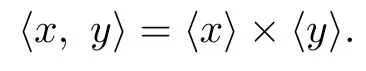
Note that xαx,yα=y,so it is easy to see that there exists no integer l such that

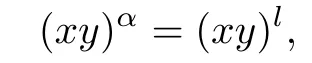
a contradiction.The proof is completed.

Lemma 2.3[12]LetGbe a solvable group.If every maximal subgroupMofGwithhas prime index,thenGis supersolvable.

Lemma 2.4A solvable generalizedPST-groupGis supersolvable.

Proof.Since

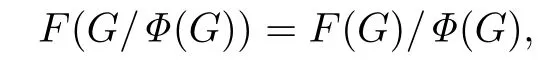
the hypothesis on G is inherited by G/Φ(G)and ifΦ(G)1,then G is supersolvable by induction.Hence we may assume thatΦ(G)=1 so that F(G)is the product of all the minimal normal subgroups of G.Let M be a maximal subgroup of G withThen there exists a minimal normal subgroup N of G such that

Clearly,N is a p-group for some prime p.Let

where P∈Sylp(M),and H is a Hall p′-subgroup of M.Since

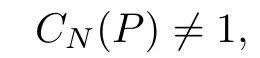
there exists a subgroup N1≤CN(P)such that

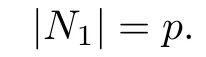
Note that N1is s-permutable in G and H is a Hall p′-subgroup of G,so it is easy to see that

and therefore

By Lemma 2.3,G is supersolvable.The proof is completed.

A subgroup H of a group G is called a direct product factor of G if there exists a subgroup K of G such that

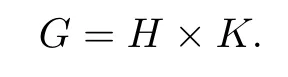
Lemma 2.5LetGbe a group such that any Sylow subgroup ofGis not a direct product factor ofG.ThenGis a solvable generalizedPST-group if and only ifGsatis fies the following two conditions:

(i)G=NHis a semidirect product of two Hall subgroupsNandH,whereNis normal abelian of odd order andHis supersolvable which contains no normal Sylow subgroup ofG, and every element ofHinduces a power automorphism inN;

(ii)For any primepdividing|H|,everyp′-element ofHinduces a power automorphism inOp(G).

Proof.The sufficiency of the condition is clear,we only prove the necessity.

Suppose that G is a solvable generalized PST-group.First we claim that for any prime p dividing|G|,every p′-element g of G induces a power automorphism in Op(G).In fact, let T be a Hall p′-subgroup of G containing g.If A≤Op(G),then

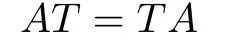
by the hypothesis,and so

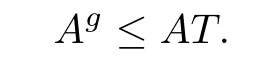
It follows that

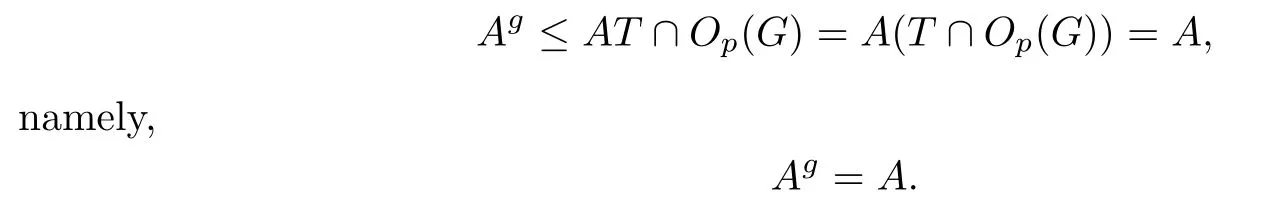
Hence our claim holds.Now let P be a normal Sylow subgroup of G.Suppose that P is a p-group for some prime p.Since P=Op(G)and P is not a direct product factor of G,there exists a p′-element g of G such that g induces a non-trivial power automorphism in P.By Lemma 2.2,P is abelian of odd order.Moreover,as any p′-element of G induces a power automorphism in P,every subgroup of P is normal in G.According to Lemma 2.4,G is supersolvable and so G has normal Sylow subgroups.Let N be the product of all normal Sylow subgroups of G.Clearly N is a normal abelian Hall subgroup of G of odd order,and every subgroup of N is normal in G.Let H be a complement of N in G.It is easy to see that N and H satisfy(i)and(ii).The proof is completed.

Since any group G can be decomposed into a direct product of two Hall subgroups G1and G2,where G1is nilpotent and G2has the property that any Sylow subgroup of it is not a direct product factor of G2,so we have the following result for arbitrary solvable generalized PST-groups.

Theorem 2.1A groupGis a solvable generalizedPST-group if and only ifGis a direct product of two Hall subgroupsG1andG2,whereG1is nilpotent,andG2is a group described by Lemma2.5.

Lemma 2.6If every subgroup of a groupGis a generalizedPST-group,thenGis solvable.

Proof.By induction,every maximal subgroup of G is solvable,and further by Lemma 2.4, every maximal subgroup of G is supersolvable.It follows from Theorem 9.6 of[13]that G is solvable.

Lemma 2.7LetGbe a group such that any Sylow subgroup ofGis not a direct product factor ofG.If every subgroup ofGis a generalizedPST-group,orGis solvable and every quotient group ofGis a generalizedPST-group,then the nilpotent residueLofGis an abelian Hall subgroup ofGof odd order,and every element ofGinduces a power automorphism inL.

Proof.According to Lemma 2.6,G is solvable,and by Lemma 2.5,G is a semidirect product of a normal abelian Hall subgroup N of G of odd order and a supersolvable subgroup H which contains no normal Sylow subgroup of G,and every element of H induces a power automorphism in N.We need only to prove that H is nilpotent and N is precisely the nilpotent residue of G.

(1)H is nilpotent.

Since G/N～=H,H is a generalized PST-group.Let p be the largest prime dividing|H| and P the normal Sylow p-subgroup of H.As in the proof of Lemma 2.5,we can similarly obtain that every p′-element of H induces a power automorphism in P.

Now we prove that P is a direct product factor of H.Assume the contrary.Then there would exist a q-element a∈H with qp such that a induces a non-trivial power automorphism in P.By Lemma 2.2,

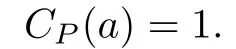
Also since P is not normal in G,the action of P on N is non-trivial.Hence there exists an element b∈P and an r-element c∈N with c1 such that b acts non-trivially on hci, where p,q and r are distinct primes.By Lemma 2.2 again,

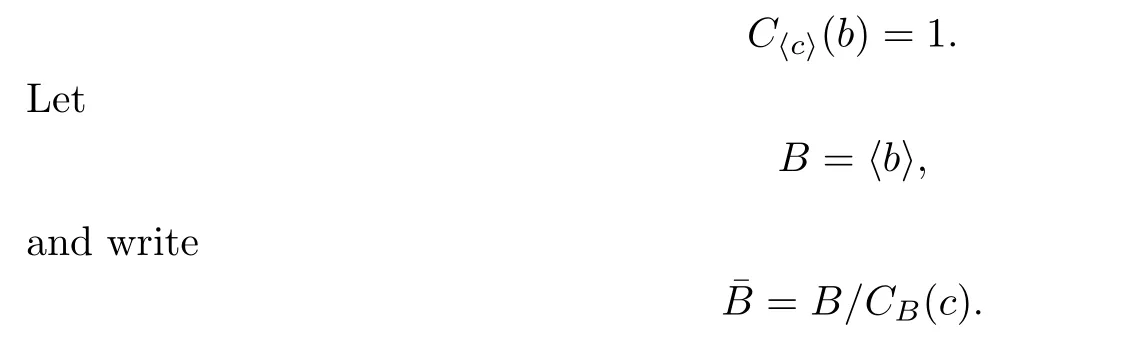
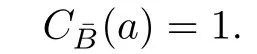
Let E be the semidirect product ofand hai.Clearly,E is non-abelian.Let E act on hci in the natural way.Denote E/CE(c)by.Since

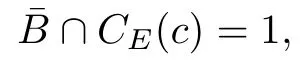
CE(c)can only be a q-group,and so

then

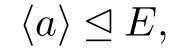
and therefore

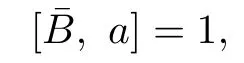
a contradiction.If

Let K be the normal Hall p′-subgroup of H.As NPG and G/NPK,K is also a generalized PST-group,and any Sylow subgroup of K cannot be normal in G.By induction, K is nilpotent,from which it follows that H is nilpotent.

(2)N is the nilpotent residue of G.

Let L be the nilpotent residue of G.Since H is nilpotent,we have

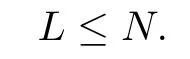
If L＜N,then there exists a Sylow subgroup S of N such that

Since S is not a direct product factor of G,there exists an element h∈H such that h induces a non-trivial power automorphism in S.By Lemma 2.2 once more,

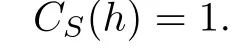
It follows from Theorem 7.19 of[14]that

and therefore

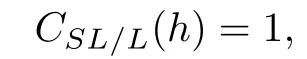
contradicting the fact that G/L is nilpotent.Hence

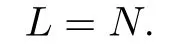
The proof is completed.

Lemma 2.8LetNbe a normal Hall subgroup of a groupGand assume that the following hold:

(i)G/Nis aPST-group;

(ii)Every subnormal subgroup ofNis normal inG.

ThenGis aPST-group.

Proof.Let H be a subnormal subgroup of G.We show that H is s-permutable.By(ii), H∩NG and G/(H∩N)satis fies(i)and(ii).By induction on|G|we can assume that H∩N=1.Let M be a complement of N in G.Since

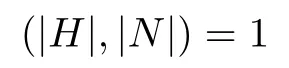
and H is subnormal,we have

and therefore

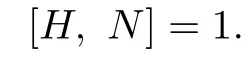
Let P be any Sylow subgroup of G.If P≤N,then

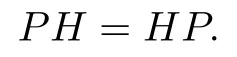
If P∩N=1,then there exists g∈G such that

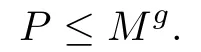
Note that

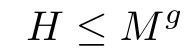
and MgG/N is a PST-group,so

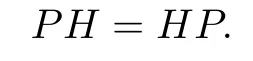
The proof is completed.

Proof of Theorem 1.1Since PST-groups are generalized PST-groups,and quotient groups of PST-groups are still PST-groups,we need only to prove that condition(ii)or(v) can imply condition(iii),and condition(iii)can imply conditions(i)and(iv).

First suppose that G satis fies condition(ii)or(v),we prove that G satis fies(iii).In fact,let G1be the product of all Sylow subgroups of G that are direct product factors of G. Then G1is a normal nilpotent Hall subgroup of G and there exists another Hall subgroup G2of G such that

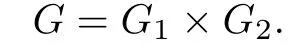
Clearly,any Sylow subgroup of G2is not a direct product factor of G2.If G satis fies (ii),then G2satis fies(ii).If G satis fies(v),then for any normal subgroup N of G2,since G2/N ～=G/G1N,we see that G2also satis fies(v).By Lemma 2.7,the nilpotent residue L2of G2is an abelian Hall subgroup of G2of odd order,and every element of G2induces a power automorphism in L2.It is clear that L2,being a Hall subgroup of G,is also the nilpotent residue of G and every element of G induces a power automorphism in L2.This shows that G satis fies(iii).

Now suppose that G satis fies(iii).It is clear that G is solvable.Let K be any subgroup of G.Since nilpotent groups are PST-groups and

by Lemma 2.8,K is a PST-group.Thus every subgroup of G is a PST-group.Especially G itself is a solvable PST-group.This completes the proof.

[1]Deskins,W.E.,On quasinormal subgroups of finite groups,Math.Z.,82(1963),125–132.

[2]Agrawal,R.K.,Finite groups whose subnormal subgroups permute with all Sylow subgroups,Proc.Amer.Math.Soc.,47(1975),77–83.

[3]Alejander,M.J.,Ballester-Bolinches,A.and Pedraza-Aguilera,M.C.,Finite soluble groups with permutable subnormal subgroups,J.Algebra,240(2001),705–722.

[5]Ballester-Bolinches,A.and Esteban-Romero,R.,On finite soluble groups in which Sylow permutability is a transitive relation,Acta Math.Hungar.,101(2003),193–202.

[6]Robinson,D.J.S.,A Course in the Theory of Groups,Springer-Verlag,New York,1982.

[7]Beidleman,J.C.,Brewster,B.and Robinson,D.J.S.,Criteria for permutability to be transitive in finite groups,J.Algebra,222(1999),400–412.

[9]Zacher,G.,I gruppi risolubili fi niti in cui i sottogruppi di composizione coincidono con i sottogruppi quasi-normali,Atti Accad.Naz.Lincei Rend.Cl.Sci.Fis.Mat.Natur.,37(1964), 150–154.

[10]Gheorghe,P.,On the structure of quasi-Hamilton groups,Acad.Repub.Pop,Romˆane.Sti., 1(1949),973–979.

[11]Zhang,Q.H.and Wang,J.X.,Finite groups with only quasinormal and selfnormal subgroups,Acta Math.Sinica(Chinese Series),38(1995),381–385.

[12]Kramer,O.U.,Uber durchschnitte von untergruppen endlicher au flsbarer gruppen,Math. Z.,148(1976),88–97.

[13]Huppert,B.,Endliche Gruppen I,Springer-Verlag,Berlin,1968.

[14]Kurzweil,H.,Endliche Gruppen,Springer-Verlag,Berlin and New York,1977.

Communicated by Du Xian-kun

20D10,20D20

A

1674-5647(2011)04-0360-09

date:Sept.2,2010.

The NSF(11071155)of China,the Science and Technology Foundation(20081022)of Shanxi Province for Colleges,and the Team Innovation Research Foundation of Shanxi University of Finance and Economics.