InAs管状团簇及单壁InAs纳米管的结构、稳定性和电子性质
2011-11-30刘志锋祝恒江刘立仁
刘志锋 祝恒江 陈 杭 刘立仁
(新疆师范大学物理与电子工程学院,乌鲁木齐830054)
InAs管状团簇及单壁InAs纳米管的结构、稳定性和电子性质
刘志锋 祝恒江*陈 杭 刘立仁
(新疆师范大学物理与电子工程学院,乌鲁木齐830054)
采用密度泛函理论研究了InnAsn(n≤90)管状团簇以及单壁InAs纳米管的几何结构、稳定性和电子性质.小团簇InnAsn(n=1-3)基态结构和电子性质的计算结果与已有报道相一致.当n≥4时优化得到了一族稳定的管状团簇,其结构基元(In原子与As原子交替排列的四元环和六元环结构)满足共同的衍化通式.团簇的平均结合能表明横截面为八个原子的管状团簇稳定性最好.管状团簇前线轨道随尺寸的变化规律有效地解释了一维稳定管状团簇的生长原因,同时也说明了实验上之所以能合成InAs纳米管的微观机理.此外,研究结果表明通过管状团簇的有效组装可得到宽带隙的InAs半导体单壁纳米管.
InnAsn管状团簇;InAs纳米管;密度泛函理论;几何结构;稳定性;电子性质
1 Introduction
Recently,low-dimensional semiconductor materials are of great interest to researchers in the field of nanoscience.1-5Among these materials,the III-V group semiconductor com-pounds havs been paid much attention due to their paramount technological potential applications,6-17such as optoelectronic device,photonics integrations,ultra high frequency microwave device,ultra high-speed microelectronic device,and photovoltaic solar cells.
Indium arsenide(InAs),as a substantial member of the III-V group semiconductor compounds,has many distinctive properties within a narrow band gap of 0.36 eV at 300 K,such as low electron effective mass,high-electron mobility 33000 cm2·V-1· s-1,and high-saturation drift velocity,which make it ideal for optoelectronic,low power,and ultra high-speed device application.Moreover,it is the advantageous material for making nanoscale devices due to its surface Fermi level pinning in the conduction band without becoming an insulator because of depletion of carriers.Hence,for the past decades,there have been a number of studies18-24which examined the chemical and physical properties of its nanostructure.For instance,Persson et al.22fabricated the dense and uniform InAs nanowire arrays.Cirlin et al.23investigated the formation of InAs quantum dots on a silicon(100)surface.Mohan et al.24reported the realization of ordered arrays of single crystalline InAs nanotubes based on lattice-mismatchedInP/InAscore-shellnanowires,which showed that the synthesized nanotubes are highly uniform and conductive with well-defined size and shape.Costales et al.25,26studied the structure,geometry,and vibrational frequencies of InnAsnclusters with n=1-3 with DFT.
Although several theoretical and experimental studies have been carried out,there have been few studies of the structures, stabilities,and electronic properties of InnAsntubelike clusters and infinite single-walled InAs nanotubes(InAsNTs).As we know,the nanoclusters or nanotubes can be considered as the transitional forms between atoms and bulk,and their fundamental properties depend crucially on their size.27It is possible to tune physical and chemical properties of these nanostructures by changing their size,making them promising materials for numerous applications.Apparently,to understand the unique properties of tubular InAs nanostructures on different nanometer scale,the structures and electronic properties of InAs tubelike clusters and nanotubes should be clarified first.Therefore,this study intends to investigate stoichiometric InnAsn(n≤90)tubelike clusters and InAsNTs with DFT method,especially to seek the answer for the following questions.(1)When can the stable tubelike clusters be found?(2)Are there any laws of the structuresʹgrowth of the clusters and InAsNTs?(3)How about their stabilities and electronic properties,such as binding energy,HOMO-LUMO energy gaps,and energy band structures?(4)Why can the stable one-dimensional tubular structures be obtained in the previous experimental study and our theoretical calculation?(5)Are the small-bore tubelike clusters and InAsNTs still conductive or semiconductive with a narrow band gap?
2 Computational methods
This research examines the structures and characteristics of tubelike InnAsn(n≤90)clusters by using the DFT with unrestricted B3LYP exchange-correlation potential.28,29For better describing the bonding and geometrical features of heavy atoms,the basis set,effective core potential LanL2DZ,30was adopted by this study for both arsenic(As)and indium(In)atoms.Under this basis set,the outermost valence electrons 3s23p63d104s24p3for As,4s24p64p105s25p1for In were described and their core electrons were not taken into account.The processes of optimization were based on energy system convergence,and the convergence of energy was better than 10-6a.u.. In order to save time and improve efficicency,the processes of optimization were divided into two steps,the first step was to optimize the structures with a prescribed bond length,bond angle,and dihedral angle of the initial value in the convergence criterion of 10-4a.u.;the second step was to optimize the InAs structure by using the result of the first step in the convergence criterion of 10-6a.u..
Based on the optimized tubelike clusters,the unit cells of the infinite InAsNTs were built by removing some atoms,and the same theory level was employed to optimize the repeated units with the periodic boundary conditions calculations.All these calculations were implemented with the Gaussian 03 program package.31
3 Results and discussion
3.1 Structures of InnAsnclusters
To test the suitability of DFT method and basis sets in our systems,the bond length,the vibrational frequency,and the angle of As―In―As for InnAsn(n=1-3)were calculated and compared with the results of the previous studies.25,26Results show that for the monomer of InAs,the electronic state is3Σ; the bond length is 0.284 nm,and the vibrational frequency is 168 cm-1.These results are consistent with earlier studies at the GGA/DNP(3Σ,0.280 nm,and 171 cm-1,respectively25)and BPW91/DZVP(3Σ,0.279 nm,and 171 cm-1,respectively26)levels.For dimer of In2As2,enough isomers have been generated and the most stable one has D2hrhombus structure,which is also in agreement with the previous results.25,26The bond length of In―As is obtained as 0.295 nm(0.290 nm in literature25) and the angle of As―In―As is 48.5°(48°in literature25).The lowest energy isomer of trimer In3As3presents a face-capped trigonal bipyramid with the Cssymmetry group.The In―As bond length ranges from 0.281 to 0.316 nm,and the result of GGA/DNP study was 0.278-0.309 nm.25All these consistence suggests that the computational scheme we chose will be reasonable for decribing the interatomic bonding and electronic properties of InAs clusters.
As for the structures of InnAsn(n=4,6,8,10,12)clusters, Fig.1 shows that all of these structures share the following common characteristics:(1)all of them are double-ring structures;(2)each As(In)atom has three In(As)neighbors to fully fill a sp3type hybridization bond;(3)all structures consist of two polygons with In-As alternating arrangement.Meanwhile, the two polygons are parallel to each other and there is a relative angle of 2π/n between them.Thus,the numbers of fourmember-rings(4MRs)between the two polygons are found to be n.Specifıcally,for In6As6,the double-ring is composed of two parallel hexagons and six 4MRs,which is similar to the structure of isovalent III-V Ga6As6,32Al6As6,33In6P6,34and Al6P6.35Taking the structures of InnAsn(n=4,6,8,10,12)as the basic framework,we stacked them along the central axis of the double-ring to form finite tubelike clusters.Then five sorts of stable tubular clusters with different sizes of section(from 0.651 to 1.558 nm)have been obtained by full optimization. The growth behaviors of these tubelike clustersʹstructures are shown in Fig.1.Through the figure,one can find that all of these structures contain two parallel polygons and p 4MRs(on the wall of the tube)at the two ends,where p is the number of atoms for the polygon.Furthermore,on the assumption that k is the number of atomic layer in the structure,the quantity of six-membered-ring(6MR),h,on wall of the tube can be inferred by,

Fig.1 Top view of the optimized InnAsnclusters(the first row;n=4,6,8,10,12)and the side view of tubelike clusters Inpk/2Aspk/2 (all the rest;p=4,6,8,10,12;k=2,3,4,…,15)

It means that for the determinate p the longer the tubelike cluster is,the more 6MRs are.Meanwhile,for the determinate k the larger diameter of the tubelike cluster is,the more 6MRs are. Hence,we can conclude that the 6MRs are the building blocks in the construction of the tubelike clusters.According to these results,all the tubelike clusters in Fig.1 can be classified by the common molecular formula,Inpk/2Aspk/2or In(p+k)As(p+k),where p is equal to 4,6,8,10,12 and k ranges from 2 to 15.It is noteworthy that for k>3 all of the structures have point group Spsymmetry when it is even,while the Cph/2symmetry when it is odd.
Even though all the structures abide by some common rules, there are some differences of the structural parameters such as the In―As average length,As―In―As angles,and the tube length for different p or k in the clusters.The mean bond lengths of nearest neighbor In―As(R),bond angle ofAs―In―As(θ), total length of the cluster(L),and the symmetry of these molecules(S)are all listed in Table 1.As is shown in Table 1,the cluster In4As4holds the largest In-As bond length of 0.278 nm and the smallest As―In―As bond angle of 106°,which indicates that the cluster of In4As4is less stable and more reactive than the others.That would be the reason why In4As4can be considered as the structure of evolutionary origination for this system.When the number of layers k≥4 and if it is fixed,the value of R for the tublike cluster with p=4(TLCP4)is larger than the others,and the total length L order is L(p=4)>L(p=6)>L(p=8)>L(p=10)>L(p=12).Hence,one can also conjecture that the TLCP4 are quite unstable compared with TLCP6(p= 6),TLCP8(p=8),TLCP10(p=10),and TLCP12(p=12).Further proof will be presented in the later discussion of binding energies.Moreover,the mean angle of As―In―As in the structure is all about 119°,which is very close to the 120°of sp3.For TLCP12,when k=13,14,15,the mean In―As bond length is equal to 0.263 nm which is very close to the 0.262 nm of the length in InAs crystal.36
3.2 Size dependence of structural stability and electronic properties
Based on the optimized tubelike structures of InnAsnclusters, the average binding energy(Eb)are calculated to measure the relative stability of the clusters as well as the influence of the length and width.Ebis defined as the energy gained in assembling the Inpk/2Aspk/2cluster from its isolated constituent atoms. It can be calculated by,

where Et(In),Et(As),and Et(Inpk/2Aspk/2)represent the total energies of In atom,As atom,and Inpk/2Aspk/2cluster,respectively.
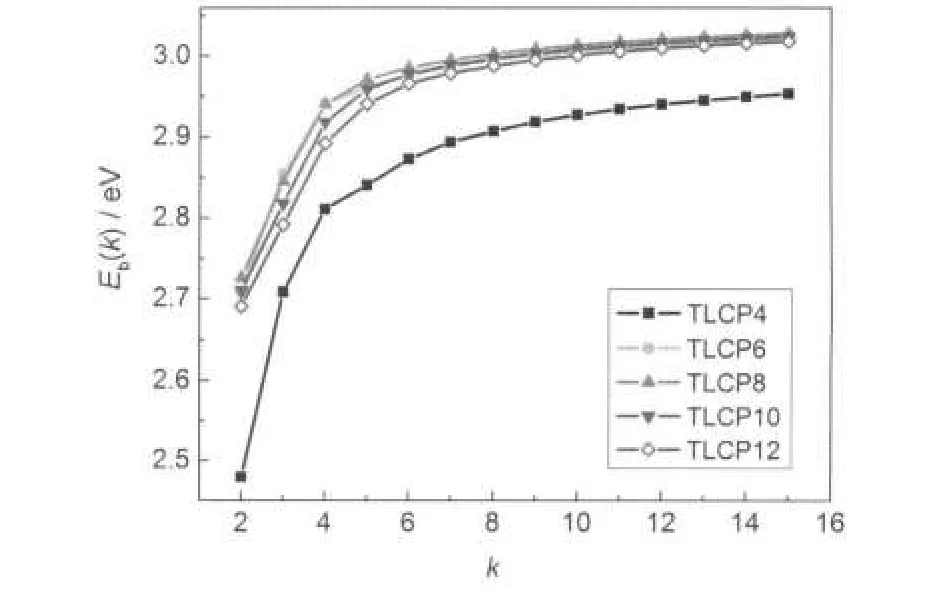
Fig.2 Average binding energy of the clusters InnAsnas a function of size k

Table 1 Structural parameters of InnAsn(4≤n≤90)tubelike clusters
Fig.2 shows the binding energy Eb(k)as a function of size k. As is illustrated in Fig.2,the binding energy of the tubelike cluster increases gradually as the length increases for a certain p.This correlation indicates that the cluster becomes more stable as it becomes longer.However,binding energy of the longest cluster,relative to that of the InAs bulk state(4.487 eV37), is still very small.It is well known that 6MR is the most frequent structural motif in an immense variety of bulk III-V compound materials38.The optimized structures in this paper also possess the same structural motif,and when the tubelike cluster becomes longer the number of 6MRs h will be increased, hence the increasing of h may be beneficial for the tubesʹstability.On the other hand,the stabilities of the tubes do not depend linearly on the tubesʹradii,which are represented by p.For any k,except k=3,the most stable tube is the TLCP8,while the most unstable tube is TLCP4 lagging far behind the others.Furthermore,for all kinds of tubes(with different p),there is a transition of the curves at k=4,where the proportion between p and h is 1:1,i.e.,the number of 4MR is equal to that of 6MR in the structures.When k≤4,the proportion p:h is less than 1,the slope of curve is abrupt.Whereas starting from k=5,the proportion of p:h is beyond 1,the ascending tendency of the curve becomes smooth.This is another evidence of the fact that the content of 6MRs in the structures will affect the stability of the tube.It is worthy to mention that the binding energy of TLCP6, TLCP8,and TLCP10 are almost overlap each other when k>4, which indicates that their stabilities are similar.This similarity could arise from their similar structural parameters,as bond length(about(2.65±1)nm)and As―In―As bond angle(about 119°±1°).
In order to understand the electronic properties of these tubelike clusters the size-dependent HOMO-LUMO energy gaps (Eg),Fermi energy(EF),and some energy levels nearby frontier orbits are calculated and plotted in Fig.3.The Egand EFare respectively de ned as

where the EHOMOrepresents the energy of the highest occupied molecular orbital,and the ELUMOis the energy of the lowest unoccupied molecular orbital.As is seen in Fig.3,when the layer of k increases,the distance between the neighboring energy levels decreases,and the energy levels are gradually approaching the level of EHOMOor ELUMO.In addition,for all sorts of the tubes,there exists an obvious HOMO-LUMO energy gap,and the Fermi energy EFis all around a constant of-5.1 eV.Thereby,the stability of the tubelike clusters may be attributed to the corresponding larger Eband Eg.In Fig.3,one can also find that there are some differences of electronic structure among these various tubes.As is shown in Fig.3(a),with the increase of the length there is a stair-like gradual increase for the energy gaps between HOMO and LUMO,while for all of the other tubes there is also an obvious transition at k=4 resembling their Eb. When k>4,the trends are different,there is a stair-like gradual increase of the Eg,yet as for TLCP8 the curve of gaps almost approaches to a horizontal line with a small oscillation behavior;for both TLCP10 and TLCP12 there is a trend of decrease (when 4≤k≤8)and after k=8 the gaps also tend to be flat with slight oscillation.These differences may remind us to adjust the electronic properties by forming different size cluster.Even though the electronic structures are different to some extent for different kinds of tubes,the large HOMO-LUMO energy gap for all of them ranging from 1.25 to 2.15 eV shows their semiconductor characteristics in common,which is different from the conductive characteristic of the synthesized InAsNTs in experiment.24This fundamental change stems from the quantum size effect,27that is because the largest tubelike cluster in this study have only about 0.2%length of the InAsNTs,and about 2%diameter.
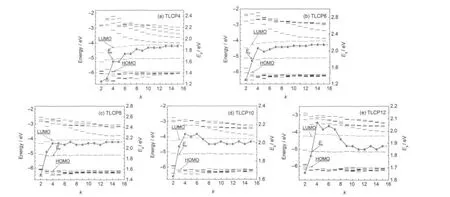
Fig.3 Energy gaps,Fermi energies,and energy levels nearby frontier orbital of InnAsntubelike clustersThe energy level nearby frontier obitals are HOMO-6,…,HOMO-1,HOMO,LUMO,LUMO+1,…,and LUMO+6.
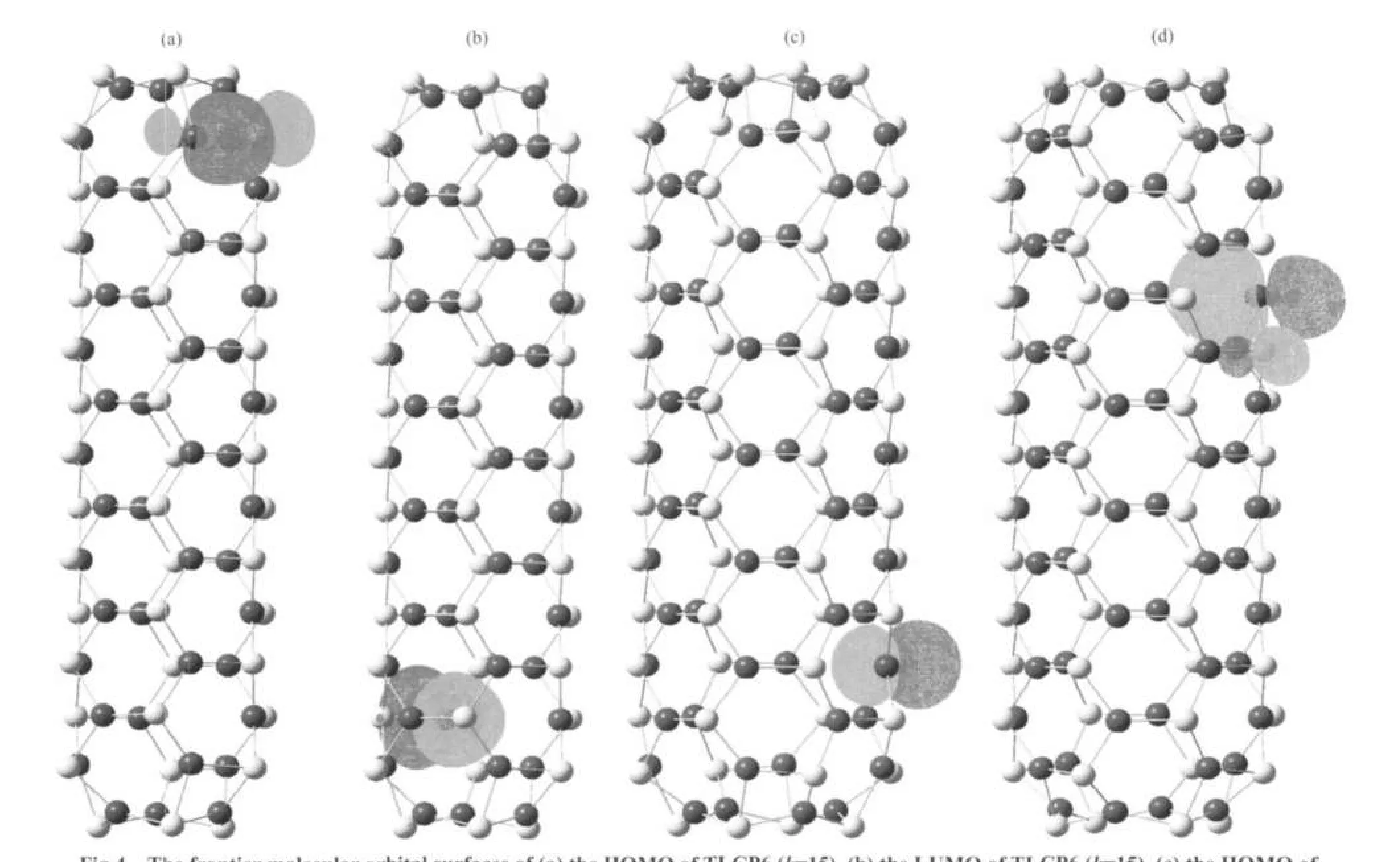
Fig.4 The frontier molecular orbital surfaces of(a)the HOMO of TLCP6(k=15),(b)the LUMO of TLCP6(k=15),(c)the HOMO of TLCP8(k=15),and(d)the LUMO of TLCP8(k=15)
Besides,we have generated the HOMO and LUMO molecular orbital surfaces for all of the tubelike clusters.To give the surfaces of In45As45and In60As60as examples Fig.4 shows that the main components of the HOMO and LUMO comes from 4p-orbital of As atom and 5p-orbital of In atom.For instance, the HOMO of TLCP4 is contributed by the pure p-orbital of In except In6As6,and the LUMO of TLCP4 comes from pure p-orbital of As.In the case of the HOMO of TLCP6,As p-orbital forms a σ-bond with In p-orbital,the LUMO consists of pure p-orbital of In and small p-orbital ofAs.In TLCP8,the p-orbital of As is the main contributor to HOMO,while for the LUMO there is a π-bond comprised of p-orbitals of In and As atom.As for TLCP10 and TLCP12 the results are the same,As p-orbital for HOMO and a π-bond for LUMO.According to these results,it suggests that the poor stability of TLCP4 may come from the absence of a molecular bond between In p-orbital and As p-orbital.For the other kinds of nanotube clusters the similar stability is found(shown in Fig.2),and the relative high stability may come from the π-bond or σ-bond both consisting of p-orbitals of In andAs atom.
Even though there are somewhat differences for both HOMO and LUMO among these various tubes,the characteristics of changes in common can be summarized as follows:with the tubesʹlength increasing,the HOMO and LUMO gradually transfer from the central toward the two terminals of the structure.The electron density distributions of HOMO and LUMO indicate that the chemical activity of the tubelike clusters at the two ends are stronger,which makes the cluster propitious to grow longer.That is why we can successfully attain the long stable tubelike clusters.Moreover,the experiment results of synthesized multi-walled InAs nanotubes show that the nanotubes are highly uniform with well-defined size and shape:the length of 2 μm,inner diameter of 70 nm,and the wall thickness of 10 nm.24Although the tubelike clusters are different in size,all of them belong to one-dimensional nanostructure.It is supposed that these one-dimensional nanostructures have the same microscopic growth mechanism:the chemical activity of the tubes at the two ends are stronger,which makes the system being propitious to grow longer in one dimension.In fact,using the frontier molecular orbital theory to study the growth behavior of clusters is a simple and effective method,which have been developed into an optimum valence bond scheme.39
As for bonding characteristics of the clusters,the Mulliken population analysis40is conducted,and the average on-site charge on In or As atoms are all listed in Table 2.One can see that for a determinate number of k,the charge grows larger with the increasing of p.It means that the charge transfer from In to As is increasing with the broaden tube radius,since the electronegativity of As(2.18)is higher than that of Indium (1.78).As we have discussed,when p is the smallest one the In―As bond length is the largest one for a certain k.Thereby, the bond lengths of the tubelike cluster are affected by the charge distribution on the atoms.Furthermore,the increase of the on-site charge leads to the increase of ionicity of the cluster.When p is fixed the charge begins to increase and then decrease with the increasing numbers of the layer k,approaching the corresponding infinite InAsNTsʹvalue(0.420,0.475,0.503, 0.520,and 0.530).
3.3 Infinite InAsNTs
The increasing stability of the tubelike clusters with an increase of the number of layers k allows us to examine furtherstability of the corresponding infinite nanotubes with a stoichiometric InnAsn.These infinite nanotubes(InAsNT)are built from the homologous tubelike Inpk/2Aspk/2(k=4)clusters with a removal of two parallel polygons at the two ends of the structures to form the corresponding repeated unit.These units, with wire axis lying in the center,can be repeated by translation vector along the axis.The values of the translation vectors are given by 0.433,0.434,0.435,0.437,and 0.438 nm for InAsNTP4(p=4),InAsNTP6(p=6),InAsNTP8(p=8), InAsNTP10(p=10),and InAsNTP12(p=12),respectively. Fig.5 shows the four repeated cells of the infinite tubes with different radii.All of these structures have the 6MR organizational units in common.Full structure relaxation indicates that the infinite InAsNTs have similar geometric structures to finite tubelike clusters,but some structural parameters have slight change.The bond length of In―As and angles of As―In―As with the atoms in two or three neighboring layers are listed in Table 3.Compared with Table 2,one can find that In―As bond lengths are almost the same as the finite tubelike clusters, but the tube lengths are different to a certain extent for the same layers(k=8).The lengths of tubelike clusters gradually decrease with the radii increasing from 1.536 to 1.339 nm, while the lengths of InAsNTs increase from 1.518 to 1.530 nm. In addition,the length of InAsNTP4 becomes 0.018 nm longer than the TLCP4,but for InAsNTP6,InAsNTP8,InAsNTP10, and InAsNTP12,the length becomes 0.008,0.044,0.083,and 0.131 nm shorter.TheAs―In―As angle with the atoms on two neighboring layers is decreasing(from 109°to 112°)with the increase of p,while the angle with the atoms on three neighboring layers is increasing(from 125°to 120°),all of which are approaching the 120°of sp3.

Table 2 Average on-site charges on In orAs atoms in InnAsn(4≤n≤90)clusters
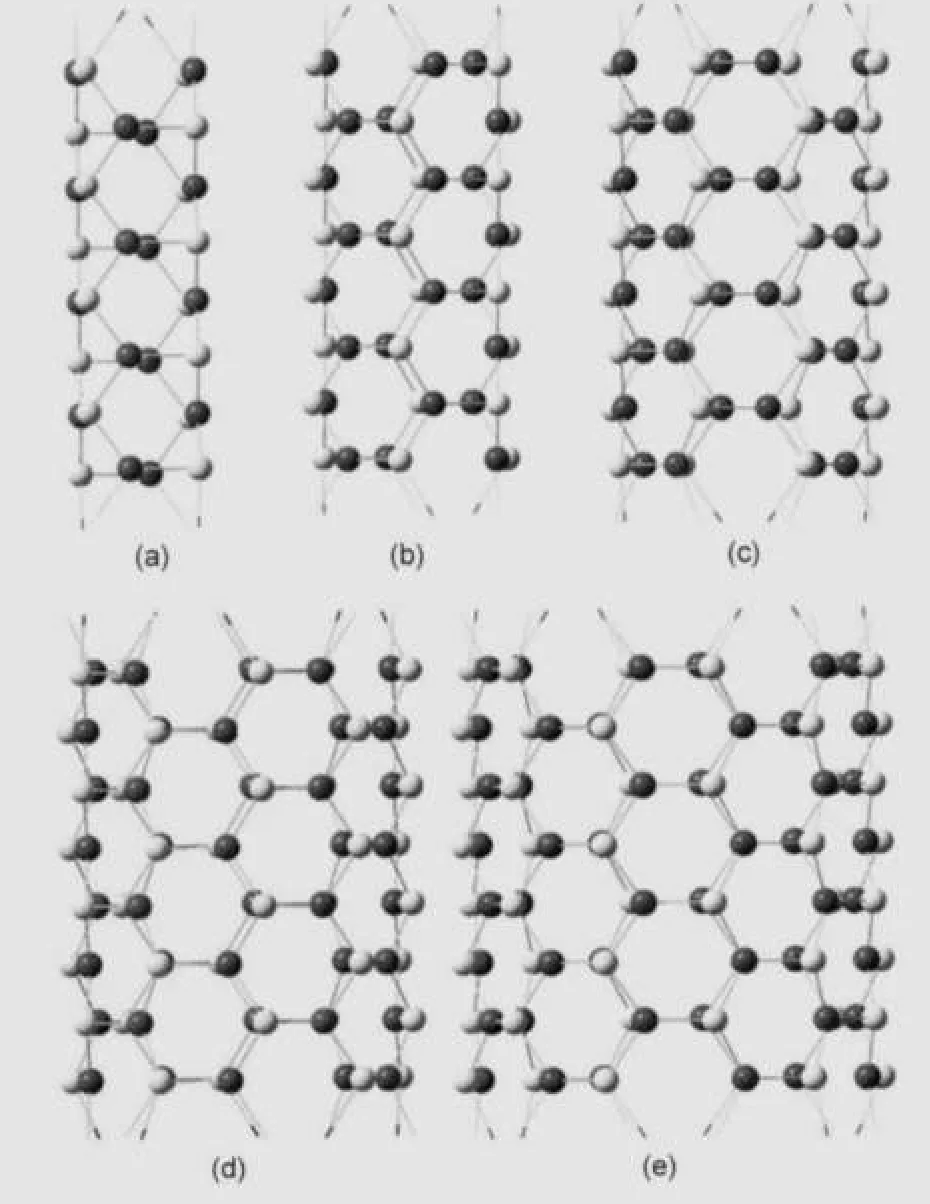
Fig.5 Geometry-optimized structures of the(a)InAsNTP4,(b) InAsNTP6,(c)InAsNTP8,(d)InAsNTP10,(e)and InAsNTP12

Table 3 Structural parameters of InAs nanotubes
The binding energies of the infinite tubes shown in Fig.6 are slightly larger than those of finite tubelike clusters,which means that it is possible to synthesize tubes long enough.It should be mentioned that the binding energies of NTP8 is larger than those of the other tubes with different sizes,while that of NTP4 is smaller,which is similar to the case of tubelike cluster.
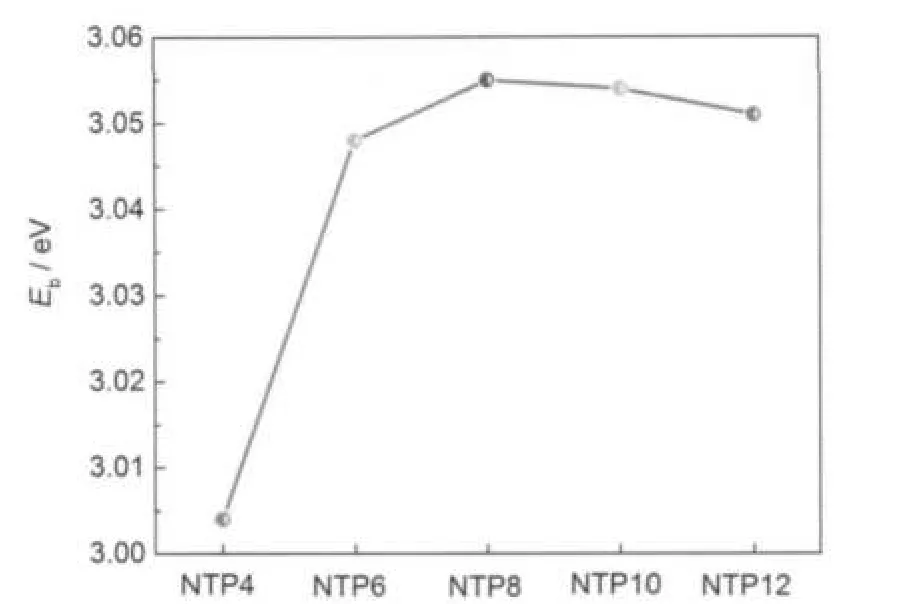
Fig.6 Binding energy of infinite InAsNTs including NTP4, NTP6,NTP8,NTP10,and NTP12

Fig.7 Energy band structures(dispersion along Γ-X direction of the Brillouin zone)of the five sorts of InAsNTs (a)NTP4,(b)NTP6,(c)NTP8,(d)NTP10,(e)NTP12;The Fermi level is set to zero energy and indicated by the horizontal dashed line.
As for the electronic energy band structures of InAs nanotubes,Fig.7 indicates that the five-band structures clearly show the finite gap,the widths of which are 2.052 eV for Fig.7(a), 2.036 eV for Fig.7(b),2.203 eV for Fig.7(c),2.127 eV for Fig.7 (d),and 2.061 eV for Fig.7(e).This implies that these nanotubes are indirect wide gap semiconductor.In fact,all of these gaps are a lot larger than that of InAs bulk state(B3LYP theory value:0.55 eV41or the values of experiment:0.417 eV,42narrow gap semiconductor).But compared with HOMO-LUMO energy gap widths of the tubelike clusters shown in Fig.3,there are not essential changes for the similar structures.These results present a good way to adjust the gap width by forming different nanostructures.In order to further explain that the different structures will have different electrical conductivities,the comparison are made with the hybrid density functional study of armchair SiC single-wall nanotubes.43In the study,singlewalled type 1(4,4)SiC nanotube corresponding to NTP8 have narrower tube diameters(0.702 nm)than NTP8(1.062 nm), and larger band gap(2.889 eV)than NTP8(2.203 eV).
4 Conclusions
This paper reports the results of an investigation of InAs tubelike clusters and infinite InAsNTs.The different properties of the optimized clusters and nanotubes,such as the structures, binding energy,Mulliken charge population,HOMO-LUMO energy gaps,frontier molecular orbital surface,and the energy band structures were analyzed.Based on the results,several points can be summarized as follows,
(1)A family of stable tubelike InnAsnclusters is observed when n≥4.The molecular formula of these clusters can be classified as Inpk/2Aspk/2.During the course of the clustersʹgrowth, the two parallel polygons and p 4MRs should be considered as the skeleton of the structures,and 6MRs should be regarded as the building blocks.
(2)The analysis of binding energies shows that when p=8, the systems are more stable than other sizes for both of tubelike clusters and infinite nanotubes.The finite tubes have large HOMO-LUMO energy gaps.In addition,the electron density distributions of HOMO and LUMO indicate that the chemical activity of the tubelike clusters at the two ends is strong,which makes the clusters being conducive to grow longer.
(3)Infinite InAsNTs have similar geometric structures to the finite tubelike clusters and present wide gap semiconductivity due to their large band gaps.
(1)Neumann,W.Mater.Chem.Phys.2003,81,364.
(2)Dobrowolski,W.;Kossut,J.;Story,T.Handb.Magn.Mater. 2003,15,289.
(3) Kamat,P.V.Chem.Rev.1993,93,267.
(4) Cox,S.D.;Gier,T.E.;Stucky,G.D.;Bierlein,J.J.J.Am. Chem.Soc.1988,110,2986.
(5)Wang,Y.;Herron,N.J.J.Phys.Chem.1988,92,4988.
(6) Cooke,M.III-Vs Rev.2006,19,18.
(7) Jenkins,P.P.;Macinnes,A.N.;Tabibazar,M.;Barron,A.R. Science 1994,263,1751.
(8) Dick,K.A.;Caroff,P.;Bolinssonl,J.;Messing,M.E.; Johansson,J.;Deppert,K.;Wallenberg,L.R.;Samuelson,L. Semicond.Sci.Technol.2010,25,024009.
(9) Cirlin,G.E.;Dubrovskii,V.G.;Samsonenko,Y.B.; Bouravleuv,A.D.;Durose,K.;Proskuryakov,Y.Y.;Mendes, B.;Bowen,L.;Kaliteevski,M.A.;Abram,R.A.;Zeze,D.Phys. Rev.B 2010,82,035302.
(10) Perera,S.;Pemasiri,K.;Fickenscher,M.A.;Jackson,H.E.; Smith,L.M.;Yarrison-Rice,J.;Paiman,S.;Gao,Q.;Tan,H.H.; Jagadish,C.Appl.Phys.Lett.2010,97,023106.
(11) Patriarche,G.;Glas,F.;Tchernycheva,M.;Sartel,C.;Largeau, L.;Harmand,J.C.;Cirlin,G.E.Nano.Lett.2008,8,1638.
(12) Song,B.;Cao,P.L.Phys.Lett.A.2002,300,485.
(13)Gutsev,G.L.;OʹNeal,R.H.;Saha,B.C.;Mochena,M.D.; Johnson,E.;Bauschlicher,C.W.,Jr.J.Phys.Chem.A 2008, 112,10728.
(14) Bai,Q.G.;Song,B.;Hou,J.Y.;He,P.M.Phys.Lett.A 2008, 372,4545.
(15) Goldberger,J.;He,R.;Zhang,Y.;Lee,S.;Choi,H.J.;Yang,P. Nature 2003,422,599.
(16) Xu,Z.;Golberg,D.;Bandoa,Y.Chem.Phys.Lett.2009,480, 110.
(17) Guo,Y.H.;Yan,X.H.;Yang,Y.R.Phys.Lett.A 2009,373,367.
(18) Louail,L.;Maouche,D.;Hachemi,A.Mater.Lett.2006,60, 3269.
(19) Bolshakova,I.;Kost,Y.;Makido,O.;Shurygin,F.J.Cryst. Growth 2008,310,2254.
(20) Wernersson,L.E.;Lind,E.;Lembke,J.;Martinsson,B.;Seifert, W.J.Cryst.Growth 2005,280,81.
(21) Tomioka,K.;Mohan,P.;Noborisaka,J.;Hara,S.;Motohisa,J.; Fukui,T.J.Cryst.Growth 2007,298,644.
(22) Persson,A.I.;Fröberg,L.E.;Samuelson,L.;Linke,H. Nanotechnology 2009,20,225304.
(23) Cirlin,G.E.;Dubrovskii,V.G.;Petrov,V.N.;Polyakov,N. K.;Korneeva,N.P.;Demidov,V.N.;Golubok,O.;Masalov,S. A.;Kurochkin,D.V.;Gorbenko,O.M.;Komyak,N.I.; Ustinov,V.M.;Egorov,A.Y.;Kovsh,A.R.;Maximov,M.V.; Tsatsulʹnikovz,A.F.;Volovikz,B.V.;Zhukov,A.E.;Kopʹev,P. S.;Alferov,Z.I.;Ledentsov,N.N.;Grundmann,M.;Bimberg, D.Semicond.Sci.Technol.1998,13,1262.
(24) Mohan,P.;Motohisa,J.;Fukui,T.Appl.Phys.Lett.2006,88, 013110.
(25) Costales,A.;Kandalam,A.K.;Franco,R.;Pandey,R.J.Phys. Chem.B 2002,106,1940.
(26) Costales,A.;Pandey,R.Chem.Phys.Lett.2002,362,210.
(27) Sarkar,P.;Springborg,M.Phys.Rev.B 2003,68,235409.
(28) Becke,A.D.Phys.Rev.A 1988,38,3098.
(29) Lee,C.;Yang,W.;Parr,R.G.Phys.Rev.B 1988,37,785.
(30) Wadt,W.R.;Hay,P.J.J.Chem.Phys.1985,82,284.
(31) Frisch,M.J.;Trucks,G.W.;Schlegel,H.B.;et al.Gaussian 03, Revision B.03;Gaussian Inc.:Wallingford,CT,2004.
(32) Zhao,J.J.;Hou,X.L.;Xie,R.H.Phys.Rev.B 2006,74, 035319.
(33) Guo,L.Comput.Mater.Sci.2008,42,489.
(34) Wang,L.;Zhao,J.J.J.Mol.Struct.-Theochem 2008,862,133.
(35) Zhao,J.J.;Wang,L.;Jia,J.M.;Chen,X.S.;Zhou,X.L.;Lu, W.Chem.Phys.Lett.2007,433,29.
(36) Phillips,J.C.Bond and Bands in Demiconductors;Academic: New York,1973.
(37) Hellwege,K.H.;Madelung,O.Landolt-Börnstein,New Series, Group III;Springer:Berlin,1982;p 17.
(38) Zhang,S.L.;Zhang,Y.H.;Huang,S.P.;Liu,H.;Tian,H.P. Chem.Phys.Lett.2010,498,172.
(39)Shen,X.Y.;Xu,Y.G.;He,C.L.;Liu,H.T.;Li,J.M.Eur.Phys. J.D 2005,34,109.
(40) Mulliken,R.S.J.Chem.Phys.1955,23,1841.
(41) Tomic,S.;Montanari,B.;Harrisona,N.M.Physica E 2008,40, 2125.
(42)Lacroix,Y.;Tran,C.A.;Watkins,S.P.;Thewalt,M.L.W. J.Appl.Phys.1996,80,6416.
(43)Alam,K.M.;Ray,A.K.Phys.Rev.B 2008,77,035436.
May 16,2011;Revised:July 1,2011;Published on Web:July 7,2011.
Structures,Stabilities and Electronic Properties of InAs Tubelike Clusters and Single-Walled InAs Nanotubes
LIU Zhi-Feng ZHU Heng-Jiang*CHEN Hang LIU Li-Ren
(School of Physics and Electronic Engineering,Xinjiang Normal University,Urumchi 830054,P.R.China)
The geometric structures,stabilities,and electronic properties of InnAsntubelike clusters at up to n=90 and single-walled InAs nanotubes(InAsNTs)were studied by density functional theory(DFT) calculations.The lowest-energy structures and electronic properties of the small InnAsn(n=1-3)clusters are consistent with those found in earlier studies.A family of stable tubelike structures with In-As alternating arrangement was observed when n≥4 and their structural units(four-membered rings and sixmembered rings)obey the general developing formula.The average binding energies of the clusters show that the tubelike cluster with eight atoms in the cross section is the most stable cluster.The sizedependent properties of the frontier molecular orbital surfaces explain why we can successfully obtain long and stable tubelike clusters.They also illustrate the reason why InAsNTs can be synthesized experimentally.We also found that the single-walled InAsNTs can be prepared by the proper assembly of tubelike clusters to form semiconductors with large bandgap.
InnAsntubelike cluster;InAs nanotube;Density functional theory;Geometric structure; Stability; Electronic property
O641
∗Corresponding author.Email:zhj@xjnu.edu.cn;Tel:+86-13999940158;Fax:+86-991-4332557.
The project was supported by the Key Subject of Theoretical Physics of Xinjiang Uygur Autonomous Region,China,Natural Science Foundation of Xinjiang UygurAutonomous Region,China(2010211A21),and Key Project of Xinjiang UygurAutonomous Region Higher Education,China
(xjedu2009i27).
理论物理新疆维吾尔自治区重点学科基金,新疆维吾尔自治区自然科学基金(2010211A21)和新疆维吾尔自治区高校教育重点项目基金
(xjedu2009i27)资助项目
