有限迭代级整系数线性微分方程解的性质

张晓梅,孙道椿

(华南师范大学数学科学学院,广东广州 510631)

有限迭代级整系数线性微分方程解的性质

张晓梅,孙道椿

(华南师范大学数学科学学院,广东广州 510631)

研究了有限迭代级整系数的复线性微分方程,应用Nevalinna和Ahlfors的角域理论,得到了有关解的迭代级,零点迭代收敛指数以及角域中的零点分布的结果.

微分方程;迭代级;迭代收敛指数;角域分布

1 引言及主要结果

本文考虑微分方程

其中A(z)为迭代级有穷的整函数.自1982年以来,当A(z)为多项式或σ(A)＜∞时,人们对方程(1.1)的解的零点分布做了许多研究.而对于一般的情况当σp(A)＜∞(1＜p＜∞,p∈N) 时,其结果甚少.因此作者在本文主要讨论当系数A(z)为迭代级有穷的整函数时,方程(1.1)的解的零点分布.

我们使用Nevanlinna理论以及复方程理论中的标准记号和基本内容.为了叙述结果,还需要以下定义和记号.

定义1[1]整函数f(z)的迭代级σp(f)定义为

2 主要引理

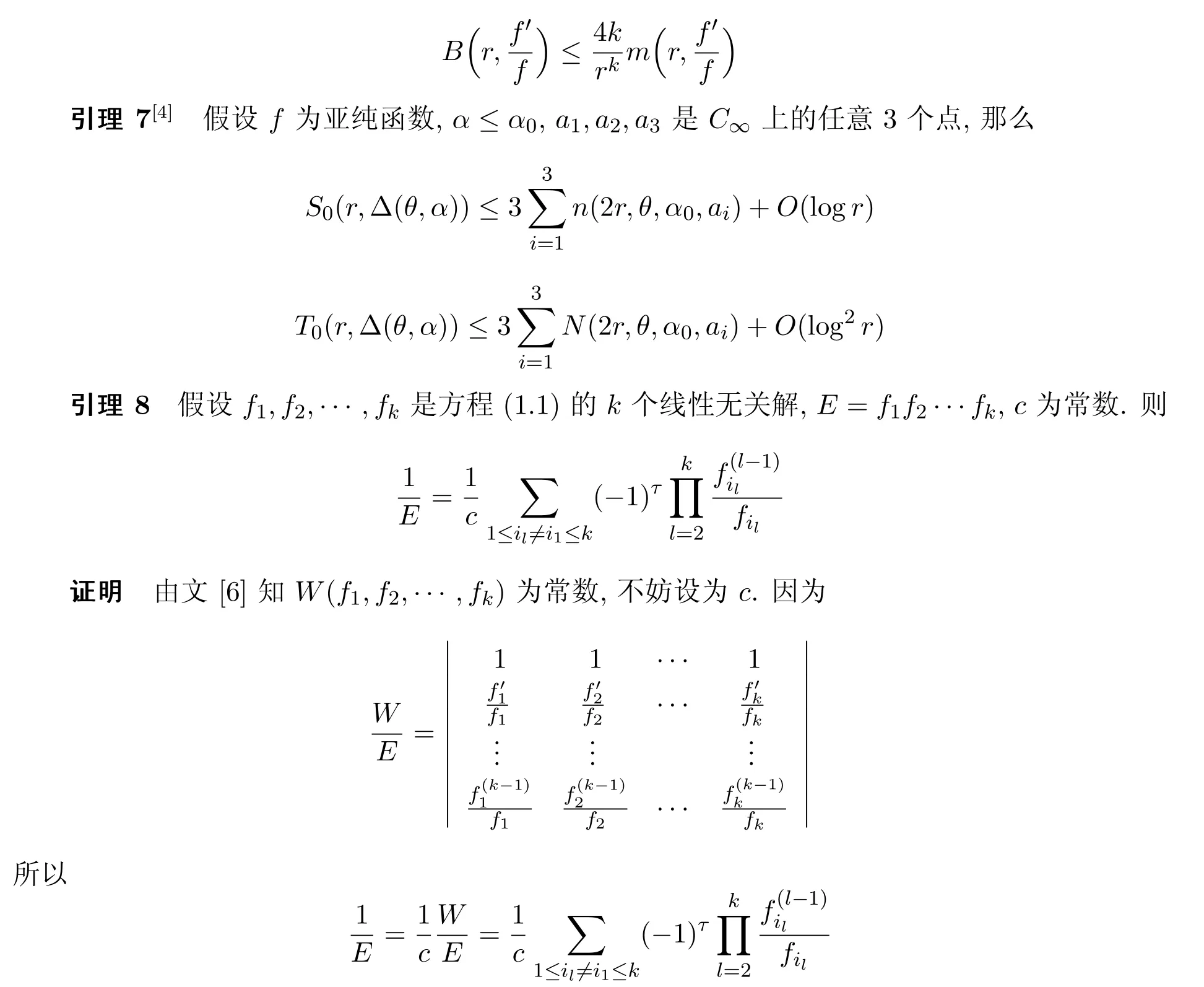
3 定理的证明

定理1的证明根据引理1,有

[1]K innunen L.Linear differential equations with solutions of finite iterated order[J].Southeast Asian Bu ll. M ath.,1998,22:385-405.

[2]Wu S J.On the location of zeros of solution of f''+A f=0 where A(z)is entire[J].Math.Scand,1994, 74:293-312.

[3]Goldberg A A,Ostrovskii IV.The Distribution of Value of Meromorphic Functions[M].Moscow:Izdat Nauk press,1970(in Russian).

[4]Tsu jiM.Potential Theory in Modern Function Theory[M].Tokyo:M aruzen p ress,1959.

[5]Haym an W K.M eorm orphic Functions[M].Oxford:C larendon Press,1964.

[6]Lain I.Nevanlinna Theory and Com p lex Differential Equations[M].New York:Walter de Gruyter,1993.

[7]梁建军,刘名生.高阶亚纯系数非齐次线性微分方程的复震荡[J].纯粹数学与应用数学,2006,22(4):464-470.

The properties of solu tions of linear differen tial equations with entire coefficients of finite iterated order

ZHANG Xiao-mei,SUN Dao-chun

(School of Mathem atical Sciences,South China Norm al University,Guangzhou 510631,China)

The com p lex linear differential equations with entire coefficients of finite iterated order are investigated.By app lying the theory in an angular domain of Nevalinna and Ahlfors,some results concerning the iterated order of solutions,the iterated convergence exponent of the zeros of solutions and the zeros distribution of solutions in an angular domain are given.

differential equation,iterated order,iterated convergence exponent,angular distribution

O174.52

A

1008-5513(2009)02-0237-07

2007-09-25.

国家自然科学基金(10471048),广东省自然科学基金(04010474).

张晓梅(1981-),博士,研究方向：函数论.

2000M SC:30D 35