Simulation of ion cyclotron wave heating in the EXL-50U spherical tokamak based on dispersion relations
2024-03-19HaojieMA马好杰HuashengXIE谢华生andBoLI李博
Haojie MA (马好杰),Huasheng XIE (谢华生) and Bo LI (李博),*
1 School of Physics,Beihang University,Beijing 100191,People’s Republic of China
2 Hebei Key Laboratory of Compact Fusion,Langfang 065001,People’s Republic of China
3 ENN Science and Technology Development Co.Ltd.,Langfang 065001,People’s Republic of China
Abstract This study investigates the single-pass absorption (SPA) of ion cyclotron range of frequency(ICRF) heating in hydrogen plasma of the EXL-50U spherical tokamak,which is an upgraded EXL-50 device with a central solenoid and a stronger magnetic field.The reliability of the kinetic dispersion equation is confirmed by the one-dimensional full-wave code,and the applicability of Porkolab's simplified theoretical SPA model is discussed based on the kinetic dispersion equation.Simulations are conducted to investigate the heating effects of the fundamental and second harmonic frequencies.The results indicate that with the design parameters of the EXL-50U device,the SPA for second harmonic heating is 63%,while the SPA for fundamental heating is 13%.Additionally,the optimal injection frequencies are 23 MHz at 0.9 T and 31 MHz at 1.2 T.The wave vector of the antenna parallel to the magnetic field,with a value of k‖=7.5 m-1,falls within the optimal heating region.Simulations reveal that the ICRF heating system can play an important role in the ion heating of the EXL-50U.
Keywords: ion cyclotron range of frequency,single-pass absorption,spherical tokamak
1.Introduction
The spherical tokamak is a promising candidate for achieving controlled nuclear fusion with high beta [1-3].The EXL-50U is an upgraded device of the EXL-50 spherical tokamak [4-6],having a central solenoid and a stronger magnetic field,that was designed to enhance the plasma performance.The primary objectives of the EXL-50U device are to achieve ion temperatures between 2 and 5 keV and to ensure that the ion temperature in the plasma core exceeds the electron temperature,with a target ratio ofTi/Te~1.5 [6].
Auxiliary heating power is crucial,especially for spherical tokamaks,which have limited internal space and which results in a small volt-seconds value for the central solenoid[7].Ion cyclotron range of frequency (ICRF) heating is the only auxiliary heating method that directly heats ions [8-13],making it a critical component in achieving high ion temperatures.Single-pass absorption (SPA) analysis provides a convenient method to quickly understand heating effects,and provides the total absorption proportions,which are not available with a full-wave code [14-17].The fundamental theory of ICRF fast wave heating in toroidal plasma is extended in [14,15],where Porkolab’s simplified theoretical SPA model is obtained based on the local approximation of the real and imaginary parts of the wave dispersion relation.Additionally,the absorption of ICRF fast waves with moderate to high ion cyclotron harmonics on DIII-D [16]and the ICRF heating effect of the HL-2M device were investigated using Porkolab’s SPA model [17].The SPA was calculated using the method and equations in [14] and used to evaluate ICRF core wave absorption in SPARC [18,19].Recently,the BON code for calculating the dispersion equation has been developed and initially used to analyze the accessibility of ion cyclotron waves [20].The dispersion relation is also widely used in the analysis of ICRF heating[8,21-24].
Similar to other tokamaks [1-3],the plasma pre-heating scheme for the EXL-50U experiment involves using ohmic heating of the central solenoid to generate the initial plasma,followed by primarily utilizing a 2 MW neutral beam injection heating system.Additionally,ICRF heating will be attempted to achieve the main parameters [6].The 3-26 MHz ICRF system with a power of 100 kW was initially tested in the EXL-50 experiment,and is scheduled to be installed in the EXL-50U.Additionally,a 2 MW ICRF system with 10-40 MHz is being designed.In this work,the kinetic dispersion relations and the SPA at the mid-plane are obtained using the BON code [20].The on-axis heating SPAs of hydrogen plasma at fundamental and second harmonic frequencies in EXL-50U are calculated to evaluate the heating effect of ICRF.Moreover,optimalk‖and wave frequencies are provided for the experiments.The applicability of Porkolab’s simplified theoretical SPA model is discussed in comparison with the results obtained from the BON code.Furthermore,the absorption partitions of each species are shown using the SEMAL code,which solves the linearized Vlasov-Maxwell wave equation for all orders on the Larmor radius [25-28].
This paper is organized as follows.The physical model is introduced in section 2.The heating schemes at a magnetic field of 0.9 T are presented in section 3,while the heating schemes at a magnetic field of 1.2 T are discussed in section 4.A summary and discussion of the results are presented in section 5.
2.Physical model description
2.1.Introduction of BON code
The BON code is used for calculating the dispersion relations with the cold,warm multi-fluid and kinetic model [20].The kinetic dispersion relation,which is based on the Maxwell distribution,is utilized in this study to calculate the SPA heating effects of the ICRF,with
Here,Zis the dispersion function and the subscriptsindicates the species,Γn(b)=In(b)e-b,where ωpsand ωcsare the plasma frequencies and cyclotron frequencies of species.Inis the modified Bessel function.Lzz=1 and the other term is 0 in the tensorL.In equations (1)-(3),the plasma parameters are included in ωpsand ωcs.Given the plasma parameters,k‖and the initialk⊥,thek⊥and SPA are obtained by solving the equation (1) in BON code using a Newton iteration method,where the subscripts ‖ and ⊥ denote parallel and perpendicular to the magnetic field,respectively.
2.2.The parameters and profiles of the EXL-50U device
As illustrated in table 1,the two main sets of plasma parameters for the EXL-50U are provided by the tokamak system code [6,29].For the SPA in this work,the magnetic field at the mid-plane is defined as
whereB0is the magnetic field at the major radiusR0.Indeed,according to the two-dimensional Grad-Shafranov equation calculated in [6],the correction to equation (4) at the mid-plane due to the poloidal magnetic field is negligible.The temperature and density profiles of electrons and hydrogen ions are of the following type:
whereT0andn0are the temperature and density atR0,respectively,xedgeis the coordinate of the edge of the profiles,x+R0is the radial coordinate,andsis the stepvalue of the profile atx=xedge.Figure 1 illustrates the temperature and density profiles obtained from equations (5)and (6),using parameters similar to those of EXL-50U (a) in table 1,wheres=0.95 andxedge=0.53 are fixed.

Table 1.Main parameters of EXL-50U device.
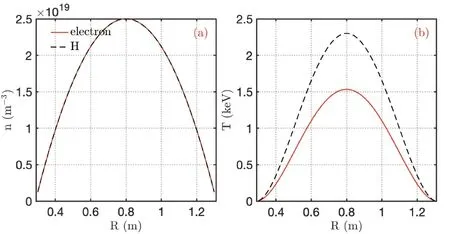
Figure 1.(a) 1D profiles of electrons and H ion densities with n0=2.5×1019.(b) 1D profiles of electrons and H ion temperature with Ti0=2.3×103 eV and Te0=1.53×103 eV.
3.Heating schemes at a magnetic field of 0.9 T
The two ICRF systems are used in the EXL-50U discharge operation within the frequency range of 3-40 MHz,and the initial plasma consists of pure hydrogen.The on-axis heating frequencies for the fundamental and second harmonic frequencies of hydrogen are 13.7 MHz and 27.4 MHz,respectively.Subsequently,the second harmonic on-axis heating scheme is described in section 3.1,and the fundamental frequency on-axis heating scheme is presented in section 3.2.Finally,the optimal wave frequency is discussed in section 3.3.
3.1.On-axis heating scheme for second harmonic frequency
As presented in [14,17],the SPA of Porkolab’s simplified theoretical model is calculated by 1-exp(-2η) ,where η is the tunneling factor that indicates the transferred wave power.In the case of hydrogen plasma in EXL-50U,the tunneling factor for the second harmonic heating is given by the following form [14,17]:
where ωH,ω0,k‖,candRare the plasma frequency of hydrogen,the wave frequency,the parallel wave number of the antenna,the speed of light and the major radius,respectively.The beta for hydrogen ions is given by,wherenHandTHare the density and temperature of hydrogen,respectively,andB0denotes the magnetic field.Additionally,vtHrepresents the thermal speed of H ions.
Figure 2 indicates the SPA effect for second harmonic heating,which is calculated using equations (7) and (8).As illustrated in figure 2(a),the efficiency of plasma core heating increases with the higher temperature and largerk‖of the antenna.Additionally,the pentagram in figure 2(b) indicates that the SPA efficiency for the EXL-50U design parameters is below 30% with the current system operating atk‖=7.5 m-1.
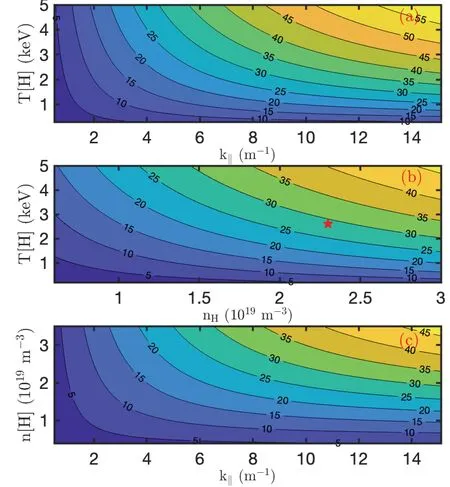
Figure 2.SPA for H second harmonic heating obtained by Porkolab's simplified theoretical model.(a) SPA versus k‖ and ion temperature,where nH=2.5×1019 m-3.(b) SPA versus density and ion temperature,where k‖=7.5 m-1.The pentagram is the SPA within the design parameters.(c) SPA versus k‖ and density,where T= 2.0 keV.
The imaginary part of the wave vector perpendicular to the magnetic field (ki⊥) is obtained using the BON code [20],and the SPA is obtained using[30,31].The kinetic dispersion equation and the applicability of the BON code are discussed in Appendix A.Figure 3 presents the kinetic dispersion relations obtained using the BON code,along with the SPA of the wave.These results are obtained with the typical EXL-50U parameters,specificallyTi0=2.3 keV,Te0=1.53 keV andn0=2.5×1019m-3.As shown in figure 3(b),in the on-axis heating scheme at the second harmonic,although the fundamental and third harmonic resonance layers are present in the plasma region,the energy deposition is minimal.The main energy deposition occurs in the vicinity of the second harmonic resonance layer.Furthermore,as the plasma temperature increases,the energy deposition region widens,which can be attributed to the enhanced effect of the Doppler shift with ω-k‖v‖-nωci=0 [32],wherenis the number of harmonics of the wave.
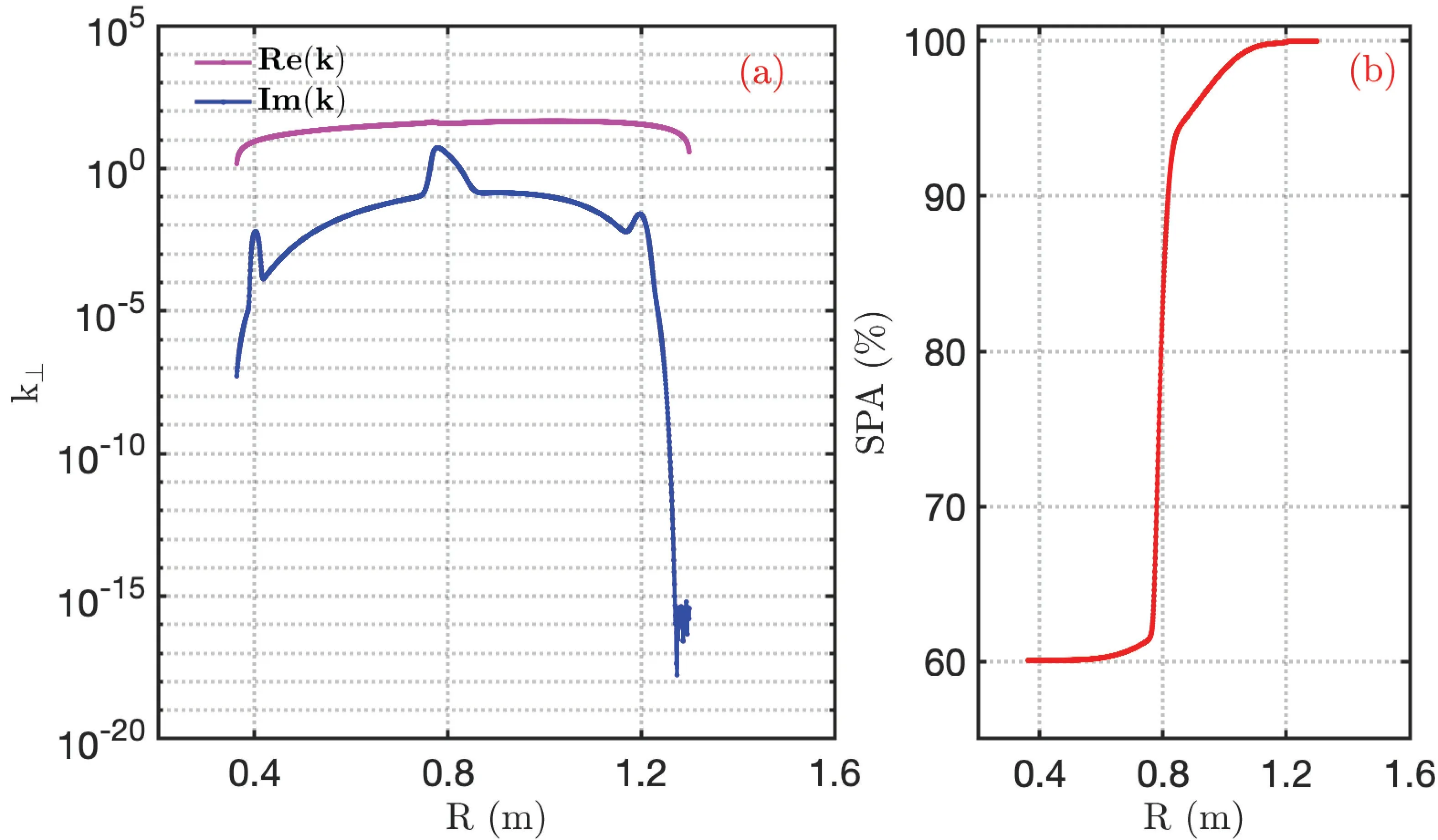
Figure 3.(a) Numerical solution of k⊥ for the kinetic plasma dispersion equation with a fixed k‖=7.5 m-1 from the BON code.(b) The corresponding SPA heating efficiency.
The absorption proportions of electrons and hydrogen ions as a function of the radius are also calculated using the SEMAL code for ICRF propagation from the low-field region,as shown in figure 4.It is found that the absorption of H ions occurs only near the magnetic axis.Although the absorption of electrons is present in a wide region,the main absorption occurs within a distance of 0.2 m from the magnetic axis.The results obtained from the SEMAL code provide further evidence of the reliability of the BON results.
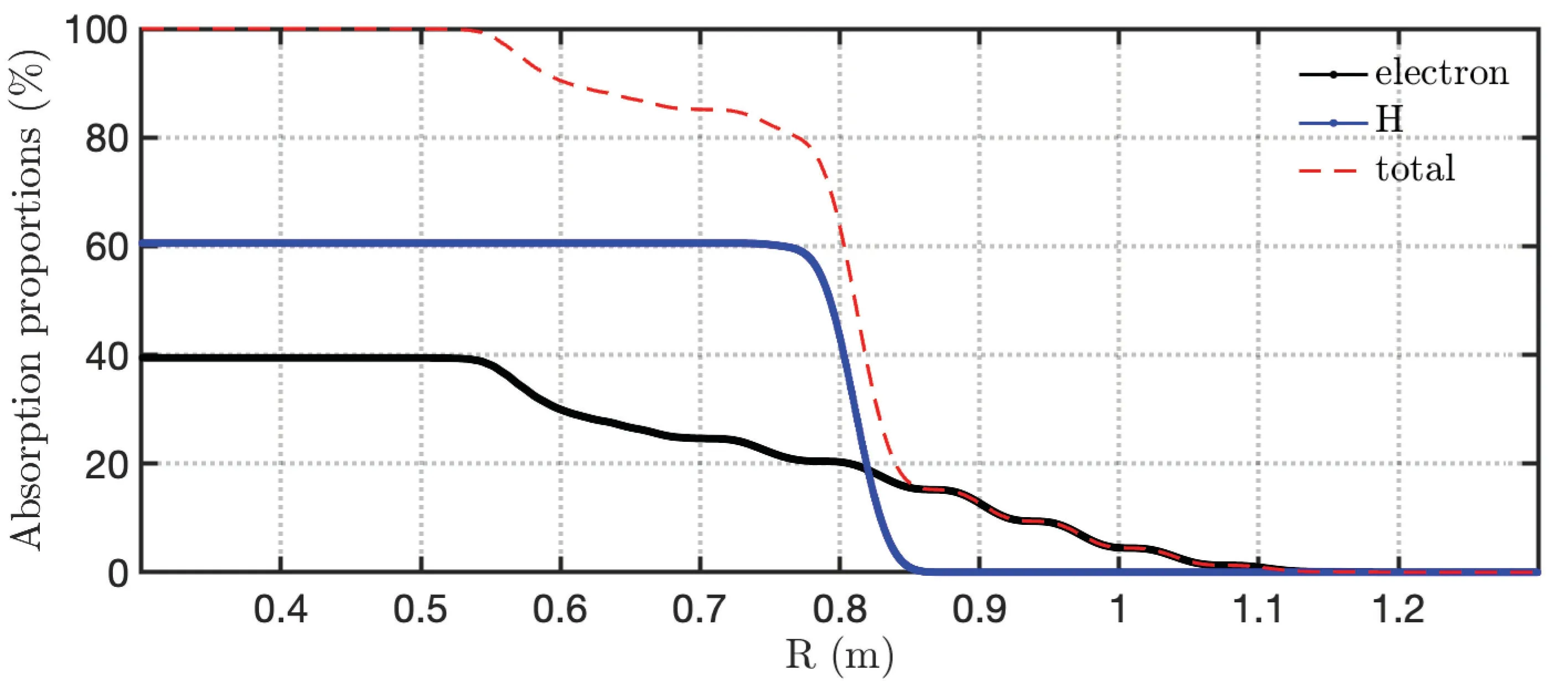
Figure 4.The absorption proportions of electrons and H ions obtained from the SEMAL code,where the parameters are set the same as in figure 3.
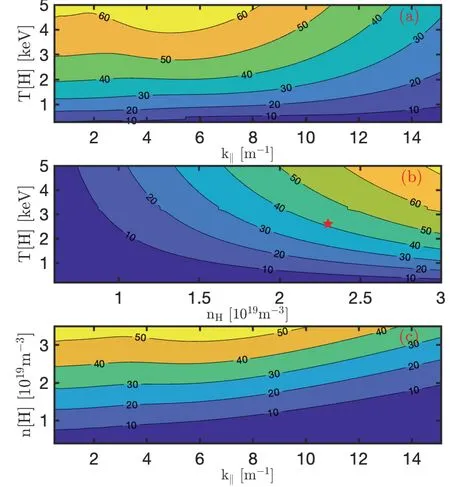
Figure 5.SPA for H second harmonic heating obtained by the BON code.(a) SPA versus k‖ and ion temperature,where nH=2.5×1019 m-3.(b) SPA versus density and ion temperature,where k‖=7.5 m-1 .(c) SPA versus k‖ and density,where T =2.0 keV.
Figure 5 illustrates the SPA effect of the second harmonic heating withk‖,density and temperature at the magnetic axis,calculated using the BON code.The region considered isR-R0=[-0.2,0.2] m,andTe=Tiis assumed.Results from figures 5(a) and (c) suggest that a better heating performance is achieved with a smallerk‖for lower temperature and density.Furthermore,an optimal range ofk‖exists as the temperature or density increases,which is consistent with the findings reported in [18,19].Additionally,k‖=7.5 m-1for the antenna is in the optimal region.As indicated by the pentagram in figure 5(b),the SPA for the EXL-50U design parameters is 40%.The heating effect of the second harmonic over the plasma region has also been obtained and is similar to the results near the magnetic axis,as presented in Appendix B..It is found that Porkolab’s simplified theoretical SPA model is different from the ones obtained from the BON code when comparing figures 2 and 5.The results of the BON code are obtained by accurately solving the full kinetic dispersion relation,whereas Porkolab's theoretical formulation is derived from some simplified assumptions.The differences between the two approaches may stem from these disparities.For instance,the theoretical model only calculates the wave polarization and SPA at the resonance layer.The details of these differences are beyond the scope of this study and could be addressed in future research.
3.2.On-axis heating scheme for the fundamental frequency
Although the heating effect of the fundamental frequency in hydrogen plasma is poor due to the zero wave polarizationE+[12,17] at the resonance layer,the heating effect of H ions at the fundamental frequency has been obtained using the BON code.Figure 6 presents the SPA heating effect near the magnetic axis for H fundamental frequency on-axis heating,calculated using the BON code withTe=Ti.The region considered in figure 6 is the same as in figure 5,i.e.R-R0=[-0.2,0.2]m.It is found that in the on-axis heating scheme with the fundamental frequency,the SPA is less than 10%,which is significantly lower than the SPA obtained for second harmonic heating.
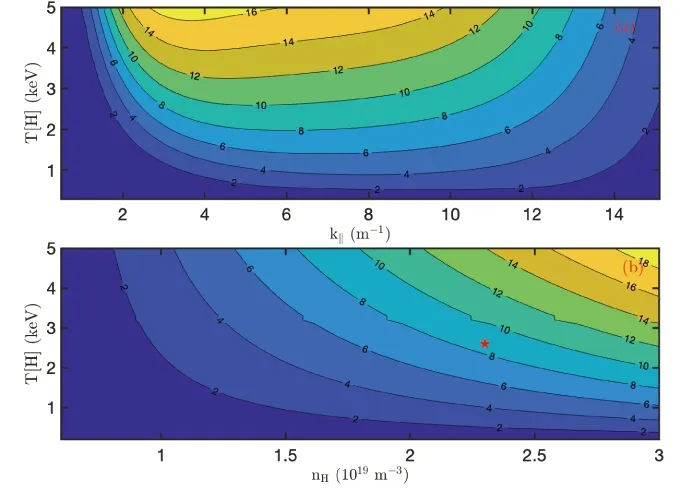
Figure 6.SPA for H fundamental frequency heating obtained by the BON code.(a) SPA versus k‖ and ion temperature,where nH=2.5×1019 m-3.(b) SPA versus density and ion temperature,where k‖=7.5 m-1.
3.3.Optimal frequency for ICRF injection
Figure 7 presents the heating effect of the SPA,with frequencies based on the target parameters of EXL-50U (a)in table 1.The absorption of H ions and electrons is obtained by setting the electron and H ion temperatures to 0.1 eV,respectively.It is found that the heating efficiency of SPA is significantly enhanced when the second harmonic resonance layer is present in the plasma,i.e.f>17 MHz.As the frequency increases,the second harmonic resonance layer moves closer to the magnetic axis from the boundary,and the heating efficiency of SPA increases.Furthermore,the best heating effect is achieved around the on-axis heating frequency.From figures 2-7,it is found that the heating efficiency of ICRF is less than 50% in the discharge mode of EXL-50U (a) in table 1.
Figure 8 presents the absorption proportions of electrons and H ions obtained using the SEMAL code for wave frequencies ranging from 10 to 60 MHz.Consistent with the findings presented in figure 7,the proportion of H ion absorption undergoes a significant increase atf>17 MHz.Furthermore,the proportion of ion absorption surpasses that of electron absorption at 18.5 <f< 32.5 MHz,with the secondary cyclotron resonance layer moving from the lowfield side across the magnetic axis to the strong-field side.The absorption of ions is dominant again at 34.5 <f<38.5 MHz due to the third cyclotron resonance layer in the vicinity of the magnetic axis.

Figure 7.The SPA heating efficiency versus the wave frequencies,where k‖=7.5 m-1.
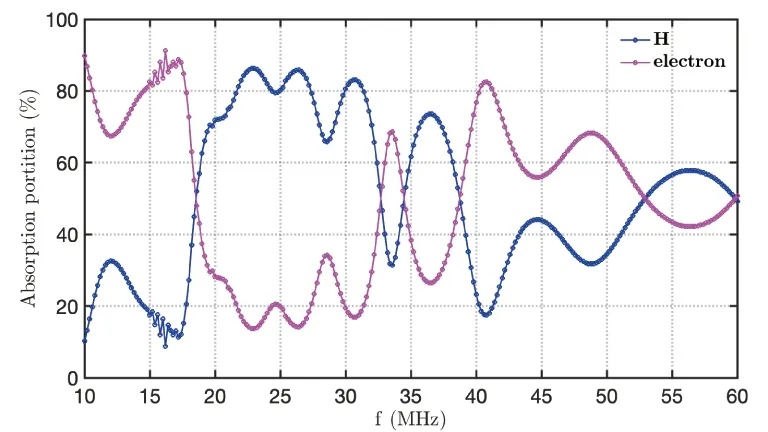
Figure 8.The absorption proportions of electrons and H ions with wave frequency obtained from the SEMAL code,with parameters identical to those used in figure 7.
4.Heating schemes at a magnetic field of 1.2 T
4.1.On-axis heating scheme for second harmonic frequency
The on-axis heating frequencies for the fundamental and second harmonic frequencies of H ions are 18.3 MHz and 36.6 MHz,respectively.Figure 9 presents the SPA effect obtained using equations (7) and (8) at 1.2 T for the second harmonic frequency.Comparison of figure 9 with figure 2 indicates that the SPA heating effect is approximately the same for both discharge modes under the second harmonic heating scheme for H ions.Figure 10 shows the SPA obtained using the BON code for H second harmonic on-axis heating with the region considered asR-R0=[-0.15,0.15]m andTi=Teassumed.The SPA for the EXL-50U design parameters is more than 60%,as indicated by the pentagram in figure 10(b),which is significantly different from the SPA results predicted by Porkolab’s simplified theoretical model.Additionally,thek‖of the antenna is in the optimal range,which differs from the SPA results obtained theoretically in figure 9.Moreover,the heating effect of the second harmonic obtained from equations (7) and (8) is lower than that obtained from the kinetic dispersion relation.
4.2.On-axis heating scheme for the fundamental frequency
In the fundamental on-axis heating scheme,the second harmonic resonance layer is located outside the plasma.Figure 11 illustrates the SPA obtained for H fundamental onaxis heating with the region considered asR-R0=[-0.15,0.15] m andTi=Teassumed.The SPA of the EXL-50U design parameters is less than 14%,andk‖=7.5 m-1is within the optimal heating region.
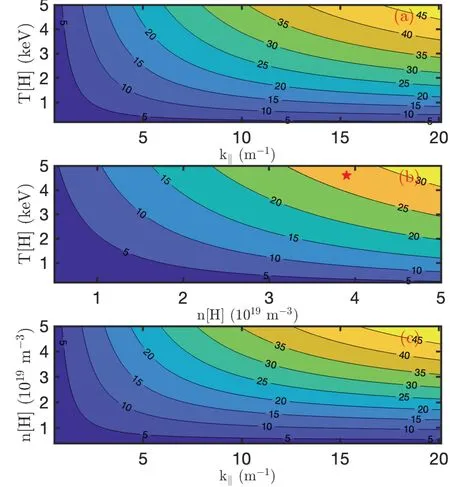
Figure 9.SPA for H second harmonic heating obtained by Porkolab’s simplified theoretical model.(a) SPA versus k‖ and ion temperature,where nH=4.0×1019 m-3.(b) SPA versus density and ion temperature,where k‖=7.5 m-1 .(c) SPA versus k‖ and density,where T =4.0 keV.

Figure 10.SPA for H second harmonic heating obtained by the BON code.(a) SPA versus k‖ and ion temperature,where nH=4.0×1019 m-3.(b) SPA versus density and ion temperature,where k‖=7.5 m-1 .(c) SPA versus k‖ and density,where T =4.0keV.

Figure 11.SPA for H fundamental frequency heating obtained by the BON code.(a) SPA versus k‖ and ion temperature,where nH=4.0×1019 m-3.(b) SPA versus density and ion temperature,where k‖=7.5 m-1.
4.3.Optimal frequency for ICRF injection
Figure 12 presents the heating effect of SPA with frequencies calculated based on the target parameters ofTi0=4.5 keV,Te0=3 keV ,andne0=4×1019m-3.It is found that the heating efficiency of SPA is significantly enhanced when the second harmonic resonance layer is present in the plasma,i.e.f>24.5 MHz.The optimal frequency is at 31 MHz,at which the resonance layer is located in the low-field region near the magnetic axis.There is a wide frequency range where SPA is greater than 50%,which is important for engineering debugging and the effectiveness of ICRF.

Figure 12.SPA heating efficiency versus wave frequencies,where k‖=7.5 m-1.
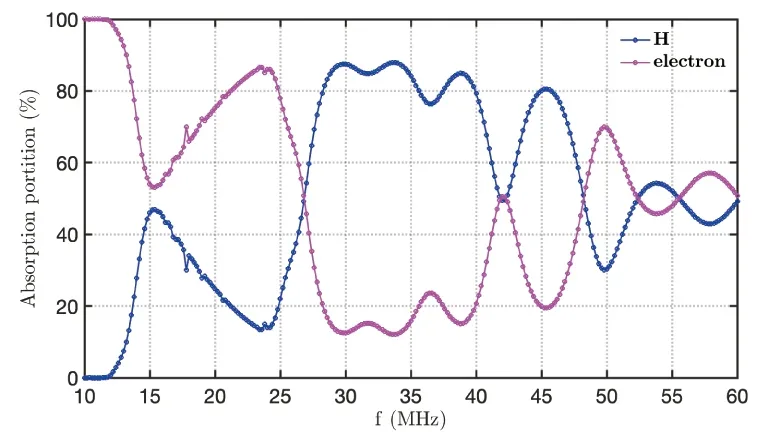
Figure 13.The absorption proportions of electrons and H ions with wave frequency obtained from the SEMAL code,with parameters identical to those used in figure 12.
Figure 13 displays the absorption proportions of electrons and H ions in the range of 10-60 MHz.It is found that the absorption of H ions exceeds 50% at wave frequencies of 27-48 MHz.The results of a rapid increase in the proportion of H ion absorption atf>24.5 MHz,along with the proportion of H ion absorption surpassing electron absorption atf>27 MHz,are consistent with the findings presented in figure 12.
5.Summary
The SPA of ICRF heating in hydrogen plasma of the EXL-50U is investigated in this work using the kinetic dispersion equation.A comparison between Porkolab’s simplified theoretical SPA model and the kinetic dispersion relation reveals that the former yields smaller values.Furthermore,an optimal region ofk‖is identified during core heating,indicating that largerk‖values are not necessarily better.The SPA heating simulations for the fundamental and second harmonic frequencies of EXL-50U show that the antenna’sk‖=7.5 m-1is within the optimal heating region,and the optimal frequency ranges are 18.5-32.5 MHz at 0.9 T and 27-48 MHz at 1.2 T.These results provide a useful reference for debugging and experiments of the ICRF system.The absorption proportions of electrons and H ions are also provided using the SEMAL code,indicating that the ICRF heating system can play a significant role in ion heating of the EXL-50U.This study focused on the fundamental and second harmonic frequency heating of hydrogen plasma.It is important to note that the reabsorption of the remaining unabsorbed power from the ICRF at wall reflections is not taken into account.Moreover,the presence of unabsorbed ICRF power can lead to sputtering and impurity production from the wall,introducing new ions into the plasma.In addition,the dispersion relation and SPA heating simulations are only applicable for a convenient and preliminary assessment of the heating effect of the ICRF.Therefore,it is necessary to investigate the ICRF heating effect of multiple ions and perform two-dimensional full-wave simulations to gain a more comprehensive understanding of the ICRF heating performance in EXL-50U.
Acknowledgments
This work was supported by the National Magnetic Confinement Fusion Energy Program of China (No.2018 YFE0311300),the High-End Talents Program of Hebei Province,Innovative Approaches Towards Development of Carbon-Free Clean Fusion Energy (No.2021HBQZYCSB 006) and the Compact Fusion Project of the ENN Group.
Appendix A.The accuracy of BON code
The SPAs of the ray-tracing code and the BON code for ICRF heating at the mid-plane of the device are compared based on the same parameters.The BORAY code is a tool used for RF wave propagation and energy deposition,and it is also capable of performing ICRF propagation and heating simulations [30,31].In this study,the BORAY code is used to calculate the wave trajectory and energy deposition of ICRF at 27.4 MHz (i.e.second harmonic frequencies of H ion) near the mid-plane with an initial position set atR=1.25 m andZ=0 m,as well ask‖=8 m-1.The results are presented in figure A1,although the local approximation assumption for dispersion equation and ray-tracing may not be satisfied due to the comparable wavelengths of the ICRF to the device.The dispersion relation and SPA are calculated using the BON code with the same settings as in figure A1.This results indicates that the SPA calculated by the BON code at the mid-plane is in agreement with that by the BORAY code,as shown in figure A2.
Appendix B.SPA for the full space
The SPA heating effect of ICRF fast waves was calculated throughout the plasma region using the same parameters as in figure B1,as shown in figure 5.Compared to figure 5,it is found that the SPAs calculated near the magnetic axis and throughout the plasma region show little difference in the multi-harmonics heating of hydrogen plasma.

Figure A2.(a) Numerical solution of k⊥ for the hot plasma dispersion equation using the same settings as in figure A1 of the BON code.(b) The corresponding SPA heating efficiency.

Figure B1.SPA for H second harmonic heating obtained by the BON code throughout the entire plasma region.(a) SPA versus k‖and ion temperature,where nH=2.5×1019 m-3.(b) SPA versus density and ion temperature,where k‖=7.5 m-1 .(c) SPA versus k‖and density,where T =2.0 keV.
猜你喜欢
杂志排行
Plasma Science and Technology的其它文章
- Detection of Al,Mg,Ca,and Zn in copper slag by LIBS combined with calibration curve and PLSR methods
- Experimental study on the effect of H2O and O2 on the degradation of SF6 by pulsed dielectric barrier discharge
- Phase field model for electric-thermal coupled discharge breakdown of polyimide nanocomposites under high frequency electrical stress
- Non-thermal atmospheric-pressure positive pulsating corona discharge in degradation of textile dye Reactive Blue 19 enhanced by Bi2O3 catalyst
- Airfoil friction drag reduction based on grid-type and super-dense array plasma actuators
- Characteristics of laser-induced breakdown spectroscopy of liquid slag
