Non-static plane symmetric perfect fluid solutions and Killing symmetries in f(R,T)gravity

Preeti Dalal,Karanjeet Singh and Sachin Kumar,*

1 Department of Mathematics and Statistics,Central University of Punjab,Bathinda,151401,Punjab,India

2 Department of Mathematics,Jaypee University of Information Technology,Solan,173234,Himachal Pradesh,India

Abstract In this paper,the non-static solutions for perfect fluid distribution with plane symmetry in f(R,T)gravitational theory are obtained.Firstly,using the Lie symmetries,symmetry reductions are performed for considered vector fields to reduce the number of independent variables.Then,corresponding to each reduction,exact solutions are obtained.Killing vectors lead to different conserved quantities.Therefore,we figure out the Killing vector fields corresponding to all derived solutions.The derived solutions are further studied and it is observed that all of the obtained spacetimes,at least admit to the minimal symmetry group which consists of ∂y,∂z and-z∂y+y∂z.The obtained metrics,admit to 3,4,6,and 10,Killing vector fields.Conservation of linear momentum in the direction of y and z,and angular momentum along the x axis is provided by all derived solutions.

Keywords: Einstein field equations,perfect fluid solutions,f(R,T) gravity,killing symmetries

1.Introduction

The curiosity to know about gravitation is still one of the famous mysteries in physics.This is also obvious,as gravity is inherent in spacetime rather than other forces of nature which are described by the fields defined on spacetime [1].General relativity (GR) is a consistent theory of gravity.According to GR,gravity is a demonstration of the curvature of spacetime rather than being a force.

GR gives a new notion to the Universe.Still,several drawbacks of GR such as the accelerating rate of the expansion of the Universe,spacetime singularities [2] were found and scientists began surprising whether GR is the only successful fundamental gravitational theory.So there are serious challenges to GR,that have to be figured out yet.

In order to figure out these challenges,two approaches are in use these days.Modifying the gravity theory is the first approach.The second approach is to follow the concepts of GR along with the introduction of dark energy/matter [3,4].

In this paper,we study about a modified theory of GR,namely f(R,T) [5,6] gravitational theory.Field equations(FEs)for this gravity theory depend on both R and T,where R and T are the scalar curvature and the trace of the matter tensor Tabrespectively [7].

The real world is a messy place,and we have no hope of finding a metric that describes the actual universe with perfect precision [1].Rather,we consider spacetime via many approximations using symmetry.Use of symmetry allows us to take a basic form of the metric,which is then calculated by solving the FEs.Here,we have considered the non-static plane symmetric metric.A solution of FEs is a metric,which is said to be exact if its components can be written in form of the holomorphic functions [8].We will go by using this definition.However,in general,there is no universal definition of exact solution.

As FEs are highly nonlinear differential equations(DEs),so there is no universal method to solve these.However,when it comes to non-linearity of DEs,the Lie symmetry method [9] has been proved most effective.Many authors have derived exact solutions of FEs in GR using this method[10–14].Jyoti et al[15]has used symmetry analysis in order to find the perfect fluid solutions for Einstein field equations.The exact vacuum accelarating non-static solutions have also been derived by Jyoti et al[16]using Lie symmetry approach.In this article,Lie symmetry analysis is used to find the nonstatic perfect fluid plane symmetric solutions of FEs in f(R,T).

Killing vector fields and continuous symmetries of the metric on the manifold are in one-to-one correspondence [1].For every Killing vector,there exists a corresponding conserved quantity.In fact,the metric remains unchanged along the direction of the Killing vector.Conservation of energy and momenta is provided by the existence of timelike and spacelike Killing vector respectively [1].

The outline of the current study is as follows: in section 2,form of FEs in f(R,T)gravity with perfect fluid has been introduced.Thereafter in section 3,the DEs corresponding to the FEs obtained in section 2,are considered.The section 4 provides the description of symmetry analysis method and the exact solutions to the system of partial DEs approaching via three different vector fields.Along with,the exact solutions to the FEs in f(R,T)theory,which are metrics,are also discussed.Killing vector fields are also obtained for the different cases.Finally,conclusion has been made based on the work done,in section 5.

2.f(R,T) gravity field equations with perfect fluid matter

GR is one of the successful theories of gravity.However,when it comes to the late time acceleration of the Universe,it faces challenges.GR also breaks down to explain the spacetime singularities [2].Therefore,the understanding of gravity beyond general relativity seems to be more pertinent in order to explain the observations.f(R,T)[6]is also one of such modified theories of gravity,in which Lagrangian is a function of R and T,where R is Ricci Scalar and T is trace of the energy-momentum tensor.f(R,T)gravity[6]is defined by using the action

Variation of the action S with respect to the components of metric tensor gabgives the following equations of motion for f(R,T) gravitational theory

Here ∇aand ∇bdenotes covariant derivatives,fTand fRdenotes the partial derivatives with respect to T and R,respectively,Tabis the stress tensor arising from the matter and energy term,and θabis the symmetric(0,2)tensor given as

The stress tensor for perfect fluid matter distribution [1] is given by

where Ua,p and ρ are 4-velocity vector,rest frame pressure and energy density,respectively.

Using the values of θaband Tabin (2) with some simplifications [17],we have

3.Plane symmetry in f(R,T) gravity

The form of the metric [8,18] in the rest frame of the fluid element for non-static spacetimes that exhibits plane symmetry can be written as

The only non-zero components of Rabfor the metric (6) are

Using (5a) and system (7),we have the following relations

Using (5b) and (7) in (8),and taking f(R,T)=-8πGT+eR leads to the following system of equations with p and ρ can take any value

From equation (9c),we have

where C is a constant.Using this,equation (9a) leads to

where A is constant.By use of(10)and(11)in(9b),leads to the following equation

4.Lie symmetry analysis and Killing vectors

Now we will perform Lie symmetry analysis of differential equation (12).Let us consider one parameter Lie group of transformations [9] for (12)

where ξ1,ξ2and η1are infinitesimals.The group of transformations (13) is generated by the vector field

Using (13) in equation (12),and then solving the system of corresponding determining equations gives the following infinitesimals

where Ci,(i=1,2,3)are arbitrary constants and Fj(t),(j=1,2,3)are arbitrary functions of t only.Therefore Lie algebra of equation (12) is spanned by the vector fields

Let us consider the following linear combinations of vector fields:

1.V1+αV2,where α is an arbitrary constant.

2.V1+V3.

4.1.Vector field V1+αV2

For vector field V1+αV2,the corresponding characteristic equation is

Solving the characteristic equation (14),the following similarity variables are obtained

where P is the new dependent variable of independent variable r.Using these relations in (12),the reduced ordinary differential equation (ODE) is

where ′ denotes the differentiation with respect to r.This ODE leads to the following solutions

where C3=CC2.So,the corresponding solution of FEs(2)is given as

For any line element

ξ is said to be a Killing vector if the Lie derivative of the metric tensor is zero i.e.,

where i,j=0,1,2,3.The expanded form of this equation for the metric in equation (6) gives the following system of Killing equations

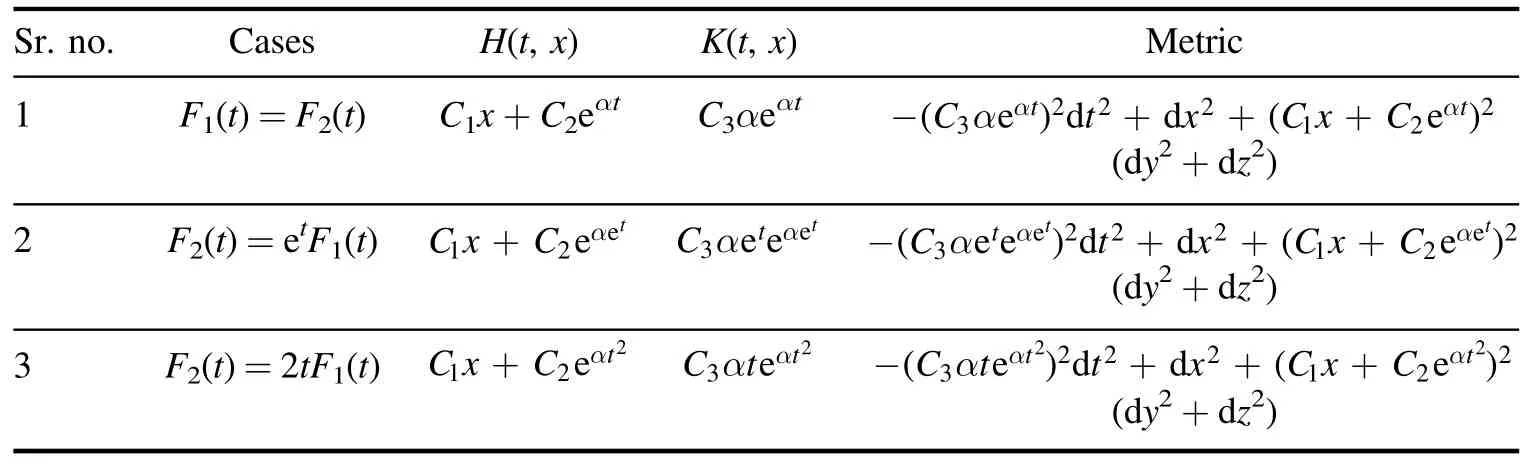
Table 1.Some particular forms of metrics for V1+αV2.

Table 2.Killing vector fields for V1+αV2.

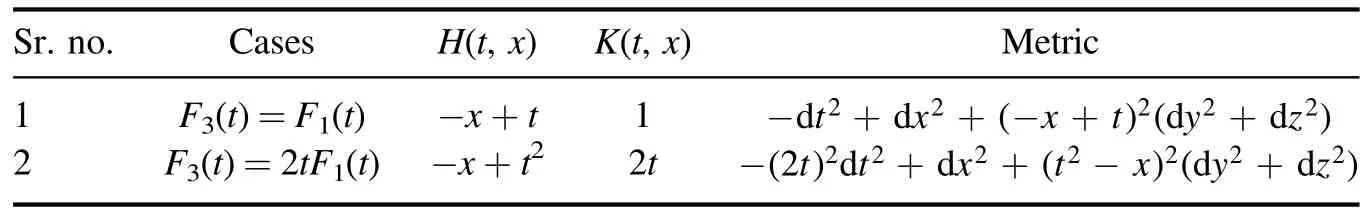
Table 3.Some particular forms of metrics for V1+V3.

Killing vector fields obtained by solving the Killing equations(17a)–(17j)corresponding to some particular forms of metric given in table 1,are represented in table 2.

4.2.Vector field V1+V3

For vector field V1+V3,the corresponding characteristic equation is

Now solving the characteristic equation (17),we obtain the following similarity variables

where P is the new dependent variable of independent variable r.Using these relations in equation (12),the reduced ODE is

where ′ denotes the differentiation with respect to r.

This ODE leads to the following solutions:

where C1=A1+A2/C,C2=-1/C and A1,A2are arbitrary constants.Using the transformation (19) and (10),we have

where C3is constant.

Now,the metric corresponding to these values is given by

Let C1=0,C3=C2=1.Using this,Killing vector fields and Lie algebra obtained by solving the Killing equations (17a)–(17j)corresponding to some particular forms of metrics given in table 3,are represented in table 4.

5.Conclusion

Finding exact solutions to FEs in modified gravitational theories is still a difficult task.In order to achieve this,various approaches are in use.When it comes to the Lie symmetry method,this gives us many new solutions to the considered system of DEs.Here also,this method has been applied to find a variety of new solutions of considered FEs.This study results in many important classes of metrics,including exponential nature.The spacetimes found here can work as important models for many useful physical systems,some of them may arise by appropriate values of constants.

Corresponding to each solution,Killing vectors are also found which can be used to find the conserved quantities.All spacetimes at least admit the minimal symmetry group which consists of ∂y,∂zand-z∂y+y∂z.The conservation of linear momentum is given by the spacelike Killing vectors ∂yand ∂zin the direction of y and z,respectively.Angular momentum conservation along the x axis is achieved for all obtained solutions.As our results involve the general functions of t and x,so more Killing vectors,and hence more conserved quantities can be found for special values of these functions.Solutions discussed in section (4.2) are rich with Killing vector fields,and hence with conserved quantities.

Acknowledgments

P D is very much thankful to UGC for providing financial support in the form of the JRF fellowship via letter NTA Ref.No.: 201610006334.S K wants to acknowledge the financial support provided under the scheme‘Fund for Improvement of S&T Infrastructure (FIST)’ of the Department of Science &Technology (DST),Government of India via letter No.SR/FST/MS-I/2021/104 to the Department of Mathematics and Statistics,Central University of Punjab.

ORCID iDs