THEORETICAL RESULTS ON THE EXISTENCE,REGULARITY AND ASYMPTOTIC STABILITY OF ENHANCED PULLBACK ATTRACTORS:APPLICATIONS TO 3D PRIMITIVE EQUATIONS∗
2023-12-14王仁海
(王仁海)
School of Mathematical Sciences, Guizhou Normal University, Guiyang 550001, China
E-mail: rwang-math@outlook.com
Boling GUO (郭柏灵)) Daiwen HUANG (黄代文)†
Institute of Applied Physics and Computational Mathematics, Beijing 100088, China
E-mail: gbl@iapcm.ac.cn; hdw55@tom.com
Abstract Several new concepts of enhanced pullback attractors for nonautonomous dynamical systems are introduced here by uniformly enhancing the compactness and attraction of the usual pullback attractors over an infinite forward time-interval under strong and weak topologies.Then we provide some theoretical results for the existence,regularity and asymptotic stability of these enhanced pullback attractors under general theoretical frameworks which can be applied to a large class of PDEs.The existence of these enhanced attractors is harder to obtain than the backward case [33],since it is difficult to uniformly control the long-time pullback behavior of the systems over the forward time-interval.As applications of our theoretical results,we consider the famous 3D primitive equations modelling the largescale ocean and atmosphere dynamics,and prove the existence,regularity and asymptotic stability of the enhanced pullback attractors in V×V and H2×H2 for the time-dependent forces which satisfy some weak conditions.
Key words 3D primitive equations;pullback attractors;regularity;flattening;stability
1 Introduction
Attractor theories and applications are two important topics in the study of the long-time behavior of solutions to partial differential equations of evolution type.Classically,a global attractor is a time-independent compact invariant attracting set in the underling space that can be used to capture the long-time dynamics of autonomous evolution equations.Recent decades have witnessed a great flourishing development of theories and applications regarding global attractors;see Ball [1],Hale-Raugel [11],Robinson [22],Temam [23],Sunet al.[25–27]and many others.
Alteratively,a pullback attractor is a time-dependent family of compact invariant pullback attracting sets defined with minimality in the underling space that could be employed to investigate the long-term dynamics of nonautonomous evolution equations.In the past two decades,there is a growing literature on the investigation of theories and applications of pullback attractors,see Caraballoet al.[2,3,34],Carvalho-Langa-Robinson [4],Kloedenet al.[13,14],Wang [28,29,32],and the references therein.
LetXandYbe two Banach spaces.We do not assume any embedding or limit-identical conditions onXandYas in [5,8,18,19].The usual pullback (X,Y)-attractor for a (X,Y)-process Φ={Φ(t,s):t ≥s ∈R} is the following:
Definition 1.1([4]forX=Y) A familyA={A(t)}t∈RinX ∩Yis called a pullback(X,Y)-attractor for Φ if
(i)A(t) is compact inX ∩Y,
(ii)Ais invariant,that is,Φ(t,s)A(s)=A(t) for allt ≥s ∈R,
(iii)A(t) is a pullback (X,Y)-attracting set,i.e.,for eacht ∈R and bounded setB ⊆X,
(iv)Ais the minimal one among the collection of closed pullback (X,Y)-attracting sets.
Note that a pullback attractor is a family of time-dependent sets,but in the aforementioned literature and many other places,some important properties of pullback attractors,such as compactness,attraction,stability,as well as fractal or Hausdorffdimensions,have been studied for each fixed time-sectionA(t).In this work we study the time-dependent properties of pullback attractors.
Enhancing the compactness and attraction of the usual pullback attractors inX ∩Yuniformly over the backward time-interval (-∞,t],the authors of [33](see also [5,17]) introduced the concepts of backward-uniformly compact and attracting pullback (X,Y)-attractors for Φ.They established several necessary and sufficient criteria for the existence,regularity and asymptotic stability of those attractors.
In the present paper,we introduce the forward-uniformly compact and attracting pullback(X,Y)-attractors for Φ by improving the compactness and attraction of the usual pullback attractors inX ∩Yuniformly over the forward time-interval [t,+∞),see Definitions 2.2–2.3.Note that the abstract results in the backward case [33]cannot be extended in a parallelled way to the forward cases because of the following two reasons:
•One can uniformly control the long-time pullback behavior of Φ inX ∩Yover the backward time-interval (-∞,t]by a backward-uniformly absorbing pullback absorbing set,since the directions of the “pullback behavior of Φ” and the “time-interval (-∞,t]” are the same;see [33].
•It is difficult or even impossible to uniformly control the large-time pullback behavior of Φ over the forward time-interval[t,+∞)if we only assume the existence of a forward-uniformly absorbing pullback absorbing set due to the opposite directions of the“pullback behavior of Φ”and the “time-interval [t,+∞)”.
In this article we will solve this non-parallelism problem,and establish several new theoretical criteria for the existence,regularity and asymptotic stability of the forward-uniformly compact and attracting pullback (X,Y)-attractors of Φ.As in the backward case [33],we can show that if Φ has a compact forward-uniformly attracting pullback(X,Y)-attracting set,then Φ has a forward-uniformly compact and attracting pullback(X,Y)-attractor;see Theorem 2.4.In general,such a compact attracting set is hard or even impossible to obtain in many applications,especially for weakly dissipative PDEs [31]and PDEs defined on unbounded domains[18].In order to solve this problem,we establish alternative abstract results for the existence and regularity of these enhanced pullback attractors inX ∩Yunder different conditions on Φ:(i)Φ is continuous from[s,∞)×XtoX ∩Y;(ii)Φ has a globally absorbing pullback absorbing set.
In the applications,although the global-uniformly absorbing condition is stronger than the forward-uniformly absorbing one,we can always obtain the existence of a bounded globaluniformly absorbing pullback (X,X)-absorbing set if we impose a global-uniform assumption on the forces,as in (4.18).The continuity of Φ from [s,∞)×XtoX ∩Y,however,is hard to verify for many PDEs.One may ask a natural question: what kinds of results can be established under a weak condition: (i)Φ is continuous from[s,∞)×XtoX;(ii)Φ has a bounded forwarduniformly absorbing pullback (X,X)-absorbing set? In this paper we will solve this problem under the frameworks of the weak topology ofX ∩Y.
A weak pullback (X,Y)-attractor for Φ with respect to the weak topology ofX ∩Yis the following:
Definition 1.2(see[28,29]X=Y) A familyAw={Aw(t)}t∈RinX ∩Yis called a weak pullback (X,Y)-attractor for Φ if it is the minimal one among the collection of sets satisfying
(i)Aw(t) is weakly compact inX ∩Y;
(ii)Awis a pullback weakly attracting set inX,i.e.,for eacht ∈R,bounded setB ⊆Xand weak neighborhoodNw(Aw(t)) ofA(t) inX ∩Y,there existsT=T(t,B,Nw(Aw(t)))>0 such that Φ(t,t-ι)B ⊆Nw(A(t)) for allι ≥T.
Uniformly enhancing the weak compactness and attraction of the usual weak pullback(X,Y)-attractors over[t,∞)in the weak topology ofX ∩Y,we introduce the forward-uniformly weakly compact and attracting weak pullback (X,Y)-attractors;see Definitions 3.1–3.2.Note that the invariance of these weak attractors is difficult to verify in applications,and so we do not require these weak attractors to be invariant,but we do require the minimality,in order to deal with the lack of invariance.Our main purpose is to establish some general theoretical results on the existence and regularity of the enhanced weak pullback(X,Y)-attractors under the theory of the weak topology.More specifically,letX ∩Ybe a reflexive Banach space,we will prove that if Φ has a bounded,closed and convex forward-uniformly absorbing pullback(X,Y)-absorbing set,then Φ has forward-uniformly weakly compact and attracting weak pullback (X,Y)-attractors.There are two advantages of these abstract results: one is that we do not need the strong compactness of the absorbing set and the asymptotic compactness of Φ inX ∩Y,and another one is that the frameworks can be applied to many dissipative or weakly dissipative PDEs defined on bounded or unbounded domains.It is worth mentioning that we assume thatX ∩Yis reflexive,but not separable.However,ifX ∩Yis further assumed to be separable,then the weak topology on a bounded subset ofX ∩Ycan be metrizable.Then the attraction of weak attractors can be defined in terms of the weak Hausdorffsemi-distance.In such a case,the existence of weak attractors can be proved by the standard approaches,as in [4].Meanwhile,ifX ∩Yis not separable,then we know that the weak topology of a bounded subset ofX ∩Yis not metrizable,and hence the attraction of weak attractors can not be defined in the usual sense.Nevertheless,we know that the weak topology ofX ∩Yhas a weak neighborhood base in the sense of (3.1);see Rudin [24].Based on this fact,we define the attraction according to the weak neighborhood base,and prove the existence of these enhanced weak attractors under the weak topology ofX ∩Y;see Theorem 3.4.
Another work is to apply the theoretical results to the well-known 3D primitive equation governing the large-scale dynamics of the ocean and atmosphere.Fixingh>0 and lettingMbe a smooth bounded domain in R2,we consider a 3D cylindrical domain Ω=M×(-h,0)⊆R3with a boundary∂Ω=Γu ∪Γb ∪Γsgiven by
Then we consider the following non-autonomous viscous primitive equation defined in Ω fort>τ ∈R:
with the homogeneous boundary conditions
and the initial conditions
In (1.1),v=(v1,v2),w,(v1,v2,w),pandTare called the horizontal velocity field,the vertical velocity,the 3D velocity field,the pressure and the temperature,respectively.f0=f0(β+y) is the Coriolis rotation frequency with aβ-plane approximation,k is a unit vector in the vertical direction,k×v=(-v2,v1),g=(g1,g2) andQare the given time-dependent external force field and heat source,respectively,are the horizontal gradient and Laplace operators,respectively,are the viscosity and heat diffusion operators,whereRe1>0 andRe2>0 are the horizontal and vertical Reynolds numbers,Rt1>0 andRt2>0 are the horizontal and vertical heat diffusion,respectively.In (1.2),α>0 is a constant and n is the normal vector with respect to Γs.
The primitive equations are used to model the large-scale dynamics of the atmosphere and ocean.Roughly speaking,these equations were derived from the Navier-Stokes equations with the rotation coupled with thermodynamics and salinity diffusion-transport equations under the Boussinesq and hydrostatic approximations based on the scale analysis argument.The global existence of weak solutions to (1.1)–(1.3) was investigated in the literature,the uniqueness of weak solutions is still unsolved.The global existence and uniqueness of strong solutions to(1.1)–(1.3) were systematically solved by Cao-Titi [7].The existence of attractors of (1.1)–(1.3) was examined by Guo and Huang [9,10],see also [35,36].More recently,the existence,regularity and asymptotic stability of backward-uniformly compact and attracting pullback(V×V,H2×H2)-attractors of (1.1)–(1.3) were investigated in [33].
Under different conditions on theL2-norms (notH1-norms) of g andQ,we prove the existence,regularity and asymptotic stability of the usual and enhanced pullback attractorsA,A,A,A,Aw,Awand Awin the sense of Definitions 1.1,2.1,2.2,2.3,3.1 and 3.2 under the strong and weak topology of V×Vand H2×H2.To prove the existence of these attractors in V×V,we derive forward-uniform estimates and “flattening properties” of the solutions in V×V.To prove the regularity of these attractors in H2×H2,we prove the forward-uniform Hölder continuity of the solutions from V×Vto H2×H2with respect to the initial data.To prove the asymptotic stability of these attractors,we show that the solution to (1.1)–(1.3) is forward asymptotically stable to the solution to an autonomous version of (1.1)–(1.3)in V×Vwhen g andQconverge to two time-independent functions.For the convenience of the reader,we summarize our main results via the following two tables.In Table 2,A∞:=∩t∈RA(t),andA∞is a global attractor of an autonomous version of (1.1)–(1.3).
The rest of this paper is organized as follows.In Sections 2–3,we establish several abstract results on the existence,regularity and asymptotic stability of the enhanced pullback(X,Y)-attractors under the strong and weak topology ofX ∩Y.In Section 4,we apply our abstract results to the 3D primitive equations (1.1)–(1.3),and prove the existence,regularity and asymptotic stability of enhanced pullback attractors for(1.1)–(1.3)in V×Vand H2×H2.
2 Abstract Results on Enhanced Pullback Attractors Under Strong Topology
In this section we establish several abstract results on the existence,uniqueness and regularity of enhanced pullback attractors for non-autonomous dynamical systems under the strong topology.These frameworks can be applied to many kinds of non-autonomous PDEs defined on bounded or unbounded domains,including dissipative or weakly dissipative equations.In particular,we will apply these abstract results to the 3D primitive equation (1.1) for different conditions on g andQ.
Let(X,‖·‖X)and(Y,‖·‖Y)be two Banach spaces,where we do not restrict any embedding or limit-identical conditions onXandY;see [8,18].Denote byB(X) the collection of all nonempty bounded sets inX,and by distX∩Y(·,·) the Hausdorffsemi-distance of two sets inX ∩Y.A non-autonomous dynamical (X,Y)-system Φ={Φ(t,s) :t ≥s,s ∈R} is a family of mappings fromXtoX ∩Y,satisfying,for alls ∈R,Φ(s,s) is an identity mapping and the process property Φ(t,s)=Φ(t,r)Φ(r,s) for allt ≥r ≥s.In what follows,we always assume,unless stated otherwise,that Φ is continuous from[s,∞)×XtoX,that is,Φ(tn,s)xn →Φ(t,s)xinX,provided thattn →tandxn →xinX.
By improving the compactness of the usual pullback attractors inX ∩Yat infinity,we introduce an enhanced version of the usual pullback attractors.
Definition 2.1A familyA={A(t)}t∈RinX ∩Yis called a forward asymptotically compact pullback(X,Y)-attractor for Φ if it is a usual pullback(X,Y)-attractor,and is forward asymptotically compact inX ∩Y,that is,any sequencewithsn →+∞has a convergence subsequence inX ∩Y.
By enhancing the compactness and attraction of the usual pullback attractors inX ∩Yuniformly over the infinite interval [t,∞),we introduce two enhanced versions of the usual pullback attractors.
Definition 2.2A familyA={A(t)}t∈RinX ∩Yis called a forward-uniformly compact pullback (X,Y)-attractor for Φ if it is a usual pullback (X,Y)-attractor,andis compact inX ∩Y.
Definition 2.3A family A={A(t)}t∈RinX ∩Yis called a forward-uniformly attracting pullback (X,Y)-attractor for Φ if it is the minimal one among the collection of sets satisfying
(i) A(t) is compact inX ∩Y,
(ii) A is a forward-uniformly attracting pullback (X,Y)-attracting set,that is,
Note that the attractorsA,AandAin Definitions 1.1,2.1 and 2.2 (if they exist) must be equal due to their minimality and invariance.The only difference is that the compactness ofAorAis stronger than that ofA.In the backward case [33],item (iv) of Definition 1.1 can be deduced from items (i)–(iii) ifAis backward compact.But,in the forward case,one cannot deduce item (iv) of Definition 2.2 by using items (i)–(iii) ifAis forward compact.Note that “A exists”⇒“Aexists”⇒“Aexists”⇒“Aexists”.Compared with the backward case in [33],the existence of these enhanced pullback (X,Y)-attractors is hard to obtain,since it is very difficult to uniformly control the large-time pullback behavior of Φ over [t,+∞).
For eacht ∈R andB ⊆X,we define two setsAs in [33],we find that a pointy ∈X ∩Ybelongs to∈ω(B,t)if and only if there existιn →+∞andxn ∈Bsuch that‖Φ(t,t-ιn)xn-y‖X∩Y →0 asn →∞,and that a pointy ∈X ∩Ybelongs to∈Ω(B,t)if and only if there existιn →+∞,sn ≥tandxn ∈Bsuch that‖Φ(sn,sn-ιn)xn-y‖X∩Y →0 asn →∞.
The following theorem provides a sufficient criterion for the existence of the enhanced pullback (X,Y)-attractors in the sense of Definitions 2.2 and 2.3.The proof is similar to [33],and is hence omitted:
Theorem 2.4If Φ has a compact forward-uniformly attracting pullback(X,Y)-attracting set,then Φ has forward-uniformly compact and attracting pullback (X,Y)-attractorsA={A(t)}t∈Rand A={A(t)}t∈Rgiven by
In applications,such a compact attracting set is hard to obtain,so we further develop another sufficient criterion for the existence of such enhanced pullback (X,Y)-attractors.For this purpose,we need the following enhanced versions:
Definition 2.5We say a familyK={K(t)}t∈RinX ∩Yis a forward-uniformly absorbing pullback(X,Y)-absorbing set for Φ,if for eacht ∈R andB ∈B(X),there existsι0=ι0(t,B)>0 such that
Definition 2.6We say a setKinX ∩Yis called a global-uniformly absorbing pullback(X,Y)-absorbing set for Φ,if for eachB ∈B(X),there existsι0=ι0(B)>0 (independent ofs) such that
Definition 2.7We say Φ is forward-uniformly pullback asymptotically(X,Y)-compact if for eacht ∈R andB ∈B(X),the sequencehas a convergent subsequence inX ∩Yfor allsn ≥t,ιn →+∞andxn ∈B.
Unlike the backward case in [33],we establish a necessary and sufficient criterion for the existence of the enhanced pullback (X,Y)-attractors in Definition 2.3 if there exists a bounded global-uniformly absorbing pullback (X,X)-absorbing set.
Theorem 2.8Assume that Φ has a bounded global-uniformly absorbing pullback(X,X)-absorbing setK={K(t)}t∈R.Then Φ has a forward-uniformly attracting pullback (X,Y)-attractor A={A(t)}t∈Rif and only if Φ is forward-uniformly pullback asymptotically (X,Y)-compact.In this case,A is given by A(t)=Ω(K(t),t).
Proof NecessityThe proof is similar to that of the backward case [33],and so we do not repeat it.
SufficiencyAs in[33],we can prove the compactness of A inX ∩Y.Next,we show that A is a forward-uniformly attracting pullback (X,Y)-attracting set.If this is not the case,then there existδ>0,sn ≥t0∈R,ιn →+∞andxn ∈B0∈B(X) such that
By the global-uniform attraction ofK,there existsn1:=n1(t0,B0)∈N such that,for alln ≥n1,
By the forward-uniform pullback asymptotic (X,Y)-compactness of Φ,there existy0∈X ∩Yand a subsequencesuch that Φ(snk,snk-ιnk)xnk →y0inX ∩Yask →∞.Then,by the process property of Φ,we know thatznk=Φ(snk,snkιnk)xnk →y0inX ∩Y.This,together with the compactness of A(t0) inX ∩Y,(2.2) and the character of Ω(K(t0),t0),gives thaty0∈Ω(K(t0),t0)=A(t0),which stands in contradiction to (2.1).The minimality of A can be proven as in [33].The proof is completed.
Due to the structure of A(t)=Ω(K(t),t),such a forward-uniformly attracting pullback(X,Y)-attractor A={A(t)}t∈Rmust be decreasing;that is,A(t1)⊆A(t2) fort1≥t2.This property is useful for discussing the stability of A(t) inX ∩Yast →+∞.
In Theorem 2.8,we cannot control the long-time pullback behavior of Φ uniformly over the forward interval [t,+∞) if we assume the existence of a forward-uniformly absorbing pullback absorbing set.In the next result,we will show the existence of an enhanced pullback (X,Y)-attractor in the sense of Definition 2.2,where we do not assume the existence of a globaluniformly absorbing pullback absorbing set but do need the continuity of Φ from [s,∞)×XtoX ∩Y.
Theorem 2.9Assume that Φ is continuous from [s,∞)×XtoX ∩Y;that is,that Φ(tn,s)xn →Φ(t,s)xinX ∩Yprovided thattn →tandxn →xinX.Suppose that the following two conditions hold:
(i) Φ has a bounded and decreasing pullback (X,X)-absorbing setK={K(t)}t∈R;
(ii) Φ is forward-uniformly pullback asymptotically (X,Y)-compact.Then Φ has a forward-uniformly compact pullback (X,Y)-attractorA={A(t)}t∈Rgiven byA(t)=ω(K(t),t).
ProofBy (i) and (ii) we can show thatA,given byA(t)=ω(K(t),t),is just a usual pullback (X,Y)-attractor in the sense of Definition 1.1.Then,by Definition 2.2,it suffices to prove thatis pre-compact inX ∩Yfor eacht ∈R.Take a sequencefromThen there existssn ≥tsuch thatyn ∈A(sn) for eachn ∈N.
By the continuity assumption on Φ,we know that Ψ is continuous from [t,s0]×A(t) toX ∩Y.This,together with (2.4),implies thatis compact inX ∩Y,and thereby,has a convergent subsequence inX ∩Y.
Corollary 2.10If Φ is continuous from[s,∞)×XtoX ∩Y,then any compact invariant setA={A(t)}t∈RinXmust be locally-uniformly compact inX ∩Y;that is,∪s∈IA(s) is compact inX ∩Yfor every compact intervalI ⊆R.
In the backward case [33],we do not need the continuity of Φ from [s,∞)×XtoX ∩Y.When we apply Theorem 2.9 to some PDEs,it is relatively easy to obtain the continuity of Φ from [s,∞)×XtoX.However,the continuity of Φ from [s,∞)×XtoX ∩Yis hard to verify in general ifX≠Y.Nevertheless,in light of the proof of Theorem 2.9,we can obtain the existence of forward asymptotically compact pullback (X,Y)-attractors if Φ is continuous from [s,∞)×XtoX.This is useful for discussing the asymptotic stability of the time-section of the enhanced pullback attractors inX ∩Ywhen the time variable towards to infinity along the future times.
Corollary 2.11Assume that Φ is continuous from [s,∞)×XtoX.If (i) and (ii)of Theorem 2.9 hold,then Φ has a unique forward asymptotically compact pullback (X,Y)-attractor in the sense of Definition 2.1.
In what follows,we present several results for the relations and topology properties of these enhanced pullback attractors.Note that the proofs are similar to those of the backward case in [33],and are hence omitted.
Proposition 2.12If Φ has a forward-uniformly attracting pullback(X,Y)-attractor A={A(t)}t∈R,then Φ has a forward-uniformly compact pullback (X,Y)-attractorA={A(t)}t∈Rsuch that
Proposition 2.13If Φ is forward-uniformly pullback asymptotically (X,Y)-compact,then a forward-uniformly compact pullback(X,X)-attractor must be a forward asymptotically compact pullback (X,Y)-attractor.
Proposition 2.14If Φ is continuous from[s,∞)×XtoX∩Y,and Φ is forward-uniformly pullback asymptotically (X,Y)-compact,then a forward-uniformly compact pullback (X,X)-attractor must be a forward-uniformly compact pullback (X,Y)-attractor.
Proposition 2.15If Φ is forward-uniformly pullback asymptotically (X,Y)-compact,and Φ has bounded global-uniformly absorbing pullback (X,X)-absorbing set,then a forwarduniformly attracting pullback(X,X)-attractor must be a forward-uniformly attracting pullback(X,Y)-attractor.
In order to discuss the asymptotic stability of the enhanced pullback attractors inXandX ∩Y,we next establish some general theoretical results for the asymptotic stability of timedependent sets inXandX ∩Y,respectively.LetTbe a(X,Y)-semigroup.We say Φ is forward asymptotically stable to the semigroupTfromXtoXif
We say that a non-autonomous setA={A(t)}t∈Ris forward asymptotically stable to an autonomous setA∞inX ∩Yif
The proofs of the next results are similar to those of [16,33],and are thus omitted here.
Theorem 2.16LetA={A(t)}t∈Rbe an invariant set of Φ inX.LetA∞be a compact attracting set forTinX.If (2.5) holds,thenAis forward asymptotically stable toA∞inXif and only ifAis forward-uniformly compact inX.
Theorem 2.17Assume thatA={A(t)}t∈RandA∞are two compact sets inX∩Y.ThenAis forward asymptotically stable toA∞inX ∩Yif and only ifAis forward asymptotically stable toA∞inXandAis forward asymptotically compact inX ∩Y.
Theorem 2.18Let A={A(t)}t∈Rbe a monotonically decreasing family of nonempty compact sets inX ∩Y.Then the set A∞:=∩s∈RA(s)is the minimal one among the collection of sets satisfying that
Remark 2.19In applications,the sets in Theorems 2.16–2.18 can be chosen as the enhanced pullback attractors in Definitions 2.2–2.1.For the asymptotic stability of the timesectionAε(t)of the usual pullback attractors with respect to the external parameterε,we refer the reader to Carvalho-Langa-Robinson [4].
3 Abstract Results on Enhanced Pullback Attractors Under Weak Topology
In Theorem 2.8,we assume the existence of a global-uniformly absorbing pullback absorbing set to uniformly control the pullback limiting behavior of system Φ over [t,+∞).In Theorem 2.9,we require that Φ is continuous from [s,∞)×XtoX ∩Yin order to obtain the forward-uniform compactness of the attractors.Note that we do not need such conditions in the backward case [33],since the long-time pullback behavior of system Φ can be uniformly controlled over (-∞,t]by a backward-uniformly absorbing pullback absorbing set.
In sharp contrast,it is natural to ask: what kinds of results can be obtained if we only assume that (i) the existence of a forward-uniformly absorbing pullback absorbing set;and (ii)the continuity of Φ from [s,∞)×XtoX? In this section we establish some unified abstract results on the existence,uniqueness and regularity of enhanced pullback attractors for Φ under the weak topology ofX ∩Y.
Before starting our main results,we first recall some notations and results regarding the theory of the weak topology;see [24].LetEbe a Banach space.For eachf∗∈E∗,there exists a linear functionalϕf∗:E →R defined byforx ∈E,wheredenotes the duality pairing ofE∗andE.Denote by (ϕf∗)f∗∈E∗the collection of all maps fromEinto R.Then the weak topology onEdenoted byσ(E,E∗) is the coarsest topology associated with the collection (ϕf∗)f∗∈E∗.The next results play an important role in the study of the existence of pullback attractors under the weak topology.(1) The weak topologyσ(E,E∗) is Hausdorff.(2) Ifxn →xweakly inσ(E,E∗),thenxnis bounded inEand(3)In infinite-dimensional spaces the weak topologyσ(E,E∗)is not metrizable.(4) The weak topology on a bounded subset ofXis metrizable if and only ifEis separable.(5) A convex subset ofEis closed in the weak topology if and only if it is closed in the strong topology.(6) IfEis a reflexive Banach space,then any bounded,closed and convex subset ofEmust be weakly compact inE.(7)Eis reflexive and separable ifE∗is reflexive and separable.(8) The weak topology ofEhas a neighborhood base at a pointx0∈Eforσ(E,E∗),given by the collection:
Recall that a weak neighborhood of a pointx0∈E(or a setB ⊆E) denoted byNw(x0)(Nw(B)) is a weakly open set containingx0(B).
Strengthening the compactness and attraction of the weak pullback (X,Y)-attractors uniformly over [t,∞) in the weak topologyσ(X ∩Y,(X ∩Y)∗),we introduce the following two enhanced versions of Definition 1.2:
Definition 3.1A familyAw={Aw(t)}t∈RinX ∩Yis called a forward-uniformly weakly compact weak pullback (X,Y)-attractor for Φ if it is the minimal one among the collection of sets satisfying that
(i) bothAw(t) andare weakly compact1The closure is taken in the weak topology of X ∩Y.inX ∩Y;
(ii)Awis a pullback weakly (X,Y)-attracting set;i.e.,for eacht ∈R,B ∈B(X) and weak neighborhoodNw(Aw(t)) ofAw(t) inX ∩Y,there existsT=T(t,B,Nw(Aw(t)))>0 such that Φ(t,t-ι)B ⊆Nw(Aw(t)) for allι ≥T.
Definition 3.2A family Aw={Aw(t)}t∈RinX ∩Yis called a forward-uniformly weakly attracting weak pullback (X,Y)-attractor for Φ if it is the minimal one among the collection of sets satisfying that
(i) Aw(t) is weakly compact inX ∩Y;
(ii)Awis a forward-uniformly weakly attracting pullback(X,Y)-attracting set;that is,for eacht ∈R,B ∈B(X) and weak neighborhoodNw(Aw(t)) of Aw(t) inX ∩Y,there existsT=T(t,B,Nw(Aw(t)))>0 such thatfor allι ≥T.In order to investigate the existence and uniqueness of these enhanced weak pullback(X,Y)-attractors,we introduce the concept of the weak pullbackωw(Ωw)-limit sets.For eacht ∈R andB ⊆X,we define that
The following results are concerned with the properties of the weak pullbackωw(Ωw)-limit sets:
Lemma 3.3For eacht ∈R andB ⊆X,the weak pullbackωw(Ωw)-limit sets have the following properties:
(i) A pointy0∈X ∩Ybelongs toωw(B,t) if and only if,for everyε>0 and,···,∈(X ∩Y)∗,there existιn →+∞andxn ∈Bsuch thatfor alln ∈N.
(ii)A pointy0∈X ∩Ybelongs to Ωw(B,t),if and only if for everyε>0 and,···,∈(X ∩Y)∗,there existιn →+∞,sn ≥tandxn ∈Bsuch thatfor alln ∈N.
ProofThe proof of (i) is almost identical with that of (ii).Thus,we only prove (ii).
SufficiencyTakey0∈X ∩Y.LetNw(y0)be an arbitrary weak neighborhood ofy0with respect to the weak topology ofX∩Y.Since the collection given by(3.1)is a neighborhood base aty0with respect to the weak topology ofX ∩Y,there existε>0 and,···,∈(X ∩Y)∗such thatNotice that there existsn ≥t,ιn →+∞andxn ∈Bsuch thatfor alln ∈N.Then we find that Φ(sn,sn-ιn)xn ∈Nw(y0)for alln ∈N.On the other hand,for eachr ≥0,byιn →+∞,there existsN ∈N such thatιn ≥rfor alln ≥N.Then,for alln ≥N,
By the character of the weak Ωw(ωw)-limit sets,we next establish the existence of the enhanced weak pullback (X,Y)-attractors for Φ in the sense of Definition 3.2,provided that we have the existence of a bounded,closed and convex forward-uniformly absorbing pullback(X,Y)-absorbing set.
Theorem 3.4Assume thatX ∩Yis a reflexive Banach space.If Φ has a bounded,closed and convex forward-uniformly absorbing pullback (X,Y)-absorbing setK={K(t)}t∈Rin the sense of Definition 2.5,then we have the following two conclusions:
(i) Φ has a forward-uniformly weakly attracting weak pullback (X,Y)-attractor Aw={Aw(t)}t∈Rgiven by
(ii) Φ has a forward-uniformly weakly compact weak pullback (X,Y)-attractorAw={Aw(t)}t∈Rgiven by
Proof(i) First,we show the weak compactness of AwinX ∩Y.We assert Ωw(B,t)⊆K(t) for allt ∈R andB ∈B(X).If this is false,then there existt0∈R,B0∈B(X) andy0∈X ∩Ysuch thaty0∈Ωw(B0,t0)K(t0).This implies that
Note that for eacht ∈R,K(t)is closed,bounded and convex inX ∩Y,so it is weakly compact,and hence weakly closed inX ∩Y.Then (X ∩Y)K(t0) is weakly open,and thus there exists a weak neighborhoodNw(y0) ofy0inX ∩Ysuch thatNw(y0)⊆(X ∩Y)K(t0).On the other hand,by the neighborhood base aty0∈X ∩Ygiven by (3.1),there existε>0 and,···,∈(X ∩Y)∗such thatThen we obtain that
By Lemma 3.3 andy0∈Ωw(B0,t0),there existsn ≥t0,ιn →+∞andxn ∈B0such that
Consider the weak neighborhood ofK(t0) given bySinceKis a forward-uniformly absorbing pullback (X,Y)-absorbing set for Φ,it is a forwarduniformly weakly attracting pullback (X,Y)-attracting set for Φ.Thus,there existsT1=T1(t0,ε,,···,,K,B0)≥0 such that,for allt ≥T1,
We now show the forward-uniform weak (X,Y)-attraction of Aw;that is,for eacht ∈R,B ∈B(X) and weak neighborhoodNw(Aw(t)) of Aw(t) inX ∩Y,there existsT=T(t,B,Nw(Aw(t)))>0 such that ∪s≥tΦ(s,s-ι)B ⊆Nw(Aw(t)) for allι ≥T.If this is not the case,then there existt0∈R,B0∈B(X)ιn →+∞and a weak neighborhoodNw(Aw(t0)) of Aw(t0) inX ∩Ysuch that ∪s≥t0Φ(sn,s-ιn)B0⊆Nw(Aw(t0)) is false.It follows that there exist two further sequences,sn ≥t0andxn ∈B0,such that
Note thatKis a forward-uniformly absorbing pullback (X,Y)-absorbing set for Φ,and bytn →+∞,there existsN2=N2(t0,B0,K)∈N such that Φ(sn,sn-ιn)xn ∈K(t0) for alln ≥N2.SinceK(t0) is weakly compact in the reflexive Banach spaceX ∩Y,as we mentioned before,there existy0∈X ∩Yand a subsequence which we do not relabel such that
Notice that(X ∩Y)Nw(Aw(t0))is weakly closed,sinceNw(Aw(t0))is weakly open.By(3.9)and (3.10),we get that
We finally prove that Awis the minimal one among the collection of sets satisfying (i)and (ii) of Def.3.1.Letbe a set satisfying (i) and (ii) of Def.3.1.We want to show Aw(t)⊆(t) for allt ∈R.If this is not the case,then there existt0∈R andy0∈X ∩Ysuch thaty0∈Aw(t0)(t0),and hence
Byy0∈Aw(t0),there existsB0∈B(X)such thaty0∈Ωw(B0,t0).Then,by Lemma 3.3,there existsn ≥t0,ιn →+∞andxn ∈B0such that
Remark 3.5(i) The results in Theorem 3.4 can also be extended to the backward and global cases.(ii)If Φ has a forward-uniformly weakly attracting weak pullback(X,Y)-attractor Aw,then Φ has a forward-uniformly weakly compact weak pullback (X,Y)-attractorAwsuch that(iii) In Theorem 3.4,we assume that the spaceX ∩Yis reflexive but not separable.However,if we further assume thatX ∩Yis separable,then the weak topology on a bounded subset ofX ∩Yis metrizable.In this case,the attractions of the pullback attractors can be defined in terms of the corresponding weak metric,and hence the existence of weak pullback attractors can be proven by the standard methods used in [4].(iv)The weak pullback attractors are defined without invariance,since we do not assume that Φ is weakly continuous fromXtoX.(v) An advantage of Theorem 3.4 is that we do not require the strong compactness ofKand Φ.
4 Applications to 3D Primitive Equations
In this section we will apply the theoretical results achieved in Sections 2–3 to the 3D primitive equation.In particular,we will present and prove our main results on the existence,regularity and asymptotic stability of the enhanced pullback attractors for problem (4.1)–(4.3)in V×Vand H2×H2when g andQsatisfy different conditions.
4.1 Non-autonomous Dynamical (V×V,H2×H2)-systems
As in [7],we have a new formulation for v andT
with the homogeneous boundary conditions
and the initial conditions:
with the boundary conditions
and the initial condition
with the boundary conditions
and the initial condition
As in [7],we can prove that for everyτ ∈R,T>0,(vτ,Tτ)∈V×V,g∈(R,L2(Ω)),Q ∈(R,L2(Ω)),problem (4.1)–(4.3) has a unique strong solution (v(t,τ,vτ),T(t,τ,Tτ))which satisfies (4.1) in the weak sense,and
In addition,the solution continuously depends on(vτ,Tτ)from V×Vto V×V.Forι ≥0 andt ∈R,we may write (v(t,t-ι,vt-ι),T(t,t-ι,Tt-ι)) as the solutions to (4.1)–(4.3) at timetwith initial timet-ιand initial data (vt-ι,Tt-ι).Define a mapping from V×Vto V×Vby
Then Φ is a continuous(V×V,H2×H2)-process.In what follows,the letterc>0 will denote a generic constant which may change its value in different places.The following uniform Grönwall lemma will play an important role in deriving all necessary uniform estimates in V×Vand H2×H2.
Lemma 4.1Givens ∈R,lety(r)≥0 andh1(r)≥0,h2(r) andh3(r)≥0 be locally integrable functions on [s-1,+∞) such thatThen
4.2 Enhanced Pullback Attractors in V×V
Our first result is concerned with the existence and uniqueness of enhanced pullback attractors in the sense of Definitions 2.2,2.3 and 3.2 withX=Y=V×V.Other direct results can be found in Table 1.
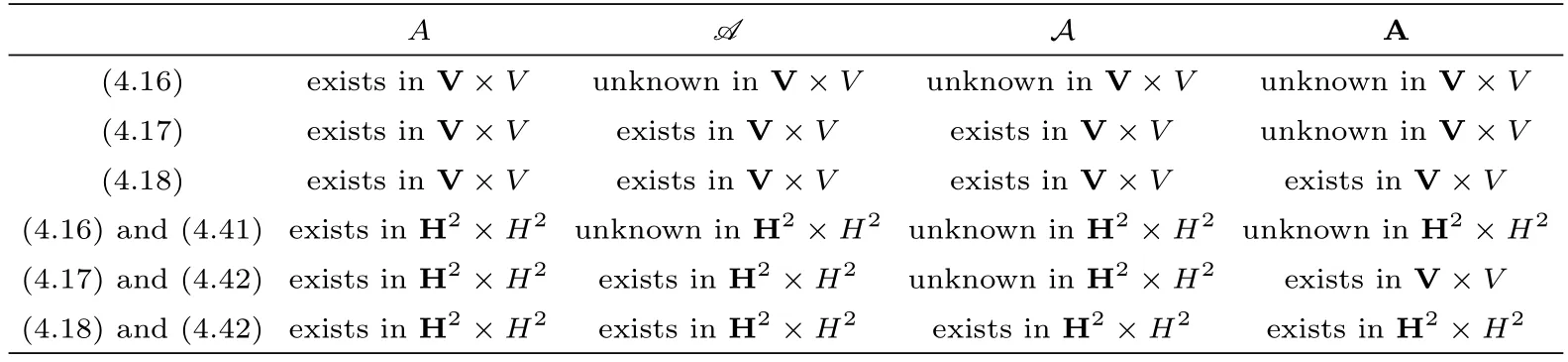
Table 1 Pullback attractors of (1.1)–(1.3) under strong topology
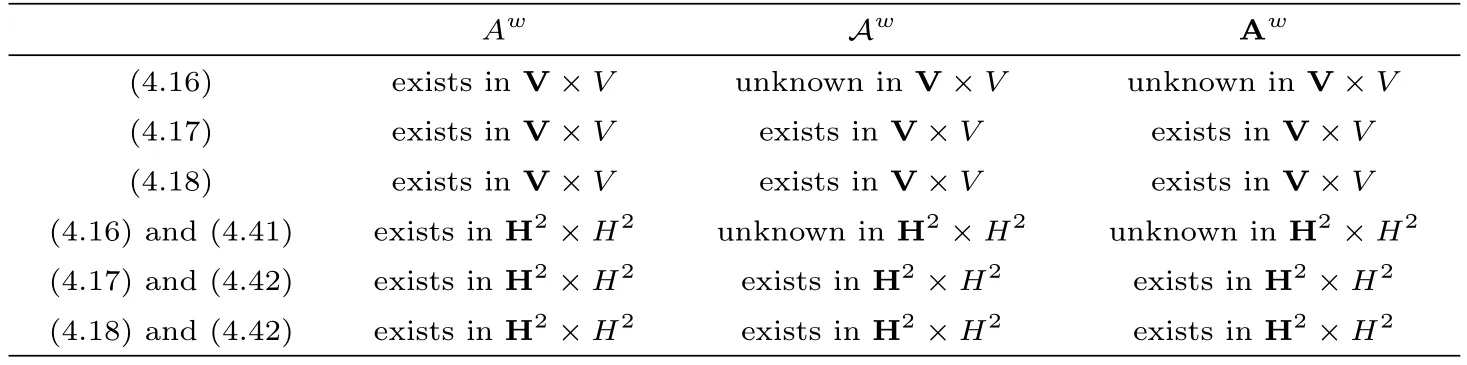
Table 2 Pullback attractors of (1.1)–(1.3) under weak topology
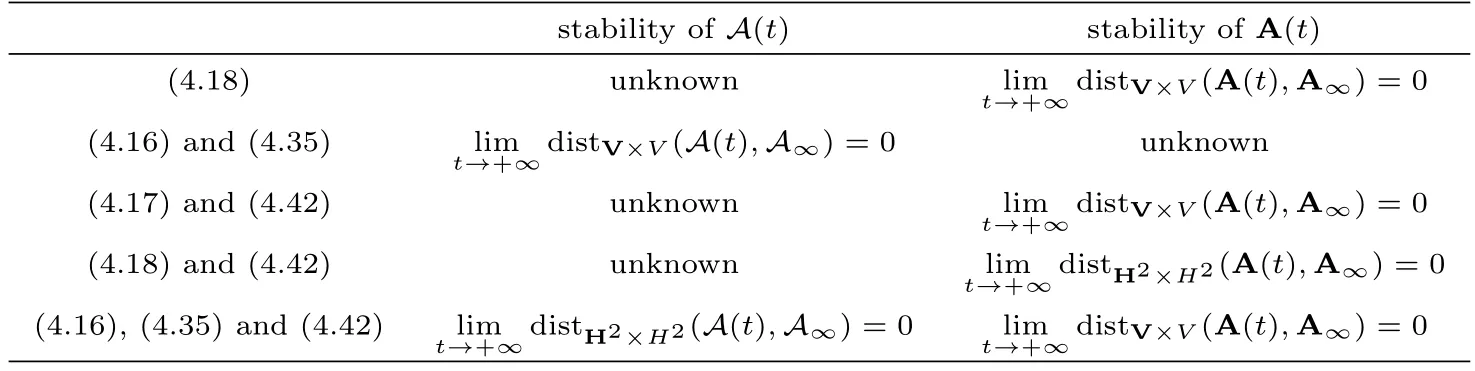
Table 3 Asymptotic stability of pullback attractors of (1.1)–(1.3)
Theorem 4.2(i)If (4.17)holds,then Φ has a unique forward-uniformly compact pullback(V×V,V×V)-attractorA={A(t)}t∈Rgiven by,whereK={K(t)}t∈Ris a closed,bounded and decreasing pullback (V×V,V×V)-absorbing set of Φ,given by
In order to prove Theorem 4.2,according to our theoretical criteria for the existence of enhanced pullback attractors,we should show the forward-uniform pullback asymptotic compactness of Φ in V×Vover [t,+∞).As mentioned in [33],Ball’s idea of energy equations[1]and the Aubin-Lions compactness approach [15]can be used to prove the usual pullback asymptotic compactness of Φ in V×V.At present,it seems that these methods are not useful for proving the uniform pullback asymptotic compactness of Φ over the infinite time-interval[t,+∞).However,we will use the spectral theory to derive the forward-uniform “flattening properties” of the solutions in V×V.This further implies the uniform pullback asymptotic compactness of Φ in V×V.
We borrow the following energy inequalities from [33]:
Proposition 4.3The strong solutions of problem (4.1)–(4.3) satisfy the energy inequalities
By applying the uniform Grönwall’s Lemma 4.1 to these energy inequalities,we have the following large-time uniform estimates of (4.1)–(4.3) in V×V.
Lemma 4.4For eacht ∈R and B×B ∈B(V×V),we have the following conclusions.
(i) If (4.17) holds,then there existsT=T(t,B×B)≥1 such that
(ii) If (4.18) holds,then there existsT=T(B×B)≥1 such that
ProofWe only prove(i).For each fixedt ∈R,we letσ ∈[s-1,s],(vs-ι,Ts-ι)∈B×Bands ≥t.Applying the Grönwall Lemma 4.1 to (4.19) and (4.20),by (4.17),we find that,there existsT:=T(t,B×B)≥1 such that,for allι ≥T,
Applying the Lemma 4.1 to (4.21),we find from (4.27) that,for allι ≥T,
Applying Lemma 4.1 to (4.22),we infer from (4.27) and (4.28) that,for allι ≥T,
Applying Lemma 4.1 to (4.23),we infer from (4.27),(4.28) and (4.29) that,for allι ≥T,
Applying Lemma 4.1 to (4.24),we deduce from (4.27),(4.28) and (4.30) that,for allι ≥T,
Applying Lemma 4.1 to (4.25),we deduce from (4.27),(4.28),(4.30) and (4.31) that,for allι ≥T,
Applying Lemma 4.1 to(4.26),we infer from(4.27),(4.28),(4.30)and(4.32)that,for allι ≥T
Then we find that (i) is just a direct consequence of (4.31)–(4.33).
Next,we use the spectral theory on compact symmetric operators to show that the solutions to (4.1)–(4.3) have uniform “flattening effects” in V×V over the infinite time-interval [t,∞).Define a(·,·) : V× V→R anda(·,·) :V×V →R by a(v,u)=andThere are two operators A:V→V∗andA:V →V∗such that
Lemma 4.5If (4.17) holds,then,for eacht ∈R and B×B ⊆B(V×V),we have
For each fixedt ∈R,applying Lemma 4.1 to (4.34),we deduce from Lemma 4.4 that,for allσ ∈[s-1,s],s ≥t,ς ≥Tand (vs-ι,Ts-ι)∈B×B,
This completes the proof.
Now we want to prove Theorem 4.2 by using Lemmas 4.4 and 4.5.
Proof Theorem 4.2(i) Let (4.17) hold true.By Lemma 4.4,we know thatKis a bounded decreasing pullback (V×V,V×V)-absorbing set of Φ in V×V.By Lemmas 4.4 and 4.5,we can show that Φ is forward-uniformly pullback asymptotically(V×V,V×V)-compact.Thus,by Theorem 2.9 withX=Y=V×V,we can complete the proof of (i).
(ii) Let (4.17) hold true.By Lemma 4.4,we know thatKis a bounded,closed and convex forward-uniformly absorbing pullback (V×V,V×V)-absorbing set for Φ.Then,by Theorem 3.4 we can complete the proof of (ii).
(iii) Let (4.18) hold true.By Lemma 4.4,we know that K is a bounded,closed and globaluniformly absorbing pullback absorbing set of Φ in V×V.Noting that (4.18) implies (4.17),by (i),we know that Φ is forward-uniformly pullback asymptotically compact in V×V.Thus,by Theorem 2.8 withX=Y=V×V,we can conclude the proof of (iii).
4.3 Asymptotic Stability of Enhanced Pullback Attractors in V×V
Assume that g∈(R,L2(Ω)) andQ ∈(R,L2(Ω)) are asymptotically stable to two given time-independent functions∈L2(Ω) and ˙Q ∈L2(Ω) in the following sense:
Let us consider the autonomous primitive equations defined on Ω fort>0:
with the boundary conditions
and the initial conditions
Our second result is concerned with the forward asymptotic stability of the time-sections of the enhanced pullback attractorsA(t) and A(t) in V×Vast →+∞.
Theorem 4.6(i)If (4.16)and(4.35)hold,then the forward-uniformly compact pullback attractorA={A(t)}t∈Rin(i)of Theorem 4.2 is forward asymptotically stable toA∞in V×V:
(ii)If (4.18)holds,then the forward-uniformly attracting pullback attractor A={A(t)}t∈Rin (iii) of Theorem 4.2 is forwal limit set A∞=:among the collection of sets satisfying
Lemma 4.7Suppose that (4.35) holds.Then Φ is forward asymptotically stable toTfrom V×Vto V×V,that is,if
Therefore,I1(τ) is bounded asτ →+∞.Then,by (4.40),we can complete the proof.
Proof of Theorem 4.6(i) Since conditions (4.16) and (4.35) imply condition (4.17),we know that the results of (i) of Theorem 4.2 hold true.This,together with Lemma 4.7 and Theorem 2.16 withX=Y=V×V,completes the proof of (i).
(ii) This is a direct consequence of (iii) of Theorem 4.2 and Theorem 2.18.
4.4 Enhanced Pullback Attractors in H2×H2
In order to prove the existence of enhanced pullback attractors in H2×H2,we assume the following conditions:
Theorem 4.8(i)If (4.17)and(4.42)hold,then the forward-uniformly compact pullback(V×V,V×V)-attractor in(i)of Theorem 4.2 is just a forward asymptotically compact pullback(V×V,H2×H2)-attractor.
(ii) If (4.17) and (4.42) hold,then Φ has unique forward-uniformly attracting pullback(V×V,V×V)-attractor.
(iii) If (4.17) and (4.42) hold,then Φ has unique forward-uniformly weakly compact and attracting weak pullback (V×V,H2×H2)-attractors in the sense of Definitions 3.1 and 3.2,respectively.
(iv)If (4.18)and(4.42)hold,then the forward-uniformly attracting pullback(V×V,V×V)-attractor in(iii)of Theorem 4.2 is just a forward-uniformly attracting pullback(V×V,H2×H2)-attractor.
The main difficulty to proving Theorem 4.8 is figuring out how to establish the forwarduniform pullback asymptotic compactness of Φ in H2×H2over [t,+∞).To this end,we first derive the uniform estimates of solutions in H2×H2,and then establish the uniform Hölder continuity of solutions to (4.36)–(4.38) from V×Vto H2×H2with respect to the initial data over [t,+∞).The following energy inequalities are borrowed from [33]:
Proposition 4.9The solutions of (4.36)–(4.38) satisfy the energy inequalities
Lemma 4.10Suppose that (4.17) and (4.42) hold.Then for eacht ∈R and B×B ⊆B(V×V),there existsT=T(t,B×B)≥1 such that
ProofFor each fixedt ∈R,we letσ ∈[s-1,s],(vs-ι,Ts-ι)∈B×Bands ≥t.Applying Lemma 4.1 to (4.43),we find from Lemma 4.4 that,for allι ≥T,
Applying 4.1 to (4.44),we find from (4.47) and Lemma 4.4 that,for allι ≥T,
Integrating (4.45) with respect torover (s-1,s) fors ≤t,we infer from Lemma 4.4 that,for allι ≥T,
Applying Lemma 4.1 to (4.46),we find from (4.47),(4.48),(4.49) and Lemma 4.4 that,for allι ≥T,
By [33]we find that
Then the desired result follows from (4.47),(4.48),(4.50) and Lemma 4.4.
Givent ∈R,B×B ∈B(V×V) andi=1,2,we let (vi(σ,s-1,vs-1,i),Ti(σ,s-1,Ts-1,i))withσ ∈[s-1,s]ands ≥tbe two solutions to (4.1)–(4.3).As in [33],we have the following uniform Hölder continuity:
Lemma 4.11For eacht ∈R and B×B ⊆B(V×V),if the conditions in Lemma 4.10 hold,then,for allσ ∈[s-1,s]ands ≥t,there exists a constantM(t,B×B) independent ofssuch that
Proof of Theorem 4.8(i) Let (4.17) and (4.42) hold true.By Lemmas 4.10 and 4.11,we can prove,as in[33],that Φ is forward-uniformly pullback asymptotically(V×V,H2×H2)-compact.Thus,by(i)of Theorem 4.2 and Corollary 2.13 and withX=V×VandY=H2×H2,we can complete the proof of (i).
(ii) Let (4.17) and (4.42) hold true.By Lemma 4.10 and compact Sobolev embedding H2×H2V×V,we know that Φ has a compact forward-uniformly(V×V,V×V)-attracting set.This,together with Theorem 2.4,completes the proof of (ii).
(iii) Let (4.17) and (4.42) hold true.By Lemma 4.10,we know that Φ has a bounded,closed and convex forward-uniformly absorbing pullback(V×V,H2×H2)-absorbing set for Φ.This,along with Theorem 3.4,completes the proof of (iii).
(iv) Let (4.18) and (4.42) hold true.Noting that (4.18) implies (4.17),we know that Φ is forward-uniformly pullback asymptotically (V×V,H2×H2)-compact.Then,by (iii) of Theorem 4.2 and Theorem 2.8 withX=V×VandY=H2×H2,we can conclude the proof of (iv).
Remark 4.12By the argument of Lemma 4.11,we can show that Φ is continuous from V×Vto H2×H2with respect to the initial data.However,we cannot show that Φ is continuous from[s,∞)×V×Vto H2×H2.Therefore,we cannot prove that the forward-uniformly compact pullback(V×V,V×V)-attractor in(i)of Theorem 4.2 is a forward-uniformly compact pullback(V×V,H2×H2)-attractor.
4.5 Asymptotic Stability of Enhanced Pullback Attractors in H2×H2
Theorem 4.13We have the following assertions.
(i) If (4.16),(4.35) and (4.42) hold,then the forward-uniformly compact pullback (V×V,V×V)-attractorA={A(t)}t∈Rin (i) of Theorem 4.2 is forward asymptotically stable toA∞under the topology of H2×H2,that is,
(ii) If (4.18) and (4.42) hold,then the forward-uniformly attracting pullback (V×V,H2×H2)-attractor A={A(t)}t∈Rin (iv) of Theorem 4.8 is forward asymptotically stable to the minimal limit set A∞=:∩t∈RA(t) among the collection of sets satisfying that
Proof(i) This is just a direct consequence of (i) of Theorem 4.6,(i) of Theorem 4.8 and Theorem 2.17 withX=V×VandY=H2×H2.
(ii) This is a direct consequence of (iv) of Theorem 4.8 and Theorem 2.18 withX=V×VandY=H2×H2.
Conflict of InterestBoling GUO is an editorial board member for Acta Mathematica Scientia and was not involved in the editorial review or the decision to publish this article.All authors declare that there are no competing interests.
杂志排行
Acta Mathematica Scientia(English Series)的其它文章
- BIFURCATION CONTROL FOR A FRACTIONAL-ORDER DELAYED SEIR RUMOR SPREADING MODEL WITH INCOMMENSURATE ORDERS∗
- ENTIRE SOLUTIONS OF LOTKA-VOLTERRA COMPETITION SYSTEMS WITH NONLOCAL DISPERSAL∗
- NOTES ON THE LOG-BRUNN-MINKOWSKI INEQUALITY∗
- THE REGULARITY CRITERIA OF WEAK SOLUTIONS TO 3D AXISYMMETRIC INCOMPRESSIBLE BOUSSINESQ EQUATIONS∗
- A DERIVATIVE-HILBERT OPERATOR ACTING ON HARDY SPACES∗
- RELATIVE ENTROPY DIMENSION FOR COUNTABLE AMENABLE GROUP ACTIONS∗
