Generalized uncertainty principle from long-range kernel effects:The case of the Hawking black hole temperature

Rami Ahmad El-Nabulsi and Waranont Anukool

1Center of Excellence in Quantum Technology,Faculty of Engineering,Chiang Mai University,Chiang Mai 50200,Thailand

2Quantum-Atom Optics Laboratory and Research Center for Quantum Technology,Faculty of Science,Chiang Mai University,Chiang Mai 50200,Thailand

3Department of Physics and Materials Science,Faculty of Science,Chiang Mai University,Chiang Mai 50200,Thailand

4Institute of Hydrobiology,Biology Centre of the Czech Academy of Sciences, ˇCeské Budˇejovice,Czech Republic

Keywords: long-range kernel effects,generalized uncertainty principle

Spatial nonlocality has largely been studied in the literature based on various phenomenological approaches.[1,2]It plays a fundamental role in quantum entanglement, where a measurement on one of the entangled particles separated by certain unlimited distances instantaneously modifies the quantum state of the other particle.Theoretically,this modification is due to the change of the density matrix to a pure state.[3]Such a quantum paradox has been solved using the framework of the“many-worlds interpretation”.Although the nonlocal aspect of quantum mechanics has been observed in the well-known Aharonov–Bohm effect through the emergence of global topological features,[4]it is considered one of the strangest properties of the quantum world.Several research papers have been published claiming to solve this fundamental problem, and to prove that quantum nonlocality does not exist (being one of the relics of the Copenhagen interpretation)in which each observed quantity has precisely one value at any instant.[5–8]No matter what the future will reveal for us, we believe that nonlocality is of particular significance in quantum mechanics and should be incorporated into the strange world of quantum theory.From the analytical side,nonlocal effects are usually accompanied with higher-order derivative(HOD)terms in the dynamical equations of motion,e.g.,the Schr¨odinger equation.[9,10]In general,the incorporation of HOD terms in the Schr¨odinger equation may be performed without passing by the nonlocal approach, e.g., using the Doebner–Goldin modification,[11–14]which is limited to the 4th-order term.Nevertheless, several interesting implications and outcomes have been obtained by studying the consequential 4th-order Schr¨odinger equation.[15–19]

The aims of the present study are: first, to construct a quantum diffusion equation by taking into account the longrange kernel effects which are considered in reaction-diffusion theory used in biological systems[20–23]and neutron diffusion processes[24]among others;[25–27]and second, to show that such a kernel approach is associated with the generalized uncertainty principle (GUP), which was introduced to account for the existence of minimal and maximal lengths in nature.[28–36]The presence of a minimum length suggests, in general, that space–time may be fundamentally discrete, and hence, Planck-scale corrections to the basic Heisenberg’s uncertainty principle must be introduced.In fact,one of the main outcomes of the GUP is the modification of the commutation relation between the position and its conjugate momentum,which results in shifting of the energy levels.These modifications create extra terms for all quantum mechanical systems,which could be detected by observers.In general, the GUP reads as[28]

wherexandpare the position and conjugate momentum of a particle with the corresponding quantum observables denoted by ˆxand ˆpβ=β0l2P/¯h2,lPis the Planck’s length, ¯his the Planck’s constant,β0is a constant and, for any operator ˆO,we have ΔO2≡〈ˆO2〉-〈ˆO〉2(mean square deviation)and the bracket denotes averaging over a certain quantum state|φ〉.Recall that, in general, the position and momentum are represented as multiplication or differentiation operators acting on square integrable position-or momentum-space wave functions,φ(x):=〈x|φ〉orφ(p):=〈p|φ〉.The Heisenberg algebra is therefore modified and takes the form

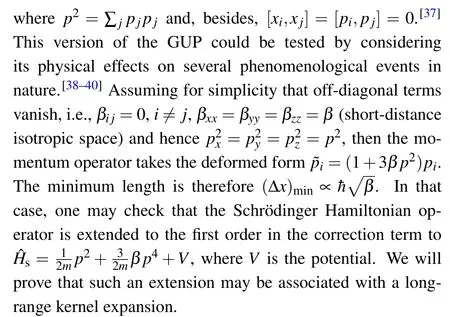
To start,we introduce the following nonlocal Schr¨odinger integro-differential equation:[22,26,41,42]

whereψ(x,t) is the wave function of the particle andK(xx′):R →Ris a symmetric kernel measuring the influence of surrounding neighbors such that

Since the kernel is assumed to be symmetric,then the following integral holds:

Introducing the moments by

we can hence write Eq.(3)as

which may be written as

Hence, the moments of the kernels are associated with the ～βparameter in the framework of the GUP.To illustrate, we select the following Gaussian kernel, although various kernels may be selected:

with(α1,α2,β1,β2)∈R+.In fact,we have considered a noncompactly supported Gaussian symmetric kernel of the formK(x)∝e-x2/b2, where 0＜a ≪1 and 0＜b ≪1, i.e., the long-range effects are weak andK(x) tends to zero quickly.In many situations the restriction to the Gaussian kernel represents a simple approximation; yet,different kernels may be used which are not necessarily symmetric.In general,a Gaussian kernel function is used to describe quantum nonlocal diffusion processes.[26]Recall that equation (3) is comparable to a nonlocal diffusion equation with imaginary time.If the kernel has a second moment, then the Cauchy condition in 1Dholds;yet,by symmetry,the associated first moment is zero.The Fourier transform of the kernel ˆK(w)=F.T.(K(x))accordingly takes the asymptotic form(w →0)and the form ˆK(w)≈1-|w|2+O(|w|2).Hence,the nonlocal Schr¨odinger diffusion equation obeys the Gaussian lawK(x)= e-x2.If,for instance,we consider a more general expansion of ˆK(w)of the form ˆK(w)≈1-c|w|α+O(|w|α),c ＞0,0＜α ＜2.This special form leads,in general,to a nonlocal fractional Laplace parabolic equation.[43]If we choose ˆK(w)≈1+cw2lnw,c ＞0,then it has been proven in[43]that the asymptotic behavior is still given by a solution of the diffusion equation,yet is viewed in a different time scale.Although asymmetric kernels are very common in nonlocal diffusion and dispersal processes,[44]they deserve to be considered in quantum mechanics.This will be discussed in a forthcoming work.

After simple integration,we find

The constraintsM2＞0 andM4＜0 are verified if

and

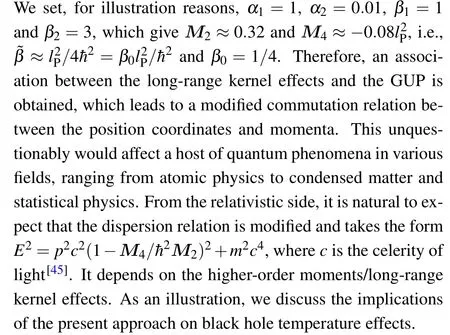
In fact, it has been argued that, at large scales, the GUP takes the subsequent form[46]

This leads to a generalized Hawking’s temperature for evaporating black holes,which is given by

We observe that, forM4/M2=whereγis a real parameter,equation(20)is reduced to

and therefore,forM ≥γMP,we getT ≈MPc2/4πγ.For high values of the parameterγ,the temperature of the black hole is less thanMPc2,e.g.,exotic cold black holes.[47]For the Gaussian kernel(13)withM2≈0.32 andM4≈-we find

and hence, forM ≥MP/2,T ＜Mc2.This is the temperature of the black hole remnants.[48,49]One may select different numerical values, which may give rise to lower black hole temperatures.

To conclude, we have proved that the quantum diffusion equation obtained through the framework of“long-range kernel effects” may be correlated to the Generalized Uncertainty Principle predicted from quantum gravity effects.This is in agreement with the approach introduced in Refs.[50,51],where quantum gravity states may remain coherent at very large scales and they could play a critical role in the understanding of macroscopic gravitational effects.This correlation between the GUP and long-range effects may have interesting consequences in several fields, including higher-dimensional black holes,[52]cosmology,[53]dark energy[54,55]and quantum geometry[56,57]among others.[58–64]A variable family of nonnegative integrable kernels may be chosen which are not necessarily symmetric, which may give rise to various phenomenological quantum effects.Work in these directions is under construction.