High-performance chiral all-optical OR logic gate based on topological edge states of valley photonic crystal

Xiaorong Wang(王晓蓉), Hongming Fei(费宏明),†, Han Lin(林瀚), Min Wu(武敏), Lijuan Kang(康丽娟),Mingda Zhang(张明达), Xin Liu(刘欣), Yibiao Yang(杨毅彪), and Liantuan Xiao(肖连团)

1College of Physics,Taiyuan University of Technology,Taiyuan 030024,China

2Key Laboratory of Advanced Transducers and Intelligent Control System,Ministry of Education,Taiyuan University of Technology,Taiyuan 030024,China

3School of Science,RMIT University,Melbourne,Victoria 3000,Australia

Keywords: topological photonics,topological edge state,valley photonic crystal,all-optical logic gate

1.Introduction

Due to the development of augmented reality(AR)/virtual reality (VR) and big data, the increase in information traffic demands large bandwidth and reliable information processing.Optical communication and information processing have the advantages of large bandwidth,fast speed,high energy efficiency, and low heat generation.An all-optical network[1]is that the signal always exists in the form of light during the propagation and information processing, which overcomes the”electronic bottleneck”phenomenon caused by the previous optical-electrical-optical conversion.[2]It is necessary to develop an entire family of different all-optical components for all-optical networks, such as waveguides, optical switches,[3–8]wavelength division multiplexing (WDM)devices, optical delay lines, logic gates, and so forth.Currently, information processing is realized by electrical logic gates (AOLGs) based on electro-optic conversion all the time[9,10]due to the limited performance of current AOLG designs that cannot meet the requirements in applications.As a result, the speed is ultimately limited by the relatively slow electric logic gates.Therefore, designing high-performance AOLGs has become a stringent request and is key to realizing all-optical information processing.

An ideal logic gate performs operations on Boolean values, which should dissipate no power and change states instantaneously, similar to a step function.Such gates operate on discrete logic inputs and should have no propagation delay.For AOLGs, the propagation delay is neglectable due to the high speed of light.In addition,power dissipation can be minimized by achieving high transmittance.Furthermore,for photonic integrated circuit (PIC) operation, AOLGs should have a miniaturized footprint for high-density integration.In general,AOLGs that work with different spin states of photons are required for quantum computing.Therefore, AOLGs should have chiral responses to work with spin-up(right-handed circularly polarized,RCP)and spin-down(left-handed circularly polarized,LCP)photons.

Many designs of AOLGs based on different working principles have been demonstrated, such as semiconductor optical amplifier (SOA),[11–14]periodically poled lithium niobate(PPLN)waveguide,[15,16]ring resonators,[17–19]and photonic crystals(PCs).[20–22]The performance of SOA-based AOLGs is limited by spontaneous emission noise and high integration complexity.In comparison, the AOLGs based on semiconductor ring resonators have the advantages of simplicity and low input power.But the speed is slow.Meanwhile,the AOLGs based on PPLN waveguides have fast switching speeds and low spontaneous emission noise.However,those AOLGs strongly depend on the working temperature and the incident light’s polarization states,which hinder their applications.All the AOLGs mentioned above have relatively large footprints,which makes them unsuitable for highdensity on-chip integration.In comparison, the footprint of AOLGs can be significantly reduced by using nanostructures,in which PC structures[23–32]are the most widely used.Different effects and principles of PC structures have been applied to design AOLGs, such as self-collimation,[33,34]multimode interference,[35,36]interference waveguides,[37,38]and nonlinear effects.[39,40]However, all the designs above have relatively low transmittance due to the scattering loss introduced by structural defects.In addition,none of the AOLG designs,including all conventional and PC designs,can work with circularly polarized light(CPL)to maintain the spin states,which is important for quantum computing.[41–43]

The recent development of topological photonic crystals(TPCs)[44–50]allows defect-immune unidirectional transmission of topological edge states, which opens new possibilities in designing photonic devices.Among different TPC designs, valley photonic crystals(VPCs)can be achieved based on dielectric materials without an external magnetic field,thus pushing the working wavelength to the telecommunication and the visible region.[51,52]Here based on the unique spin-valley locking effect of VPCs, we design a high-performance integratable all-optical logic OR gate based on the tunable topological edge states of VPCs.We tune the working bands of the two input channels to minimize their interference,achieving stable and high-fidelity outputs.The spin-valley locking effect allows robust unidirectional transmission of CPL.The transmittance of both channels is higher than 0.8, which meets the requirements of an ideal all-optical logic gate.In addition, an ultrahigh contrast ratio of 28.8 dB is achieved.Currently, the contrast ratio of AOLGs based on conventional PC structures is mainly in the range of 10 dB–20 dB.So our design has a much higher contrast ratio than those AOLGs in recent references.[37]Furthermore, the chiral responses of the AOLGs allow using CPL with different handedness as logic inputs (logic “1” or logic “0”), which meets the requirements of quantum computing.[41–43]The footprint of the entire AOLG device is as small as 18 µm×12 µm,allowing high-density on-chip integration.The device can be manufactured by the mature complementary metal–oxide–semiconductor (CMOS) nanofabrication technique and will find broad applications in optical information processing and quantum computing.

2.Design of the all-optical OR gate

An OR logic gate (symbolized by “‖” or “+”) outputs a logic“1”if one or both the inputs are logic“1”; otherwise,it outputs a logic“0”.Here,the logic“1”in an AOLG is defined as the input or the output having an incident or transmitted optical wave with a transmittance higher than 0.7.And the logic“0”is determined when the criterion is not met.According to this definition, an all-optical OR gate based on a VPC structure is designed and shown in Fig.1(d).The gate comprises two mirror-symmetrical VPC structures,consisting of two input waveguides (INA and INB) and two output waveguides(OUT1 and OUT2).To minimize the interference between the two inputs, we tune the working bands of INA (centered atλ1,figures 1(a)and 1(c))and INB(centered atλ2,figures 1(b)and 1(c)) by adjusting the radii of the lattice at the boundary(highlighted by different colors in Fig.1(d)).Meanwhile,the working band of the output waveguide OUT1 can cover the two working bands of INA and INB.In contrast,the OUT2 is designed to have extremely low transmittance in those working bands to prevent the undesired loss through the waveguide to maximize the transmittance through OUT1.The schematics in Fig.1 show the four possible cases of the AOLG, namely 1‖0=1, 0‖1=1, 1‖1=1, and 0‖0=0.The two numbers on the left side correspond to the inputs from INA and INB,respectively.The number on the right side shows the output of OUT1.

Fig.1.The working diagrams of an all-optical OR logic gate: (a) light inputs only from INA, as 1‖0=1; (b) light inputs only from INB, as 0‖1=1; (c) light inputs from both INA and INB, as 1‖1=1; (d) light inputs from neither INA nor INB,as 0‖0=0.

The design process started with creating a honeycomb PC structure(Fig.2(a))composed of circular air holes embedded in a free-standing silicon substrate.The refractive index of silicon is shown in supporting information S1.The thickness of the free-standing silicon substrate(the dispersive refractive index of pure silicon is used) ish=220 nm.The unit cell of this structure is composed of two sets of air holes (A and B)with the same radius(rA=rB=80 nm),which has aC6Vrotational symmetry.The lattice constant isa=450 nm.Here we use commercial three-dimensional (3D) time–domain finite difference(FDTD)software(Lumerical FDTD Solutions)to calculate the photonic band structure.The band diagram of the transverse electric(TE)mode is shown in Fig.2(c)by the dashed lines,which show a Dirac point.Then theC6Vsymmetry is reduced toC3symmetry by simultaneously increasingrA(to 120 nm) and decreasingrB(to 40 nm) to introduce a topological photonic bandgap(1410 nm–1676 nm)due to the degeneracy of theKandK′valleys,indicated by the blue dot lines in Fig.2(c).In this way, VPC1 is designed (left panel in Fig.2(b)),which can be converted to VPC2(right panel in Fig.2(b)) by applying mirror symmetry operation.The values of the Berry curvature and topologically invariant valley Chern numberCVcorresponding to the two VPCs were calculated,which areCV=−1 for VPC1 andCV=1 for VPC2,[51]respectively.

Fig.2.Structure parameters and photonic band diagrams of PCs.(a)3D schematics of the honeycomb PC structure;(b)3D schematics of VPC1 and VPC2;(c)the photonic band diagram of the honeycomb PC(red dot line)and VPC1(blue dot line),the blue shaded area marks the bandgap;the gray region marks the air cone.

3.Tuning of topological edge states

There are two possible types of boundaries, the zigzagshape and beard-shape boundaries, which can support topological edge states.The study of the edge states of the two boundaries shows that the working bandwidth of the beardshape boundary is narrower than the bandgap, which allows tuning the position of the working bands within the bandgap.The detail is shown in supporting information S2.In this study,we choose the beard-shape boundary as required to achieve different working bands within the bandgap.Depending on the small or large circles at the boundary,two kinds of beard-shape boundaries can be constructed: S-interface and L-interface.Due to the high transmittance of the L-interface, it is chosen for INA, INB, and OUT1.Figure 3(a) shows the schematic of a straight waveguide using the L-interface boundary.We define the radius of the large air holes at the boundary asre.By tuning there,the working band of each waveguide can be controlled.As shown in Fig.3(b),the decrease ofre(120 nm,116 nm,110 nm)results in a redshift of the working bands due to the increased effective refractive index of the edge states.

We performed numerical simulations on 11 sets of straight waveguides using RCP incident light, withregradually decreasing from 120 nm to 110 nm at a step of 1 nm.The transmittance spectra are shown in Fig.3(c).The tuning aims to separate the working bands of INA and INB to minimize interference and achieve a stable output.According to the definition of logic “1”, the working band is defined as where the forward transmittance is higher than 0.7(TF> 0.7).The plot of the working bandversusdifferent radii is shown in Fig.3(d).The working band is quite sensitive to the radius change,which presents a linear relationship withre(the slope is 5.792).Therefore, whenredecreases by 1 nm, the central wavelength of the working band increases by 5.792 nm.We find threerevalues meeting our requirements,which arere=120 nm(the working band is 1476 nm–1512 nm),re= 116 nm (the working band is 1489.7 nm–1542 nm), andre=110 nm (the working band is 1522 nm–1582 nm),respectively.As one can see,the working bands in the cases ofre=120 nm andre=110 nm can be well separated.Meanwhile,the working band in the case ofre=116 nm overlaps with bothre=120 nm andre=110 nm.Therefore,we user1=120 nm for INA andr2=110 nm for INB, respectively.Meanwhile,r3=116 nm is used for OUT1.As a result, inputs from INA and INB can highly efficiently transmit through OUT1.In addition,to minimize the light leakage from OUT2,the air holes at the boundary are removed.Thus,the transmittance of OUT2 approaches zero in the range of 1450 nm–1530 nm.The detail is shown in supporting information S3.

We design Z-shape waveguides with INA or INB combined with OUT1 to study the transmission properties of different combinations.The schematics of the Z-shape waveguides are shown in Figs.4(a)and 4(b),respectively.The green and red circles in the Z1 and Z2 waveguides (Figs.4(a) and 4(b)) haver1=120 nm andr2=110 nm, respectively.The purple circles in both waveguides have anr3=116 nm.The transmittance spectra are plotted in Fig.4(e).Here we choose two working wavelengths for INA and INB to achieve the function of an AOLG, which areλ1= 1490 nm andλ2=1528 nm (marked by the dashed lines in Fig.4(e)), respectively.Those wavelengths are chosen due to the high transmittance (TF>0.75).The intensity distributions in the Z-shape waveguides at the two working wavelengths in Figs.4(c)and 4(d) show that the light waves are well confined within the waveguides.

Fig.3.(a)A schematic diagram of a straight waveguide based on the L-interface boundary(the big circles in the purple area are the air holes at the boundary, and the radius is re).(b) Band diagram of the valley-dependent edge states (the green, purple, and red lines correspond to re =120 nm,re =116 nm,and re =110 nm,respectively).(c)Transmittance spectra of straight waveguides with different re.(d)The plot of working band versus different re.

Fig.4.Z-shape waveguide designs and transmittance.(a) Schematic diagram of Z1 waveguide composed of INA and OUT1.(b) Schematic diagram of Z2 waveguide composed of INB and OUT1.(c)The electric field intensity distributions in Z1 waveguide at λ1=1490 nm.(d)The electric field intensity distributions in Z2 waveguide at λ2=1528 nm.(e)Transmittance spectra of the two Z-shape waveguides.

4.Performance analysis of the designed alloptical OR gate

We then combine the two Z-shape waveguides to form the all-optical OR gate,shown in Fig.5(a).The boundary conditions of FDTD are PML in thex–ydirection and symmetric in thezdirection.The input light source is RCP, a superposition of two dipole sources.The transmittance spectra of the AOLG are shown in Fig.5(b), in which one can see different input cases from INA(1‖0),INB(0‖1),and INA+INB(1‖1).In all three cases, the output from OUT1 has a transmittance higher than 0.7 at eitherλ1=1490 nm orλ2=1528 nm.The corresponding intensity distributions are plotted in Figs.5(c)–5(f).When the light waves at the designed working wavelengths are input from INA(Fig.5(c))and INB(Fig.5(d))separately,they can highly efficiently transmit through the AOLG.The transmittances are 0.86 and 0.90 at the wavelengths ofλ1=1490 nm orλ2=1528 nm, respectively.In comparison, due to the well-separated work bands of INA and INB,when light waves at the two wavelengths are input from both INA and INB (1‖1) (Figs.5(e) and 5(f)), they do not interfere.Because the light waves atλ1=1490 nm can not transmit through INB.Similarly, the light waves atλ2=1528 nm have very low transmittance in INA.Here we notice that there is a small portion of light transmitting from one input to the other input (for example, from INA to INB atλ1=1490 nm and from INB to INA atλ2= 1528 nm) at the junction of INA, INB, and OUT1 (shown in Figs.5(c) and 5(d)).However,this effect does not influence the overall performance of the AOLG,as shown in Table 1.

Table 1.All-optical logic or gate truth table.

Fig.5.The designed AOLG and its function.(a) 3D structural diagram of the ALOG gate.(b) Transmittance spectra of the AOLG in three different cases.Electric field intensity distributions at the wavelength of λ1=1490 nm input from INA alone.(c)(1‖0=1)and both INA and INB(e)(1‖1=1),respectively.Electric field intensity distributions at the wavelength of λ2=1528 nm input from INB alone.(d)(0‖1=1)and both INA and INB.(f)(1‖1=1),respectively.

The performance of the AOLG can be further studied by using the logic gate contrast function,which is defined as

whereP1andP0are the transmittances of the output and the unexcited input,respectively.Most applications desire a high contrast ratio,which is challenging based on reported designs.The contrast ratio curves are shown in Figs.6(a)and 6(b)for the inputs from INA and INB, respectively.The wavelength ranges are decided according to the working bands of INA and INB.The contrast ratios are 28.8 dB atλ1=1490 nm in INA and 20.5 dB atλ2=1528 nm in INB,which are much higher compared to state-of-the-art AOLGs.[34,36,37,40]

To show the advantages of using two well-separated working bands for the two inputs, we also perform the simulation on the logic gate composed of identical straight waveguides(details in supporting information S4).In this case, the transmittance in each case is relatively lower compared to the separate design.That is due to the light transmitting from one input (INA or INB) to the other (INB or INA).Because both INA and INB can support the same edge states.The situation can be avoided by using different working bands for INA and INB, as demonstrated in this work.In this way, the forward transmittance of each input can be maximized,which is essential for any AOLGs for quantum computing since single photon transmission is necessary.Further,to demonstrate our design can work with different spin states(LCP or RCP light)due to the spin-valley locking effect, we perform the simulation on the input with different handedness (details in supporting information S5).Here LCP and RCP light is defined as logic “1” and “0”, respectively.The output transmittance(TF>0.7) is still designated as logic “1”.Due to the spinvalley locking effect, the LCP and RCP light propagates in opposite directions, resulting in high and low transmittances corresponding to the logic“1”and“0”states,respectively.In this way, the chirality of the logic gate allows using different spin states as different logic states, which is achieved among various AOLGs based on PC structures for the first time to the best of our knowledge.

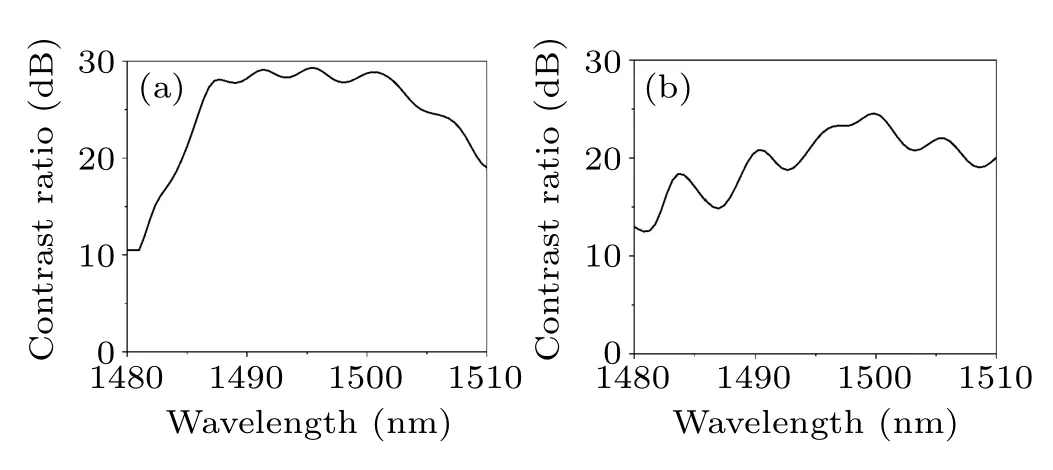
Fig.6.Contrast ratio plots when light is only input from INA(a)and INB(b).

In order to demonstrate the unique defect immune transmission property of the our topological OR gate structures,we simulate the light transmission in the topological waveguides and the logic gates with defects, which could be introduced by fabrication errors.Here we simulated two types of defects: (i) Missing air holes in the waveguide (as shown in Fig.S7(a)in supporting information);(ii)Changing the radius of air holes(as shown in Fig.S7(b),10%reduction in radius).The resulting transmittance spectra are shown in Fig.S7(c),in which only slight changes in the transmittance spectrum are observed,confirming the robustness of our design(details in supporting information S6).This property demonstrates high feasibility in the experimental realization of the designed structures.

5.Conclusion and perspectives

We have demonstrated a high-performance AOLG design based on a VPC structure.By tuning the working bands of the two inputs, we minimize the interference and cross-coupling between the inputs to ensure high transmittance output (up to 0.9) and high contrast ratio (up to 28.8 dB).Moreover,the chiral response of the logic gate due to the spin-valley locking effect allows using different spin states as inputs for quantum computing.The device’s footprint is as small as 18µm×12µm,which is suitable for high-density on-chip integration.In addition,the device is compatible with the CMOS nanofabrication technique.The design principle can be generally applied to design other photonic devices based on VPCs for broad applications.

Acknowledgments

Project supported by the National Key Research and Development Program of the Ministry of Science and Technology of China (Grant No.2022YFA1404201), the National Natural Science Foundation of China (Grant No.11904255),and the Key Research and Development Program of Shanxi Province (International Cooperation) (Grant No.201903D421052).