ARBITRARILY SMALL NODAL SOLUTIONS FOR PARAMETRIC ROBIN (p,q)-EQUATIONS PLUS AN INDEFINITE POTENTIAL∗

Salvatore LEONARDI

Dipartimento di Matematica e Informatica, Viale A. Doria, 6 95125 Catania, Italy E-mail: leonardi@dmi.unict.it

Nikolaos S. PAPAGEORGIOU

Technical University, Department of Mathematics, Zorografou Campus, Athens 15780, Greece E-mail: npapg@math.ntua.gr

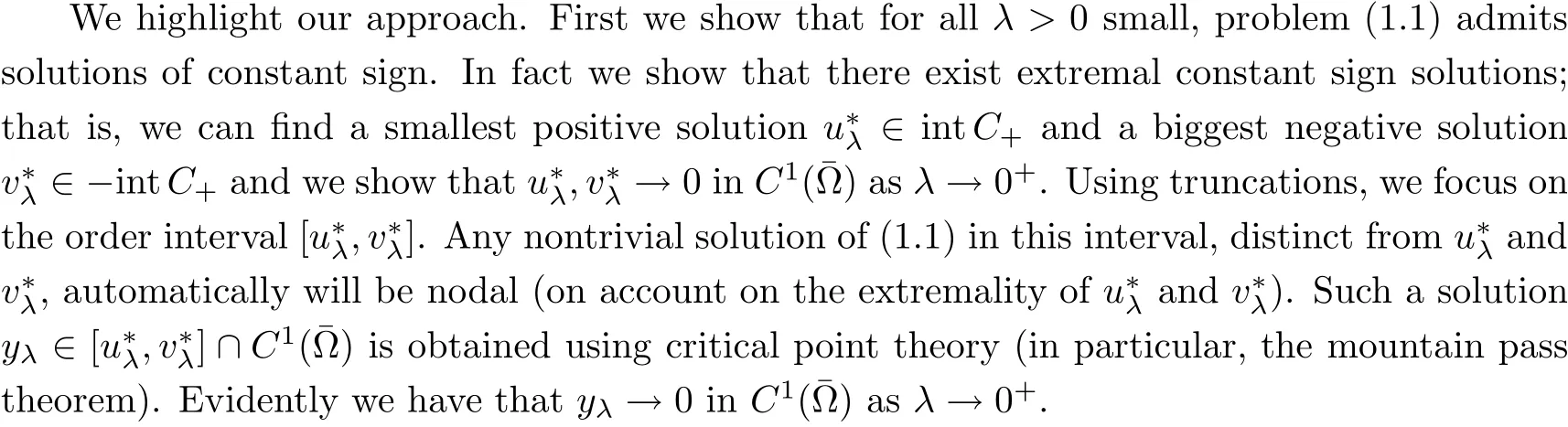
Abstract We consider a nonlinear Robin problem driven by the (p,q)-Laplacian plus an indefinite potential term and with a parametric reaction term. Under minimal conditions on the reaction function, which concern only its behavior near zero, we show that, for all λ>0 small, the problem has a nodal solution yλ ∈C1()and we have yλ →0 in C1()as λ →0+.

Key words (p,q)-Laplacian; indefinite potential; nonlinear regularity; extremal constant sign solutions; nodal solutions

1 Introduction

Let Ω ⊆RN,N ≥3,be a bounded domain with a C2-boundary ∂Ω. In this paper we study the following parametric Robin (p,q)-equation:

So in problem (1.1), the differential operator is the sum of two such operators. Hence it is not homogeneous. There is also a potential term u →ξ(z)|u|p-2u with the potential function ξ(z)∈L∞(Ω) being sign changing.

Therefore the left-hand side of problem (1.1) is not coercive.

The reaction term λf(z,x) of (1.1) is parametric, with λ > 0 being the parameter, and it is a Carath´eodory function (that is, for all x ∈R the function z →f(z,x) is measurable and for a.a. z ∈Ω the function x →f(z,x) is continuous).

The only global requirement on f(z,·) is that it has subcritical growth (this way, we can guarantee that the energy functional of the problem is C1). Otherwise, all the other conditions on f(z,·) are local and concern its behavior near zero. These conditions are minimal and do not include any symmetry assumption on f(z,·). This fact prohibits the use of any variant of the symmetric mountain pass theorem.

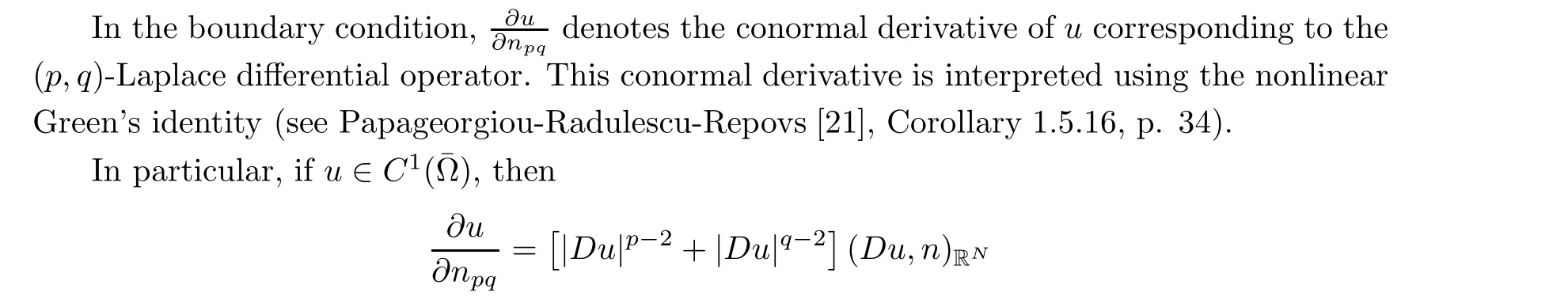
with n(·) being the outward normal on ∂Ω.

Using variational tools based on the critical point theory,together with suitable truncation and comparison techniques and use of critical groups(Morse theory), we can show that, for all λ > 0 small, problem (1.1) has a nodal (sign changing) solution yλ∈C1() and yλ→0 in C1(as λ →0+.

Recently Papageorgiou-Vetro-Vetro [23] (semilinear equations), Papageorgiou-Radulescu[18]and Papageorgiou-Radulescu-Repovs[20](nonlinear equations)proved similar results under a symmetry condition on f(z,·). An analogous result, without any symmetry assumption, can be found in Leonardi-Papageorgiou [12]. Here the reaction contains a perturbation of the parametric term and the condition on the potential function ξ(·) involves the coefficient k(·)of the perturbation (see hypothesis H0in [12]). When there is no perturbation term (that is k ≡0), as in the case here, hypothesis H0of[12] implies that the potential function ξ(·) in [12]is strictly positive and bounded away from zero. This makes the left-had side coercive.

So, the present setting is more general since our potential term is indefinite.

Finally,we mention that boundary problems involving the sum of two differential operators of different nature arise in many mathematical models of physical processes. We refer to the works of Bahrouni-Radulescu-Repovs[1],Benci-D’Avenia-Fortunato-Pisani[2],Cherfils-Ilyasov[3] and the references therein.

To have a complete overview on the literature, one can refer also to the relevant papers[7, 22]. For other kind of operators with lower order terms, see also [9-11, 13]

2 Preliminary Results. Hypotheses

The main spaces involved in the study of problem(1.1)are the Sobolev space W1,p(Ω), the Banach space C1() and the boundary Lebesgue spaces Ls(∂Ω), 1 ≤s ≤+∞.

admits a strongly convergent subsequence”.

This is a compactness type condition on the functional φ(·), which compensates for the fact that the ambient space X is not locally compact (being in general infinite dimensional). The C-condition leads to a deformation theorem from which one can derive the minimax theorems of the critical point theory. For details we refer to Papageorgiou-Radulescu-Repovs[21], Chapter 5.

with U being a neighborhood of u such that Kφ∩φc∩U ={u}.

The excision property of singular homology implies that the above definition of critical groups is independent of the choice of the isolating neighborhood U.

Next we introduce our hypotheses on the data of problem (1.1).

H0: ξ ∈L∞(Ω), β ∈C0,α(∂Ω)with 0 ＜α ＜1, β(z)≥0 for all z ∈∂Ω and ξ /≡0 or β /≡0.

Remark 2.2 Evidently the case β ≡0 is included and corresponds to the Neumann problem.

Remark 2.3 Evidently the conditions on f(z,·) are minimal and basically concern its behavior near zero.

with 1 ＜τ ＜q ＜p.

Invoking Proposition 2.10 of Papageorgiou-Radulescu[17],we have that∈L∞(Ω). Then the nonlinear regularity theory of Lieberman [14] implies that∈C+{0}. In fact via the nonlinear maximum principle of Pucci-Serrin [24] (pp. 111, 120), we have∈int C+.

Next we show the uniqueness of this positive solution. To this end, we consider the integral functional i:W1,p(Ω)→R ∪{+∞} defined by

This proves the uniqueness of the positive solution∈intC+.

3 Nodal Solutions

Next, we show that there exist extremal constant sign solutions

Proposition 3.3 If Hypotheses H0,H1hold and λ ∈]0,λ∗[, problem (1.1) has a smallest positive solution u∗λ∈intC+and a biggest negative solution v∗λ∈-intC+.

Proof From Papageorgiou-Radulescu-Repovs [19] (see the proof of Proposition 7), we know that the set S+λis downward directed. Then by Lemma 3.10,p. 178,of Hu-Papagerogiou[8], we can find a decreasing sequence {un}n≥1⊆S+λsuch that