基于焓耗散数的绝热型溶液除湿/再生器性能预测解析模型

彭冬根, 曹 卓

彭冬根, 曹 卓

(南昌大学 工程建设学院, 江西 南昌 330031)

1 前言

溶液除湿是一种利用表面水蒸气分压力较低的水溶液吸收空气中水分的除湿方法，由于驱动能源品味要求低[1-3]、可以改善室内空气品质[4]等优势被研究人员所关注。在除湿过程中，吸湿性溶液与湿空气直接接触进行水分与热量的传递[5]，因此溶液除湿过程是一个传热与传质相耦合的过程[6-8]，给除湿/再生器数学模型建立及求解造成困难。

Lazzarin等[9]分析并建立了绝热型填料塔内空气与溶液之间传热传质的一维模型。代彦军等[10]建立除湿/再生器内叉流降膜除湿的传热传质过程数学模型。Varela等[11]通过雷诺-普朗特数和雷诺-施密特数给出溶液除湿/再生过程中传热传质的一般关系式。Ren等[12]在常见的溶液除湿/再生工况下将溶液平衡湿度比与温度的关系进行线性逼近。Babakhani等[13]给出可以预测各种变量在再生器内分布情况的解析解。Zhang等[14]基于传质单元数(NTUm)模型分析了热泵驱动的溶液除湿系统性能。Liu等[15]在NTUm的基础上提出用效率表示的除湿/再生器解析模型。上述研究从传热传质角度发展了除湿/再生器数学模型，但皆未从装置不可逆性的角度进行建模，无法分析装置不可逆性程度对除湿/再生器性能影响。

式中：Q=mcT，为物体质量，kg；c为物体的比定容热容，kJ×kg-1×K-1；为热力学温度，K；Q为物体所含的热能，kJ。

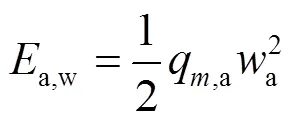
式中：q,a为干空气的质量流量，kg×s-1；a为湿空气中水蒸气与干空气的质量比。

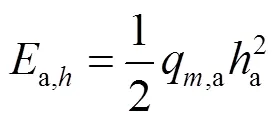
式中：q,s为溶液质量流量，kg×s-1；eq为与溶液表面平衡时的空气的等效比焓，kJ×kg-1；s为溶液比焓，kJ×kg-1；

为方便后文解析推导，在分析溶液与空气的热质交换时作如下假设：

1) 由于除湿/再生过程水分迁移率和溶液流率相比较小，假设溶液流量不变；

2) 忽略除湿/再生介质与环境之间散热损失，假设除湿/再生器为绝热；

3) 不考虑除湿/再生器内可能存在的局部积液及局部涡流现象，假设溶液与空气在填料表面均匀分布；

4) 不考虑溶液和空气沿与其流动垂直方向的热湿传递，假设顺逆流除湿/再生过程为一维模型；

5) 由于除湿/再生温湿度变化较窄，假设溶液和空气物性参数为常数；

6) 只考虑溶液与空气的热质交换最终处于稳态的结果，假设除湿/再生过程为稳态。

以逆流除湿过程为例，微元面积内的传热传质过程如图1所示，图中q,a,in、q,s,in分别为进口空气和溶液的质量流量，kg×s-1；a,in、a,out、s,in和s,out分别为进、出口空气和溶液的比焓，kJ×kg-1。

根据2.2节假设，可以得到逆流除湿过程的能量守恒微分方程如下：

积分得

式中：为在微元面积上传递的全热量，kW。

将式(6)代入式(8)得

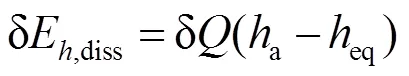
为方便计算，在此定义系数，如式(11)所示：

式中：eq,in和eq,out分别为与进、出口溶液平衡的空气比焓，kJ×kg-1。

将式(11)代入式(8)并在整个除湿/再生器的面积上进行积分，得到

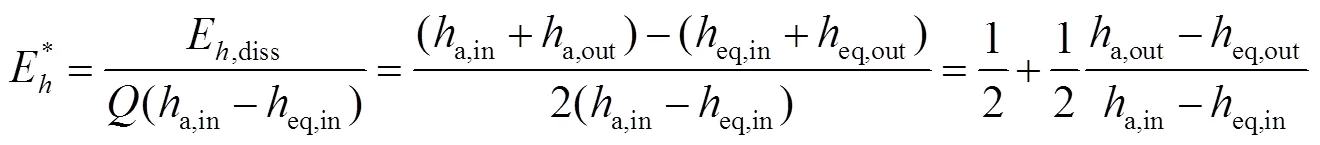
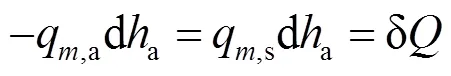
对方程(15)左侧进行积分得

在除湿/再生器中，其焓效率定义为

定义*为与溶液平衡状态下空气与溶液之间的等效热容比，如下：

式中：c,s为溶液的比定压热容，kJ×(kg×K)-1；c,eq为与溶液平衡时的空气的比热容，kJ×(kg×K)-1。

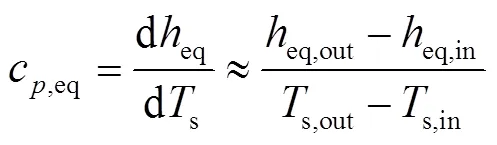
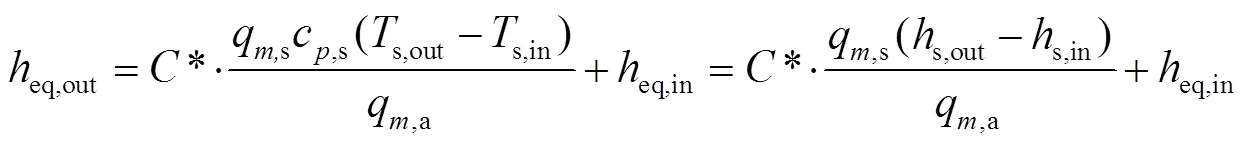
将式(20)与(17)代入式(13)可得

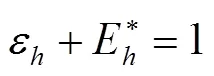
图2 除湿/再生器中焓耗散数Eh*与焓效率eh的关系

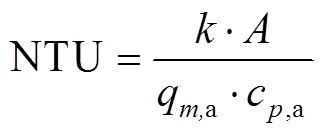
式中：为溶液与空气之间的传热系数，kW×(m2×K)-1；c,a为空气的比定压热容，kJ×(kg×K)-1。

在除湿/再生器中，NTU与焓效率的关系如式(23)和(24)所示[15,30]，且在形式上分别与文献[29]中顺流、逆流换热器的NTU和换热效率的关系式一致：

顺流：

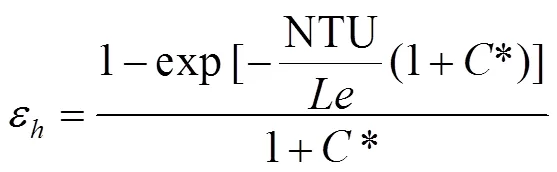
逆流：

式中：为溶液和空气间传热传质的路易斯数(近似为1[28,30])

顺流：

逆流：

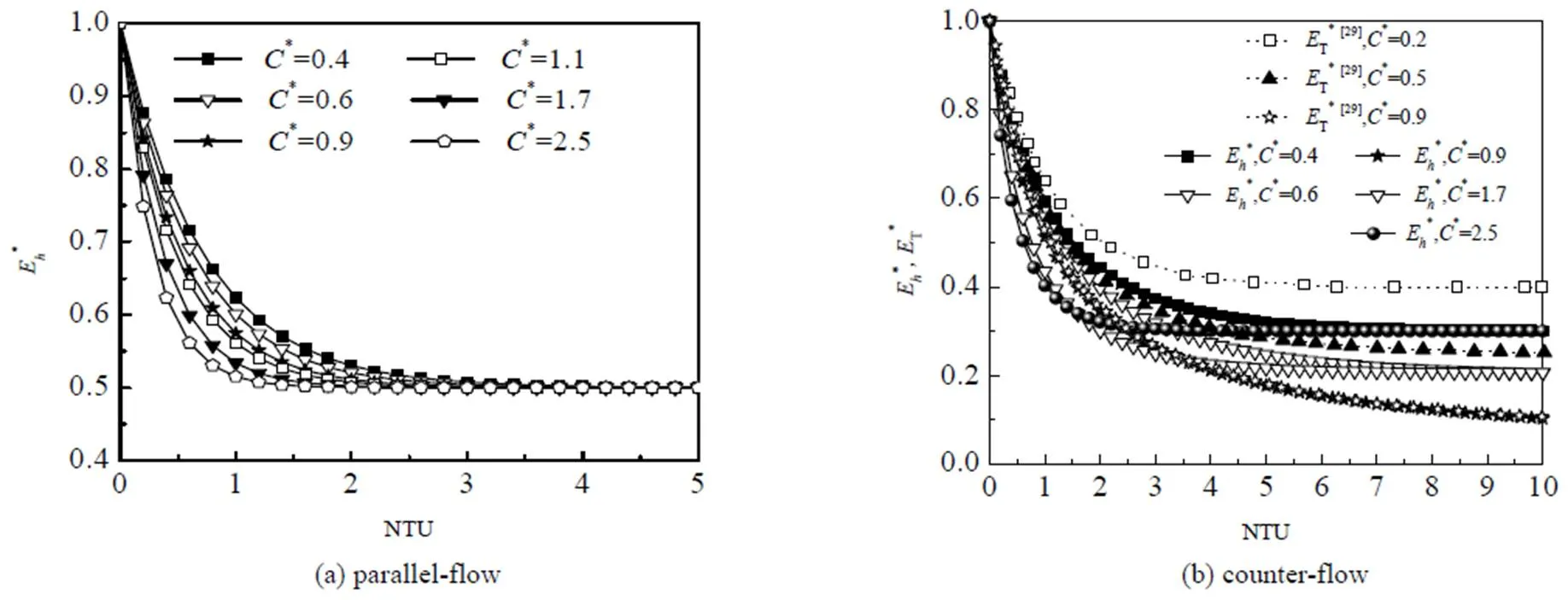
图3 除湿/再生器中焓耗散数Eh*与传热单元数NTU的关系

溶液与空气之间的能量守恒方程见式(7)。

溶液与空气之间的质量守恒方程为

式中：a,in、a,out分别为进口、出口湿空气中水蒸气与干空气的质量比；s,in、s,out分别为除湿/再生器进、出口溶液中溶质与溶液质量比。

传热系数和传质系数m可分别由空气侧的显热、含湿量和焓的增量方程决定：

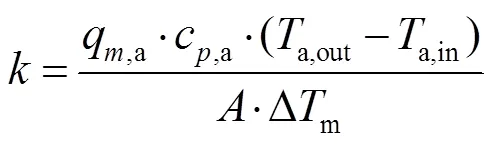
式中：a,in和a,out分别为进口、出口的空气热力学温度，K；Δm为对数平均温差，K。

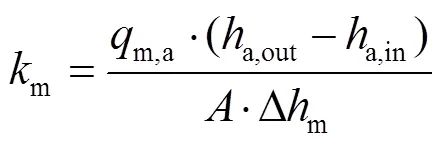
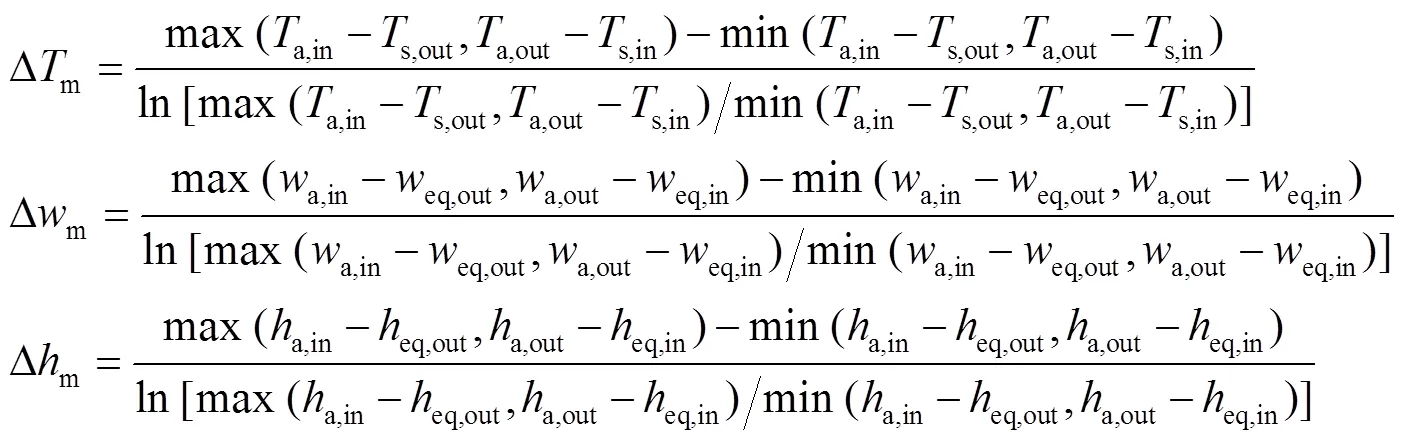
式中：eq,in、eq,out分别表示与进口、出口溶液湿热平衡的湿空气中水蒸气与干空气质量比。

对于含湿量或温度出现交叉的情况而言，采取以下选择策略：

1) 当溶液与空气之间的温度不存在交叉时，联立方程(28)、(29)与(31)可以得到方程(33)：

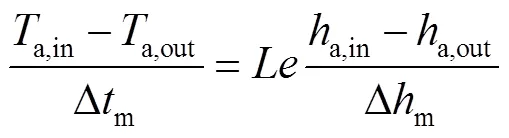
2) 当溶液与空气之间的温度存在交叉时，联立方程(30)和(31)得

联立方程(7)、(13)、(28)和(34)可以通过除湿/再生器工质进口状态计算得到其出口状态。

5 解析模型验证

为了验证除湿/再生器解析模型的精确性，本研究将该解析模型计算结果与数值模型计算结果及实验结果分别进行了对比及分析误差。

5.1 数值模型验证

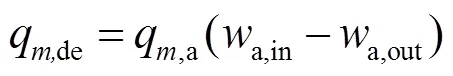
气液流量比定义为

表1 除湿/再生器基准工况

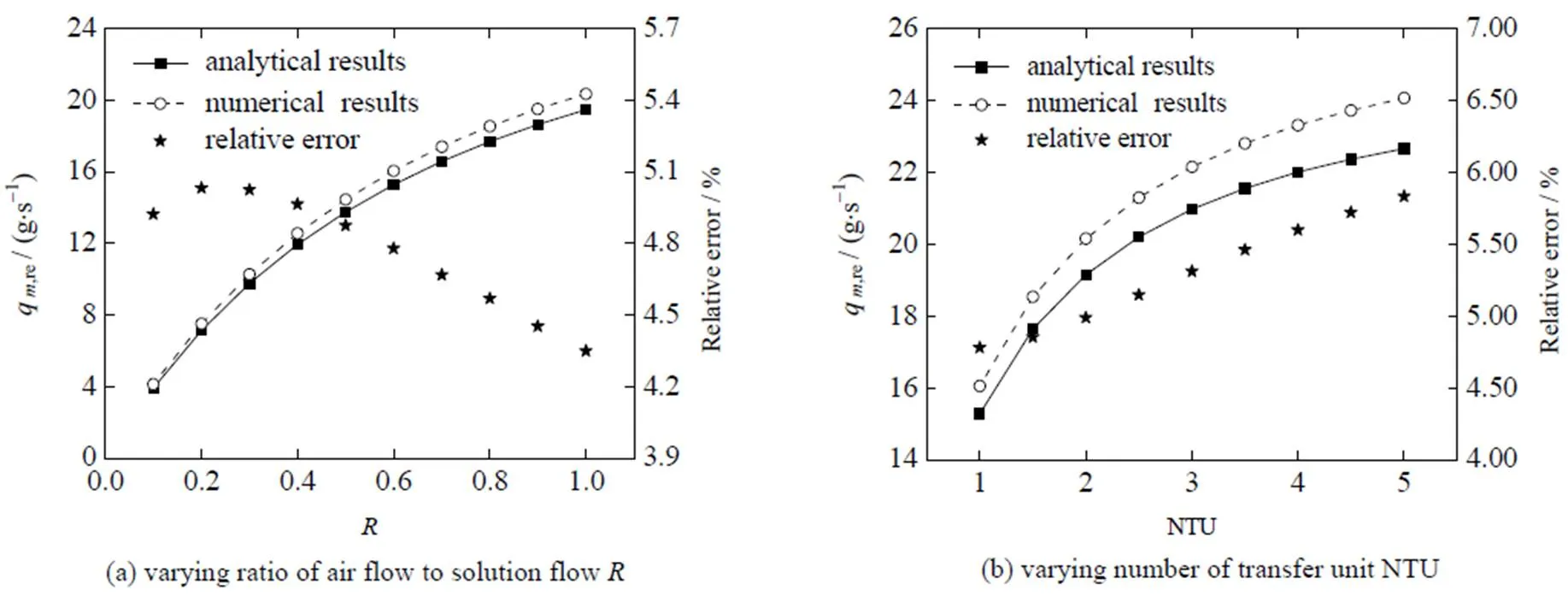
图5 再生工况下解析模型与数值模型结果对比

图4和5分别显示了通过数值模型和解析模型计算得到的除湿率和再生率及两者相对误差随气液流量比和传热单元数NTU的变化情况。从图中可以看出，当其他参数不变情况下，随和NTU的增加，除湿/再生过程中的除湿率和再生率均逐渐升高。在模拟范围内，解析模型结果与数值模型结果的相对误差在±6%以内，平均误差为3.4%。另外，图4(a)与4(b)中的相对误差分别在=0.8及NTU=1.5时发生突变，前者是因为除湿工况下，随着的增大，除湿率逐渐增加，空气释放的冷凝热也增加，溶液除湿温度随之升高。在本研究选定的基准除湿工况下，当≤0.7时，解析模型选取对数平均温差来计算。而当增大到0.8时，选取对数平均含湿量差来计算解析模型，模型切换过程导致了误差趋势的突变，但绝对值大小仍在允许范围内，结果的精度可接受。图4(b)的原因与之类似。

5.2 实验验证

文献[32]中实验得到的除湿与再生工况数据可被用于验证逆流除湿/再生器解析模型，实验分别采用聚丙烯RauschertHiflow环和LiCl水溶液作为填料和除湿剂，填料塔为圆柱体直径为25.4 cm、高为60 cm、比表面积为210 m2×m-3，实验工况见表2。

表2 文献[32]中除湿/再生器实验工况

图6 解析模型与实验结果对比

6 解析模型预测结果分析

对于除湿和再生过程来说，水分的传递量是主要考虑的问题，因此采用湿效率来评价除湿/再生器的性能：

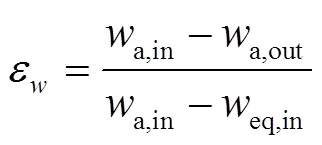
图7 不同工况下除湿/再生过程焓耗散数Eh*对湿效率ew的影响

Fig.7 Effects of the enthalpy entransy dissipation number Eh* on humidity efficiency ew under varying conditions

7 结论

[1] LIU X L, QU M, LIU X B,. Membrane-based liquid desiccant air dehumidification: A comprehensive review on materials, components, systems and performances [J]. Renewable and Sustainable Energy Reviews, 2019, 110: 444-466.

[2] SHUKLA D L, MODI K V. Hybrid solar still- Liquid desiccant regenerator and water distillation system [J].Solar Energy, 2019, 182: 117-133.

[3] 苏博生, 韩巍. 低温余热/电联合驱动的双级溶液除湿系统热力性能研究[J].工程热物理学报, 2016, 37(11): 2296-2302.

SU B S, HAN W. Performance analysis of a two-stage liquid desiccant dehumidification system driven by low temperature heat and electricity [J]. Journal of Engineering Thermophysics, 2016, 37(11): 2296-2302.

[4] CHARARA J, GHADDAR N, GHALI K,. Cascaded liquid desiccant system for humidity control in space conditioned by cooled membrane ceiling and displacement ventilation [J].Energy Conversion and Management, 2019, 195:1212-1226.

[5] QU X H, CAI W J, HE X X,. Experimental investigations on heat and mass transfer performances of a liquid desiccant cooling and dehumidification system [J]. Applied Energy, 2018, 220: 164-175.

[6] WEN T, LUO Y M, WANG M,. Comparative study on the liquid desiccant dehumidification performance of lithium chloride and potassium formate [J]. Renewable Energy, 2021, 167: 841-852.

[7] LIU J, LIU X H, ZHANG T. Analytical solution of heat and mass transfer process in internally cooled liquid desiccant dehumidifiers using refrigerant as cooling medium [J]. Energy Buildings, 2019, 190: 1-14.

[8] WOODS J, KOZUBAL E. On the importance of the heat and mass transfer resistances in internally-cooled liquid desiccant dehumidifiers and regenerators [J]. International Journal of Heat and Mass Transfer, 2018, 122: 324-340.

[9] LAZZARIN R M, GASPARELLA A, LONGGO A. Chemical dehumidification by liquid desiccants: theory and experiment [J]. International Journal of Refrigeration, 1999, 22(4): 334-347.

[10] 代彦军, 张鹤飞, 俞金娣. 错流降膜液体干燥剂除湿/再生传热传质数学模型及分析[J]. 化工学报, 2001, 52(6): 510-515.

DAI Y J, ZHANG H F, YU J D. Mathematical model and analysis on heat and mass transfer of falling film liquid desiccant dehumidification in cross-flow [J]. Journal of Chemical Industry and Engineering (China), 2001, 52(6): 510-515.

[11] VARELA R J, YAMAGUCHI S, GIANNETTI N,. General correlations for the heat and mass transfer coefficients in an air-solution contactor of a liquid desiccant system and an experimental case application [J]. International Journal of Heat and Mass Transfer, 2018, 120: 851-860.

[12] REN C Q, JIANG Y, ZHANG Y P. Simplified analysis of coupled heat and mass transfer processes in packed bed liquid desiccant-air contact system [J]. Solar Energy, 2006, 80(1): 121-131.

[13] BABAKHANI D, SOLEYMANI M. Simplified analysis of heat and mass transfer model in liquid desiccant regeneration process [J]. Journal of the Taiwan Institute of Chemical Engineers, 2010, 41(3): 259-267.

[14] SONG X, ZHANG L, ZHANG X S. NTUm-based optimization of heat or heat pump driven liquid desiccant dehumidification systems regenerated by fresh air or return air [J]. Energy, 2018, 158: 269-280.

[15] LIU X H, JIANG Y, XIA J J,. Analytical solutions of coupled heat and mass transfer processes in liquid desiccant air dehumidifier/regenerator [J]. Energy Conversion and Management, 2007, 48(7): 2221-2232.

[16] LIANG C H, LI N F, HUANG S M. Entropy and exergy analysis of an internally-cooled membrane liquid desiccant dehumidifier [J]. Energy, 2020, 192: 116681.

[17] XIONG Z Q, DAI Y J, WANG R Z. Development of a novel two-stage liquid desiccant dehumidification system assisted by CaCl2solution using exergy analysis method [J]. Applied Energy, 2010, 87(5): 1495-1504.

[18] GUAN B, ZHANG T, JUN L,. Exergy analysis and performance improvement of liquid-desiccant deep-dehumidification system: An engineering case study [J]. Energy, 2020, 196: 117122.

[19] 陈林, 陈群, 李震, 等. 溶液除湿性能分析和优化的湿阻法[J]. 科学通报. 2010, 55(12):1174-1181.

CHEN L, CHEN Q, LI Z,. Moisture resistance method for analyzing and optimizing the liquid dehumidification performance [J]. Chinese Science Bulletin, 2010, 55(12): 1174-1181.

[20] GUO Z Y, ZHU H Y, LIANG X G. Entransy—A physical quantity describing heat transfer ability [J]. International Journal of Heat and Mass Transfer, 2007, 50(13/14): 2545-2556.

[21] CHEN Q, YANG K D, WANG M,. A new approach to analysis and optimization of evaporative cooling system I: Theory [J]. Energy, 2010, 35(6): 2448-2454.

[22] ZHANG L, WEI H Y, ZHANG X S. Theoretical analysis of heat and mass transfer characteristics of a counter-flow packing tower and liquid desiccant dehumidification systems based on entransy theory [J]. Energy, 2017, 141: 664-672.

[23] NAIK B K, MUTHUKUMAR P. Energy, entransy and exergy analyses of a liquid desiccant regenerator [J]. International Journal of Refrigeration, 2019, 105: 80-91.

[24] 过增元. 热学中的新物理量[J]. 工程热物理学报, 2008, 29(1):112-114.

GUO Z Y. New physical quantities in heat [J]. Journal of Engineering Thermophysics, 2008, 29(1): 112-114.

[25] 胡帼杰, 过增元. 传热过程的效率[J]. 工程热物理学报, 2011, 32(6): 1005-1008.

HU G J, GUO Z Y. The efficiency of heat transfer process [J]. Journal of Engineering Thermophysics, 2011, 32(6): 1005-1008.

CHEN L G. Theory of entransy and the progress of its application [J]. Chinese Science Bulletin, 2012, 57(30): 2815-2835.

[27] 江亿, 谢晓云, 刘晓华. 湿空气热湿转换过程的热学原理[J]. 暖通空调, 2011, 41(3): 51-64.

JIANG Y, XIE X Y, LIU X H. Thermological principle of moist air heat and moisture conversion processes [J]. Heating Ventilating & Air Conditioning, 2011, 41(3): 51-64.

[28] 刘晓华. 溶液调湿式空气处理过程中热湿耦合传递特性分析 [D]. 北京: 清华大学, 2007.

LIU X H. Combined heat and mass transfer characteristic in air handling process using liquid desiccant [D]. Beijing: Tsinghua University, 2007.

[29] GOU J F, CHENG L, XU M T. Entransy dissipation number and its application to heat exchanger performance evaluation [J]. Chinese Science Bulletin, 2009, 54(15): 2708-2713.

[30] CHEN X Y, LIU X H, LI Z,. Analytical solution of heat and mass transfer in dehumidifier/regenerator of liquid desiccant equipment: Proceedings of the 4th International Symposium on Heating, Ventilating and Air Conditioning vol.2 [C]. Beijing: Department of Building Science, Tsinghua University, 2003.

[31] SONG X, ZHANG L, ZHANG X S. Analysis of the temperatures of heating and cooling sources and the air states in liquid desiccant dehumidification systems regenerated by return air [J]. Energy, 2019, 168: 651-661.

[32] FUMO N, GOSWAMI D Y. Study of an aqueous lithium chloride desiccant system: Air dehumidification and desiccant regeneration [J]. Solar Energy, 2002, 72(4): 351-361.

An analytical model for predicting the performance of adiabatic dehumidifier/regenerator based on enthalpy entransy dissipation numbers

PENG Dong-gen, CAO Zhuo

(School of Infrastructure Engineering, Nanchang University, Nanchang 330031, China)

In order to study the irreversibility of coupled heat and mass transfer processes of parallel/counter flow solution dehumidification/regeneration, a concept of enthalpy entransyEwas proposed，and the enthalpy entransy dissipation numberE*of solution dehumidification/regeneration was deduced. Its relationship with the equivalent heat capacity ratio*, the number of heat transfer unit (NTU) and the enthalpy efficiencywere obtained, respectively. Moreover, an analytical model of dehumidifier/regenerator based onE*was established, and the effect of the enthalpy entransy dissipation numberE*on the performance of dehumidifier/regenerator was analyzed. The results show that theE* shows negative linearity relationship with the,and it increases with the decrease of* and decreases to a stable value with the increase of NTU. The degree of heat and mass transfer processes taking place between the solution and air is deeper whenE* is smaller, while their processes tend to stop whenE* approaches 1.0. At constantE*, the solution inlet temperature and the flow-rate ratio have more significant effects on the performance of the device.

dehumidification / regeneration; enthalpy entransy; analytical model; irreversibility; heat and mass transfer

1003-9015(2022)04-0543-11

TU834.9

A

10.3969/j.issn.1003-9015.2022.04.010

2021-08-12；

2021-11-17。

国家自然科学基金(51766010)；江西省研究生创新专项资金(YC2019-S002)；南昌市高效制冷知识创新团队(2018-CXTD-004)。

彭冬根(1975-)，男，江西吉安人，南昌大学教授，博士。

彭冬根，E-mail：ncu_hvac2013@163.com

彭冬根, 曹卓. 基于焓火积 耗散数的绝热型溶液除湿/再生器性能预测解析模型[J]. 高校化学工程学报, 2022, 36(4): 543-553.

：PENG Dong-gen, CAO Zhuo. An analytical model for predicting the performance of adiabatic dehumidifier/regenerator based on enthalpy entransy dissipation numbers [J]. Journal of Chemical Engineering of Chinese Universities, 2022, 36(4): 543-553.