Revealing Chern number from quantum metric

Anwei Zhang(张安伟)

1Department of Physics,The Chinese University of Hong Kong,Shatin,New Territories,Hong Kong,China

2Department of Physics,Ajou University,Suwon 16499,Korea

Keywords: quantum metric,Chern insulator,topological physics

1. Introduction

Quantum mechanics and geometry are closely linked.When a quantum system evolves adiabatically along a cycle in the parameter space, its quantum state will acquire a measurable phase that depends only on the shape of the cycle. This phase is the geometric phase,[1,2]which is one of the most important concepts in quantum mechanics and the basis of a variety of phenomena and applications. The geometric phase is actually the holonomy in fiber bundle theory,[3]and when the quantum system evolves under an infinitesimal adiabatic cycle,it is proportional to the symplectic form on the projective space of normalized quantum states,i.e.,Berry curvature.Berry curvature is a central quantity in characterizing the topological nature of quantum matter. For instance,the topological invariants: the first[4]and second[5]Chen numbers are respectively defined by integrating the Berry curvature and its wedge product over a closed manifold in parameter space.

Berry curvature corresponds to the imaginary part of a more general quantity: quantum geometric tensor. It was introduced in order to equip the projective space of normalized quantum states with a distance.[6]This effort results in the quantum(Fubini-study)metric tensor which is the real part of quantum geometric tensor and describes the U(1)gauge invariant quantum distance between neighboring quantum states.The notion of geometric phase,Berry curvature,and quantum metric tensor can be generalized to the quantum system with degenerate energy spectra which has a far richer structure. For a given energy level,the wave function is multi-valued around the cycle in the parameter space. As a result, the geometric phase becomes non-Abelian holonomies which depend on the order of consecutive paths[7]and Berry curvature becomes a matrix-valued vector. Besides, the adiabatic evolution of the quantum degenerate system will lead to the non-Abelian quantum metric tensor[8]which measures a local U(n)gauge invariant quantum distance between two neighboring quantum states in the parameterized Hilbert space.

Quantum metric tensor plays an important role in the recent studies of quantum phase transitions[9,10]and quantum information theory.[11,12]For example, the diagonal components of the quantum metric tensor are actually fidelity susceptibilities, whose critical behaviors are of great interest.[13,14]Quantum metric tensor also appears in the research of Josephson junctions,[15]non-adiabatic quantum evolution,[16]Dirac and tensor monopoles in Weyl-type systems,[17]and bulk photovoltaic effect in topological semimetals.[18,19]Recently, it was shown that for two-dimensional topological insulator,the quantum metric is related with Berry curvature.[21-24]For a higher-dimensional topological insulator, the system will be degenerate. Whether the quantum metric is linked with Berry curvature is still unknown. Besides, up to now, the role of quantum metric in the research of topological invariant for higher-dimensional topological insulator has not been explored.

In this paper,by investigating the quantum metric in Dirac model of 2N-dimensional Chern insulator, we find that the quantum metric is equivalent to the metric on the hypersphere embedded in Euclidean parameter space.We show that there is a general relation between Berry curvature and quantum metric,which reveals that the Chern number is linked with quantum metric as well as the surface area of Brillouin zone on the hypersphere. We also show the geometrical property of quantum metric. Furthermore, we give the scheme to extract the quantum metric in our degenerate system.

2. Corresponding between quantum metric and the metric on sphere

We start with considering the Dirac model of fourdimensional insulator. This model is widely used. It is a minimal model for four-dimensional topological insulator[25]and appears in Dirac Hamiltonian, nuclear quadrupole resonance,and four-band Luttinger model, etc. This Dirac Hamiltonian has the form

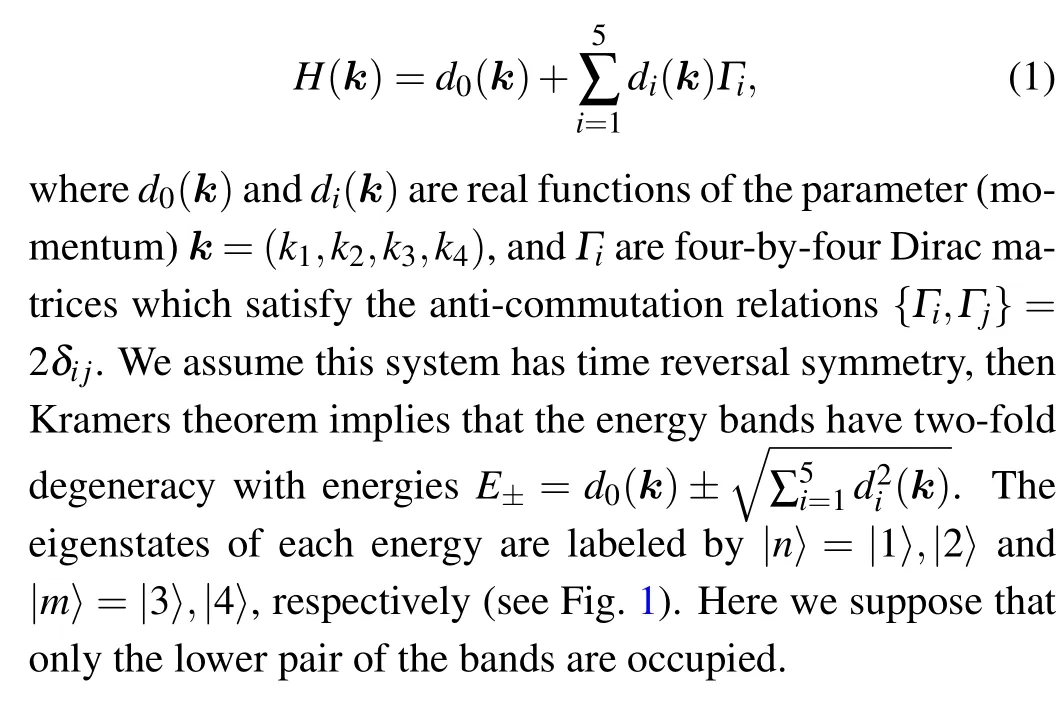
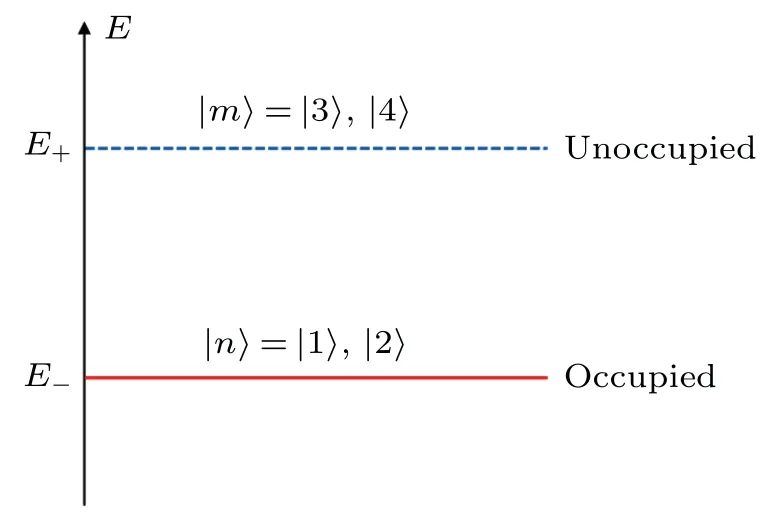
Fig. 1. Schematic illustration for the energy spectrum with a pair of doubly degenerate bands.

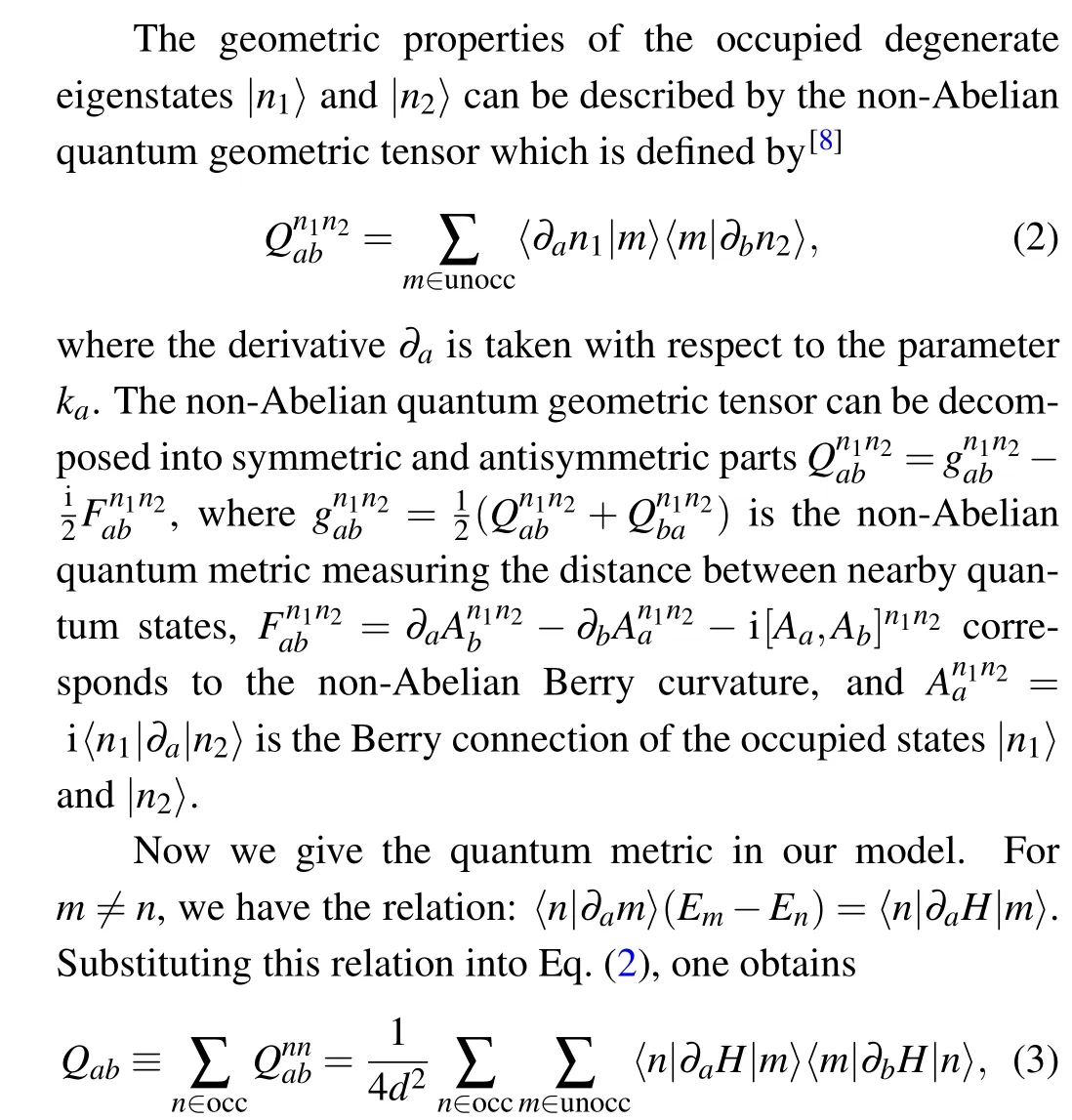
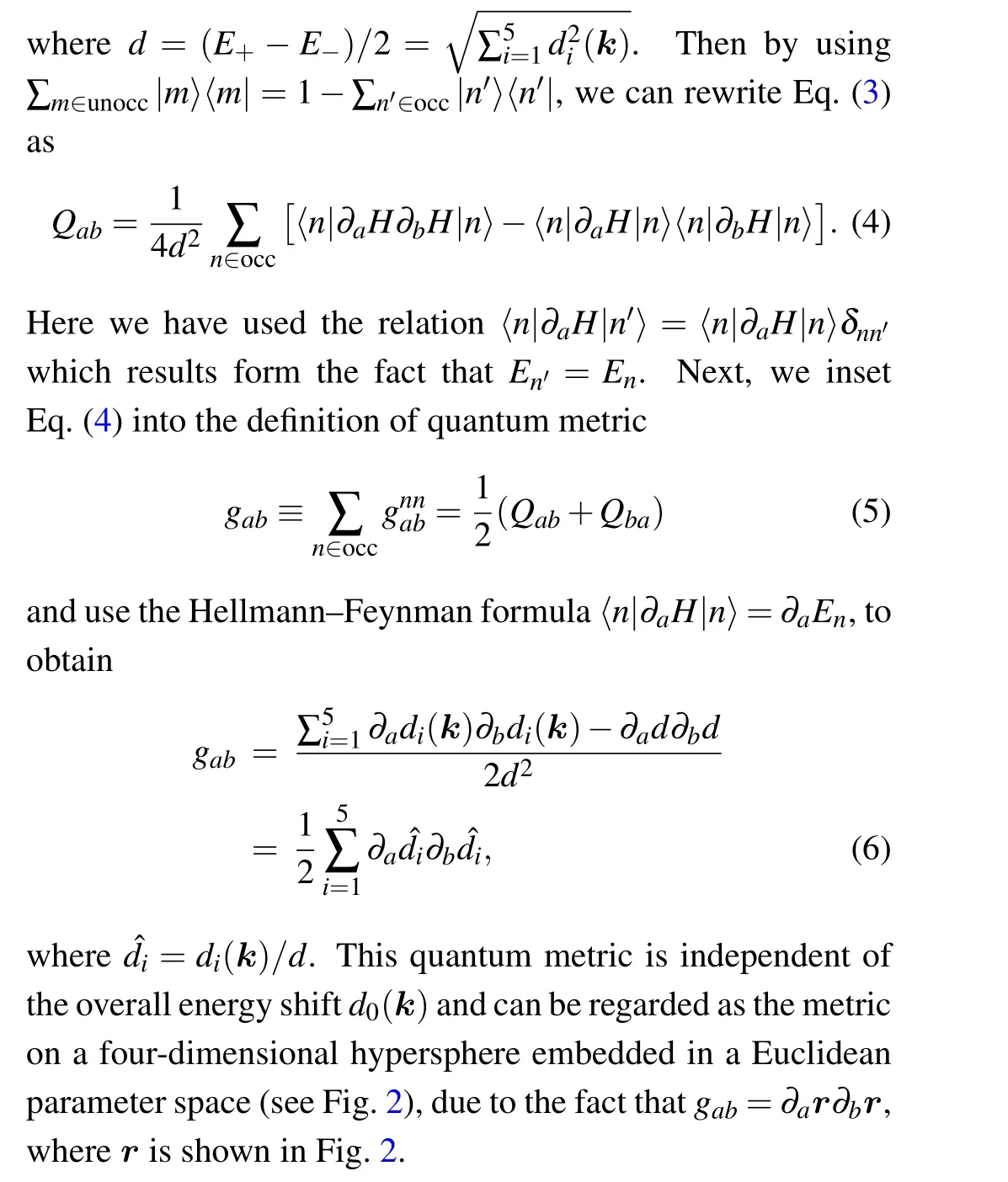
Fig. 2.Four-dimensional hypersphere embeddedinfvie-dimensional Euclideanparameter space.( dˆ1,dˆ2,dˆ3,dˆ4, dˆ5)=rand(k1,k2,k3,k4) are respectively the Cartesian coordinate and the Gauss coordinate of each point on the hypersphere with radius2/2. The metric is ∂ar·∂br which measures the distance between two neighboring points on the hypersphere.

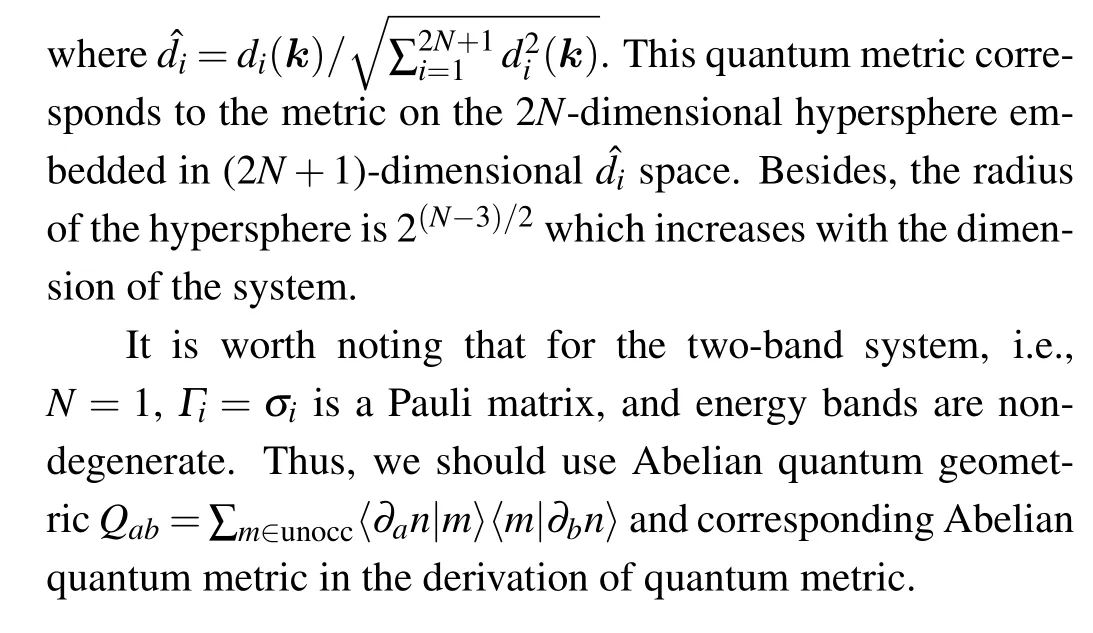
3. The link between quantum metric and Chern number

We now consider the determinant of the quantum metric and its relation with the Chern number.We first investigate the four-dimensional case. Without changing the determinant,we extend the four-by-four quantum metric 2gabto a five-by-five matrix as

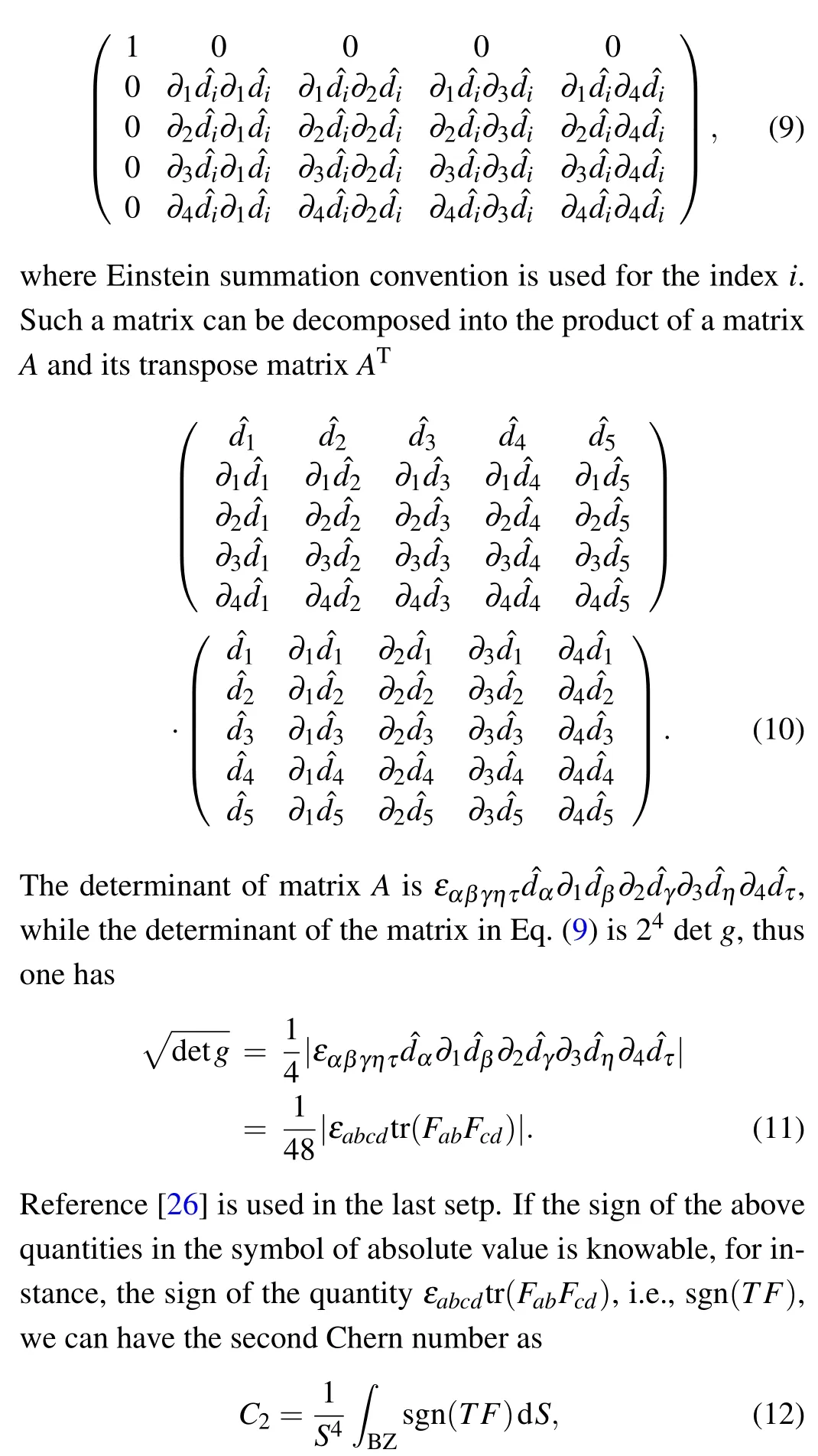
4. Geometrical property of quantum metric

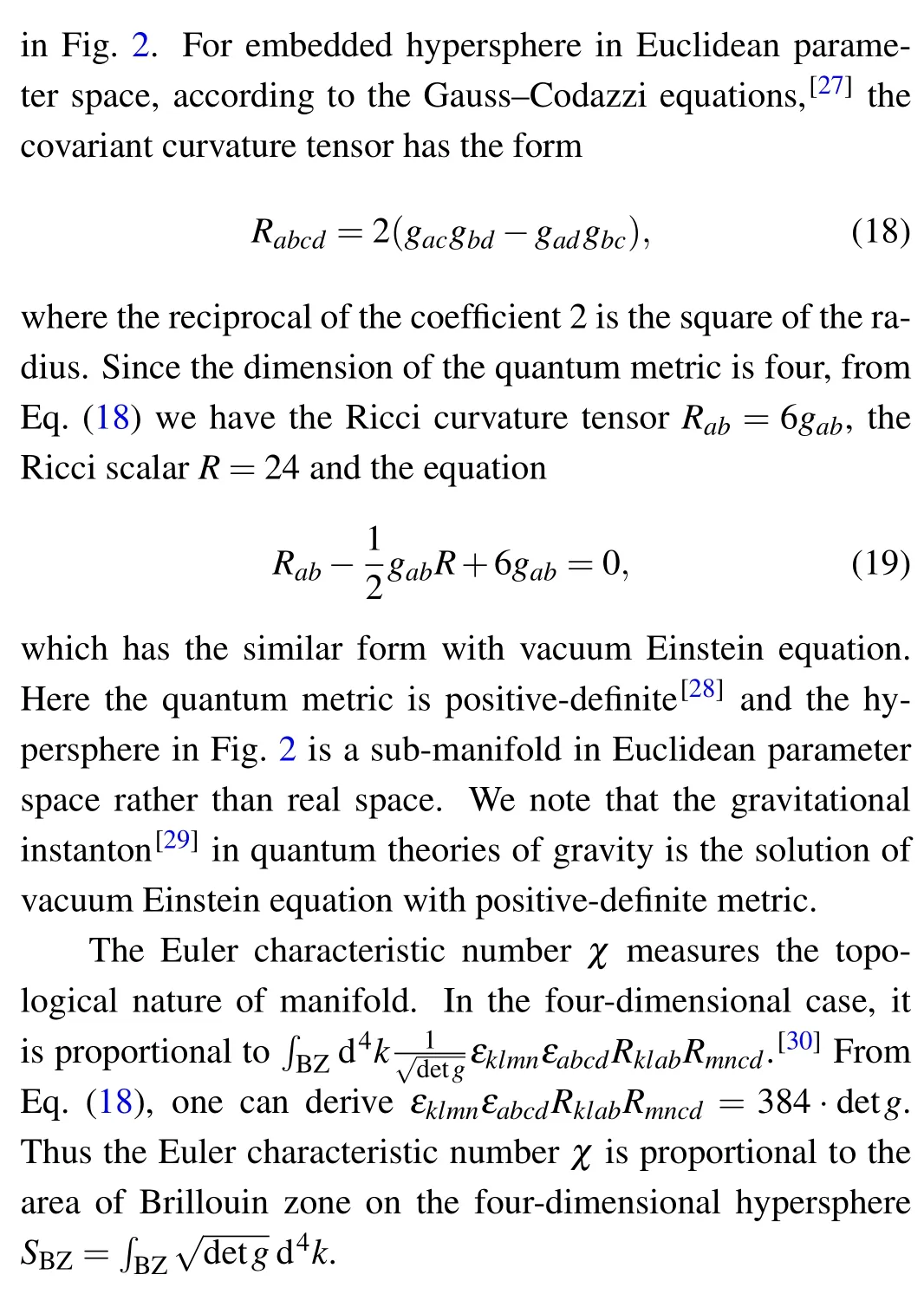
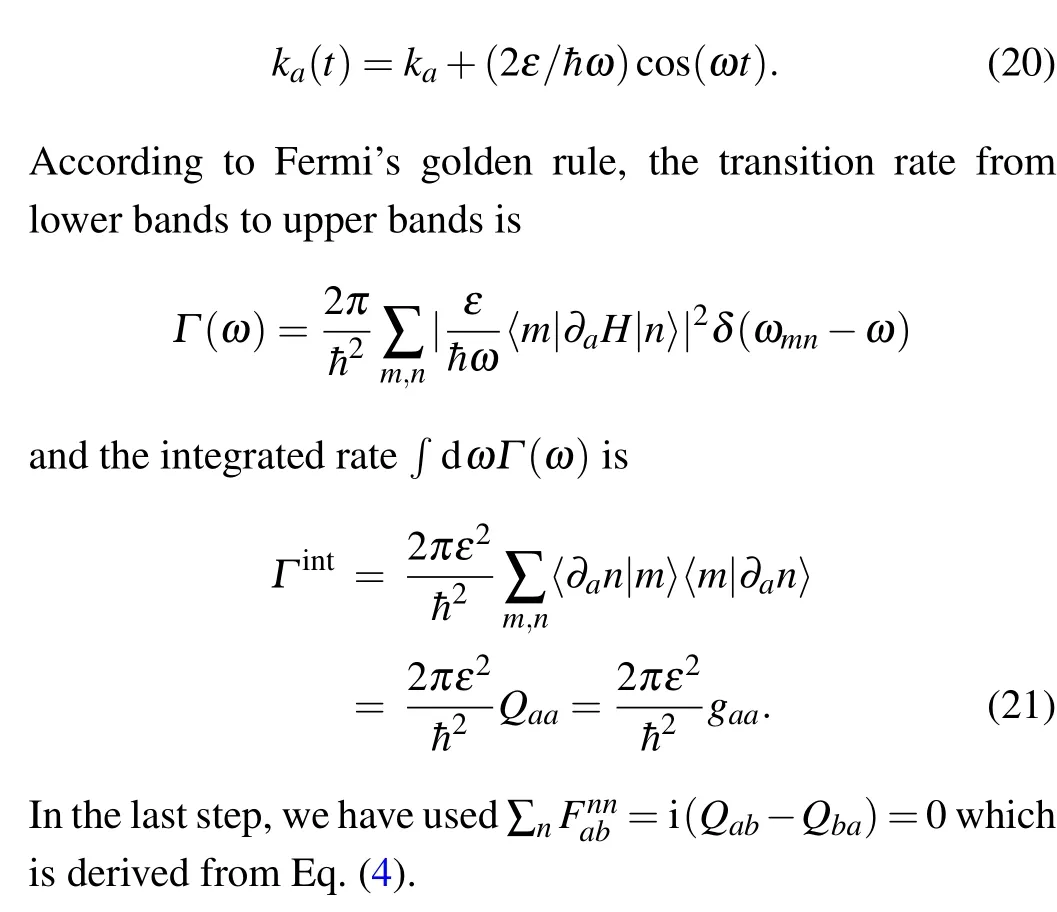
5. Implementation

The Hamiltonian Eq. (1) can be realized by using the four hyperfine ground states of rubidium-87 atoms coupled with radio-frequency and microwave fields.[31]Electric circuits[32-34]can also be used to implement this Hamiltonian.Now we consider how to extract the quantum metric in our degenerate system. Inspired by the measurement scheme for non-degenerate system,[35]we modulate the parameterkain time as

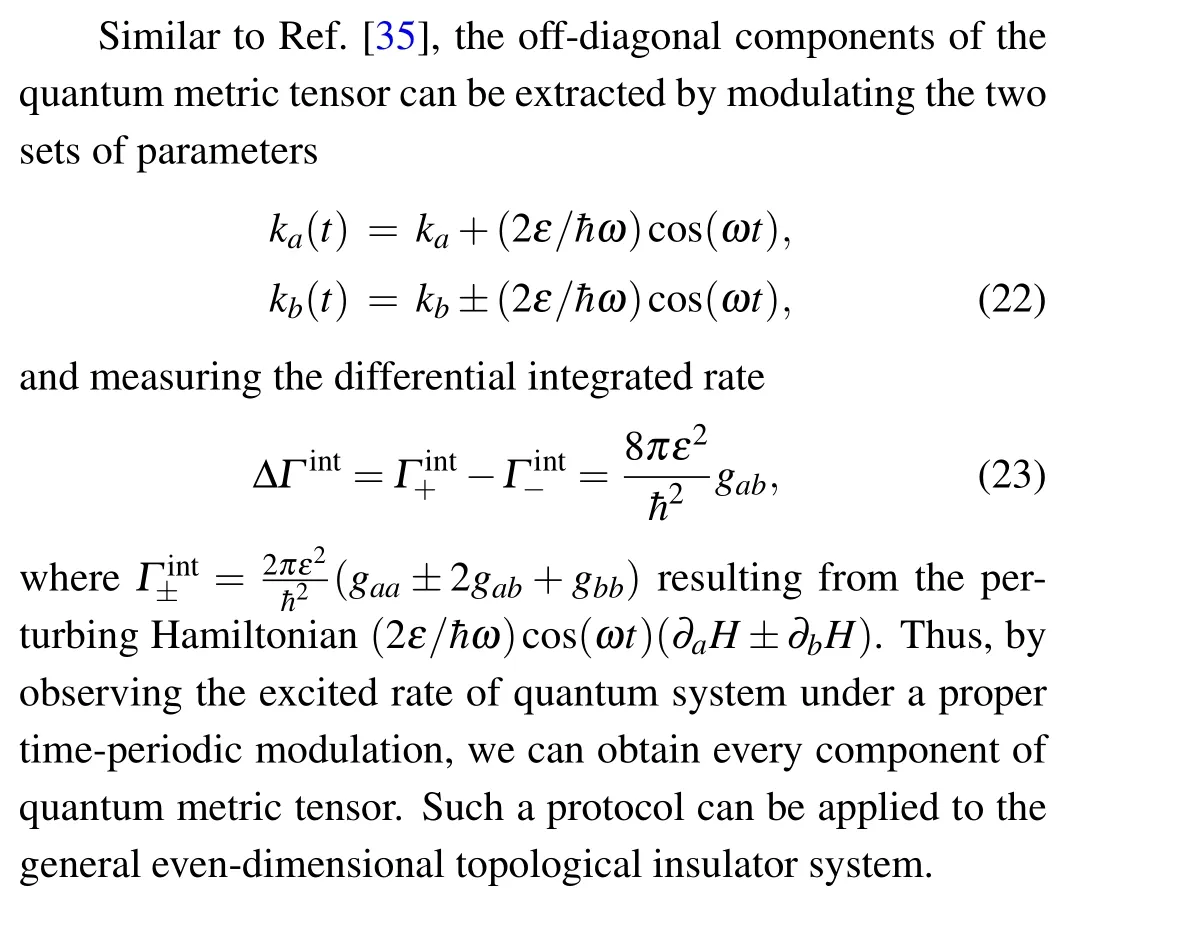
6. Concluding remarks

Acknowledgements

We would like to thank R. B. Liu for useful discussion and N.Goldman for helpful comment.