Orientation and ellipticity dependence of high-order harmonic generation in nanowires
2022-04-12FanYang杨帆YinghuiZheng郑颖辉LuyaoZhang张路遥XiaochunGe葛晓春andZhinanZeng曾志男
Fan Yang(杨帆) Yinghui Zheng(郑颖辉) Luyao Zhang(张路遥)Xiaochun Ge(葛晓春) and Zhinan Zeng(曾志男)
1State Key Laboratory of High Field Laser Physics,Shanghai Institute of Optics and Fine Mechanics,Chinese Academy of Sciences,Shanghai 201800,China
2Center of Materials Science and Optoelectronics Engineering,University of Chinese Academy of Sciences,Beijing 100049,China
Keywords: high-order harmonic generation,orientation dependence,ellipticity dependence,nanowires
1. Introduction
High-order harmonic generation(HHG)in gases has been extensively studied and its excellent time-space coherence provides the basis for a generation of tunable coherent XUV and soft x-ray sources.[1-5]However, the status of HHG in solids has not yet been fully established. Since the first observation in 2010,[6]many researchers have begun to focus on HHG in solids,[7-12]as it provides a new tool for detecting the electron energy band structure and atomic orbitals inside solids. This has opened the door to the physics of ultrafast condensed matter.[13-17]However,solid HHG is limited by the low damage threshold of the material and the harmonic yield cannot be enhanced by increasing the intensity of the driving field. Nevertheless,some recent studies have shown that lowdimensional materials have the potential to solve this problem.
In 2017, Tancogne-Dejeanet al.projected that lowlayer two-dimensional materials could effectively increase the yield of high order harmonics.[8]In the same year, Mc-Donaldet al.theoretically demonstrated that quantum confinement in nanowires has the potential to increase the harmonic efficiency.[18]Recently,by solving the time-dependent Schr¨odinger equation, Liet al.demonstrated a strong bandsensitive orientation dependence in nanowires.[19]The results showed that the maximum harmonic intensity is at the first plateau when the pump light polarization is parallel to thecaxis of the nanowires. This orientation dependence reflects a feature of the conduction band involved in harmonic generation. However, the above studies are all theoretical and no relevant experimental proof has yet been seen.
Based on this,we conducted an experimental study of the HHG in CdS NWs and compared it with previous theoretical results. Odd harmonics up to the 11th order were generated.We focused on the dependence of orientation and the ellipticity of HHG in CdS NWs.
2. Experimental details
In the experiment, CdS NWs prepared on a quartz substrate were irradiated with mid-infrared pulses (3.9 µm,~120 fs,18µJ,1 kHz),as shown in Fig.1(a). The polarization of the laser was vertical.As shown in Fig.1,the laser energy in the interaction region is limited to 18µJ by an aperture owing to the lower damage threshold of nanowires. The mid-infrared beam is focused by a concave mirror with a 100-mm focus,resulting in a peak amplitude of 7.8 TW/cm2. The generated harmonics are imaged onto the slit of a spectrometer with an ICCD (Andor intensified CCD) by using a parabolic mirror with 25.4-mm focusing length. The experimental coordinate system is shown in Fig.1(b). The CdS nanowires are synthesized by using a chemical vapor deposition(CVD)method and then transferred to a fused silica substrate.[20-22]It is wurtzite structure. Here, the blue cube indicates a quartz substrate,which is 0.13 mm thick. The yellow line indicates multiple nanowires with a diameter of about 300-400 nm and a length of a few hundred micrometers. The pump laser is transmitted along theZ-axis and is incident vertically on theXYplane of the nanowires. The orientation angle between theX-axis and the laser polarization is set toθ. Andθ=90°meaning that the laser polarization is parallel with the nanowire.
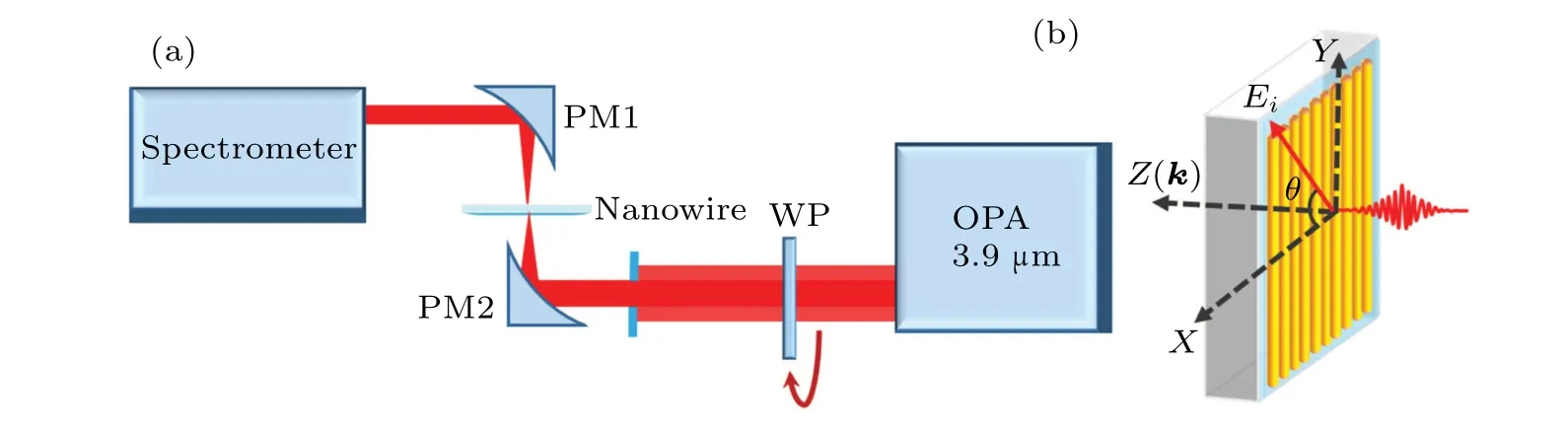
Fig.1. (a)Experimental setup schematic. PM1 and PM2 are two parabolic mirrors that focus the beam onto the spectrometer and the sample,respectively. WP is the wave plate. (b)Schematic diagram of the experimental coordinate system.
A representative spectrum generated with linearly polarized laser in CdS NWs is shown in Fig.2.Owing to the limited detection range of the CCD, we used a segmented scanning method to detect harmonics of different orders. The spectrum has significant peaks at the 7th and 9th harmonics. The intensity of the 11th harmonic drops rapidly,and a less pronounced harmonic peak is visible when the intensity of the spectrum is magnified twice at this point. The intensity of even harmonics is usually lower than that of the 11th, therefore the even harmonics cannot be detected. It can be seen that the fluorescence is a very broad spectrum, which is clearly different from the harmonics. In the experiment, the orientation angle can be changed to study the orientation response of the harmonic yield by rotating the half-wave plate. In addition, to further understand the dependence of the ellipticity,defined asε=Ex/Ey,on the harmonic yield in CdS NWs,we tuned the ellipticity of the pump laser by rotating a quarter-wave plate.
3. Results and discussion
3.1. Orientation dependence of HHG in nanowires
Figure 3 shows the responses of the normalized HHG spectra to orientation at 560 nm,which represent the 7th harmonic spectra.The solid black dots in Fig.3(b)are experimental data from integrating(a)horizontally,and the solid red line is the multi-peak Gaussian fit curve. It is evident from Fig.3 that the intensity of the 7th harmonic is significantly enhanced at 90°and 270°,and strongly suppressed at 180°. The orientation dependence of the harmonic shows a clear 90°symmetry,which is consistent with the calculated results.[19]The strong orientation-dependent transition dipole momentum combined with the weak orientation dependent population lead to the orientation dependent transition probability. However, the full widths at the half maximum of the two peaks,i.e.,the angular range where the harmonic yield is higher,are 95°and 80°,respectively.This is different from the calculation results,where the harmonic yield varies little in all orientations,except when the laser polarization is perpendicular to the nanowire. This is probably because the calculation approximates the lattice potential in theX, Y, Zdirection with a period function and ignores the strong limitation on the propagation of transverse wave packets in the nanowires.
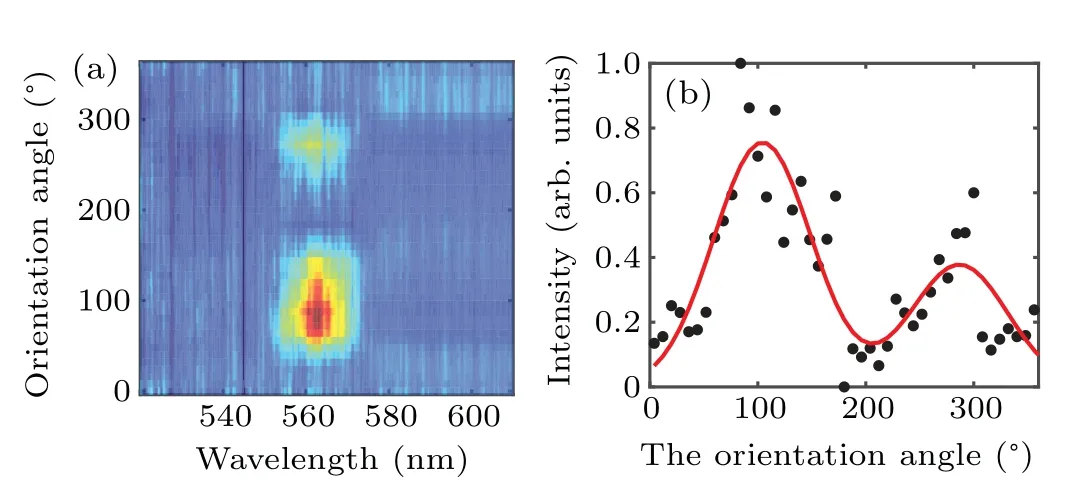
Fig.3. (a)Experimental results of angle θ dependence of 7th harmonic intensity in CdS NWs. (b)Solid black dots are experimental data from integrating(a)horizontally,and the solid red line is the fitted curve.
As demonstrated in the theoretical analysis by McDonaldet al.,[18]in nanowires,the quantum effect is not evident when the nanowire diameter increases to 50 nm. The diameters of actual CdS NWs used in our experiment were 300-400 nm,which are much thicker than those simulated in McDonald’s paper, so the quantum confinement is not significant. However,the conduction band wave packet is still affected by certain transverse confinement,and the ionization in that orientation is greatly reduced. In other words, when the laser polarization direction is changed, the number of ionization events depends on the magnitude of the pump electric field component parallel to the nanowire. When the laser polarization is parallel to the nanowire,it produces the most ionization events in this direction, at which point the harmonic yield is maximum. In other orientations,the pump intensity parallel to the nanowire decreases by cos2(θ), and the harmonic yield also decreases gradually.
This phenomenon is also similar to the results obtained from the second-harmonic generation (SHG) in single zinc sulfide nanowires (ZnS NWs)[23]and the HHG calculations of the MgO nanowire model.[19]As mentioned earlier,the intensity of the harmonics is strongest when the electric field of the pump laser lies parallel to the nanowires.Based on this,we can roughly determine the orientation of the CdS NWs. In the sample used for this experiment,the angle between the growth axis of CdS NWs and theX-axis of the coordinate system is 90°.
3.2. Ellipticity dependence of HHG in nanowires
Figure 4 shows the responses of ellipticity at 7th harmonic. The red solid curve indicates the curve fitted by a Gaussian function, which correlates with the experimental data(the black dots). As with gas,the harmonics in the NWs are sensitive to the ellipticity, with the strongest harmonics produced under linearly polarized laser and weaker harmonics produced by elliptically polarized laser. It can effectively turn off the HHG process atε=±0.4.
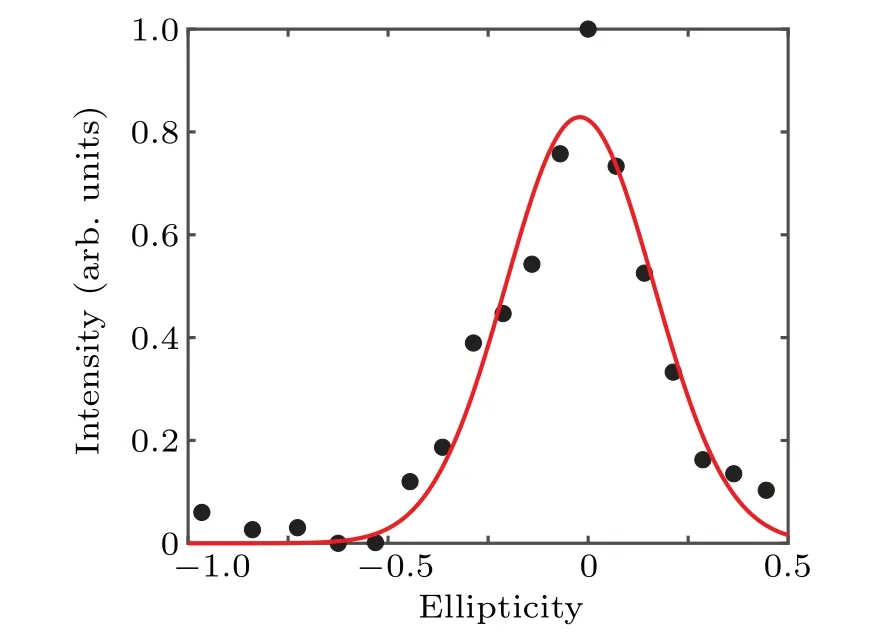
Fig. 4. Experiment results for ellipticity dependence of 7th harmonic intensity in CdS NWs. Solid black dots are the experimental data and solid red line is the fitted curve.
This is very similar to the elliptical dependence of HHG in MoS2,[10]and indicates that HHG in NWs is not in the semimetal regime. In addition, the ellipticity dependence in NWs is very different from that in bulk ZnO,where harmonic yield is not sensitive to ellipticity, and a circular polarization(ε= 1) is required to stop the HHG process.[6]This is an indication that the harmonic emission in bulk crystal is not restricted to the same position of ionization and recombination,which is not the case in the nanowire structure. As mentioned previously, transverse confinement of the conduction band wave packet strongly reduces ionization. On the other hand, the transverse confinement existing in the nanowires makes the ionization and recombination tend to be restricted to the same position in the harmonic emission. This makes the strongest HHG generate when the pump electric field is linearly polarized and parallel to the nanowires orientation, and the HHG weakens rapidly once it deviates from the linear polarization. Therefore,the HHG in CdS NWs is more sensitive to ellipticity dependence compared to bulk ZnO.
4. Conclusion and perspectives
In conclusion, we experimentally obtained a nonperturbative harmonic of up to the 11th order generated from nanowires. As the strongest harmonic intensity occurs when the laser polarization lies parallel to the nanowires,[19]we proved this experimentally, in which the direction of the nanowires could be determined. Furthermore,the experimental harmonic yield of the nanowires displays a Gaussian-like dependence on the ellipticity of the pump laser,as in the case of gases, but not in the case of bulk ZnO. Low-dimensional materials have exceptional optoelectronic properties compared with the bulk solids.[24]One-dimensional nanostructures,such as nanowires, nanoribbons, and nanotubes, are an excellent system to develop mesoscopic physics and nanodevices. Our experimental results preliminarily reveal the laser response characteristics of HHG in nanowires. They provides benchmark data for laser-driven harmonics and a better understanding of the HHG mechanism in one-dimensional materials.However, the specific mechanism of the generation of higher harmonics in one-dimensional materials needs to be further explored by more theories and experiments.
Acknowledgements
Project supported by the National Natural Science Foundation of China(Grant Nos.91950203,11874374,61690223,and 11774363), the Youth Innovation Promotion Association of the Chinese Academy Sciences, and the Strategic Priority Research Program of the Chinese Academy of Sciences(Grant No.XDB16).
猜你喜欢
杂志排行
Chinese Physics B的其它文章
- Helium bubble formation and evolution in NiMo-Y2O3 alloy under He ion irradiation
- Dynamics and intermittent stochastic stabilization of a rumor spreading model with guidance mechanism in heterogeneous network
- Spectroscopy and scattering matrices with nitrogen atom:Rydberg states and optical oscillator strengths
- Low-overhead fault-tolerant error correction scheme based on quantum stabilizer codes
- Transmembrane transport of multicomponent liposome-nanoparticles into giant vesicles
- Molecular dynamics simulations of A-DNA in bivalent metal ions salt solution
