The Investigation of the Fractional-View Dynamics of Helmholtz Equations Within Caputo Operator

Rashid Jan,Hassan Khan,Poom Kumam,Fairouz Tchier,Rasool Shah and Haifa Bin Jebreen

1Department of Mathematics and Statistics,Bacha Khan University,Charsadda,24420,Pakistan

2Department of Mathematics,Abdul Wali Khan University,Mardan,23200,Pakistan

3Department of Mathematics,Near East University TRNC,Mersin,10,Turkey

4Center of Excellence in Theoretical and Computational Science(TACS-CoE)&Department of Mathematics,

Faculty of Science,King Mongkut’s University of Technology Thonburi(KMUTT),126 Pracha-Uthit Road,Bang Mod,Thung Khru,10140,Bangkok,Thailand

5Department of Medical Research,China Medical University Hospital,China Medical University,Taichung 40402,Taiwan

6Mathematics Department,King Saud University,Riyadh,Saudi Arabia

Abstract:It is eminent that partial differential equations are extensively meaningful in physics,mathematics and engineering.Natural phenomena are formulated with partial differential equations and are solved analytically or numerically to interrogate the system’s dynamical behavior.In the present research,mathematical modeling is extended and the modeling solutions Helmholtz equations are discussed in the fractional view of derivatives.First,the Helmholtz equations are presented in Caputo’s fractional derivative.Then Natural transformation,along with the decomposition method,is used to attain the series form solutions of the suggested problems.For justification of the proposed technique,it is applied to several numerical examples.The graphical representation of the solutions shows that the suggested technique is an accurate and effective technique with a high convergence rate than other methods.The less calculation and higher rate of convergence have confirmed the present technique’s reliability and applicability to solve partial differential equations and their systems in a fractional framework.

Keywords:Fractional-order Helmholtz equations;fractional calculus;natural transform decomposition method;analytic solution

1 Introduction

The research area of mathematics interrogating the non-integer properties of derivatives and integrals is called fractional calculus.Fractional calculus has become popular in recent years due to its application in a real-world problem.However,its history is as ancient as ordinary derivative [1,2]and is developed by Leibniz,Liouville,Heaviside,Riemann,Fourier,Lagrange,Abel,Euler [3]etc.Recently,it becomes prevalent and had several real-life applications;moreover,it has been proved that the system developed from natural phenomena can be expressed more accurately through fractional derivative than the ordinary derivative.The applications of fractional calculus occur in control theory,viscoelasticity,electrical networks,diffusive transport akin to diffusion,fluid flow,rheology,optics and signals processing,dynamical processes in the porous structure,probability and statistics,electrochemistry of corrosion and many other branches of economics,physics,engineering and mathematics [4-6].

Hermann Von Helmholtz introduced the concept of the following equation

which demonstrates the time-independent structure of diffusion or wave equation achieved during the implementation of the separable variables technique and make the solution procedure much easier.The Helmholtz equation of dimensional two arises in engineering applications and physical phenomena [7-9]such as water wave propagation,acoustic radiation,heat conduction and even in biology.The importance of the fractional derivative cannot be ignored because it estimates the geodesic seafood properties,acoustic propagation in shallow water as well as at low frequencies.Fractional derivatives provide more accurate results and conceptualize different phenomena in a better way in the form of mathematical model [10-17].

It is well known that the problems in pattern formation animal coating [18]in electromagnetics are also solved through Helmholtz equation,where its two-dimensional structure becomes more applicable in different areas.Several numerical methods have been utilized to solve the above Eq.(1),in which the integral surface method and the Ritz-Galerkin method [19]consume a large unit of time by computing the problem numerically.In contrast,the finite element method [20]produces inaccurate results during computation of the problem.Therefore,we use NTDM to reduce these inaccuracies and to lessen the computational time for our problem.Here,we represent the Helmholtz equations withxandy－spacein the following manner

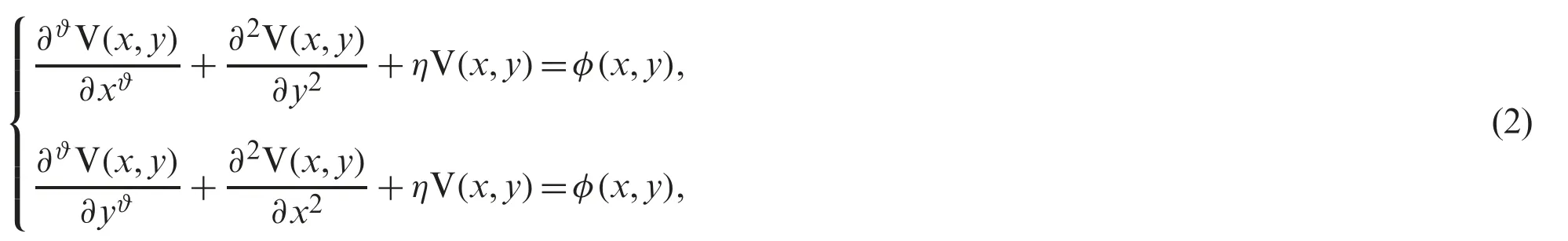
with proper initial conditions given by

V(0,y)=φy,andV(x,0)=φx,

whereϑis the fractional-order which lesson the memory requirements.In the above (2) both x and y have the choices to change the fractional order.

The Natural transform decomposition method is developed by using the two powerful methods that are Adomian decomposition and Natural transform,which solve many PDEs and FPDEs arises from physical phenomena.Specifically,numerous non-linear PDEs [21,22],nonlinear ODEs [23]and fractional-order models and equations [24-26]are solved by NTDM.It has been shown that the convergence rate of the NTDM are higher than MHPM and HPM and are more accurate than the MHPM and HPM.

In the present article,NTDM is implemented in a very simple and sophesticated manner to analyse the solutions of fractional-order Helmholtz equations.Three numerical examples were considered for analytical treatment.The successful NTDM schemes or algorithms are derived for both fractional and integer orders of the problems.First,the natural transformation is applied to reduce the given problems into simpler forms and then Adomian decomposition method is used to investigate the final solutions of the problems.The derived results are then plotted and have shown that the present solutions are in best contact with the solutions of LADM [16]and FRDTM [17].The fractional solutions graphs are plotted and the convergence phenomenan of fractional-orders solutions towards integer-order solutions is observed.Beside these it is also analysed that NTDM is very simple and straightforward with no need of discritization and required very less computational work.In the view of the above novelty,the present work can be extended to solve other nonlinear FPDEs and their systems.

The article is structured as:The fractional-order Helmholtz equations are represented withx-space andy-space in Section 1.In Section 2 of the article,we present the elementary theory of fractional calculus and Natural Transform Decomposition Method.The general idea of the NTDM is introduced and applied to the proposed fractional Helmholtz equations in Section 3.In Section 4,the proposed concept of the NTDM is applied to several numerical examples to understand the procedure of the proposed method;moreover,numerous numerical simulations are used to show the graphical results of the fractional-order problem.Finally,the overall conclusion of the article is presented in Section 5.

2 Rudimentary Theory

In this section of the article,we represent Caputo’s fractional operator to inspect our proposed problem.In addition to this,we will give the basic concept of natural transform,inverse natural transform and the natural transform ofn-thderivative for further analysis and investigation.

2.1 Definition[3,27]

Assume a functionhin a manner thath∈Ct1then the fractional derivative ofhthrough Caputo’s operator of orderϑis given by

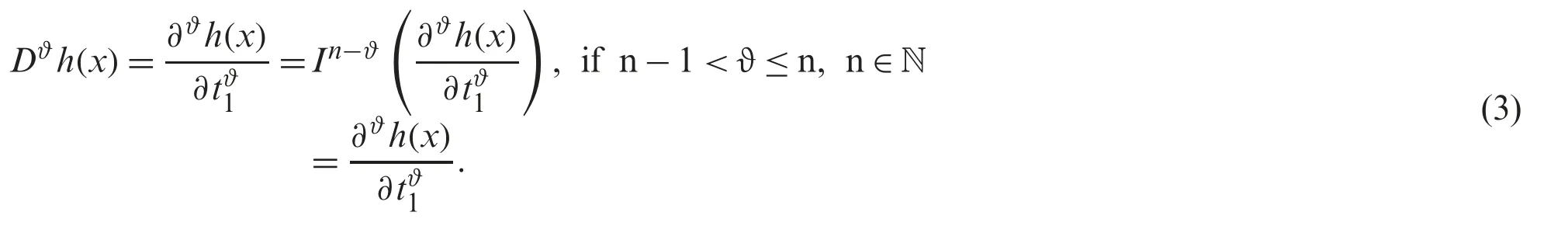
wheren∈N,x＞0,t1≥－1.

2.2 Definition[3,27]

Let ϑ＞0 andq∈Cthen the Mittag-Leffler function is symbolized by Eϑ(q) and is given by the below-mentioned series

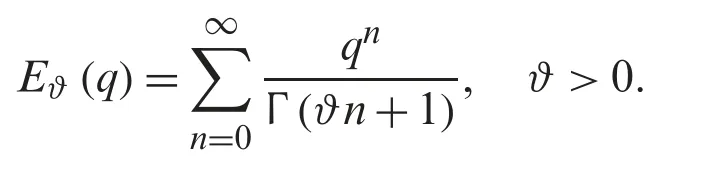
2.3 Definition[28,29]

For a given functionh,the natural transform is given by

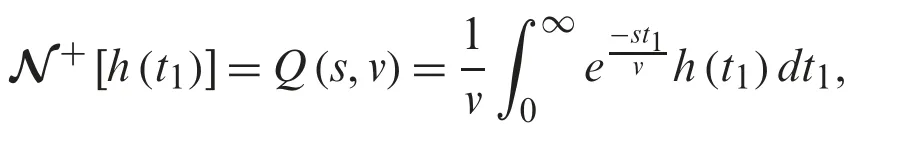
in whichsandvindicate the transform variables and are positive.

2.4 Definition[28,29]

For a given function h,the inverse natural transform is given by the following mentioned definition

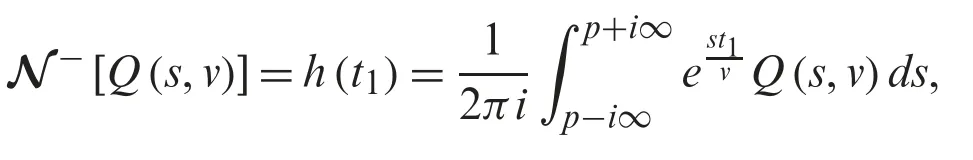
in which p is a real number,s and vindicates Natural transform variables,and the integral in the planes=x+iyis taken alongs=p.

2.5 Definition[28,29]

Let h be a function then the natural transform ofn-th derivative is defined by the following manner

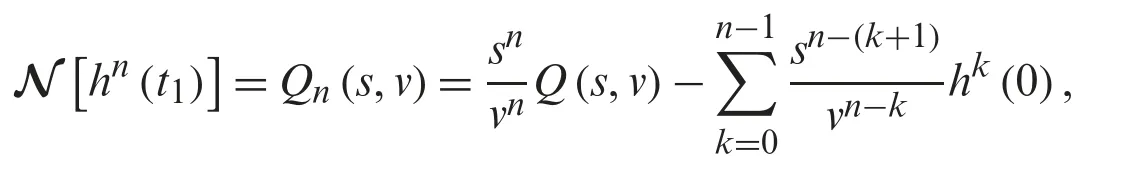
wheren≥1 in this definition.

2.6 Theorem[28,29]

Let the transform functions ofH(s,u)andL(s,u)are given byh(t1)andl(t1),then we have the following

N[h*l]=uH(s,u)L(s,u),

where the convolution of thehmentioned above andlis indicated byh*l.

2.7 Definition[28,29]

Let h be a given function then R-L fractional integral is given as

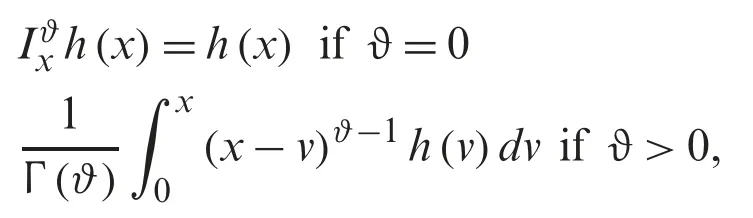
in which Γ indicates the gamma function,and is given by

In the next section of the article,we will give a general concept of fractional NTDM for the solution of Helmholtz equations.

3 Conceptualization of Fractional NTDM[21,22]

Here,we will study the standard concept and procedure of fractional Natural transform decomposition method to solve Helmholtz equations.First,we take the Helmholtz equation in fractional framework withx-space as

with the following suitable initial condition

V(0,y)=φy,

andy-space

with the below mentioned initial condition

V(x,0)=φx.

Utilizing the Natural transform decomposition method to (4),we have

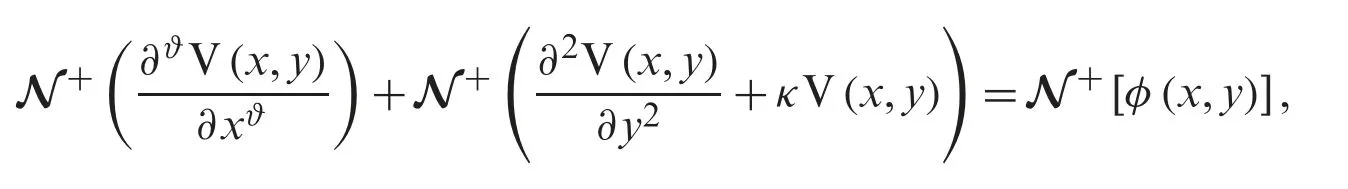
and applying the differentiation property of Natural transform decomposition,the following is obtained

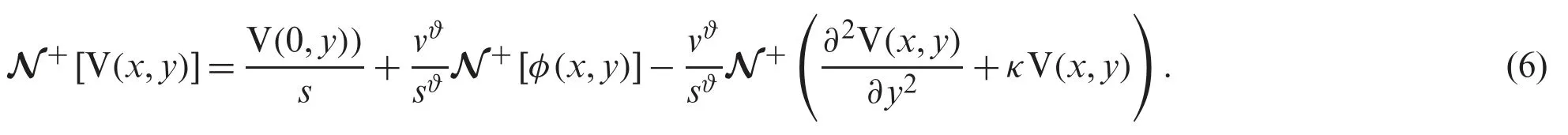
After that,the Natural transform decomposition method solution V(x,y)is given by the belowmentioned series

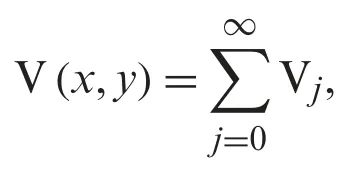
moreover,the following series of Adomian polynomials define the nonlinear term of the problem

using Natural transform decomposition method solution in (6),the following is obtained

Using the linearity of the Natural transform,we have

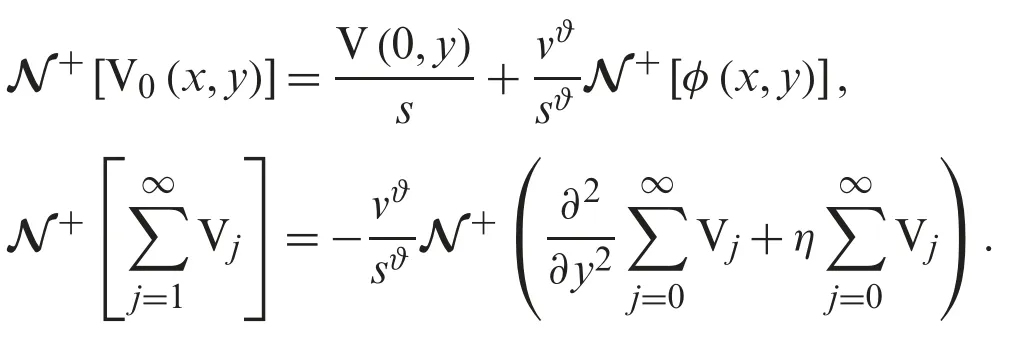
Now,utilizing the inverse of Natural transform,we can compute Vj(j≥0).

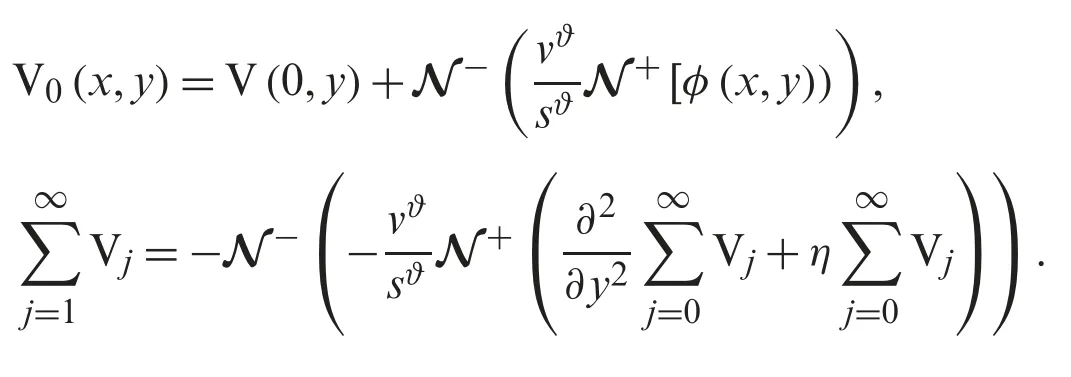
4 Applications and Numerical Simulations

In this section of the article,the method NTDM will be applied to some examples to understand the procedure of the proposed method.In the end,some numerical simulations are carried out to visualize family of Helmholtz equations through Natural transform decomposition method.

Example 1

Let us take the Helmholtz equation in fractional framework withx-space in the following form [30,31]

with the below mentioned initial value

First of all,take the Natural transform of (7),we obtain the following

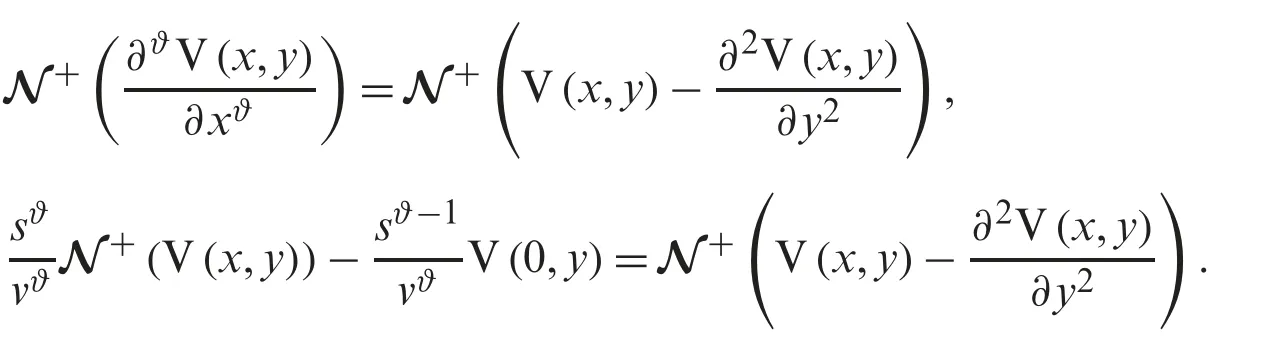
In the next step,we use the Natural inverse transform and get

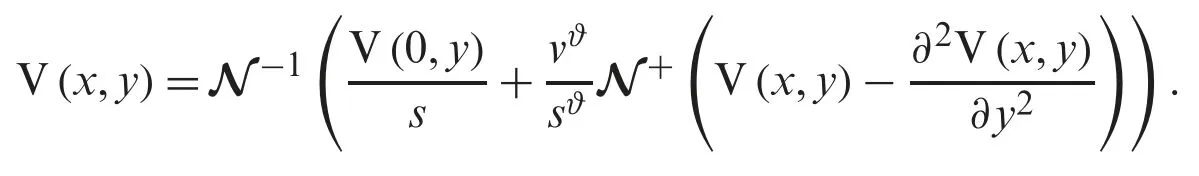
Then,applying the procedure of NTDM,the following is obtained

Forj=0,we have

The subsequent terms are

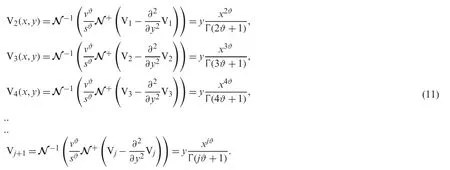
Thus the solution of (4) through NTDM is

in the case whenϑ=2,then the solution of the problem through NTDM is

V(x,y)=ycoshx.

In the same way,the solution ofy-space can be determined through NTDM as:

with the proper initial value

Thus the solution of the above (12) is given by

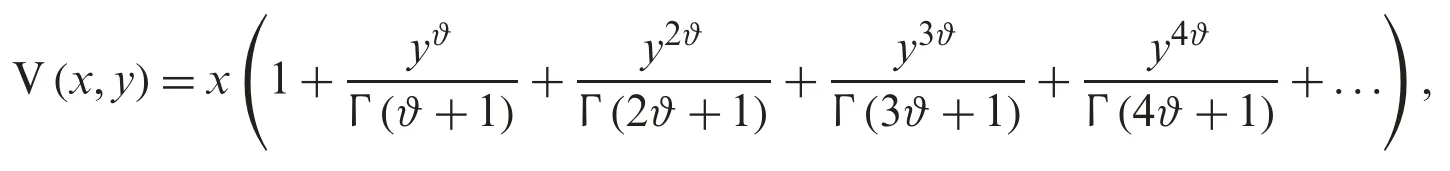
in the case whenϑ=2,then the solution through NTDM is

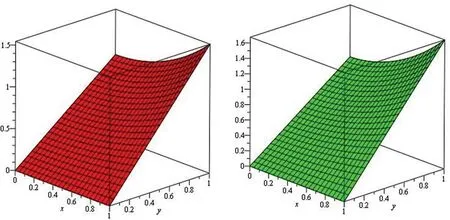
Figure 1:Illustration of solution pathways:(Left) The red figure represent the solution of Example 1 through NTDM for fractional-order ϑ=1 and (Right) The green figure represent the solution of Example 1 through NTDM for fractional-order ϑ=0.8

Example 2

Let us take homogeneous Helmholtz equation in fractional framework withx-space andη=5 as follows [30,31]

with initial values given by

Here,taking the natural transform of (15),we have

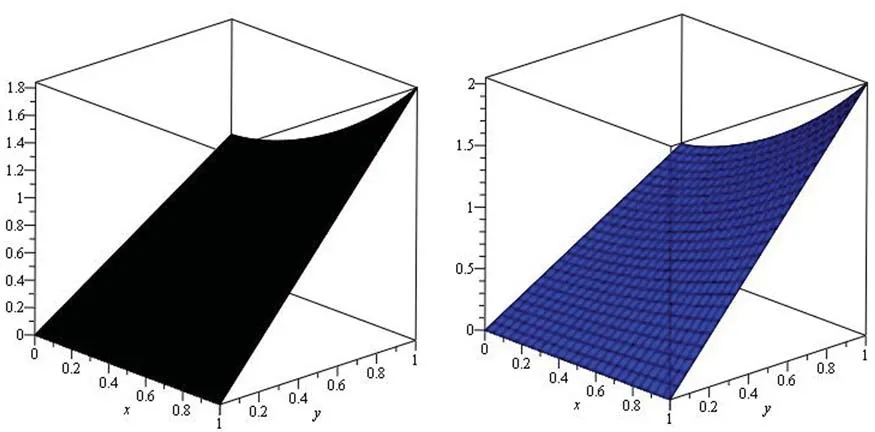
Figure 2:Illustration of solution pathways:(Left) The black figure represent the solution of Example 1 through NTDM for fractional-order ϑ=0.6 and (Right) The blue figure represents the solution of Example 1 through NTDM for fractional-order ϑ=0.4

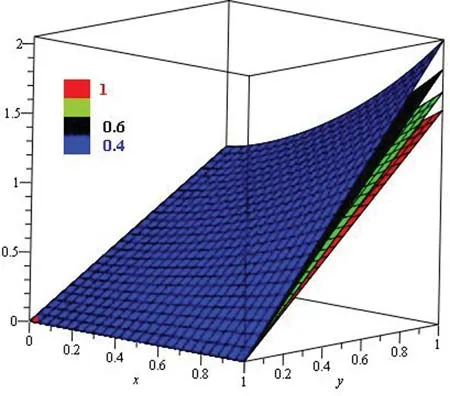
Figure 3:The solution v(x,y,t1) of our proposed Example 1 through the proposed NTDM with varies values of fractional order ϑ

After that,we apply inverse Nature transform to our problem and get

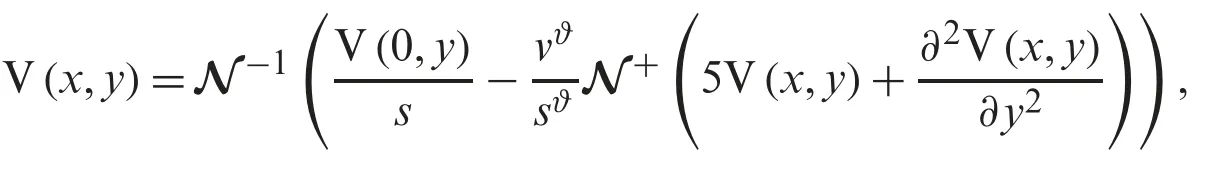
Applying the ADM procedure,we have the following

forj=0,1,2,....In the case whenj=0,we have

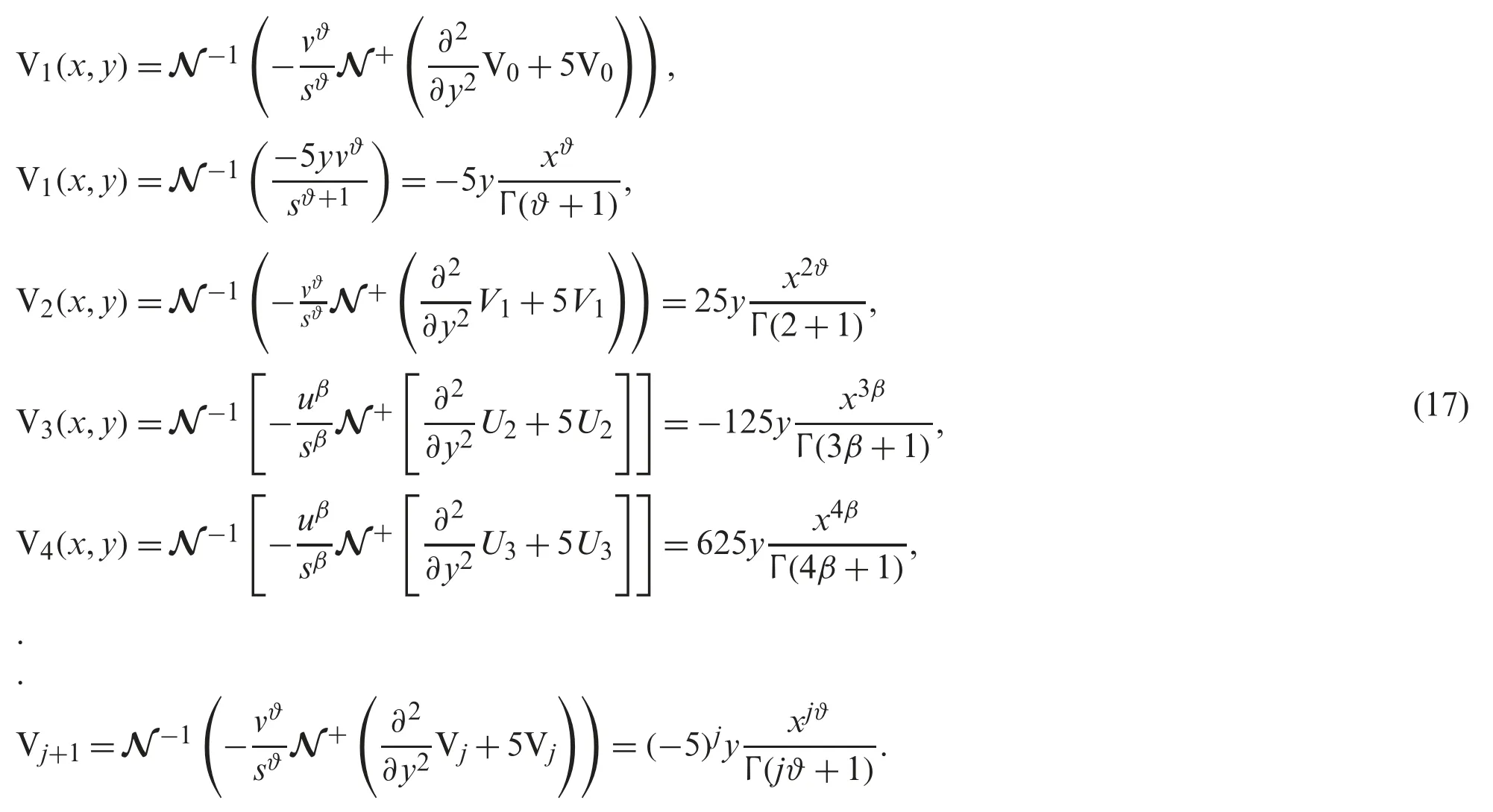
The solution of the above problem (15) through NTDM is given by

V(x,y)=V0(x,y)+V1(x,y)+V2(x,y)+V3(x,y)+V4(x,y)+...

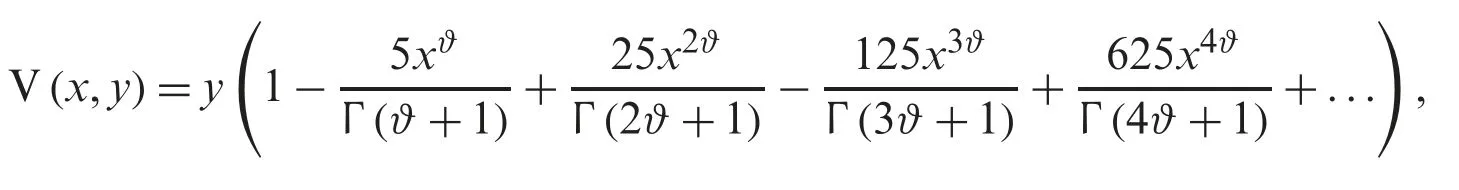
in the case whenϑ=2,then the solution through NTDM is given by

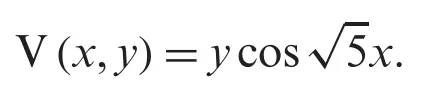
In the same way,we apply NTDM toy-space

with the initial value given by

V(x,0)=x.

having the following solution (18) through NTDM is

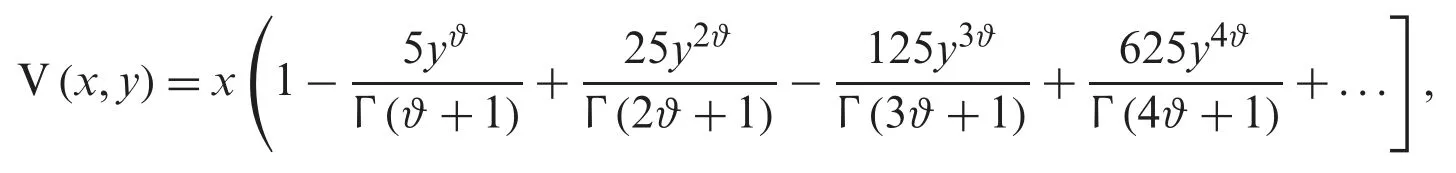
in the case whenϑ=2,the NTDM solution is given by

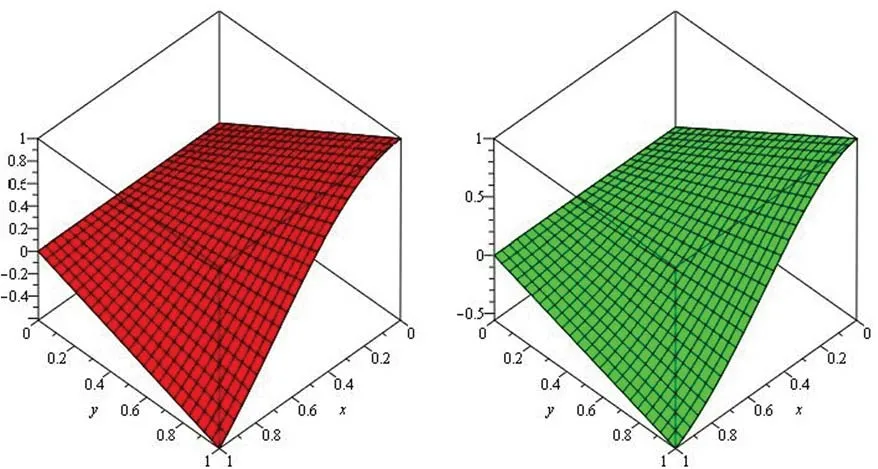
Figure 4:Illustration of solution pathways:(Left) The red figure represent the solution of Example 2 through NTDM for fractional-order ϑ=1 and (Right) The green figure represents the solution of Example 2 through NTDM for fractional-order ϑ=0.8

Example 3

Assume the Helmholtz equation withx-space andη=－2 in the fractional framework [30,31]

with initial value given by

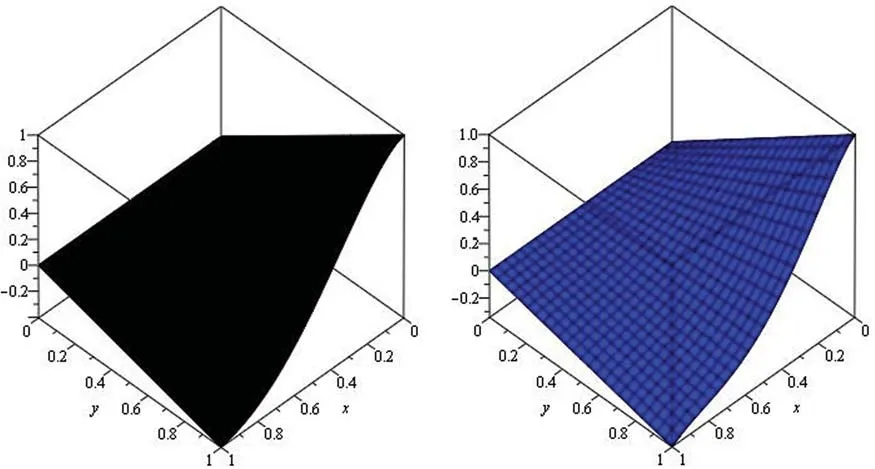
Figure 5:Illustration of solution pathways:(Left) The black figure represent the solution of Example 2 through NTDM for fractional-order ϑ=0.6 and (Right) The blue figure represent the solution of Example 2 through NTDM for fractional-order ϑ=0.4

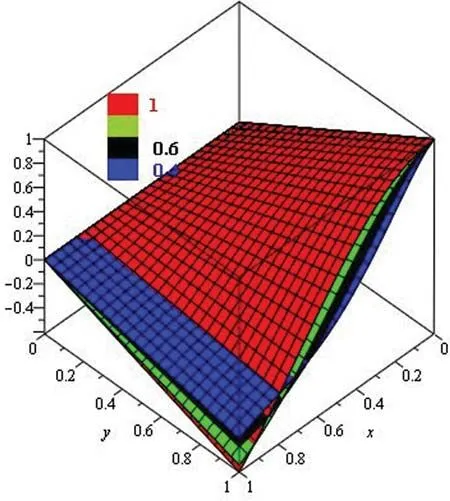
Figure 6:The solution v(x,y,t) of our proposed Example 1 through the proposed NTDM with varies values of fractional order ϑ

First of all,take the Nature transform of the above mentioned (20),we have the following

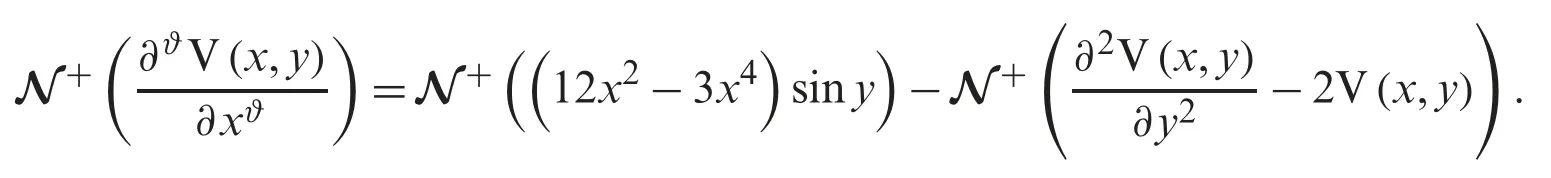
Then,the NTDM leads to the below mention

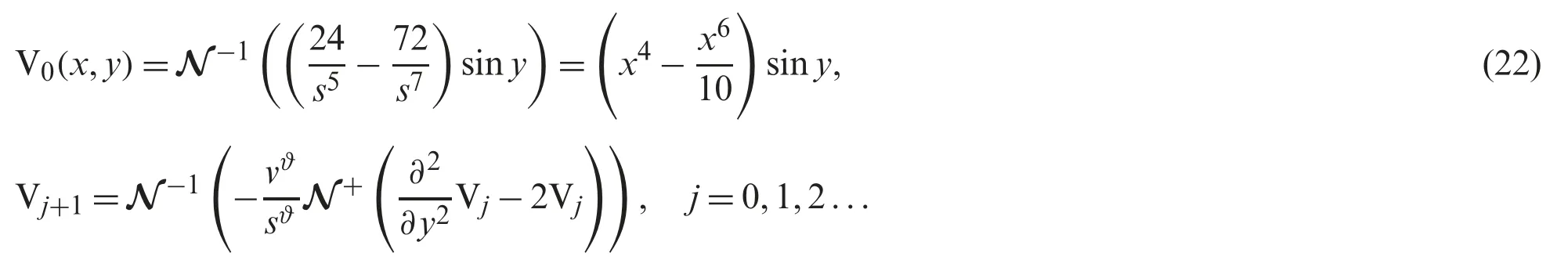
then,we have the following

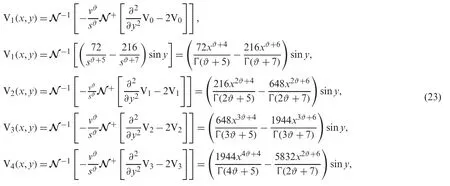
The NTDM solution of Example (3.3)

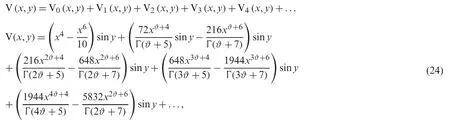
whenϑ=2,then NTDM solution is

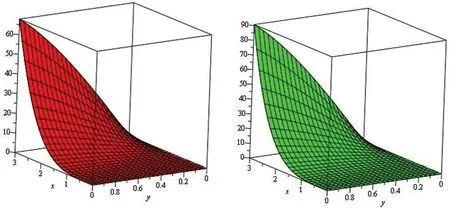
Figure 7:Illustration of solution pathways:(Left) The red figure represent the solution of Example 3 through NTDM for fractional-order ϑ=1 and (Right) The green figure represent the solution of Example 3 through NTDM for fractional-order ϑ=0.8

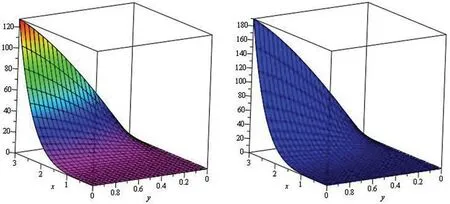
Figure 8:Illustration of solution pathways:(Left) The first figure represent the solution of Example 3 through NTDM for fractional-order ϑ=0.6 and (Right) The second figure represents the solution of Example 3 through NTDM for fractional-order ϑ=0.4

5 Results Discussion

Fig.1,represents the solution-graphs of example 1 at fractional-ordersδ=1 and 0.8,which are represented by left and right sub-graphs of Fig.1,respectively.Fig.2 is plotted to show the fractional-order solutions of example 1 at fractional-ordersδ=0.6 and 0.4 by the left and right sub-graphs,respectively.In Fig.3,the combined graph of fractional-order solutions of example 1 is presented and confirmed various dynamical behaviours of the physical phenomenon,which is modelled by the equation given in example 1.In Fig.4,two subgraphs left and right represented the solution of example 2 at fractional-orderϑ=1 and 0.8,respectively.In Fig.5,the solutions of example 2 at fractional-orderδ=0.6 and 0.4 are discussed.In Fig.6,the combined graph of fractional-orders solutions atδ=1,0.8,0.6 and 0.4 is displaced and the systematic behaviour of the solution is observed.Similarly,in Figs.6 and 7,the fractional solutions of example 3 are presented at 1,0.8 andϑ=0.6,0.4 respectively.Also,the combined graph,Fig.8,represent the solution of example 3 atϑ=1,0.8,0.6 and 0.4 collectively.Moreover,in Fig.9,the combined graphs of fractional-order solutions are represented by two subgraphs,given in Fig.9 in two and three-dimensional graphs.In both cases,we observed the correct behaviour of the solutions,which confirmed the validity of the suggested method.

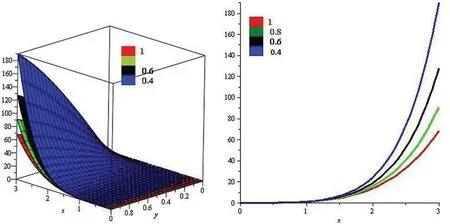
Figure 9:Illustration of the solution of Example 3 through NTDM with varies values of fractional order ϑ

6 Conclusion

In this paper,a new combination of the Adomian decomposition method with Natural transformation is made to find the analytical solutions of fractional-order partial differential equations.It is of worth interest that the implementation of the present technique is very straightforward for the solutions Helmholtz equations in its Caputo fractional-view analysis.Three numerical examples were considered for its analytical solutions by using the proposed techniques.The corresponding solutions-graphs are plotted for both fractional and integer order of the problems.The solutions revealed that the suggested method is very commited and in strong agreement with the solutions of other existing techniques.It is noted that NTDM is an easily computable,precious,accurate technique with a high rate of convergence than the other analytical methods.It is suggested that Natural transform decomposition method is the most reliable technique for solving partial differential equations in fractional framework,specifically,for fractional-order Helmholtz family of equations.The solutions of the fractional-order PDEs through NTDM are more accurate and less time consuming as compare to the ADM,VIM and DTM.

Funding Statement:Center of Excellence in Theoretical and Computational Science (TaCS-CoE)&Department of Mathematics,Faculty of Science,King Mongkut’s University of Technology Thonburi (KMUTT),126 Pracha Uthit Rd.,Bang Mod,Thung Khru,Bangkok 10140,Thailand.

Conflicts of Interest:The authors declare that they have no conflicts of interest to report regarding the present study.