Determinantal Expressions and Recursive Relations for the Bessel Zeta Function and for a Sequence Originating from a Series Expansion of the Power of Modified Bessel Function of the First Kind

Yan Hong,Bai-Ni Guoand Feng Qi

1College of Mathematics and Physics,Inner Mongolia University for Nationalities,Tongliao,028043,China

2School of Mathematics and Informatics,Henan Polytechnic University,Jiaozuo,454003,China

3School of Mathematical Sciences,Tiangong University,Tianjin,300387,China

ABSTRACT In the paper,by virtue of a general formula for any derivative of the ratio of two differentiable functions,with the aid of a recursive property of the Hessenberg determinants,the authors establish determinantal expressions and recursive relations for the Bessel zeta function and for a sequence originating from a series expansion of the power of modified Bessel function of the first kind.

KEYWORDS Determinantal representation;recursive relation;series expansion;first kind modified Bessel function;Bessel zeta function;Pochhammer symbol;gamma function;Hessenberg determinant

1 Introduction and Motivations

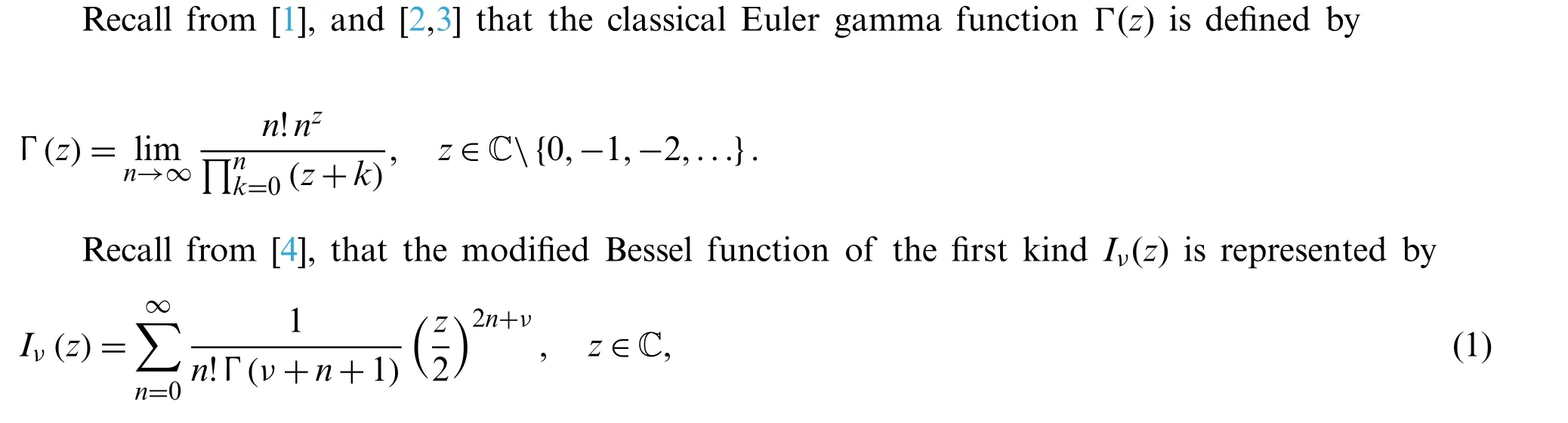
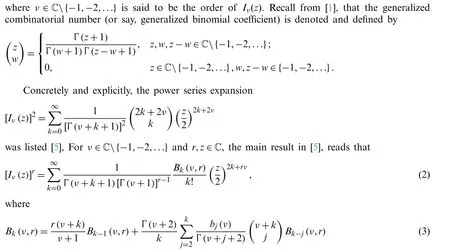
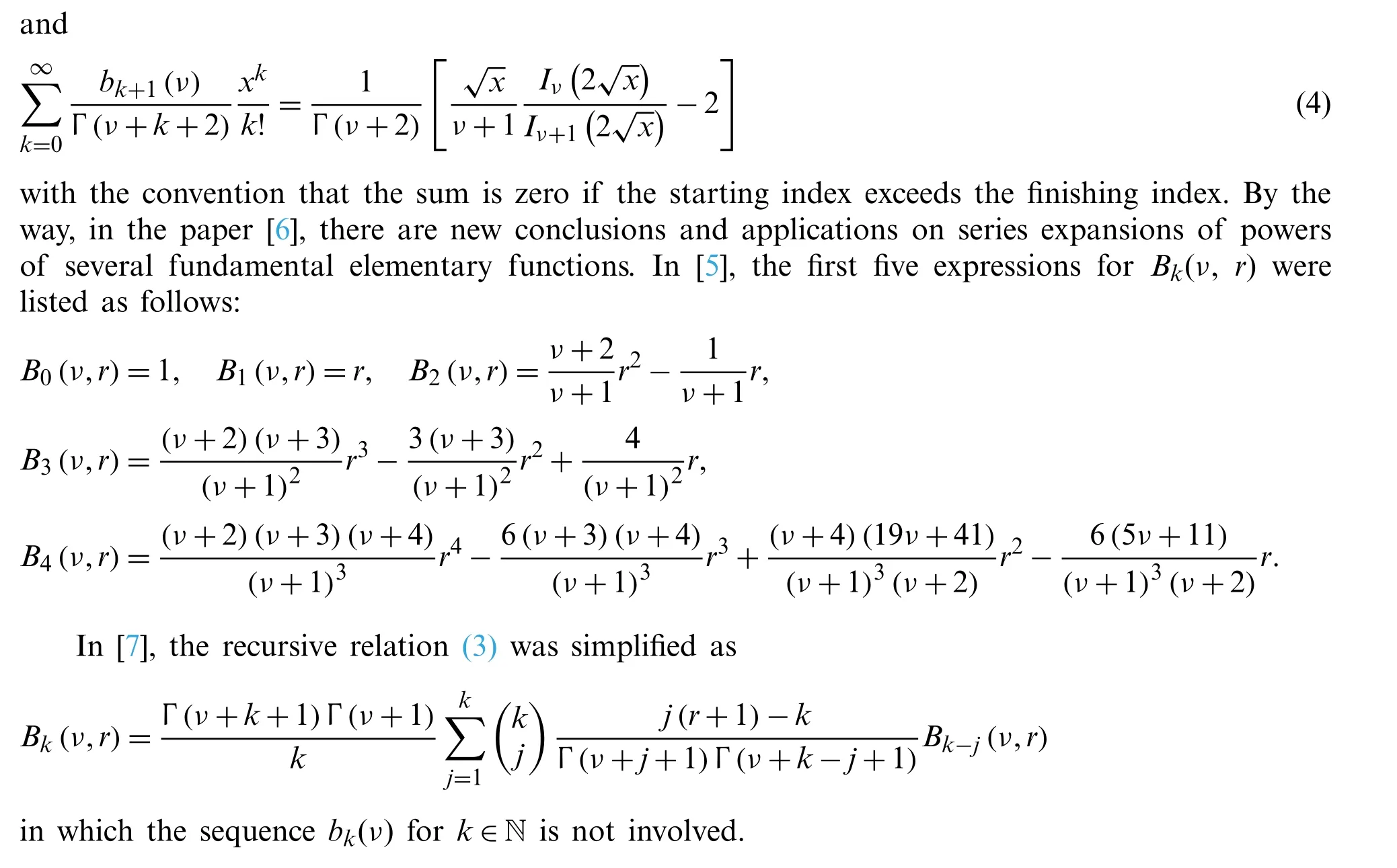
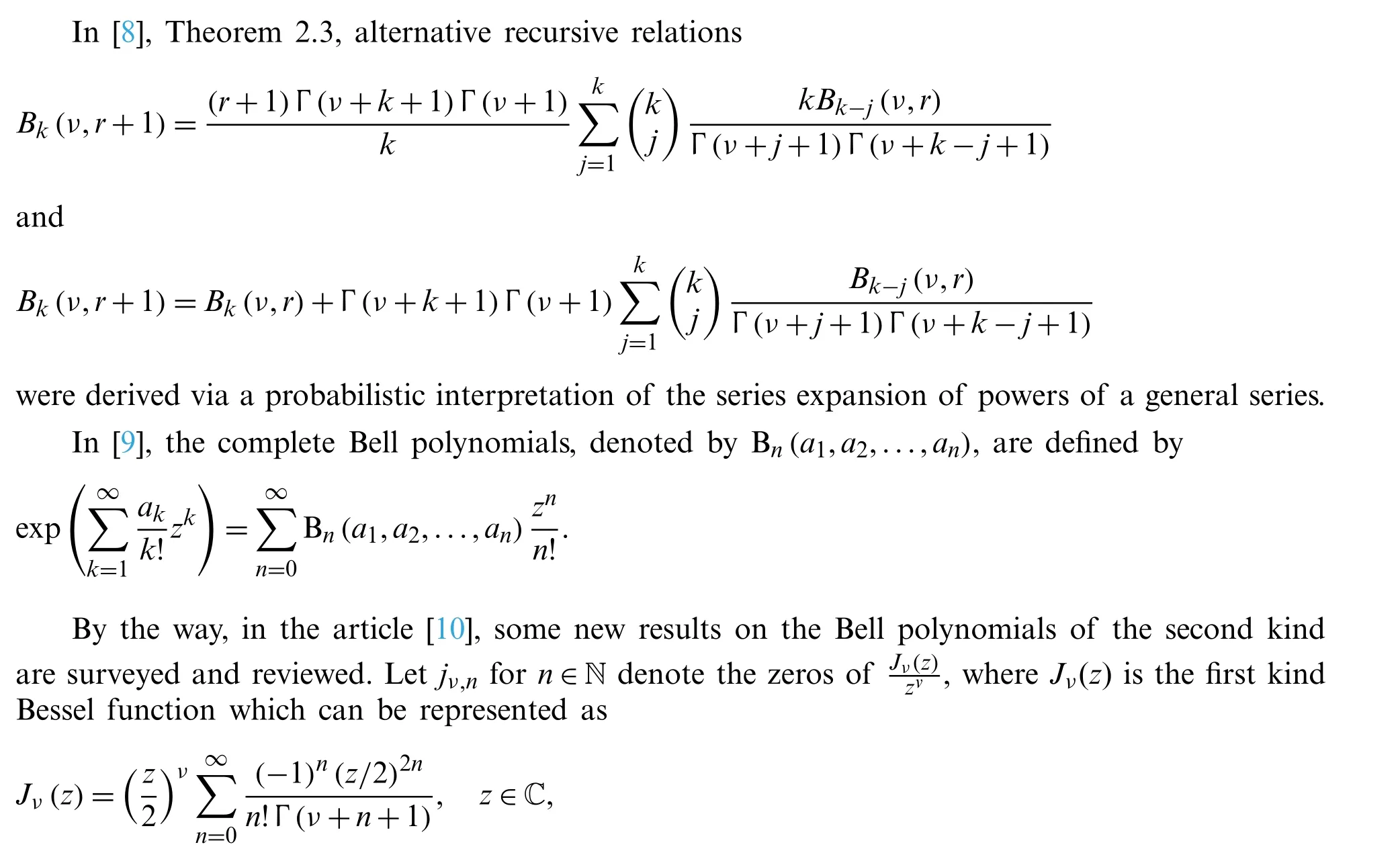
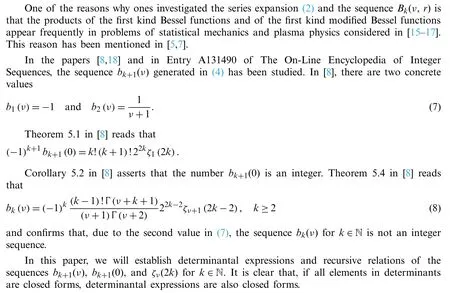
2 Determinantal Representations via Ratios of Gamma Functions

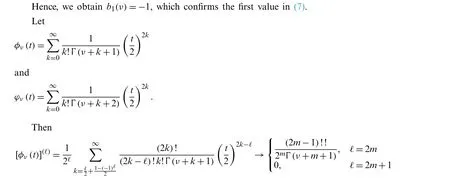
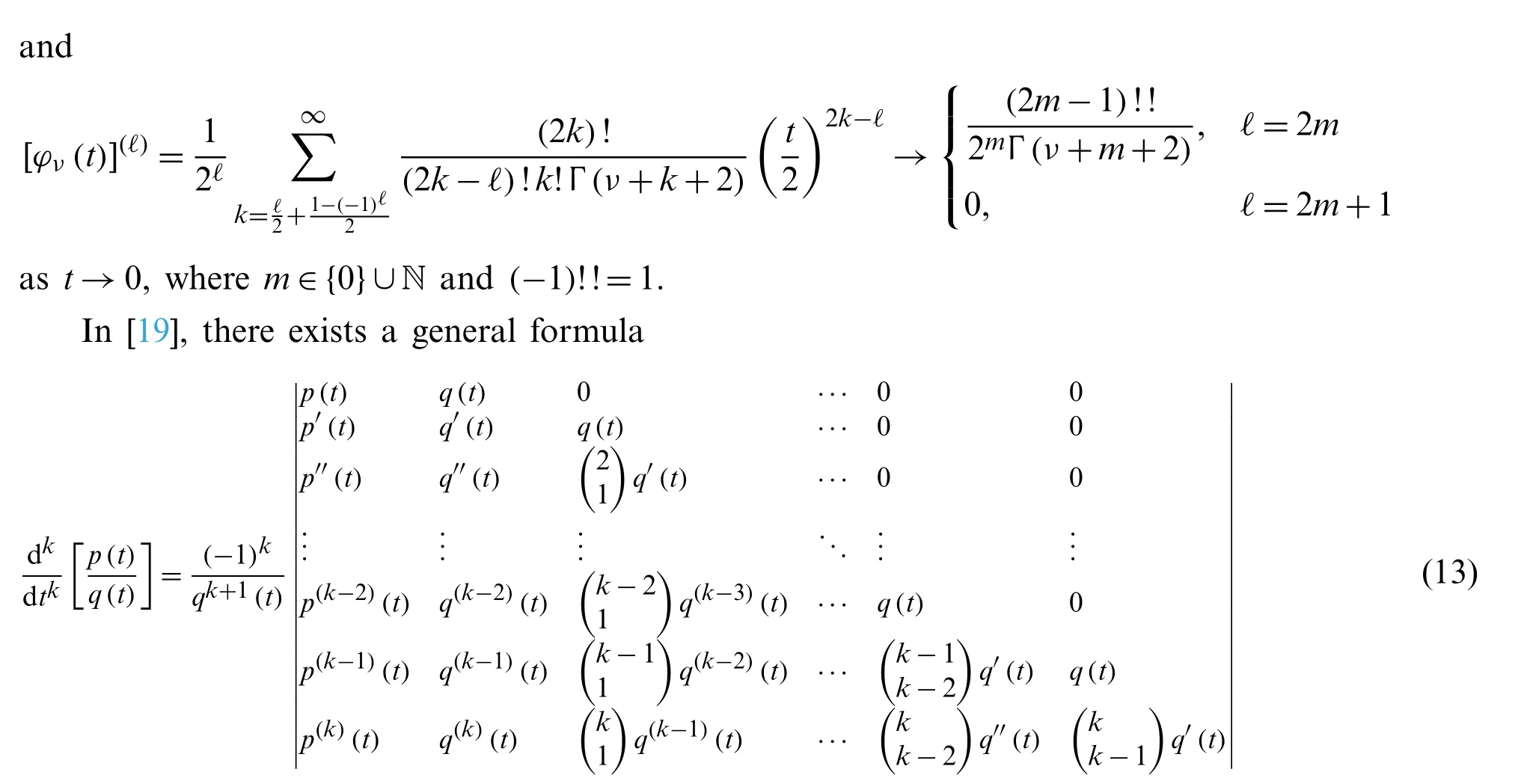
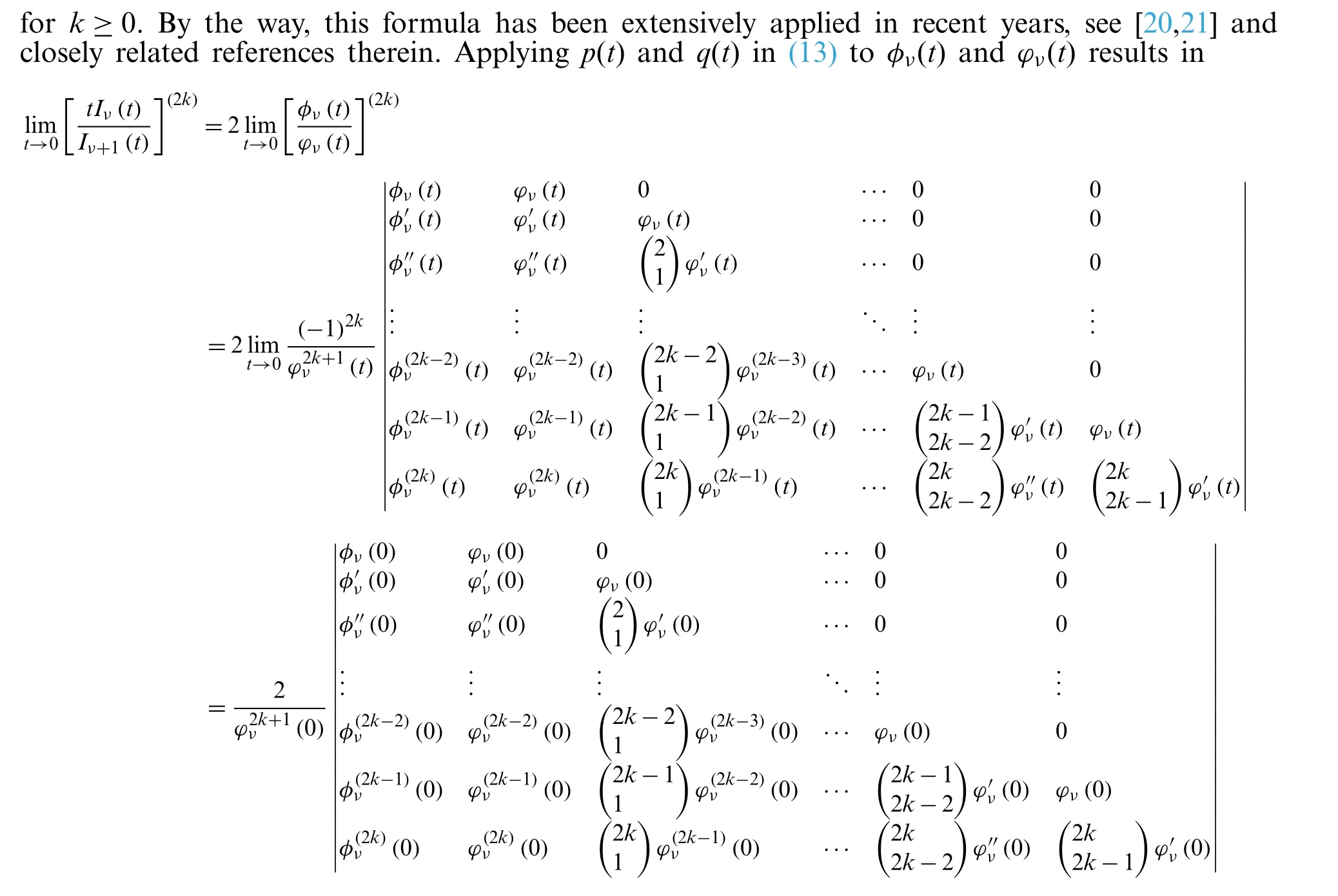
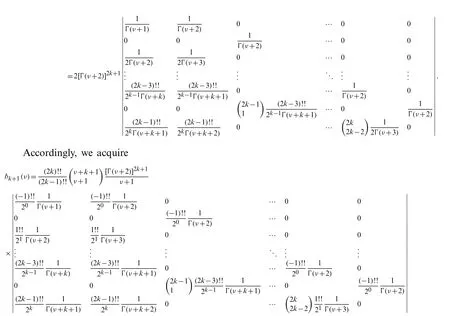
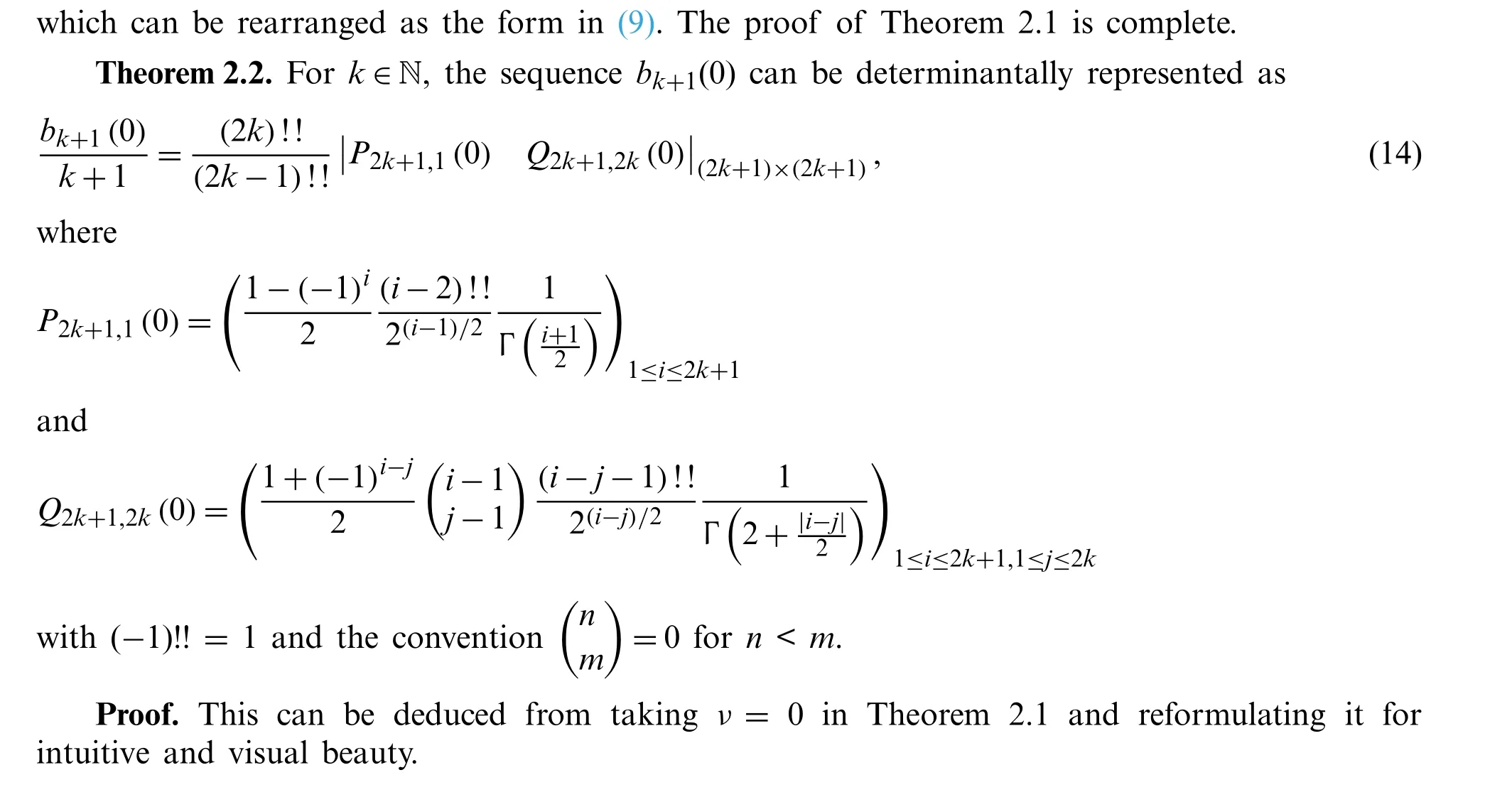
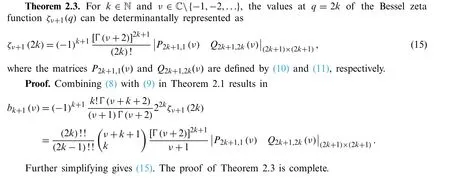
3 Determinantal Representations via the Pochhammer Symbols

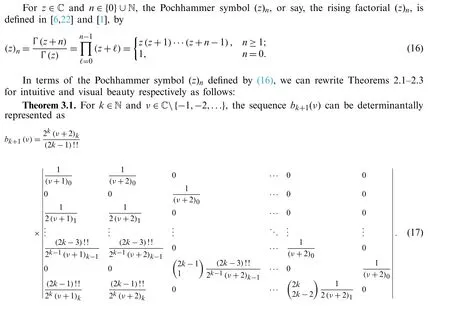
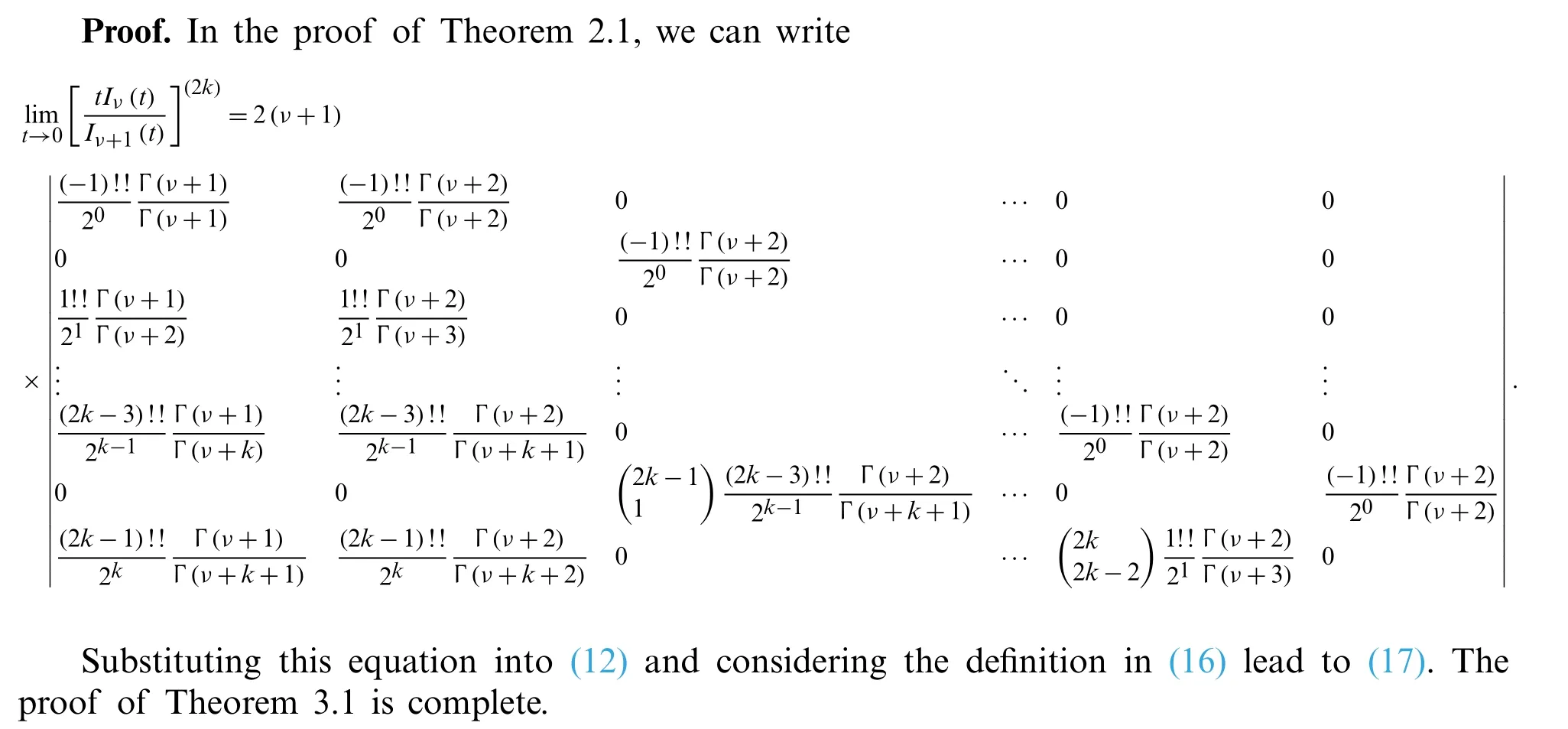
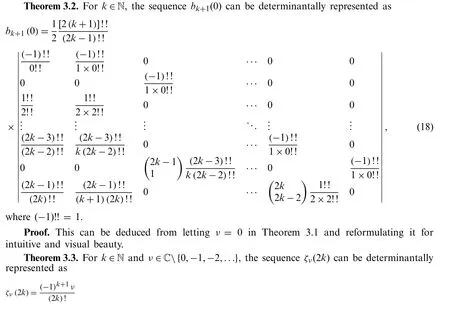
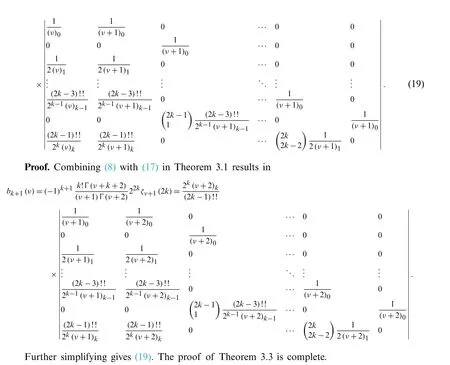
4 Recursive Relations

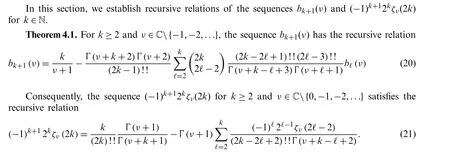
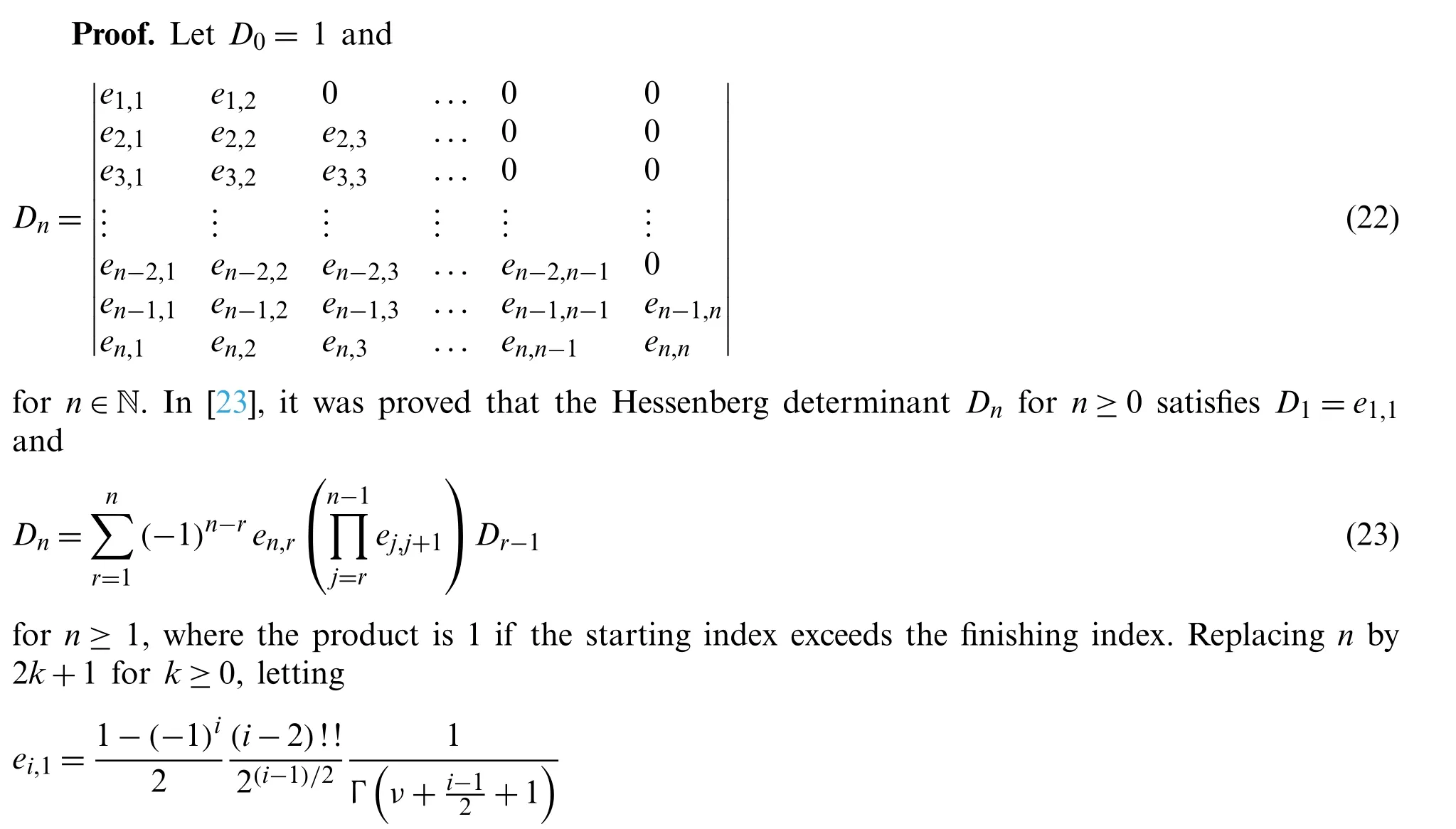
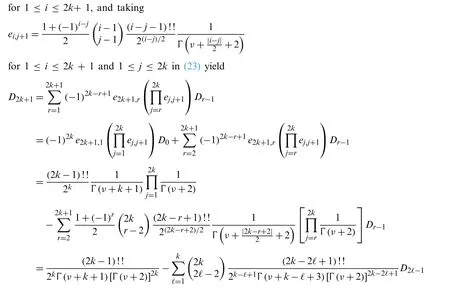
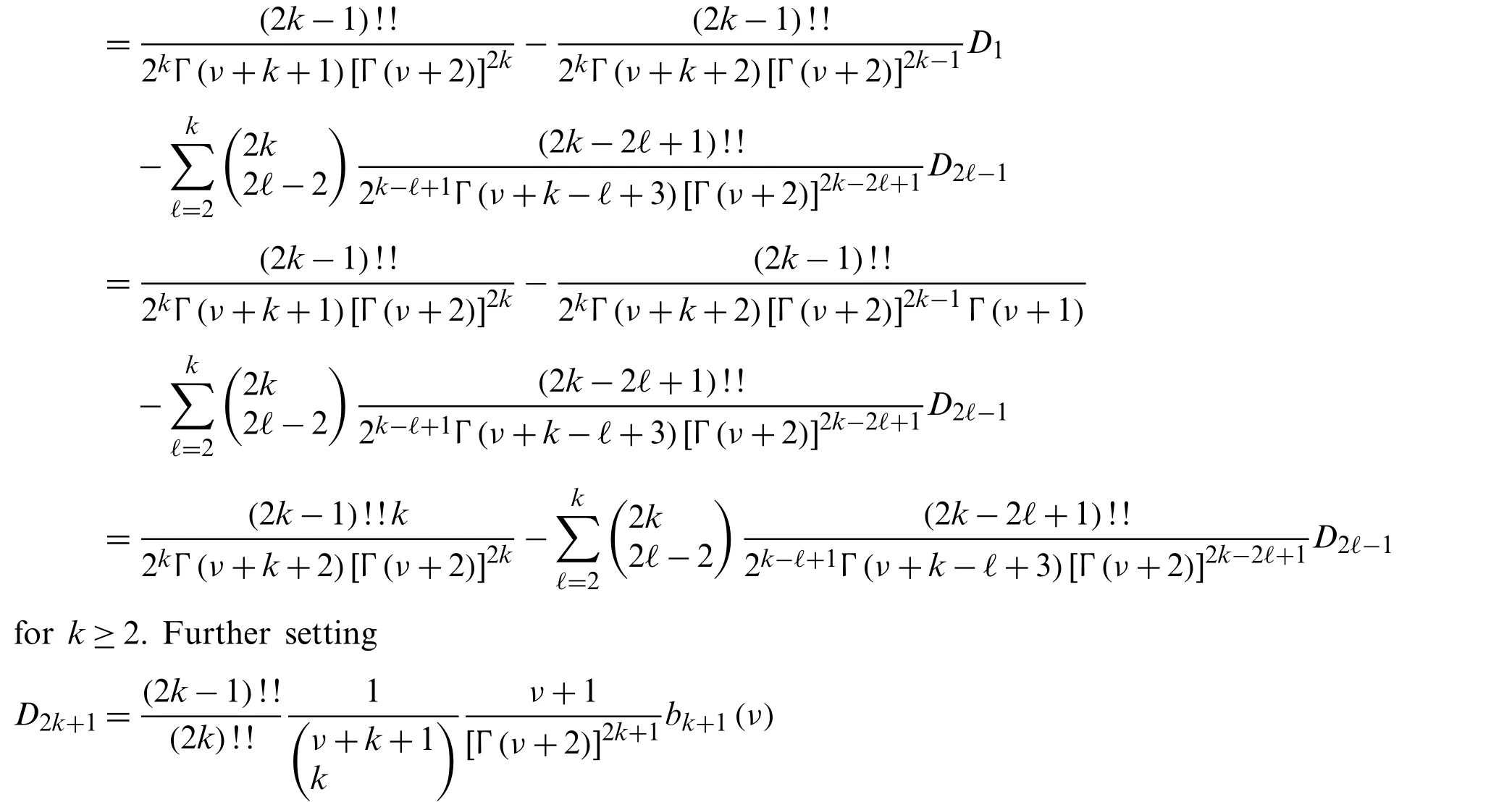
5 More Numerical Computation of the First Few Values

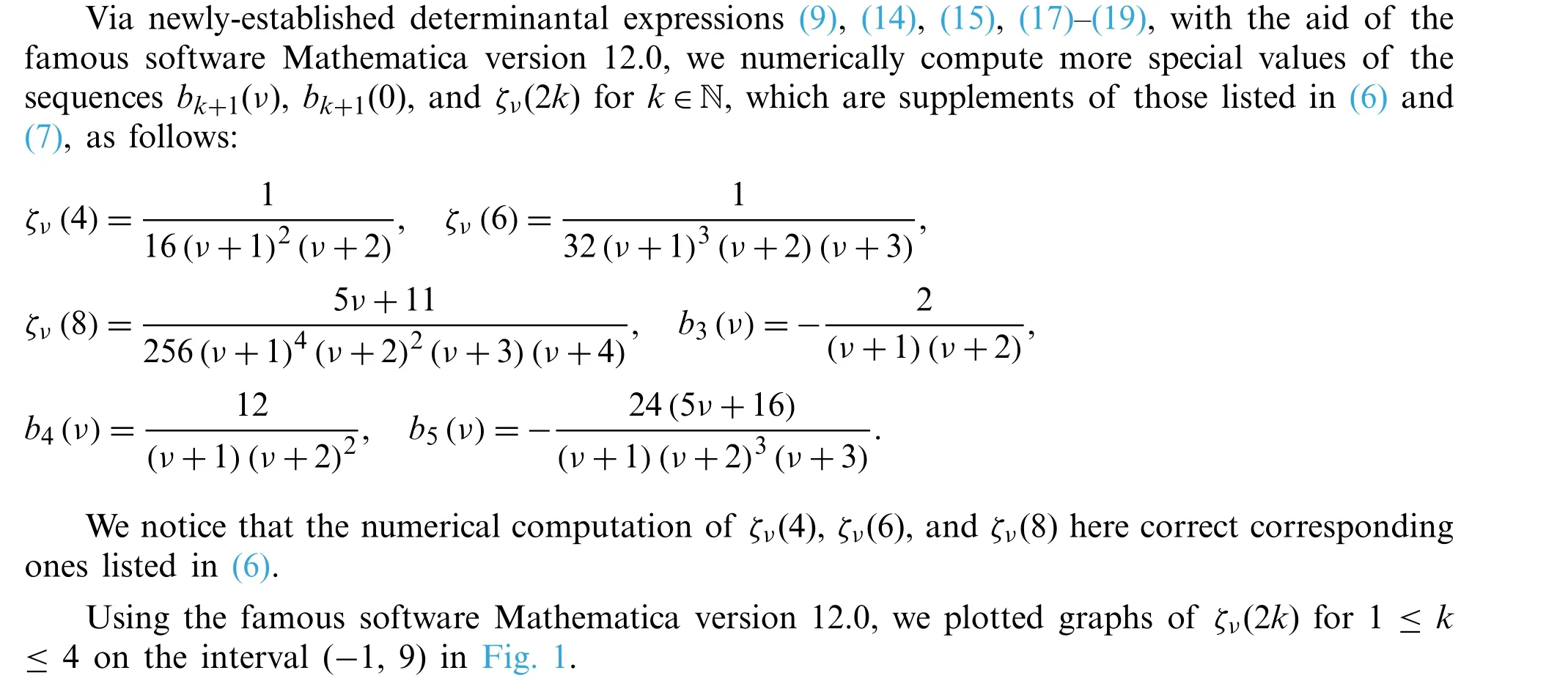
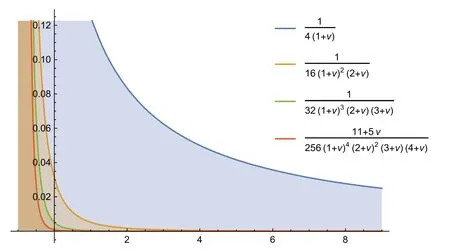
Figure 1:Graphs of ζν(2k)for 1 ≤k ≤4 on the interval(−1,9)

6 Conclusions

In this paper,by virtue of a general formula(13)for derivatives of the ratio of two differentiable functions and with the aid of a recursive property(23)of the Hessenberg determinants(22),we establish six determinantal expressions(9),(14),(15),(17)–(19),find two recursive relations(20)and(21)for the sequencebk+1(ν)defined by(4)and for the Bessel zeta functionζν(2k)defined by(5).

Acknowledgement:The authors thank 1.Jiaying Chen and Geng Li(Undergraduates Enrolled in 2018 at School of Mathematical Sciences,Tianjin Polytechnic University,China),for their valuable help downloading the papers[5,8,17]on 27 January 2021.2.Christophe Vignat(Universite d’Orsay,France;Tulane University,USA;cvignat@tulane.edu)for his sending electronic version of the paper[8]on 28 January 2021.3.Anonymous referees for their careful reading of,helpful suggestions to,and valuable comments on the original version of this paper.

Funding Statement:The first author,Mrs.Yan Hong,was partially supported by the Natural Science Foundation of Inner Mongolia(Grant No.2019MS01007),by the Science Research Fund of Inner Mongolia University for Nationalities(Grant No.NMDBY15019),and by the Foundation of the Research Program of Science and Technology at Universities of Inner Mongolia Autonomous Region(Grant Nos.NJZY19157 and NJZY20119)in China.

Conflicts of Interest:The authors declare that they have no conflicts of interest to report regarding the present study.