Steady-state teleportation fidelity and Bell nonlocality in dissipative environments
2021-08-10YuXiaXie
Yu-Xia Xie
School of Science,Xi’an University of Posts and Telecommunications,Xi’an 710121,China
Abstract We investigate quantum teleportation and Bell nonlocality for two channel qubits coupled via the Heisenberg interaction and subject to two independent dissipative environments.Compared with the case of two uncoupled qubits,it is shown that the interaction Hamiltonian is beneficial for enhancing the teleportation fidelity and Bell nonlocality,and remarkably,it can also be used to create nonclassical teleportation fidelity and Bell nonlocality even from the initial product states.Moreover,the interaction Hamiltonian guarantees the generation of steady-state nonclassical teleportation fidelity,which is independent of the initial state and therefore one can take any state as the initial channel state.
Keywords:quantum teleportation,Bell inequality,decoherence
1.Introduction
Quantum teleportation is the scheme for transmitting an unknown quantum state from one place to another with the help of local operations and classical communication[1].Different from those of the purely classical communication schemes,it does not need to transfer any particle physically,and its advantage relies crucially on the shared maximally entangled channel qubits between the sender and the receiver.In the past few decades,there have been a number of theoretical works that concentrated on studying quantum teleportation schemes[2–4],while their experimental demonstration have also been realized in various physical systems[5–10].
Apart from those perfect teleportation schemes based on different maximally entangled states[11,1,12–14],researchers also studied probabilistic teleportation using non-maximally entangled states[15]and controlled teleportation relying on the cooperation of the receiver and controllers[12].Furthermore,the connections of teleportation fidelity with Bell nonlocality[16–18],entanglement[19–21],and quantum discord[22]of the channel states were studied.Other aspects of teleportation such as constructing quantum channels for teleporting an arbitrary N-qubit state[23]and distinguishing quantum channels for nonclassical teleportation(by saying nonclassical teleportation,we mean that its fidelity is better than that achieved via any classical protocol)based on entropic uncertainty relation[3]and the nonlocal advantage of quantum coherence[24],have also been discussed.
In theory,one can implement teleportation with 100%success probability and unit fidelity,but in practice it is difficult as the preparation of maximally entangled states and the distribution of them will unavoidably be disturbed by the environments,which in general induces decoherence of the channel states[25–29],as well as decay of entanglement[30–32],Bell nonlocality[19],quantum discord[33–35],and quantum fisher information[36].Further studies show that these detrimental effects could be suppressed to some extent by virtue of the non-Markovian effects[27],correlated actions of noisy channels[37,38],and quantum-jump-based feedback[39].There are also works centered around revealing decoherence effects on quantum teleportation[40–47].In particular,in the amplitude damping channel,the decay of teleportation fidelity may be suppressed for specific channel states[48,49].The decay of teleportation fidelity can also be suppressed to some extent by virtue of the non-Markovian effects[50,51],the correlated actions of the noisy channels[52,53],and the weak measurements[54].
In this paper,we consider quantum teleportation of the one-qubit state and Bell nonlocality of the channel states in dissipative environments.Different from those of the related works which considered teleportation with the noises acting on the uncoupled channel qubits[41,40,42–47],we go one step further by considering the case for which the channel qubits are coupled via the Heisenberg interaction,and show that such an interaction can postpone decay of the teleportation fidelity and Bell nonlocality.Not only that,it can also create considerable teleportation fidelity and Bell nonlocality from the initial product states and create steady-state nonclassical teleportation fidelity which is independent of the initial states.
2.Solution of the model
We consider the system that consists of two qubits coupled via the Heisenberg interaction and subject to two independent dissipative environments.Under weak system-environment coupling and the Born-Markov approximation,its evolution is governed by the master equation[55,56]
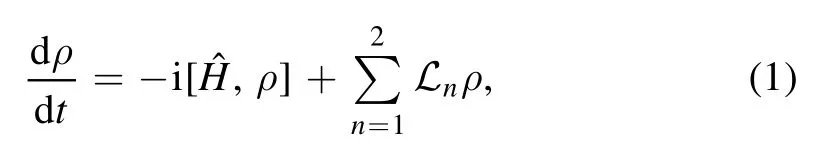
and the interaction Hamiltonian(in units ofħ)is given by
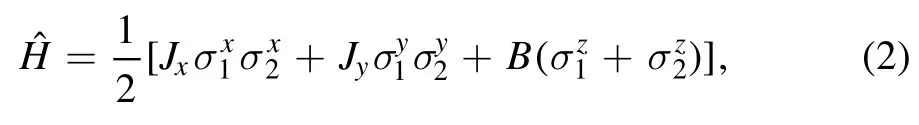
wheredenote the Pauli matrices,B is the transverse magnetic field,while Jxand Jyare the coupling constants of two qubits.We will denote by J=(Jx+Jy)/2 and Δ=(Jx-Jy)/2 for later use.Moreover,Lnρis the decoherence term of qubit n which is given by[55]

where γ is the decoherence rate of the two qubits and the Lindblad operator is given by the lowering operator
For the initial two-qubit X states(i.e.,the density operators with nonzero elements only along the main diagonal and anti-diagonal)which include the Bell and Werner states as special cases[57],the nonzero elements of ρ(t)can be obtained analytically as[58]

which remains the X type,and in the above equation,we have definedand
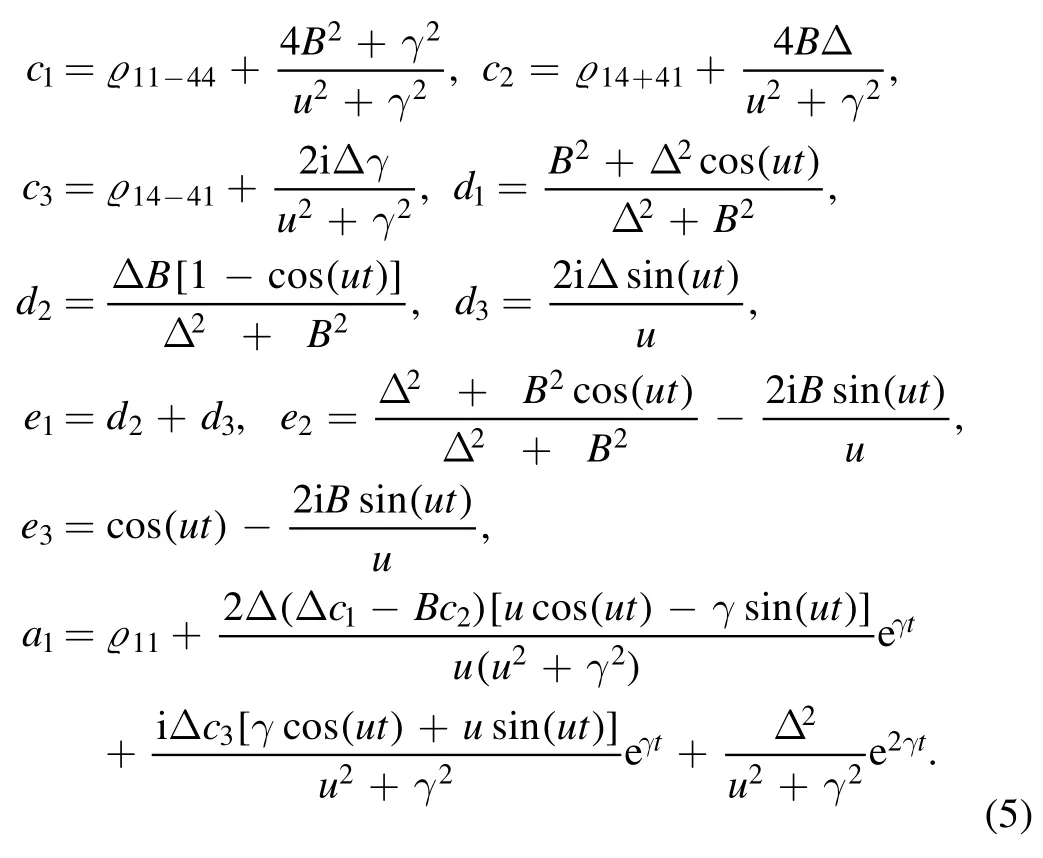
In the subsequent discussions of the average fidelity of teleportation and Bell nonlocality,we will consider all the system parameters of equation(1)in units of J.
3.Quantum teleportation in dissipative environments
In this paper,we consider teleportation of the one-qubit state(θ∈[0,π],φ∈[0,2π])[1].When a two-qubit state ρ is used as the quantum channel,the average fidelity achievable after the sender’s Bell measurements and the receiver’s optimal unitary transformation is given by[16]

where N(ρ)=‖T‖1is the trace norm of a 3×3 positive matrix T with entriesTij=tr (ρσi⊗σj).For the X-type channel state,N(ρ)can be obtained explicitly as[18]
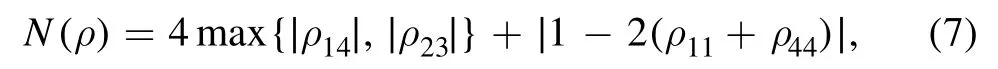
and the optimal average fidelity achievable via purely classical communication protocol is 2/3[20],hence in order to teleport|ψ〉nonclassically,we require Fav(ρ)to be larger than 2/3.By combining equations(5),(6),and(7),one can obtain that the dissipation always induce decay of Fav(ρ),and in the strong dissipation limit,Fav(ρ)will approach to its steadystate value in a very short-time scale.
In the following,we concentrate on the cases that the channel qubits are prepared initially in the Bell states

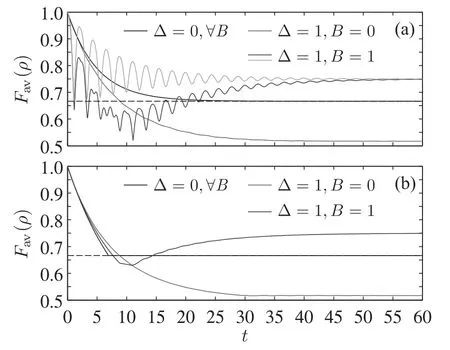
Figure 1.Fav(ρ)versus t with γ=0.1 and differentΔand B.(a)The blue(green)line corresponds to the initial state|Ψ+〉(|Ψ-〉),and the other solid lines correspond to|Ψ±〉.(b)All the solid lines correspond to|Φ±〉.The horizontal dashed lines show the critical Fav(ρ)=2/3.
and the product states|00〉and|11〉,for which one has22=33and23=32,then one can obtain from equation(4)that ρ(t)is independent of the system parameter J for these initial states.For the similar models,the dissipative effects on entanglement[31],quantum discord[58],and measurement-induced nonlocality[59]were investigated,while for the special case of two uncoupled qubits,its effects on entanglement[30,60,61]and teleportation[62]were also studied.
In figure 1,we show time dependence of Fav(ρ)for the initial Bell states with different system parameters.When Δ=0,the average fidelity can be obtained as

then one can see that for the initial states|Ψ±〉,Fav(ρ)decreases exponentially from its maximum 1 to the classically limiting value of 2/3,whereas for the initial states|Φ±〉,Fav(ρ)first decays exponentially to 2/3 at the critical timeand will remains the constant 2/3 when t>tc(see the solid black lines displayed in figure 1).When B=0,however,one can see from the red lines shown in figure 1 that for all the initial Bell states,Fav(ρ)turns out to be decreased monotonically from its maximum 1 to its asymptotic value.For the general values ofΔand B,the dependence of Fav(ρ)on time t will be different for the initial states|Ψ+〉and|Ψ-〉.As is exemplified in figure 1(a),Fav(ρ)oscillates with the time t,and for|Ψ-〉,it is always larger than 2/3,whereas for|Ψ+〉,it becomes smaller than 2/3 in the intermediate regions of time.Moreover,Fav(ρ)for|Ψ-〉is stronger than that for|Ψ+〉,and apart from the short-time region,it is also stronger than that for the two uncoupled qubits(i.e.,J=Δ=0).For the initial states|Φ±〉,as is shown by the solid blue line in figure 1(b),Fav(ρ)is smaller than 2/3 only in the intermediate region of t,and apart from this time region,it is also larger than that for the two uncoupled qubits.All these show evidently that the interaction Hamiltonian is beneficial for enhancing resistance of the channel against detrimental effects of the dissipative environments.
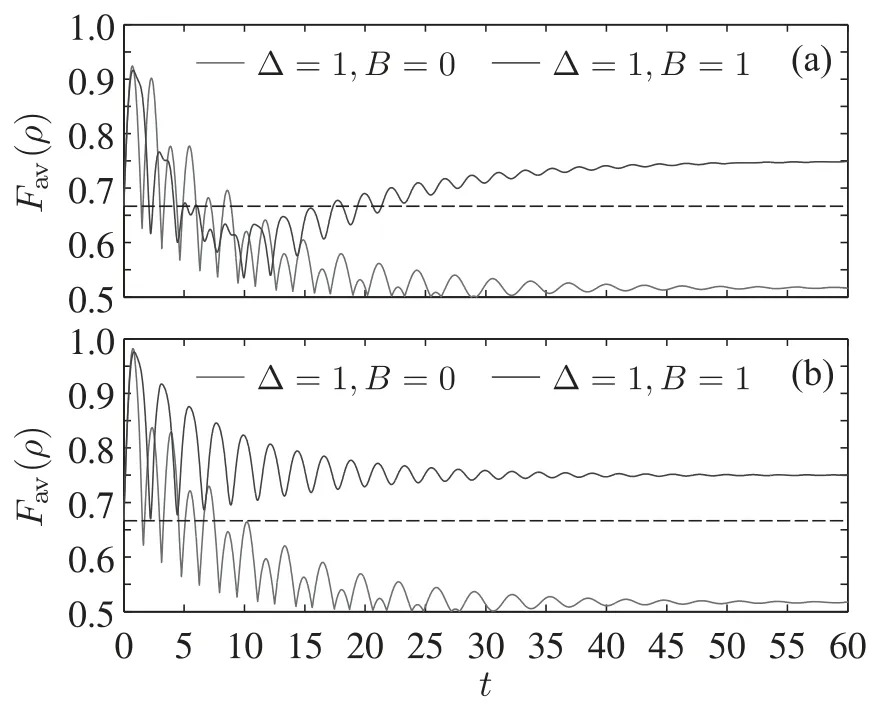
Figure 2.Fav(ρ)versus t for the initial states|00〉(a)and|11〉(b)with γ=0.1 and differentΔand B.The horizontal dashed lines show the critical Fav(ρ)=2/3.
Next,we consider Fav(ρ)for the initial states|00〉and|11〉.The results show that whenΔ=0,it is independent of B and is always smaller than 2/3(for|00〉)or remains the constant of 2/3(for|11〉).WhenΔ≠0,we exemplify in figure 2 time dependence of Fav(ρ)with different B.It can be seen that Fav(ρ)first increases from 2/3 to its peak value and then oscillates with the time t evolves.Such a peak value for the initial state|11〉is obviously larger than that for|00〉.This shows evidently that there is nonclassical teleportation fidelity being created during the time evolution process.
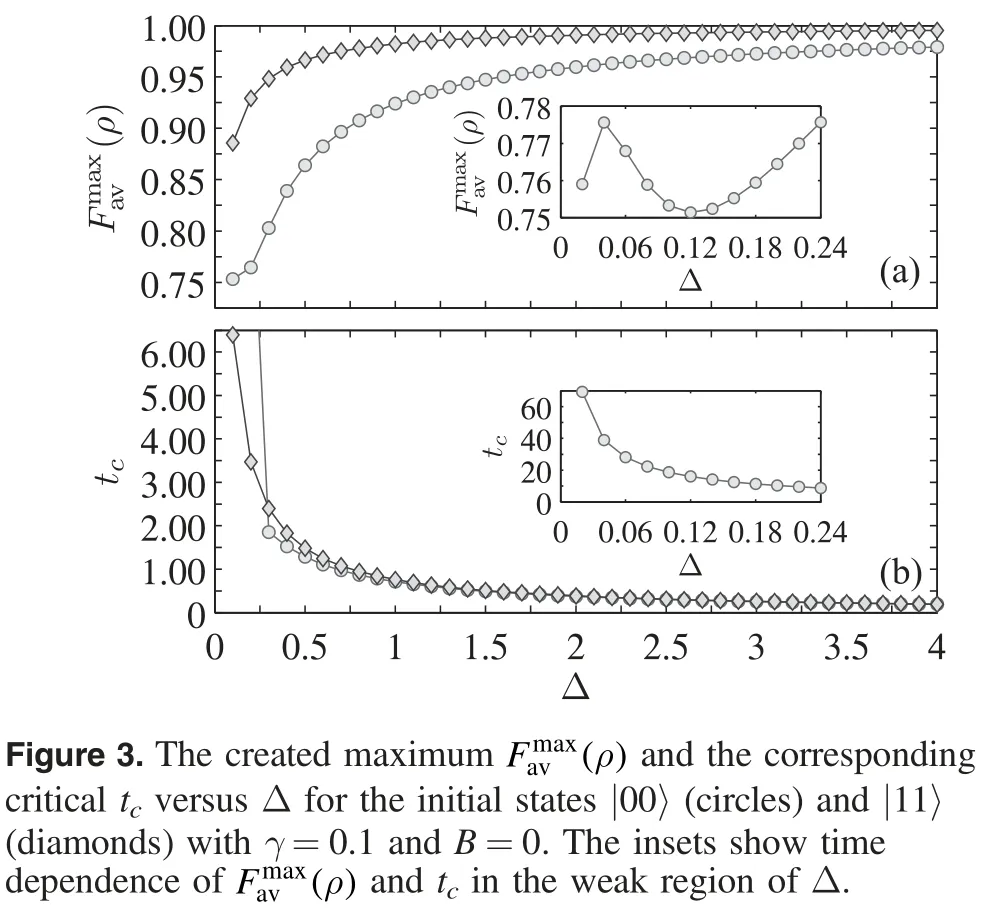
To further show the positive role of the interaction Hamiltonian in creating nonclassical teleportation fidelity,in figure 3 we show theΔdependence of the maximum average fidelitycreated from|00〉and|11〉and the critical time tcat which one attainsIt can be seen that apart from the weakΔregion,increases with the increase ofΔand approaches asymptotically to its maximum 1,whereas tcalways decreases with an increase inΔ.Moreover,the lines shown in figure 3(a)consolidates the observation of figure 2 that the initial state|11〉is more efficient than|00〉in creating nonclassical teleportation fidelity.


from which one can see that for the initial Bell states,Fav(ρ)is determined solely by the dissipation,while for the initial product states|00〉and|11〉,it will be determined by the competition between the dissipation and the spin interaction.In particular,it is direct to see that at t≃π/4Δ,ρ(t)will be very close to the maximally entangled statesand|00〉,respectively,hence one achieves the maximum teleportation fidelity in the infinite limit ofΔ.
From figures 1 and 2,one can also see that in the infinitetime limit,Fav(ρ)approaches to its steady-state value.In fact,when t→∞,one can obtain from equation(4)the nonzero elements of the steady-state ρssas

from which one can obtain
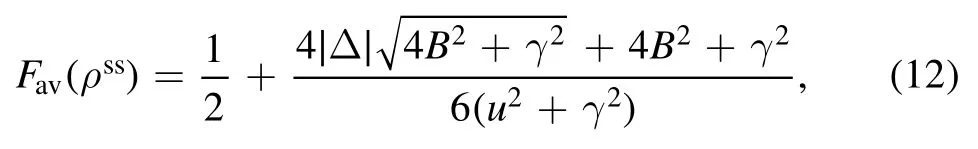
then it can be seen that the steady-state Fav(ρss)is independent of the initial states,and it is only determined by the anisotropic parameterΔ,the transverse magnetic field B,and the decoherence rate γ.ForΔ<γ,one can obtain that Fav(ρss)is always larger than 2/3.ForΔ≥γ,as is shown in figure 4(a),Fav(ρss)first increases with the increase of B and reaches its classical limiting value of 2/3 att hen it continuously increases to its maximumat another critical field Bc,after which it turns out to be decreased to the asymptotic value of 2/3.From equation(12),one can obtain the maximum of Fav(ρss)as
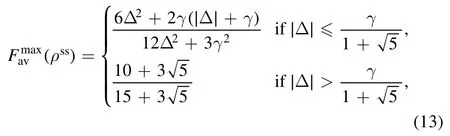
and the corresponding Bcat which Fav(ρss)takes its maximum is given by

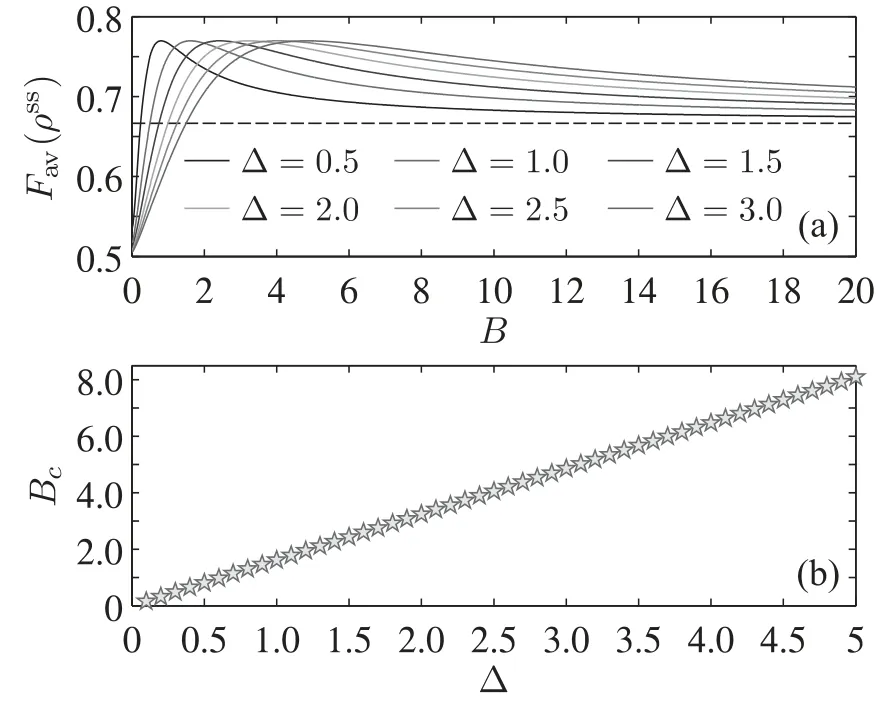
Figure 4.The steady-state Fav(ρss)versus B with differentΔ(a)and theΔdependence of the critical Bc at which Fav(ρss)takes its maximum(b)with γ=0.1.The horizontal dashed line in(a)shows the critical Fav(ρ)=2/3.
From equation(13),one can note that the maximum steady-state average fidelitywhen Δ0.3090γ,which is independent of B and is larger than the classical limiting value of 2/3.This shows that although in the short-time region,the dissipative environments induce decay of the average teleportation fidelity,the interaction Hamiltonian dominates in the long-time region.As for Bcat which Fav(ρss)takes its maximum,one can obtain from equation(14)that Bc≃1.6180ΔwhenΔ≫γ,which increases nearly linearly with the increase ofΔ,see,e.g.,the five-pointed stars shown in figure 4(b).
For ρssof equation(11),one can obtain its concurrence(a measure of two-qubit entanglement[63,64])as

then one can obtain that forΔ∈(0,γ),C(ρss)is always larger than 0,whereas forΔ≥γ,C(ρss)>0 in the region of B>Bc1.Moreover,C(ρ)takes its maximum at exactly the same critical magnetic field Bcfor that ofThis indicates that ρssguarantees a nonclassical teleportation fidelity whenever there is quantum entanglement in it,i.e.,the promotion in Fav(ρss)is a consequence of the enhanced steady-state entanglement C(ρss).But it should be note that this is not a general conclusion as for certain non-maximally entangled channel states,its entanglement cannot completely determine average fidelity of the teleportation[42].
4.Bell nonlocality in dissipative environments

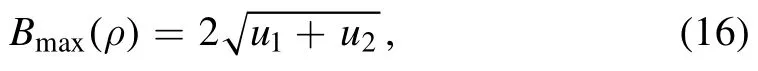
with u1≥u2≥u3denoting the eigenvalues of T†T.For the X state,Bmax(ρ)can be obtained explicitly as[18]

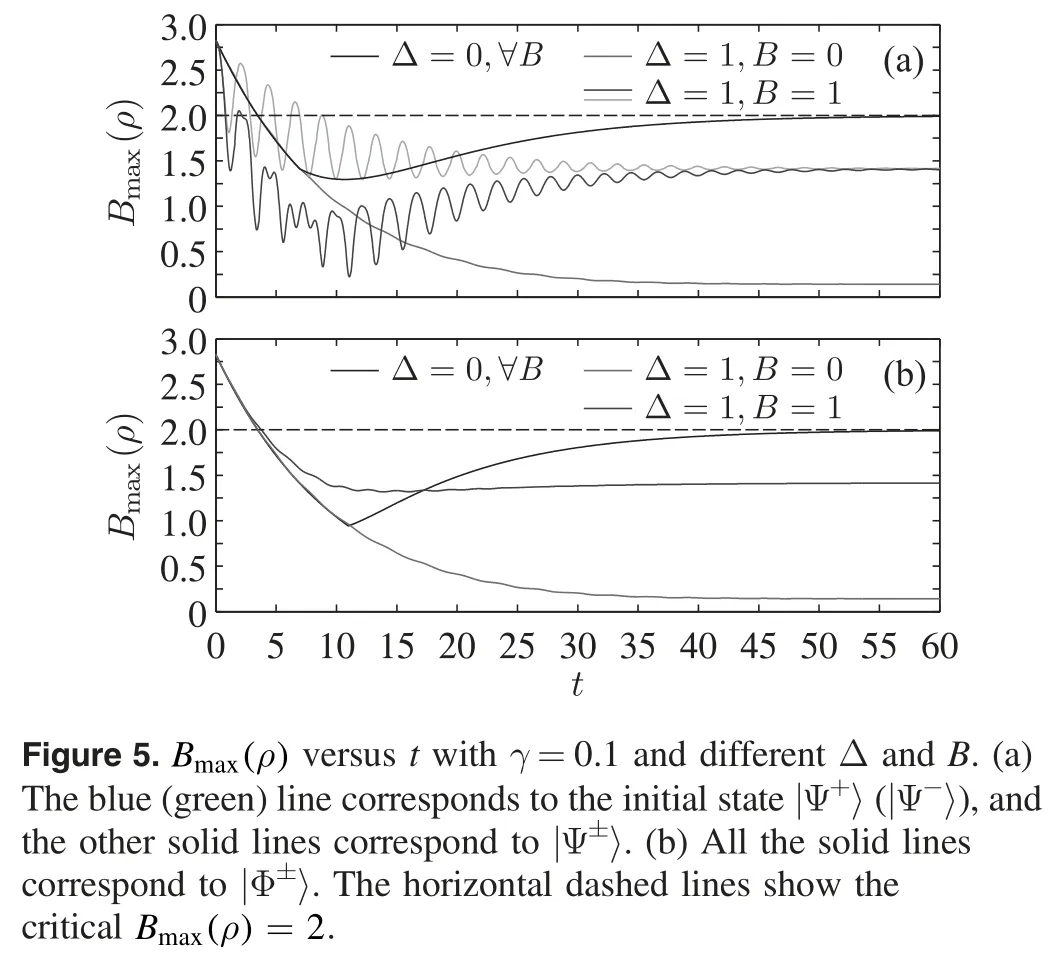
In figure 5,we show the time dependence ofBmax(ρ)with the same parameters of figure 1.WhenΔ=0,one can obtain

where p=e-γtand′=-p1p.From equation(18),one can obtain that the Bell nonlocality disappears aftert>lnsee the black lines shown in figure 5.So Fav(ρ)>2/3 even when there is no Bell nonlocality,and this confirms the fact that the existence of Bell nonlocality is a sufficient but not necessary condition for achieving the nonclassical teleportation[16].If B=0,however,Bmax(ρ)decays monotonically fromto its asymptotic value of 0 with the increase of time t,whereas for nonzero values ofΔand B,as is shown in figure 5,Bmax(ρ)oscillates with the evolving time,and its strength for the initial state|Ψ-〉is stronger than that for|Ψ+〉.Moreover,the solid black lines shown in figure 5 also correspond to the case of two uncoupled qubits(i.e.,J=Δ=0),then one can see that for the initial states|Ψ-〉and|Φ±〉,the interaction Hamiltonian is also helpful in enhancing the Bell nonlocality.
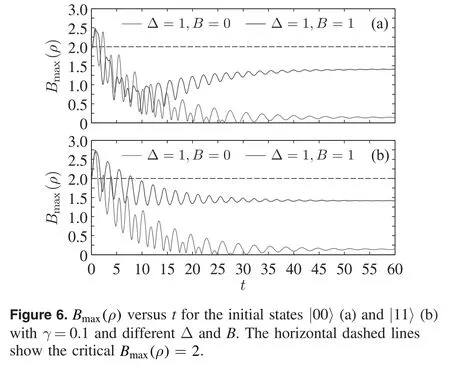
In figure 6 we show time dependence ofBmax(ρ)for the initial states|00〉and|11〉.One can see that the interaction Hamiltoniancan also be used to create considerable Bell nonlocality.Similar to Fav(ρ),the created maximumBmax(ρ)from|11〉is stronger than that from|00〉.Moreover,one can obtain from equation(10)that the created maximumBmax(ρ)approaches to the maximumwhenΔ→∞.
When considering the steady-state ρssof equation(11),Bmax(ρss)can be obtained analytically as
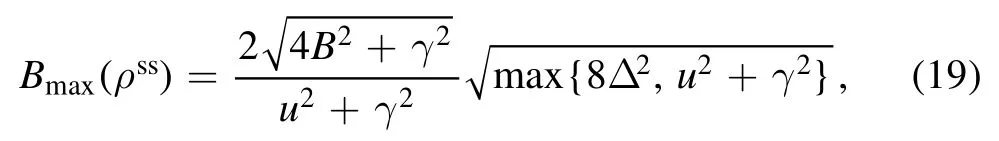
then one can obtain that for anyΔ,Bmax(ρss)is always a monotonic increasing function of B and takes its maximum 2 when B approaches infinity,hence there is no Bell nonlocality in the steady-state ρss.
5.Summary
In summary,we have investigated the fidelity of quantum teleportation and Bell nonlocality of the channel states by solving analytically the Lindblad master equation.The central system serving as the quantum channel consists of two qubits that are coupled via the Heisenberg interaction and are subject to two independent dissipative environments.For the channel qubits prepared initially in the Bell states which have maximum entanglement and the product states|00〉and|11〉which have no any correlation,we analyzed in detail dynamics of the teleportation fidelity Fav(ρ)and the Bell nonlocalityBmax(ρ).Compared with the case of two uncoupled qubits,both Fav(ρ)andBmax(ρ)can be noticeably enhanced due to the Heisenberg interaction.Not only that,such an interaction can also be used to create nonclassical teleportation fidelity and Bell nonlocality even from the initial product states,and the created Fav(ρ)andBmax(ρ)approach asymptotically to their maxima with the increasing anisotropic parameter.Moreover,in the infinite-time limit,the steady-state teleportation fidelity and Bell nonlocality are remarkably independent of the initial state.In particular,the maximum achievable steady-state teleportation fidelity is always larger than 2/3.All these results show that an efficient monitoring of quantum teleportation and Bell nonlocality in dissipative environments is possible by coupling the two channel qubits via the Heisenberg interaction.
Acknowledgments
This work was supported by the National Natural Science Foundation of China(Grant No.11675129).
杂志排行
Communications in Theoretical Physics的其它文章
- The relation between the radii and the densities of magnetic skyrmions
- Influences of flexible defect on the interplay of supercoiling and knotting of circular DNA*
- Understanding sequence effect in DNA bending elasticity by molecular dynamic simulations
- Influence of relative phase on the nonsequential double ionization process of CO2 molecules by counter-rotating two-color circularly polarized laser fields
- The upper bound on the tensor-to-scalar ratio consistent with quantum gravity
- Hawking radiation and page curves of the black holes in thermal environment
