Hawking radiation and page curves of the black holes in thermal environment
2021-08-10RanLiandJinWang
Ran Li and Jin Wang
1 School of Physics,Henan Normal University,Xinxiang 453007,China
2 Department of Chemistry,SUNY,Stony Brook,NY 11794,United States of America
3 Department of Chemistry and of Physics and Astronomy,State University of New York at Stony Brook,Stony Brook,NY 11794-3400,United States of America
Abstract As realistic objects in the Universe,the black holes are surrounded by complex environment.By taking the effect of thermal environment into account,we investigate the evaporation process and the time evolutions(page curves)of the entanglement entropies of Hawking radiation of various types of black holes.It is found that the black holes with the thermal environments evaporate slower than those without the environments due to the environmental contribution of the energy flux in addition to Hawking radiation.For Schwarzschild black hole and Reissner-Nordström black hole in flat spaces,when the initial temperature of the black hole is higher than the environment temperature,the black holes evaporate completely and the Hawking radiation is eventually purified.For Schwarzschild-AdS black hole,it will evaporate completely and the Hawking radiation is purified when the environment temperature is lower than the critical temperature.Otherwise,it will reach an equilibrium state with the environment and the radiation is maximally entangled with the black hole.Our results indicate that the final state of the black hole is determined by the environmental temperature and the temporal evolution and the speed of the information purification process characterized by the page curve of the Hawking radiation is also influenced by the thermal environment significantly.
Keywords:Hawking radiation,page curve,black hole,thermal environment,entanglement entropy
1.introduction
It is well known that Hawking radiation of the black hole[1]leads to a paradox between quantum mechanics and general relativity.Hawking argued that the evaporation process is inconsistent with the quantum principle that the evolution of quantum states is unitary and therefore information should be conserved[2],which was referred to as the black hole information paradox.It has attracted attention with a large amount of studies over the past forty years(refer to[3–7]for some recent reviews on this topic).
The black hole is assumed to be in a pure state initially.Therefore,due to the requirement of unitarity of quantum mechanics,the total system of the black hole and the radiation is also in pure state during the evaporation process.Note that the entanglement entropy and the information of the total system of the black hole and the radiation are conserved without any change under this assumption.However,The entanglement entropy of the subsystem as the black hole or the radiation can change during the process.The entropy flux of Hawking radiation is proportional to black hole temperature or inversely proportional to the mass or the size of the black hole.The entanglement entropy of the Hawking radiation(which is equal to that of the black hole)increases from zero in the early stage because the emitted radiation quanta is entangled with the interior Hawking quanta.On the other hand,the Bekensten−Hawking entropy of the black hole which is characterized by the area of the event horizon decreases during the radiation process.This may seem to lead to a conflict when the Bekenstein−Hawking entropy(which is a type of thermodynamic entropy or coarse-grained entropy)becomes less than the entanglement entropy(the fine-grained entropy)of the black hole.The rational behind is that the coarse-grained entropy,which is a measure of the total degrees of freedom of the system,sets an upper bound on the entanglement entropy,i.e.the fine-grained entropy can not be bigger than the coarse-grained entropy[7].
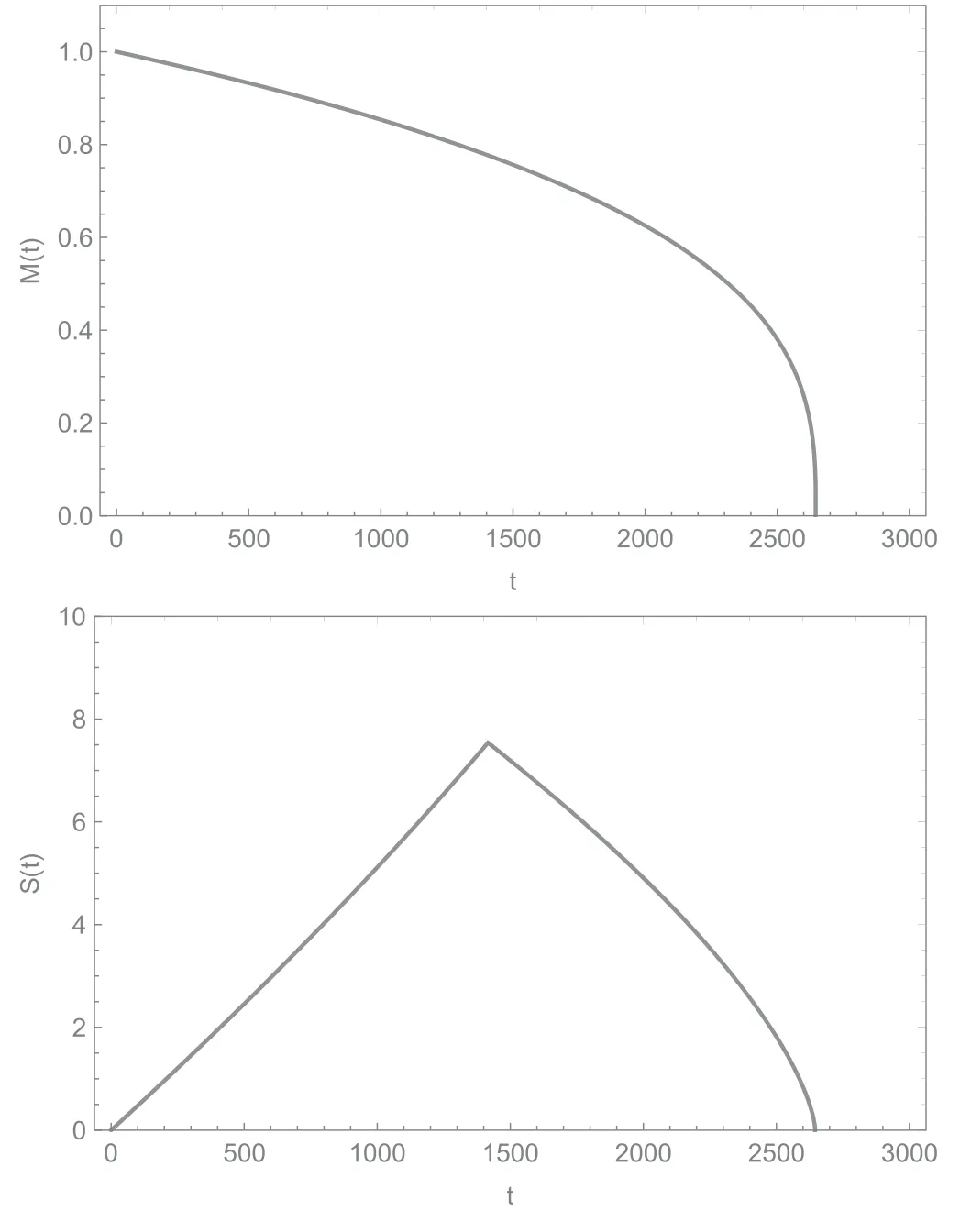
Figure 1.Mass of Schwarzscild black hole and von Neumann entropy of Hawking radiation as the functions of time t.For simplicity,the initial black hole mass M0 and the radiation constant σ are set to 1 and β is set to 1.5.
To resolve this conflict,Page suggested that the entanglement entropy of the radiation should start decreasing at the Page time when the entanglement entropy of the radiation is equal to the Bekenstein−hawking entropy[8,9].The entanglement entropy of the Hawking radiation should come back to zero and the radiation should be in a pure state again when the black hole evaporates completely.The temporal evolution curve of the radiation is conventionally referred to as page curve.It is often believed that the black hole information paradox can be resolved if the page curve for the temporal evolution of the entanglement entropy of the Hawking radiation of the black hole is correctly quantified.Recent significant progresses were made by Penington et al[10,11]and Almheiri et al[12–15],where the entanglement entropy of the Hawking radiation was calculated by taking into account of the contribution of the island inside or near the event horizon.
As realistic objects in the Universe,the black holes are surrounded by complex environment,for example the cosmic microwave background,which is the electromagnetic radiation as a remnant from an early stage of the Universe[16].Therefore it is important to investigate the effect of the environment on the evaporation process of the black hole(primordial black holes in particular).As a consequence,the time evolution curve of the entanglement entropy of Hawking radiation will also be affected by the environment.
In this paper,we will consider this aspect in a simple setup where the environment is taken to be a thermal reservoir made of radiation fields at a constant temperature.It should be noted that the environment is generally assumed to be with infinite number of degrees of freedom.Although the Hawking evaporation is a dynamical process,the backreaction of the radiation on the thermal environment can usually be ignored under the present assumptions.We reconsider the evaporation process and the time evolution curves of the entanglement entropies of the Hawking radiation.It is found that the black holes with the environments evaporate slower than those without the environments.This is due to the environment contribution of the energy flux in addition to Hawking radiation.For Schwarzschild black hole and Reissner-Nordström black hole in flat spaces,when the initial temperature of black hole is higher than the environment temperature,the black holes evaporate completely and the Hawking radiation is purified finally.In addition,the black hole does not evaporate if the temperature of the environment is higher than the initial temperature of black hole due to the competition between the environment and Hawking radiation.The reason is that absorbing energy from the environment is dominant when putting the black holes in the thermal environment at higher temperature.For Schwarzschild-AdS black hole,it will evaporate completely and the Hawking radiation is purified when the environment temperature is lower than the critical temperature.On the contrary,when the environment temperature is higher than the critical temperature,it will reach an equilibrium state with the environment and the radiation is maximally entangled with the black hole.It is concluded that the final state of the black hole is determined by the environmental temperature and the temporal evolution of the von Neumann entropy of radiation and the speed of the information purification process characterized by the page curve is also influenced by the thermal environment.
This letter is arranged as follows.We will discuss the evaporation process and the time evolution curves of the entanglement entropies of Hawking radiation of Schwarzschild black hole,Schwarzschild-Anti-de Sitter black hole,and Reissner-Nordström black hole with and without environment in the following three sections respectively.The conclusions and discussions are presented in the last section.
2.Schwarzschild black hole
We start with the thermodynamics of Schwarzschild black hole in flat space.Schwarzschild black hole is determined by only one parameter,black hole mass M.The temperature and the entropy of a Schwarzschild black hole are given respectively by
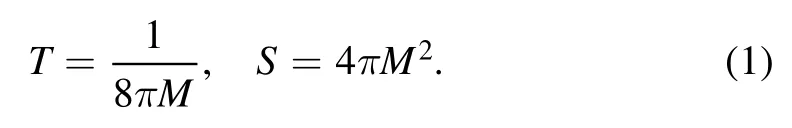
Firstly,we consider the evaporation process of a Schwarzschild black hole without the environment.Assuming the dynamical process obeys the Stefan−Boltzman law,the time evolution of the mass of the black hole is then determined by the differential equation[17]

where σ is the so-called Stefan−Boltzman radiation constant and A=16πM2is the horizon area of black hole.In general,the Stefan−Boltzman constant depends on the type of the emitted particles.Because we are studying the qualitative features of the evaporation process,we set the Stefan−Boltzman constant σ=1 without loss of generality.
The differential equation(2)can be solved analytically.The analytical solution is given by
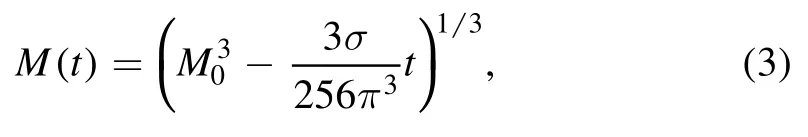
where M0is the initial mass of black hole.The mass of the black hole decays with respect to time is shown in the first panel of figure 1.In this paper,we use Planck unit where c=G=ħ=kB=1.For the astronomical black holes,these constants should be restored in the equations.For example,for the black hole with one solar mass,the unit mass in the figures of this study represents one solar mass,while the unit time in the figures of this study represents 7.6×1063years,and the unit entropy in the figures of this study represents 1077kB.
The coarse-grained entropy of a decaying black hole,which is the thermodynamic entropy of black hole horizon,can be given by using the semiclassical approximation for the black hole evaporation as
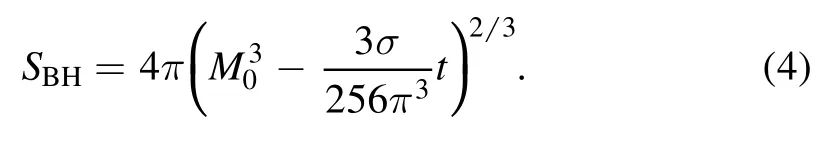
In[9],Page proposed the increasing rate of the coarsegrained entropy of Hawking radiation is proportional to the decreasing rate of the coarse-grained Bekenstein−Hawking entropy of black hole when ignoring the entanglement between Hawking radiation and black hole.In the semiclassical approximation,the coarse-grained entropy of Hawking radiation is then given by
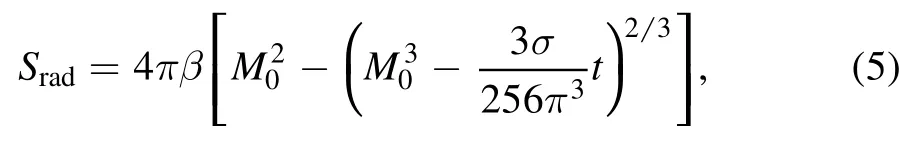
where β is the ratio of the coarse-grained entropies of Hawking radiation and black hole.
As discussed in the introduction,Page time t*is defined as the moment when the entanglement entropy of Hawking radiation is equal to the Bekenstein−Hawking entropy of the black hole.With the assumptions that the black hole starts in a pure state and the Hawking evaporation is a unitary process,Page[8,9]suggest that the von Neumann entropy(i.e.the entanglement entropy)of the Hawking radiation SvN(t)as a function of time t is the semiclassical radiation entropy Srad(t)for t
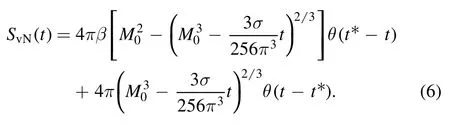
According to this proposal,one can obtain the von Neumann entropy of the Hawking radiation as a function of time as shown in the second panel of figure 1.Notice that at the beginning the entanglement entropy firstly arises up to the Page time t*and then decays to zero.Thus the Hawking radiation is purified when the black hole evaporates completely.
It should be noted that,although the entanglement entropy of the Hawking radiation(as a subsystem)is not identical to zero during the evaporation process,the entanglement entropy of the total system(black hole plus radiation)is always equal to zero under the assumptions that the initial black hole is in pure state and the evolution is quantum mechanical,with unitarity leading to the conservation of the information of the total entanglement entropy.
Now,let us consider the effect of environment on the evaporation process and time evolution of von Neumann entropy of radiation.As discussed in the introduction,the black holes are surrounded by the complex environment.For simplicity,we treat the environment as a thermal reservoir at the constant temperature Te.For example,a radiation can mimic cosmological microwave background.Considering the primordial black hole in such a background may become relevant.Then the mass evolution of equation(2)is modified to be
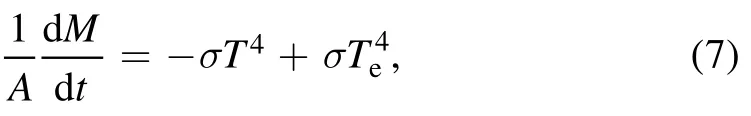
where the second term represents the energy flux from the environment.When the environmental temperature is less than the initial temperature of the Schwarzschild black hole,i.e.the semi-analytical solution of equation(7)can be expressed as

with
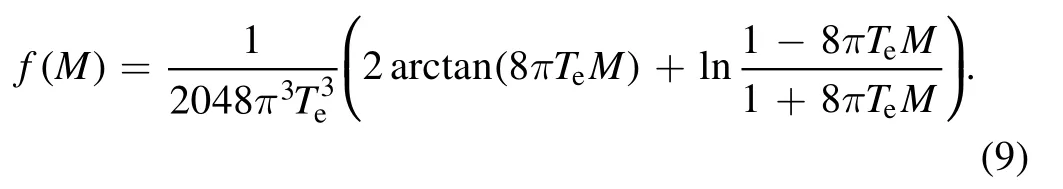

Figure 2.Mass of Schwarzschild black hole and von Neumann entropy of Hawking radiation as a function of time t with different environmental temperatures.The environmental temperatures are set to 0,0.03,and 0.034 from the left to the right,respectively.
Now,we discuss the numerical solution of equation(7).If the temperature of the thermal environment Teis higher than the initial temperature of the Schwarzschild black hole,the process of the black hole absorbing energy from the thermal environment is dominant.The mass of the black hole will increase and the evaporation process will not occur.On the contrary,when the environment temperature is lower than the initial temperature of the black hole,the black hole evaporates completely in a finite time.This is mainly caused by the thermodynamic instability of the Schwarzschild black hole where the heat capacity of the Schwarzschild black hole is negative.
The mass as a function of time t of the Schwarzschild black hole with the environment at the temperature that is lower than the temperature of the initial black hole are shown on the first panel of figure 2.Comparing with the time evolution of black hole mass without the environment(the case that Teis zero),one can conclude that the lifetime of the Schwarzschild black hole with the thermal environment is clearly longer than that of the black hole without environment.This is due to the contribution of the absorbing energy flux from the environment to the black hole.
With the mass function of Schwarzschild black hole under the thermal environment at hand,we can further calculate the time dependence of the von Neumann entropy of Hawking radiation by using the general strategy proposed by Page(i.e.the expression of von Neumann entropy in equation(6)).On the second panel of figure 2,we show the time dependence of von Neumann entropy of the Hawking radiation from the Schwarzschild black holes with the environments at different temperatures.The behaviors are qualitatively similar to that of the Schwarzschild black hole without the thermal environment.However we can see the quantitative difference here.For higher environment temperature,the black hole has slower Page time and it takes longer time to purify the Hawking radiation.
3.Schwarzschild-AdS black hole
In this section,we will consider the Hawking radiation from Schwarzschild black hole in AdS space and its time dependence of van Neumann entropy.The black holes in AdS space is of particular interest due to the recent developing of AdS/CFT correspondence[18].When imposing the absorbing boundary conditions at infinity in AdS,Hawking evaporation of Schwarzschild-AdS black hole in general dimensions was studied by Page in[19],where a finite upper bound for the lifetime of Schwarzschild-AdS black hole was found.This work has been generalized to study the evolution of AdS black hole in conformal gravity and Lovelock gravity[20,21].We now follow the line of Page to investigate the Hawking evaporation of Schwarzschild-AdS black hole in four dimensions with or without the thermal environment.
For four dimensional Schwarzschild-AdS black hole,the temperature,the mass,and the thermodynamic entropy are given respectively by[22]
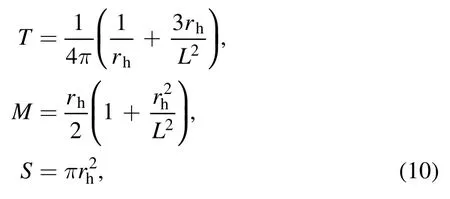
where rhis the horizon radius of Schwarzschild-AdS black hole and L is the AdS length scale.
In the geometric optics approximation,according to Stefan−Boltzmann law,one can write the Hawking emission power as[19–21]
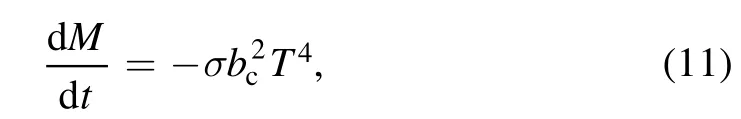
where the critical impact parameter is given by
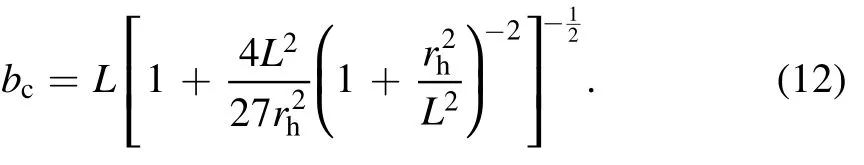
Combining with the thermodynamics of the Schwarzschild-AdS black hole,one can obtain the evolution equation of the horizon due to Hawking emission as

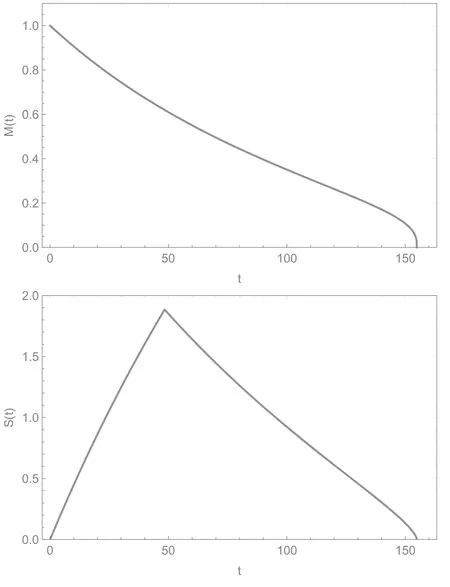
Figure 3.The first panel shows time dependence of mass of Schwarzschild-AdS black hole without environment.The second panel shows von Neumann entropy of radiation as a function of time.The initial black hole radius r(0)and the AdS length scale L are set to 1.
with the dimensionless variable x defined as
In figure 3,the first panel shows the evolution of the black hole mass.It should be noted that the black hole evaporates completely and the evaporating time is finite.The second panel shows the evolution of von Neumann entropy of Hawking radiation.Because the black hole evaporates completely eventually,one can conclude the Hawking radiation is purified to a pure state in a finite time.
We also consider the case where Schwarzschild-AdS black hole is surrounded by the thermal environment.As discussed in the last section,the environment is considered as a thermal reservoir such as the radiation field mimicking the cosmic background radiation.In this case,taking the effect of the thermal environment into consideration,the time evolution of the black hole is determined by the Hawking emission power of Schwarzschild-AdS black hole and the radiation power from the environment at temperature Te

The evolution equation of horizon of Schwarzschild-AdS black hole with the environment is then given by
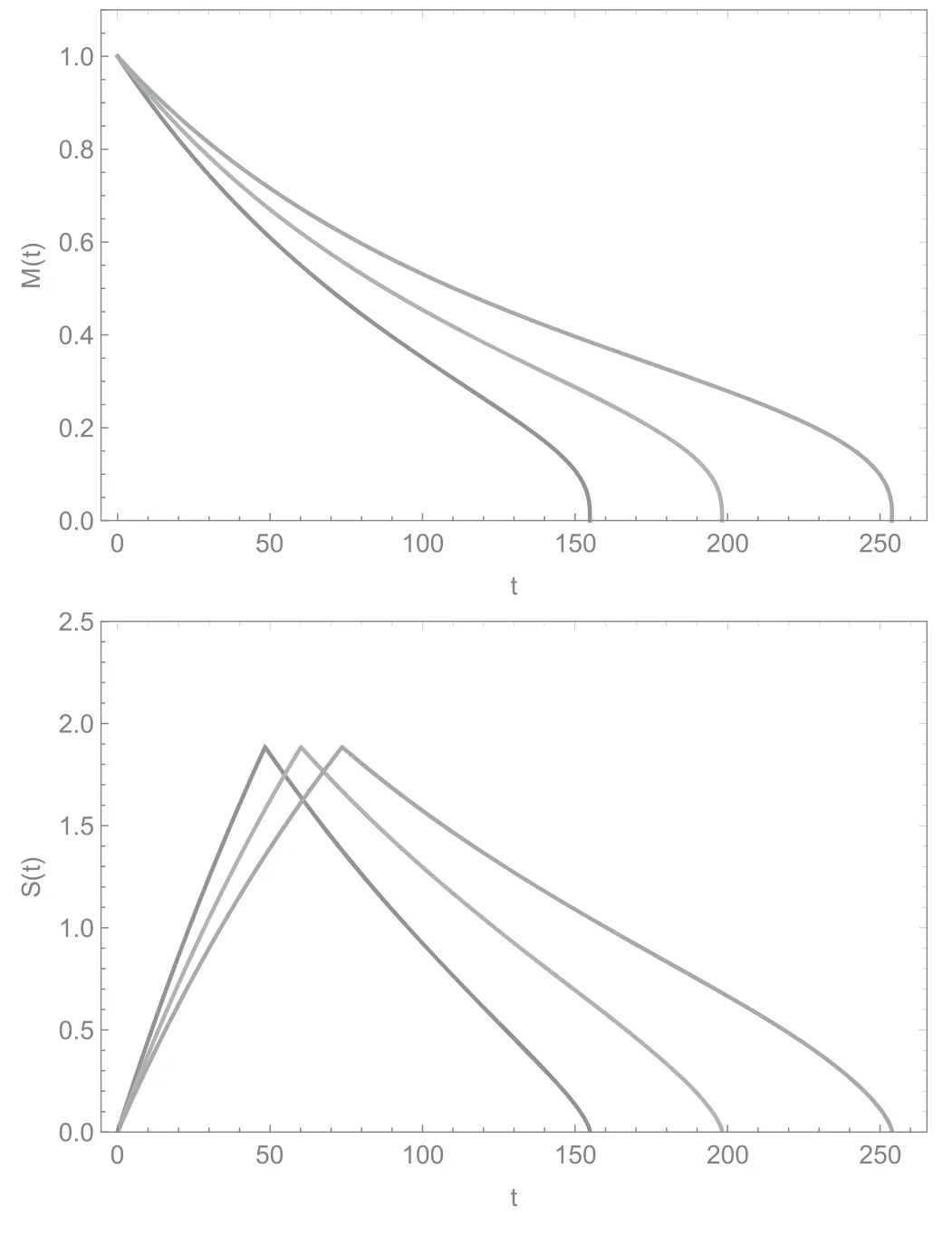
Figure 4.The first panel shows time dependence of mass of Schwarzschild-AdS black hole when the environment temperature is lower than the critical temperature.The second panel shows von Neumann entropy of radiation with environment as a function of time.From the left to the right,the environmental temperatures are set to 0,0.2,and 0.23,respectively.
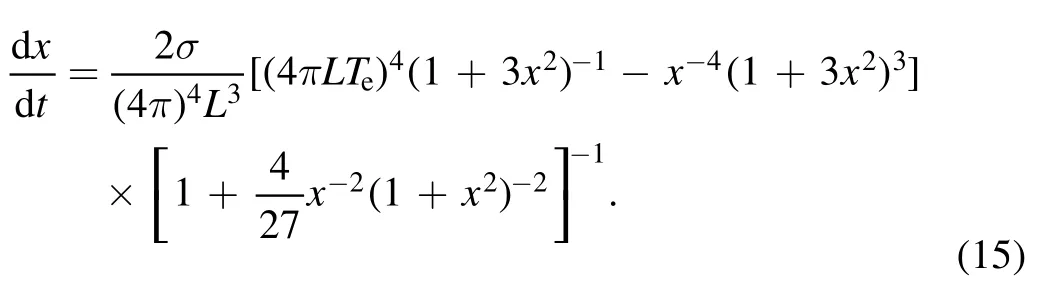
When the temperature of the environment is low enough,Schwarzschild-AdS black hole evaporates completely.The time dependence of the black hole mass and von Neumann entropy of the radiation are shown in figure 4.The conclusion is qualitatively similar to that of Schwarzschild-AdS black hole without the environment.It is also shown that the evaporation time or the lifetime of the black hole with the environment is longer than that of the Schwarzschild-AdS black hole without the environment.Von Neumann entropy of Hawking radiation goes to zero but slower and the Hawking radiation of Schwarzschild-AdS black hole with the environment is purified in a finite time.
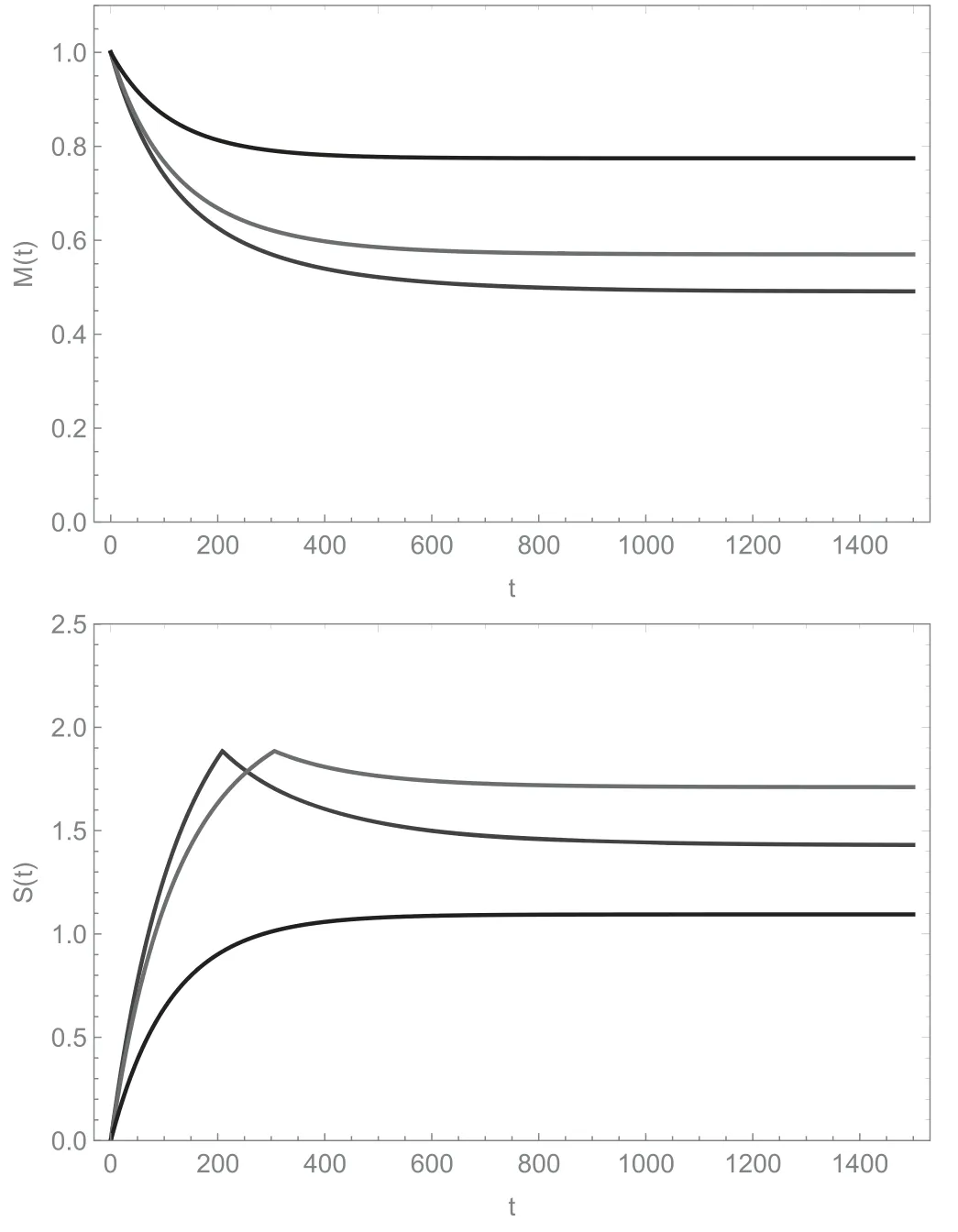
Figure 5.Mass of Black hole and von Neumann entropy of radiation for AdS black hole with environment that the temperature is higher than the critical temperature.The blue,red,and black curves correspond to the environmental temperatures Te=0.279,0.284,and 0.3,respectively.
When the environmental temperature is high enough,the Schwarzschild-AdS black hole and the environment will reach an equilibrium state eventually.In this case,the final state of the black hole is eternal.The time evolution of the mass of the black hole and von Neumann entropy of Hawking radiation are shown in figure 5.Two different types of the curves are shown when the environmental temperature takes different values.When the environmental temperatures are set to 0.279 and 0.284,the von Neumann entropy of the Hawking radiation increases at the first stage,and then decreases to a constant value finally.When the environmental temperature takes the value of 0.3,the von Neumann entropy of the radiation increases monotonically from zero to a constant value.In either case,the constant values of the von Neumann entropy of the radiation equal to the Bekenstein−Hawking entropies of the final black holes.This is consistent with the finiteness of the von Neumann entropy for an eternal black hole.
Therefore,we can conclude that the final state of Schwarzschild-AdS black hole is determined by the temperature of the thermal environment and the page curve of the Hawking radiation is significantly influenced by the thermal environment.There is a critical temperature of the thermal environmentwhich is just the minimum temperature of the Schwarzschild-AdS black hole.When the environmental temperature is above this critical temperature,the final state of the black hole is in an equilibrium state with the thermal radiation.In this case,the radiation is maximally entangled with the final Schwarzschild black hole.This behavior is qualitatively similar to the page curve of eternal Schwarzschild black hole[23],where the entanglement entropy grows linearly in time before the Page time,and remains a constant value after the Page time.When the environmental temperature is below the critical temperature,the black hole evaporates completely in finite time.The Hawking radiation is purified at last although at slower rate due to the presence of the environment and its associated radiation and therefore the information is conserved.
4.Reissner-nordström black hole
In this section,we discuss the Hawking evaporation and the page curve of Reissner-Nordström black hole without and with the thermal environment.Because the Reissner-Nordström black hole is charged,the charge loss should also be considered besides the energy loss[24–26].
For Reissner-Nordström Black hole,there are two horizons,the event horizon r+and the Cauchy horizon r−,which are given by
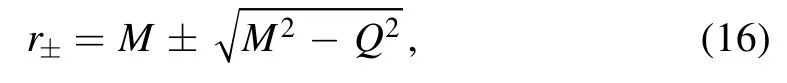
with black hole mass M and charge Q.The entropy and the temperature of Reissner-Nordström Black hole are given by
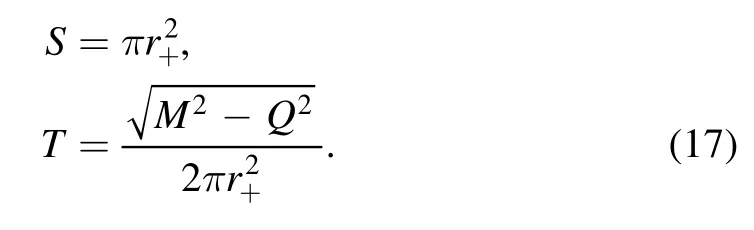
For the charged black hole,we should also consider the Schwinger effect,where electron-positron pairs are spontaneously produced in the presence of an electric field as predicted in quantum electrodynamics[27].The electronpositron pairs are spontaneously created in the presence of an electric field.The process of positron captured by the black hole leads to the charge loss as well as the mass increasing of black hole.The charge loss for the black hole with mass M and charge Q can be approximated by the Schwinger formula[24]
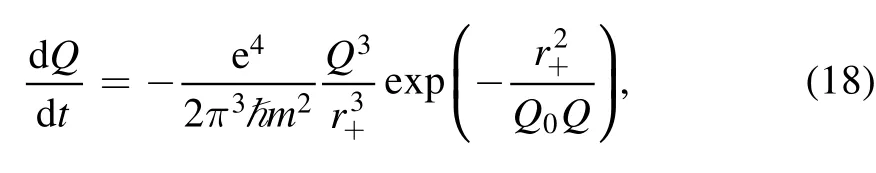
where Q0is the inverse of the Schwinger critical field Ec:=m2c3/eħ3=1.312×1016V/cm.Here m and e denote the mass and the charge of the positron,respectively.It should be noted that the Hiscock−Weems model works for sufficiently large black holes M.
In[24],Hiscock and Weems also proposed that mass loss is due to the emission of particles following the Stefan−Boltzmann law as well as the emission of electron/positron via dQ/dt term,which enters via the first law of black hole thermodynamics
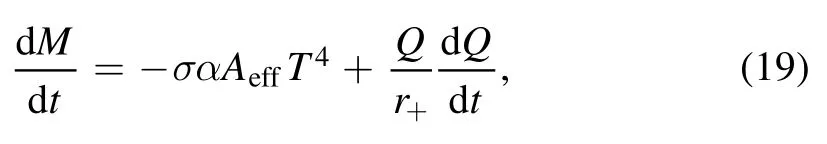
where σ is the radiation constant,α is greybody factor,and Aeffis the effective emission area.The effective emission area is given by[24]
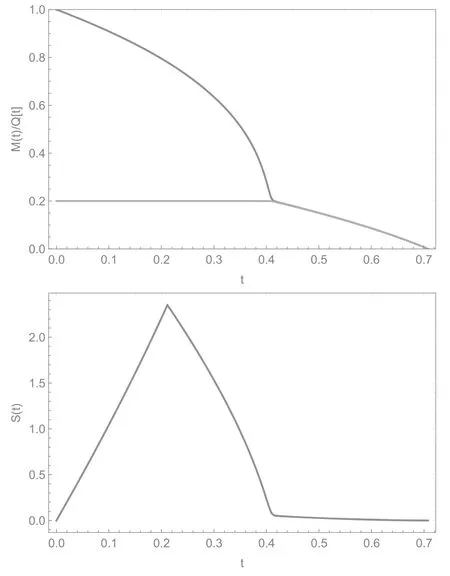
Figure 6.The first panel shows the mass(blue line)and the charge(brown line)of Reissner-Nordström black hole without environment as a function of time t and the second panel shows the time evolution of von Neumann entropy of radiation.In these plots,we have set all the constant to 1 for simplicity.
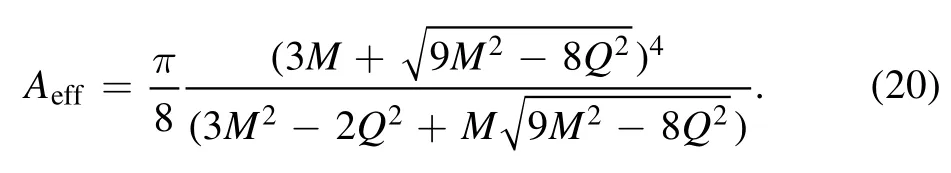
By solving the coupled ordinary differential equations,one can obtain the evolution of the black hole mass,the black hole charge,as well as the entropy numerically.In figure 6,we have plotted the evolution of the mass and the von Neumann entropy of Reissner-Nordström black hole without the thermal environment.The general behavior of the time dependence of von Neumann entropy is similar with that of Schwarzschild black hole.However,there is a long tail at the late time of evolution,which is caused by the loss of charge of Reissner-Nordström back hole.At the early time of evolution,one can see that the charge loss is slower than the mass loss for the charged black hole.In the late time of evolution,in order to preserve the cosmic censorship,the mass loss rate is largely suppressed by the charge loss.However,the Reissner-Nordström black hole eventually evaporates completely,and the Hawking radiation is purified as shown in the page curve of the black hole.
Now,we consider the effect of the thermal environment on the evaporation process and the page curve of Reissner-Nordström black hole.Considering the radiation of the thermal environment at temperature Te,the mass loss formula should be modified as
Figure 7.The first panel shows the mass and the charge of black hole with the environment at different temperatures.The second panel shows the time evolutions of von Neumann entropy of radiation correspondingly.The environmental temperatures take the values of 0,0.17,and 0.2 from the left to the right,respectively.
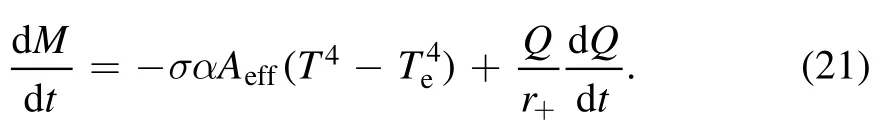
Combining equation(21)and equation(18),we can obtain the numerical solution of the mass and the charge of Reissner-Nordström black hole with the thermal environment.Then the von Neumann entropy of the radiation can also be obtained by using the same strategy as shown in equation(6).
The evolutions of the mass and the von Neumann entropy of the radiation of the Reissner-Nordström black hole surrounded by the thermal environment are plotted in figure 7.The thermal environment appears to delay the onset of the long tail at the late time of the evolution.If the environmental temperature increases,the lifetime of the Reissner-Nordström black hole will increase correspondingly.If the environmental temperature is too high,the mass of the black hole will increase instead and there is no Hawking evaporation.These behaviors are qualitatively similar to the cases of Schwarzschild black hole in flat space and in AdS space.
From the second panel of figure 7,It can be seen that the von Neumann entropy of the radiation will go to zero when the Reissner-Nordström black hole evaporates completely.This indicates the Hawking radiation is also purified finally although the purification process is slowed down by the presence of the environment and its associated radiation.
At last,let us make a simple discussion on the Reissner-Nordström AdS black hole.In principle,one can study the Hawking evaporation of the Reissner-Nordström AdS black hole by imposing the absorbing boundary condition as done for the Schwarzschild AdS black hole.Correspondingly,the von Neumann entropy of the radiation can be routinely calculated.Beside the electric charge’s influence on the evaporation process,we think the main conclusions are similar to the case of Schwarzschild AdS black hole.
5.Conclusion
In summary,we have studied the effect of the thermal environment on the evaporation process of various types of black holes and the corresponding time evolutions of the von Neumann entropies of Hawking radiations in detail.In thermodynamics and statistical physics,environment is conventionally treated as thermal reservoir.For astronomical black hole,it is surrounded by the accretion disk and radiation fields.The environment is too complex to describe.Although model here is an ideal toy one,we believe it captures the main influence of the environment on the evaporation process.We investigated the evaporation processes of Schwarzschild black hole,Schwarzschild-AdS black hole,and Reissner-Nordström black hole respectively.When taking the environment effect into account,the final state of the evaporating black hole is shown to be determined by the temperature of the thermal environment.It is also concluded that the page curve of Hawking radiation is significantly influenced by the thermal environment.
For black holes in flat space,for example,Schwarzschild black hole and Reissner-Nordström black hole,the black holes will not evaporate if putting in the environment at higher temperature than that of the initial black hole.Therefore,there is no purification and no information paradox.If the environmental temperature is lower than the temperature of the initial black hole,the black hole will evaporate completely.The higher temperature of the environment is,the longer lifetime of the black hole has.In this case,the page curves show the Hawking radiation is purified eventually although with slower speed due to the presence of the environment and its associated radiation.For Schwarzschild-AdS black hole,it will evaporate completely and the Hawking radiation is purified when the environment temperature is lower than the critical temperature.Higher temperature under this critical temperature will slow down the evaporation and the information purification process.When the environment temperature is higher than the critical temperature,it will reach an equilibrium state with the environment and the radiation is maximally entangled with the final black hole.
At last,let us make some comments on our results.For the realistic black holes in universe,the temperature of CMB is high enough compared to the temperature of a black hole with solar mass.The influence of CMB can be significant both in the early time of universe and in the present time.A black hole will evaporate completely only when its temperature is higher than the temperature of the thermal bath.Otherwise,it will attain an equilibrium state with the thermal bath as discussed in our paper.In this case,the corresponding page curve is very different from the black hole without environment.Furthermore,in the early universe,thermal baths in terms of certain matter fields can be significant.For examples,even at the electro weak scale,the temperature is already quite high.In the process of reheating during the last stage of the inflation,the temperature can also reach high values.Therefore,realistically speaking,the thermal baths can be quite significant in the early universe.
杂志排行
Communications in Theoretical Physics的其它文章
- Influence of relative phase on the nonsequential double ionization process of CO2 molecules by counter-rotating two-color circularly polarized laser fields
- Released energy formula for proton radioactivity based on the liquid-drop model*
- Deformation parameter changes in fission mass yields within the systematic statistical scission-point model
- The upper bound on the tensor-to-scalar ratio consistent with quantum gravity
- Influences of flexible defect on the interplay of supercoiling and knotting of circular DNA*
- The relation between the radii and the densities of magnetic skyrmions
