Application of a New Catalyst Deactivation Model for Residue Hydrotreating

Zhang Kui; Nie Hong; Dai Lishun

(Research Institute of Petroleum Processing, SINOPEC, Beijing 100083)

Abstract: This article reports a new catalyst deactivation model for residue hydrotreating technology (RHT) with three adjustable parameters, named as “active-region-migration model”. The active-region-migration model is proposed to describe the catalyst deactivation of RHT where the catalysts are deactivated due to metal loading. Along with the lumped reaction kinetics, the deactivation model can be applied to simulate the hydrogenation reaction performance in RHT.Industrial data from a commercial RHT unit show reasonably good agreements with the model calculations. Essentially, the active-region-migration model can separately simulate the catalytic-activity- change of each hydrogenation reaction during the whole run of RHT, with a single curve.

Key words: residue; hydrotreating; hydrodesulfurization; kinetic model; deactivation model

1 Introduction

Residue is the heaviest distillate of crude oil, and most of impurities (such as metal, sulfur and nitrogen) in crude oil are enriched in residue[1]. Through direct combustion of residue, the emission of SOxand NOxinevitably leads to air pollution, and the generated metal particles also do great harm to many living things[2].As an important technology for upgrading residue, the residue hydrotreating technology (RHT) can remove these impurities by hydrogenation reactions[3-4]. The reactions mainly include hydrodesulfurization (HDS),hydrodenitrogenation (HDN), carbon-residue reduction(HDCCR), and hydrodemetallization (HDM)[5-6]. In addition, HDM reactions include hydrodenickelation(HDNi) and hydrodevanadization (HDV). Then, RHT is combined with FCC (Fluid catalytic cracking)technology to convert residue into more valuable and environmentally-benign products[7].

However, the high contents of impurities such as metals(Ni and V) and asphaltenes can cause adverse effects on catalysts, because metals together with the coke, which is converted from asphaltenes, are deposited on catalysts during RHT[8-9]. Generally, coke can form very rapidly during the first hours of the whole run, and meanwhile the deposition of metals takes place at a longer period[10-11].For RHT, the catalyst deactivation phenomenon is divided into three stages: early deactivation due to quick coke deposition at start-of-run (SOR), middle-stage deactivation due to metal-sulfide deposits at middleof-run (MOR), and total loss of activity due to severe diffusional resistance after almost total pore plugging at end-of-run (EOR)[12-14]. Moreover, the type of catalyst deactivation due to the metals and coke deposition is more dramatic at long time-on-stream (TOS) or high reaction severity[15]. When catalyst activity reduces to a low value, unavoidable unit shut down will be carried out.Currently, fixed-bed reactors are commonly used in RHT[16]. To better estimate the performance and life of catalysts in fixed-bed reactors, researches on the model of catalyst deactivation for RHT are very necessary. According to the literature[6,17-26], various catalyst deactivation models have been reported. Much work has been done for modeling the deactivation at SOR and/or MOR, but very few reports deal with modeling the catalyst deactivation in three-stages(SOR, MOR, and EOR) together. Moreover, some models are too complex to be employed for application in RHT.So using one simple model of catalyst deactivation for modeling the three-stages together is essential for RHT.

In present work, a mechanism model, “active-regionmigration model”, for catalyst deactivation is proposed and will be described later in the article. This model can simulate catalyst deactivation with three-stages together,and has three adjustable parameters (h,bandc) for helping easily apply the model in RHT. Here the kinetic models for HDS, HDN, HDCCR, HDM, HDNi, and HDV reactions were used. Based on the kinetic models, a whole run data of a commercial RHT unit were employed in analysis of catalyst activity. Active-region-migration model can be applied to simulate the catalytic-activity change in the course of hydrogenation reactions, HDS,HDN, HDCCR, HDM, HDNi, and HDV reactions.

2 Active-Region-Migration Model

2.1 Assumptions

Continuous coking and metal deposition on the catalyst can lead to the deactivation of RHT catalyst[8,14], not only lowering the activity of catalyst particles, but also reducing the diffusion coefficient of reaction molecules[27-28]. Hereby, the deactivation performance of catalyst particle is described as follows. The concentration of reactant molecules appearing in the catalyst particle declines as the coke and metal deposition rises, and the formation and expansion of “dead zone” also occur in the catalyst particles at the same time. In the “dead zone”, metal active sites cannot be effectively utilized and the concentration of reactant molecules varies very little. Thus, the catalyst activity declines coupled with continuous coke and metal deposition.

During the operation process of industrial RHT unit, since the deposited metal on catalyst is the product of HDM reaction, the change of metal deposition on catalyst can be monitored by calculating the difference of metal content in the residue and the hydrotreated residue. However,it is difficult to analyze the change of coke content on RHT catalyst in real time. Therefore, the double adverse effects of carbon deposition and metal deposition on catalyst activity can be simplified to the only role of metal deposition when constructing the catalyst deactivation model.

Herein, a mechanism model of catalyst deactivation is constructed and further applied for RHT process. The model, “active-region-migration model”, is established on metal deposition. The following constitutes the hypotheses for the active-region-migration model:

1) The catalyst particles are cylindrical with uniform distribution of active centers, and no mass-transfer restriction exists in the axial direction of the particles.

2) The particle size of the deposited metal is much smaller than that of the catalyst. Gradual metal deposition leads to the formation and expansion of “dead zone” in the catalyst particles, and no reaction occurs in “dead zone”.Meanwhile, the volume of “dead zone” is proportional to the amount of metal deposited on the catalyst.

3) The metal deposition also can change the diffusion coefficient of reaction molecules. The reciprocal of the effective diffusivity is the arc tangent function of the amount of metal deposited on catalyst.

4) To simplify the model, it is assumed that the “dead zone” expands continuously from the center to the outer surface along the radial direction of catalyst particle, and the active zone shrinks continuously from the center to the outer surface along the radial direction at the same time.

5) In the absence of metal deposition, the particle possesses the largest active zone and zero “dead zone”, so the catalytic activity of catalyst particle is the highest at this time.

6) First-order kinetics are used for the reactions, including HDS, HDN, HDCCR, and HDM (such as HDNi and HDV) reactions.

2.2 Model equations

Figure 1 shows the active-region-migration model as described by the above assumptions. At the axial direction, since there is no mass-transfer restriction, the physical and chemical properties of catalyst are uniform and the reactant concentrations are also the same.Only the radial direction is considered here, and the concentration-distribution equations of reactant molecules in the particle active zone are shown below.

Figure 1 Active-region-migration model

According to the hypotheses for the active-regionmigration model, the volume of “dead zone” in the catalyst particle is proportional to the metal deposition.When the amount of deposited metal reaches the upper limit, the volume of “dead zone” is the maximum. So

Generally, metal depositing on the catalyst can decrease the channel size of catalyst pore as well as reduce the diffusion coefficient of reaction molecules[29]. Here,the parametersbandcare employed as the influence factors of diffusion coefficient. The parameterbisused to characterize the adverse impact of metal deposition on molecule diffusion at SOR, andcisused to characterize the adverse impact for the whole run (SOR, MOR, and EOR). The relationship between diffusion coefficient and metal deposition can be determined on the basis of the hypotheses for the active-region-migration model. The relationship is shown in the following equation.

When there is no metal deposition, the initial quantity of reactant molecules in the catalyst particle is the largest. So the activity of catalyst particle also is the highest at this time. As the hydrogenation reactions occur, the generated metal sulfides begin to deposit on the pore channel of catalyst particle. As the metal deposition increases, the“dead zone” continuously enlarges, while the quantity of reactant molecules in the catalyst particle decreases.Meanwhile, the catalytic activity of particle declines.Hereby, the ratio of the quantity of reactant molecules in catalyst particle to the initial quantity is defined as the activity factor “a” of catalyst particle. The calculation equation for activity factor “a” is presented below.

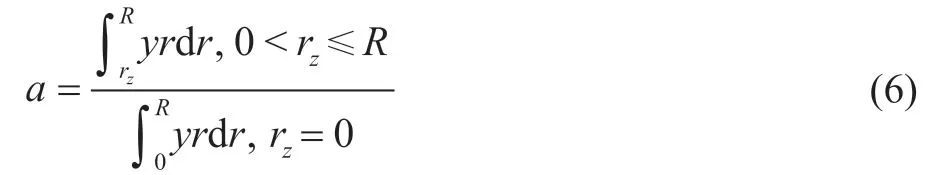
In order to simplify the calculation and further apply the model in RHT, the dimensionless processing of the above equations is very necessary. Firstly, a new parameterhis defined. This parameterhis used to characterize the compounded effect of catalyst activity and molecule diffusion. The following equation is employed to describe this relationship.

When the dimensionless radius variables are determined,

The concentration-distribution equation of reactant molecules in the active zone can be simplified as follows.

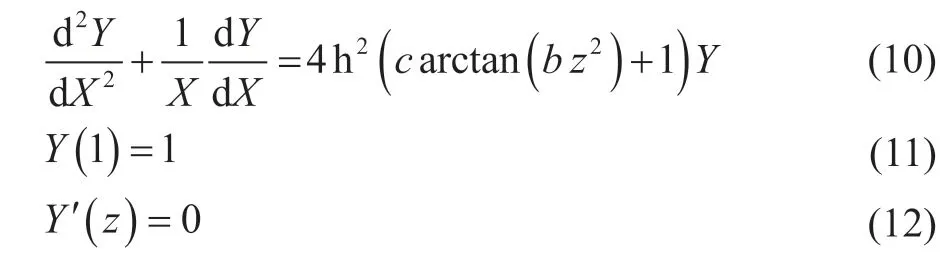
Meanwhile, the equation of activity factor “a” is simplified as follows.

Based on the analysis of the above model equations, it is found that there are three parameters (h,b,andc) existing in the equation of particle activity factor “a”. Thus, the active-region-migration model is a deactivation model with three adjustable parameters, viz.:h,b,andc. During RHT process, the hydrogenation reactions of HDS,HDN, HDCCR, and HDM (including HDNi and HDV)display the different reaction characteristics, and thus their deactivation performance varies differently with the metal deposition. By adjusting the three parameters (h,b,andc), the equation of activity factor “a” can simulate such changes and fit the deactivation curves with different shapes. Figure 2 shows typical shapes of activity as represented by the activity equation.

Figure 2 Typical shapes of activity curves using the active-region-migration model

The general deactivation performance of RHT catalyst is as follows. The catalyst activity displays a sharp initial decrease followed by a long, slow deactivation. Finally,a sudden death occurs when the metal loading in a pellet reaches its maximum. As shown in Figure 2, the curve with highhvalue conforms to the general deactivation behavior of RHT catalyst. Meanwhile, the higher thehvalue is, the greater the effect of metal on activity would be. When the diffusion coefficient of molecules declines quickly with metal loading, the catalyst activity decreases rapidly. The curve with highbmeans the diffusion coefficient decreases rapidly at SOR. The curve with highcmeans the diffusion coefficient is greatly affected by the metal deposition for the whole run. Therefore, the activity curves generated by using the active-region-migration model can better simulate the change of catalyst activity with metal loading.

2.3 Comparison with pore-filling model

Khang and Mosby[17]established a deactivation mechanism model, pore-filling model, with two adjustable parameters (hyandhz) in its model equation. By adjusting the two parameters (hyandhz), the pore-filling model can be used to simulate the change of catalyst activity with metal deposition.

Here, a set of hypothetical activity points which vary with metal deposition are taken as an example, and two models, the pore-filling model and the active-zonemigration model, are used to simulate them respectively.Figure 3 shows the comparison of simulated deactivation curves between the pore-filling model and the activezone-migration model. As shown in Figure 3, the catalyst activity decreases with the increase of metal deposition,which is in line with the deactivation performance of general RHT catalyst. Whenhyandhzare 1.29 and 15.53,respectively, the goodness of fit (R2) of the simulation curve obtained by the pore-filling model is a maximum,and the value is 0.6257. Whenh,b,andcare 6.33, 7.33,and 0.79, respectively, the goodness of fit (R2) of the simulation curve obtained by the active-zone-migration model is a maximum with the value equating to 0.9373.It can be concluded that compared with the pore-filling model, the simulation curve of active-zone-migration model has better fitting effect on activity points, which vary with metal deposition.

Figure 3 Comparison of simulated deactivation curves between the pore-filling model and the active-zone-migration model

3 Kinetic Model for RHT

Due to the composition complexity for reactants and products, it is very difficult to establish the kinetic models of RHT at the molecular level. For example, the metal,sulfur, and nitrogen content in residue can be measured,but their exact chemical compositions are difficult to determine. During the establishment of reaction kinetics,the lumping methods are usually employed[23,30-32]. Feed and product compositions can be lumped, and therefore the reaction kinetics can be constructed on the basis of those measurable lumped quantities.

During the RHT process, many hydrogenation reactions occur. For example, S- and N- compounds can be hydrogenated by H2to H2S and NH3, separately. The CCR can be converted to high H/C ratio hydrocarbons(H-CCR) by HDCCR reaction. The metal-compound (RM), such as Ni-compound (R-Ni) and V-compound (RV), can react with H2and the H2S generated during HDS reaction to form metal sulfide (M-S) including Ni sulfide(Ni-S) and V sulfide (V-S). Here, the symbol R refers to hydrocarbon molecule. The simplified reaction equations of main reactions involved in RHT process are listed as follows.

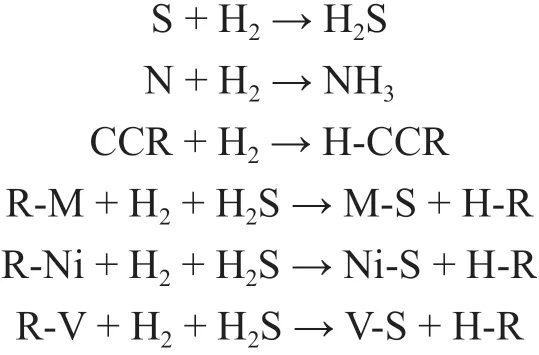
Traditionally, simple first-order or non-first-order kinetics have been used for RHT[5]. The differential equations of HDS, HDN, HDCCR, HDM, HDNi, and HDV reactions with the same form are established and listed as follows.

To integrate the differential equations, the integration equations are listed as follows.

The activity factors of HDS, HDN, HDCCR, HDM,HDNi, and HDV reactions can be defined in the same form, and the calculation equation of activity factor “a” is as follows.

Due to the different reactivity for HDS, HDN, HDCCR,HDM, HDNi, and HDV reactions on the RHT catalysts,generally the performance of their activity factors “a” also differs with the metal loading. Here, the active-regionmigration model is employed to simulate the changes of activity factor “a” for hydrogenation reactions, and they will be presented in the following sections.

4 Comparison with Industrial Data

On the basis of a whole run data for a commercial RHT unit, the active-region-migration model can be used to simulate the performance of catalyst deactivation for RHT reactions. During the whole run of the commercial RHT unit, the feed properties are basically the same, but the catalyst activity decreases with the unit run duration. For keeping the required properties of hydrotreated oil, the operation temperature needs to be increased continuously for compensating the catalyst activity loss.

The following introduces the way of applying the activeregion-migration model to simulate the performance of catalyst deactivation. Firstly, the above kinetic models were employed to calculate the catalyst activity of hydrogenation reactions, including HDS, HDN, HDCCR,and HDM (including HDNi and HDV) reactions.Then the active-region-migration model was applied to simulate the activity changes of the hydrogenation reactions. The changes of activity factors with metal loading on the catalyst are shown in Figure 4(a)―4(f),respectively. As shown in Figure 4(a)―4(f), the whole run of activity changes can be simulated effectively by the active-region-migration model, with a single simulated curve. At SOR, the activity factors of HDM, HDNi, and HDV reactions decline rapidly among the hydrogenation reactions, and HDN reaction declines more rapidly than HDS and HDCCR reactions. In the initial stage of catalyst deactivation, the adverse effect of molecule diffusion coefficients on reaction activity is generally significant[33]. For different hydrogenation reactions, the values of parameterbare 20 (HDM, HDNi, HDV), 15(HDN), 5 (HDS) and 7 (HDCCR), respectively. At SOR,the influence of molecule diffusion coefficient on activity factorsamay be different, and the possible sequence is as follows: HDM, HDNi, HDV ＞ HDN ＞ HDS, HDCCR.Meanwhile, the values of parametercare 20.50 (HDS),6.23 (HDNi), 4.86 (HDV), 3.39 (HDCCR), 3.22 (HDM)and 1.56 (HDN), respectively. Thus, during the whole run,the influence of molecule diffusion coefficient on activity factors “a” also is different, and the possible sequence is as follows: HDS ＞ HDNi, HDV, HDCCR, HDM ＞ HDN.Figures 5(a)—10(a) show the comparison between the measured content and the calculated content in the hydrotreated residue. Figures 5(b)—10(b) show the comparison of measured conversion with the calculated one.As shown in Figures 5(a)—10(a), the measured contents of S, N, CCR, M, Ni, and V fit well with the calculated ones.As shown in Figure 5(b)—10(b), the value of calculated conversions are scattered around the expected value (red line) and most of the points are within the deviation lines of ±5%. Figure 11 shows the measured and calculated value of average metal loadings on the catalyst of RHT. As shown in Figure 11, the metal-loading calculation is quite satisfactory to reach around 100% of metal loading. Thus,the active-region-migration model can simulate the catalyst deactivation for the whole run of RHT unit.

Figure 4 Activity factor a vs. metal loading a) HDS; b) HDN; c) HDCCR; d) HDM; e) HDNi; f) HDV.

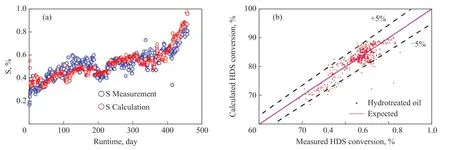
Figure 5 (a) Sulfur content, measured vs. calculated; (b) HDS conversion, measured vs. calculated.

Figure 6 (a) Nitrogen content, measured vs. calculated; (b) HDN conversion, measured vs. calculated

Figure 7 (a) CCR content, measured vs. calculated; (b) HDCCR conversion, measured vs. calculated

Figure 8 (a) M content, measured vs. calculated; (b) HDM conversion, measured vs. calculated

Figure 10 (a) V content, measured vs. calculated; (b) HDV conversion, measured vs. calculated

Figure 11 Average metal loading on catalyst,measured vs. calculated

Therefore, by coupling RHT reaction kinetic model,the active-region-migration model can simulate the deactivation performance of each hydrogenation reaction,and simultaneously can better simulate the change of lumping components on the whole run of RHT unit.

5 Conclusions

The active-region-migration model is developed to simulate catalyst deactivation for RHT. The deactivation model can be applied along with the lumped reaction kinetics to simulate the hydrogenation reactions in RHT.Industrial data from a commercial RHT unit show a reasonably good agreement with the model calculation values. Essentially, the active-region-migration model can simulate the catalyst deactivation for the whole run of RHT, with a single curve for each hydrogenation reaction.For different hydrogenation reactions, the influence of molecule diffusion coefficient on activity factors also may be different on the whole run, and the possible sequence is as follows: HDS ＞ HDNi, HDV, HDCCR, HDM ＞ HDN.

Nomenclature

a― the activity factor of catalyst particle;

A― the pre-exponential factor;

A0― the initial pre-exponential factor at SOR;

b― the influence factorbof diffusion coefficient at SOR;

c― the influence factorcof diffusion coefficient on the whole run;

C―the S, N, CCR, M, Ni, and V contents in hydrotreated residue, %;

Cin― the S, N, CCR, M, Ni, and V contents in residue,%;

Cout― the S, N, CCR, M, Ni, and V contents in hydrotreated residue, %;

D0― the initial diffusion coefficient of reactant molecule when there is no metal deposition in the catalyst particle;

D― the diffusion coefficient of reactant molecule in the catalyst particle;

E― the activation energy, kJ/mol;

h― the parameter used to characterize the compounded effect of catalyst activity and diffusion coefficient;

hy, hz―the two parameters of pore-filling model;

k0― the reaction rate constant of the reactant molecule in the catalyst particle;

LHSV― liquid hourly space velocity, h-1;

M0― the upper amount of the deposited metal at the semi-diameter of particle,R;

M― the amount of the deposited metal at the semidiameter of “dead zone”;

n― the reaction order;

p― the partial pressure of hydrogen, MPa;

p0― a unit of pressure,p0=1.0MPa;

r― the radial position of the arbitrary active site in the active zone;

rZ― the semi-diameter of “dead zone”;

R'― the Avogadro’s number,R'= 8.314 J/(mol·K);

R― the semi-diameter of the cylindrical catalyst particle;

t― residence time (t= 1/LHSV), h;

T― reaction temperature, K;

X― the dimensionless radial position of the arbitrary active site in the active zone;

y0― the concentration of reactant molecule on the external surface of the catalyst particle;

y― the concentration of reactant molecule in the active zone of the catalyst particle;

Y― the dimensionless concentration of reactant molecule in the active zone of the catalyst particle;

z― the dimensionless semi-diameter of “dead zone”;

α― the reaction index of pressure.

Acknowledgments:This work was financially supported by the SINOPEC Research Program (Grant KL20009).