Characteristics of air-water flow in an emptying tank under different conditions
2021-03-01JilingLingYiyiYiZheng
Jiling Ling , Yiyi M , , Yi Zheng
a College of Civil Engineering and Architecture, Zhejiang University, Hangzhou 310058, China
b Zhejiang Communications Construction Group Co., Ltd, Hangzhou 310053, China
Keywords:Air-water flow Bubble Emptying process Drainage efficiency Orifice
ABSTRACT This paper experimentally studied the features of air-water flow during the emptying of a water-filled prismatic tank with a bottom orifice under different conditions.The experiments were conducted with both circular and elliptical orifices, with and without ventilation.The evolution of bubbles, water pres- sure variation, and water level change with time were recorded in the experiments and analyzed.Based on the results, the evolution of bubbles could be mainly divided into three stages of formation, defor- mation, and decomposition.Ventilation was found important to the emptying process, with which the drainage efficiency was much higher than that under the unventilated condition.Additionally, under the unventilated condition, the drainage efficiency with the circular orifice was slightly higher than that with the elliptical orifice.
When emptying liquid-filled bottles, periodical generation of bubbles at the bottle opening can be observed, accompanied by a “glug-glug”sound, which isoneof thecommonest two-phaseflow phenomena in daily life.Similar phenomena can be found in many industrial applications, such as bottle rinsing [1] and filling [2] .It has been received much attention due to its effect on the liquid discharge rate, which is vital to these industrial processes.For ex- ample, it is one of the dominant factors for bottle washing/rinsing efficiency [1] .
Extensive studies have been conducted on the drainage effi- ciency of emptying bottles, tanks, pipes, or other containers [3–5] .The air-flow patterns during the emptying process can be com- monly divided into three types [6] , including no flow, oscillatory flow and counter flow.The parameters affecting the air-water flow features during the emptying process mainly include the volume, exit size, inclination of the container, and the initial liquid filling ratio, etc.[3–6] .Tang and Kubie [7] studied the emptying processes with the bottle exit orienting in various directions.Whalley [8] , Geiger et al.[9] and Rohilla and Das [10] reported that there was a critical bottle inclination angleθcrit.When the bottle inclination angleθ<θcrit, the emptying time decreased with the increase ofθwhile it turned asymptotic whenθ>θcrit.Note that the effects of the inclination degree of containers on the emptying time varied with the exit size [9] .Mayer [11] and Kordesrani and Kubie [12] re- ported that the emptying time reduced with the increase of the exit diameter.Additionally, Whalley [8] found that a higher water temperature resulted in a shorter emptying duration.Nevertheless, the height of the container and the initial liquid depth inside it had a limited effect on the drainage efficiency of the emptying process [ 4 , 13 ].Kumar et al.[3] controlled the ventilation conditions during the emptying and found that the discharge rate increased with the opening rate of the top air vent.
The drainage efficiency during the emptying processes has been defined quantitatively.Wallis [14] introduced the flooding constant, defined asC= 0.5 98to describe the relationship of gas-phase velocity and liquid-phase velocity when emptying, withρgandρlbeing the densities of gas and liquid, respectively.A largerCindicated a shorter duration of th e emp ty ing process.Whalley [8] proposed anot her constantC=based on Wallis’s work[1 4] , whereandrepresent the superficial veloc- ities of gas and liquid, respectively.
Although there have been extensive studies on emptying pro- cesses of various containers, most of them were conducted with a circular exit and those under the conditions of non-circular ex- its are limited.Non-circular orifices are also used in chemical en- gineering, like the elliptical orifice at the bottom of the hanging down camber [15] .Additionally, the majority of studies were un- der unventilated conditions, while few have explored the effects of ventilation on air-water flow features.This paper experimentally studied the air-water flow during the emptying process of a water- filled prismatic tank with a bottom orifice, focusing on the charac- teristics of air bubbles generated at the orifice and their effects on the drainage efficiency.The experiments were conducted with both circular and elliptical orifices under top-sealed and top-ventilated conditions.The impacts of exit shapes and ventilation conditions on the emptying process were discussed.The findings of the cur- rent study can provide information for optimizing relevant indus- trial processes, e.g., orifice design optimization, ventilation condi- tion control, or any other aspects.
The schematic of the experimental setup is shown in Fig.1 a, which mainly consisted of a Plexiglas prismatic tank of 200 mm ×200 mm ×500 mm, a water pump, and a param- eter measurement system (including a water pressure transducer and a data acquisition system).The experimental setup was a self- circulating system by using a submersible pump.An orifice was opened in the center of the tank bottom for water drainage.Two types of bottom orifices, i.e., circular and elliptical orifices, were tested in the experiments, as shown in Fig.1 b.The diameter of the circular orifice was 25 mm, and the dimensions of the ellipti- cal orifice were 50.4 mm ×12.4 mm.Note that the two types of orifices had the same area of 490 mm2and the effect of surface tension is considered insignificant in the current study.At the top of the tank, there was a slit of 2 mm ×20 mm (see Fig.1 a).The ventilation condition of the experiments was controlled by opening or closing the slit.
In the experiments, the water pressure at the tank bottom was monitored by a water pressure transducer placed adjacent to the bottom orifice with a measuring range of 0–5 kPa (HQ100, manu- factured by Baoji Huaqiang Inc.).The real-time water pressure data were recorded by the data acquisition system (INV306U Data Ac- quisition System, manufactured by China Orient Institute of Noise & Vibration) connected with a computer.The appearance of the air bubbles and the variation of the tank’s water level were both recorded with a high-frequency camera (Osmo Action, manufac- tured by DJI Inc.).The resolution of the camera was set to be 1980 ×1080 pixels, and the frequency was 240 frames/s.Back- lighting with a LED panel was utilized for the illumination in the experiments.
Before the experiments, the bottom orifice was sealed with wa- terproof tape, and water was pumped into the tank till a cer- tain water level ofH= 250 mm.To begin the experiment, the waterproof tape was torn carefully, and water flowed out of the tank through the bottom orifice.The experiments of tank emp- tying were conducted under four conditions, with different types of bottom orifices and ventilation conditions, as listed in Table 1 .The experiments were conducted at a room temperature of about 10 °C.
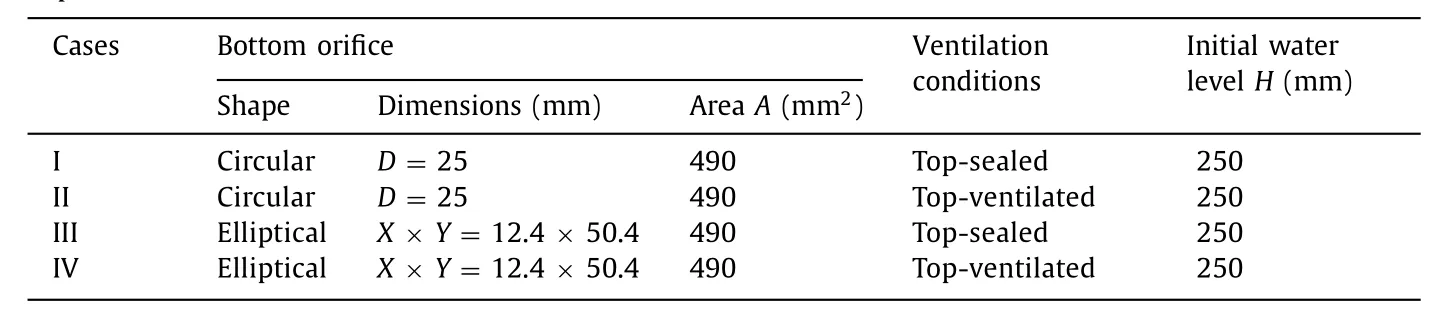
Table 1 Experimental conditions.
(1) Opening for water supply, (2) slit for ventilation, (3) tank, (4) bottom orifice, (5) water pressure transducer, (6) data acquisi- tion system, (7) computer, (8) camera, (9) submersible pump
Essentially, the emptying process of the water-filled tank is the mass exchange of the internal water and the ambient air, dur- ing which air penetrates into the tank periodically in the form of bubbles through the bottom orifice.The bubbles captured by the camera in the experiments are shown in Figs.2 and 3 .From the figures, the evaluation of bubbles in water can be mainly di- vided into three stages, including formation, deformation, and de- composition.Note that bubbles only occurred under the top-sealed condition, and none was observed under the top-ventilated condi- tion.
The evaluation of the first bubble generated at the tank bot- tom orifice in Case I, where the orifice was circular, and the top of the tank was sealed, is shown in Fig.2 .In the stage of bubble formation, as shown in Fig.2 a to 2c, the water level in the tank drops immediately as the exit is open, which results in the sud- den drop of air pressure in the upper space of the tank.Driven by such pressure difference inside and outside the tank, an air pas- sage is formed (see Fig.2 b), which allows the ambient air to enter the tank and forms a bubble at the orifice.The bubble grows grad- ually until the pinch-off(see Fig.2 c), which means the end of the formation stage.Subsequently, the air bubble, exhibiting a spher- ical shape, rises slowly in water and keeps deforming during this process, as shown in Fig.2 d to 2f.The bubble gets flattened grad- ually and its central part collapse first, which is the start of the decomposition stage, as shown in Fig.2 g.As the bubble moves up- wards further, its edge gets crushed and gradually breaks up into a group of small bubbles (see Fig.2 h to 2j).Finally, the small bub- bles reach the water surface and are released into the upper space of the tank.
When the bottom orifice is elliptical, the bubble character- istics are different from those generated at the circular one.Figure 3 shows the evaluation of the firstly observed bubble in Case III, with an elliptical orifice of 12.4 mm ×50.4 mm.The en- tire evaluation of the bubble can also be divided into three stages of formation, deformation, and and decomposition in the formation stage, a big bubble and a small bubble are generated at the bottom orifice, but they get consolidated shortly, as shown in Fig.3 a and 3 b.In the deformation stage, the geometry of the bubble changes from “spherical”to “smoke ring”, as shown in Fig.3 d.The “smoke ring”expends radially as it rises further, and at a certain location, its edge starts to be crushed (see Fig.3 e to 3 h).Compared with the bubble generated from the circular orifice, the ones formed at the elliptical orifice exhibit a wider variety of shapes.
The pressure variation at the tank bottom in the first three sec- onds of emptying is shown in Fig.4 a, and that during the entire emptying process is shown in Fig.4 b.From Fig.4 a, the pressure variation shows periodical features in Cases I and III.Once the emptying process starts, the pressure at the tank bottom drops rapidly fromP= 2450 Pa, i.e., the hydrostatic pressure for the ini- tial water depth ofH= 250 mm, to close to zero, within about 0.5 s.The pressure then rises to about 500 Pa and varies period- ically later.Additionally, the pressures measured at the bottom of the tank in Cases II and IV, with the ventilation through the slit on the top, are lower than the static pressure caused by the water depth.It indicates that negative air pressure occurs in the airspace of the tank, which is due to the limitation of air supply from the outside.The value of the negative air pressure is the difference be- tween the pressure measured at the tank bottom and the static pressure, which is about -600 Pa in both cases.
The mechanism for air bubble generation at the bottom ori- fice was studied based on the camera images and the pressure data.Once the pressure reaches the lowest, a bubble begins to be formed at the bottom orifice.As the pressure increases, the bub- ble grows and it completes the formation stage when the pres- sure reaches about 500 Pa.Afterwards, the bubble detaches from the bottom orifice and correspondingly, the pressure decreases.The water level in the tank keeps dropping and then the second bub- ble starts to be formed.Such processes are repeated until the end of the emptying process.Under the top-ventilated condition, the pressure variation becomes insignificant, as shown in Fig.4 .Based on the pressure variation, the frequency of bubble formation at the bottom orifice is about 2.5 Hz.According to the mathematical model proposed by Clanet and Searby [13] , during the emptying processes, the period of bubble formation depends on the physical properties of air and water, the water depth in the tank and the orifice geometry.
The variations of water level with time during the tank empty- ing process in Cases I–IV are presented in Fig.5 , where the solid black line is for the theoretical discharge curve.The equation for the theoretical discharge curve is derived in the following.The bot- tom orifice flow discharge rate is
Here,μ= discharge coefficient,A= area of bottom orifice, andh= water depth in the tank.Based on mass conservation, the vol- ume of water flowing out from the orifice during dt equals the reduced volume of water in the tank, i.e.,
whereA0 is the cross-sectional area of the tank.By integrating Eq.(2) and re-arranging the equation, the theoretical discharge curve is obtained:
The drainage efficiencies of the tank in Cases I–IV can be read from Fig.5 .From the results, the tank drainage efficiency under the top-sealed conditions is much lower than those under the top- ventilated conditions, which indicates the significant effect of ven- tilation on the drainage efficiency.Also, theh-tcurves under the top-ventilated conditions are still slightly lower than the theoreti- cal curve, indicating the limitation of air supply by the slit on the top of the tank in the current experiments.
In addition, theh-tcurves under the top-ventilated conditions are concave, consistent with the theoretical curve, which means that the discharge rate is larger at the early stage of the emptying process while gets smaller with time.Under the top-sealed con- ditions, theh-tcurves are convex slightly, indicating an increase in the discharge rate with time.Under the top-ventilated condi- tions, the flow discharge rate is dominated by gravity, while un- der the top-sealed conditions, the flow discharge rate is affected by the gravity, the pressure difference inside and outside the tank, as well as the bubbles.The different dominant factors lead to the two types ofh-tcurves under different ventilation conditions.
Based on Fig.5 , under the top-sealed conditions, the empty- ing time of the filled tank in Case III with the elliptical orifice is slightly larger than that in Case I with the circular orifice.Cao et al.[16] reported that for orifice flow, under the condition of the same area, a longer wetting perimeter of the orifice can result in larger frictional resistance to outflow.In the current experiments, the cir- cular and elliptical orifices have the same area, while their wetting perimeters are 78 mm and 108 mm, respectively, which indicates a smaller frictional resistance from the circular orifice to the out- flow.It is the reason for the slightly higher drainage efficiency of the circular orifice.
In conclusion, the emptying processes of a water-filled tank with both circular and elliptical bottom orifices were studied ex- perimentally in this paper.The effects of orifice shape and venti- lation conditions on the air-water flow features during the empty- ing process were investigated.The bubble features were analyzed based on the water pressure measurement and the synchronous video recording.Three stages of bubble development were divided, including the formation stage, the deformation stage, and the de- composition stage.During the emptying processes, the water pres- sure at the tank bottom presented periodical features and varied between 500 Pa and close to zero in the experiments.The wa- ter pressure variation corresponded to the generation frequency of bubbles at the orifice, which was about 2.5 Hz in the current study.The drainage efficiencies of the tank varied with the venti- lation conditions.To achieve efficient water drainage in industries, the ventilation condition should be considered carefully for suffi- cient air supply.Additionally, under the top-sealed conditions, the drainage efficiency of the tank with the circular orifice was slightly higher than that with the elliptical one, which was due to the less frictional resistance of the circular orifice to the outflow.
Declaration of Competing Interest
The authors declare that they have no known competing finan- cial interests or personal relationships that could have appeared to influence the work reported in this paper.
The authors declare that we have no financial and personal re- lationships with other people or organizations that can inappropri- ately influence our work, there is no professional or other personal interest of any nature or kind in any product, service and/or com- pany that could be construed as influencing the position presented in, or the review of, the manuscript entitled.
Acknowledgment
The writers gratefully acknowledge financial support from the Fundamental Research Funds for the Central Universities (2020QNA4017).
杂志排行
Theoretical & Applied Mechanics Letters的其它文章
- Detection of mechanical stress in the steel structure of a bridge crane
- Noether symmetry method for Birkhoffian systems in terms of generalized fractional operators
- Optimization of the forearm angle for arm wrestling using multi-camera stereo digital image correlation: A preliminary study
- Displacement reconstruction and strain refinement of clustering-based homogenization
- Validation of actuator disc circulation distribution for unsteady virtual blades model
- Effect of a rigid structure on the dynamics of a bubble beneath the free surface
