THE DECAY ESTIMATES FOR MAGNETOHYDRODYNAMIC EQUATIONS WITH COULOMB FORCE*

Wenxuan ZHENG (郑文轩)

School of Mathematical Sciences, Xiamen University, Xiamen 361005, China School of Mechanical and Electronic Engineering, Tarim University, Alar 843300, China E-mail : zwxtlmdx80@163.com

Zhong TAN (谭忠)

School of Mathematical Sciences and Fujian Provincial Key Laboratory on Mathematical Modeling and Scientific Computing, Xiamen University, Xiamen 361005, China E-mail : ztan85@163.com

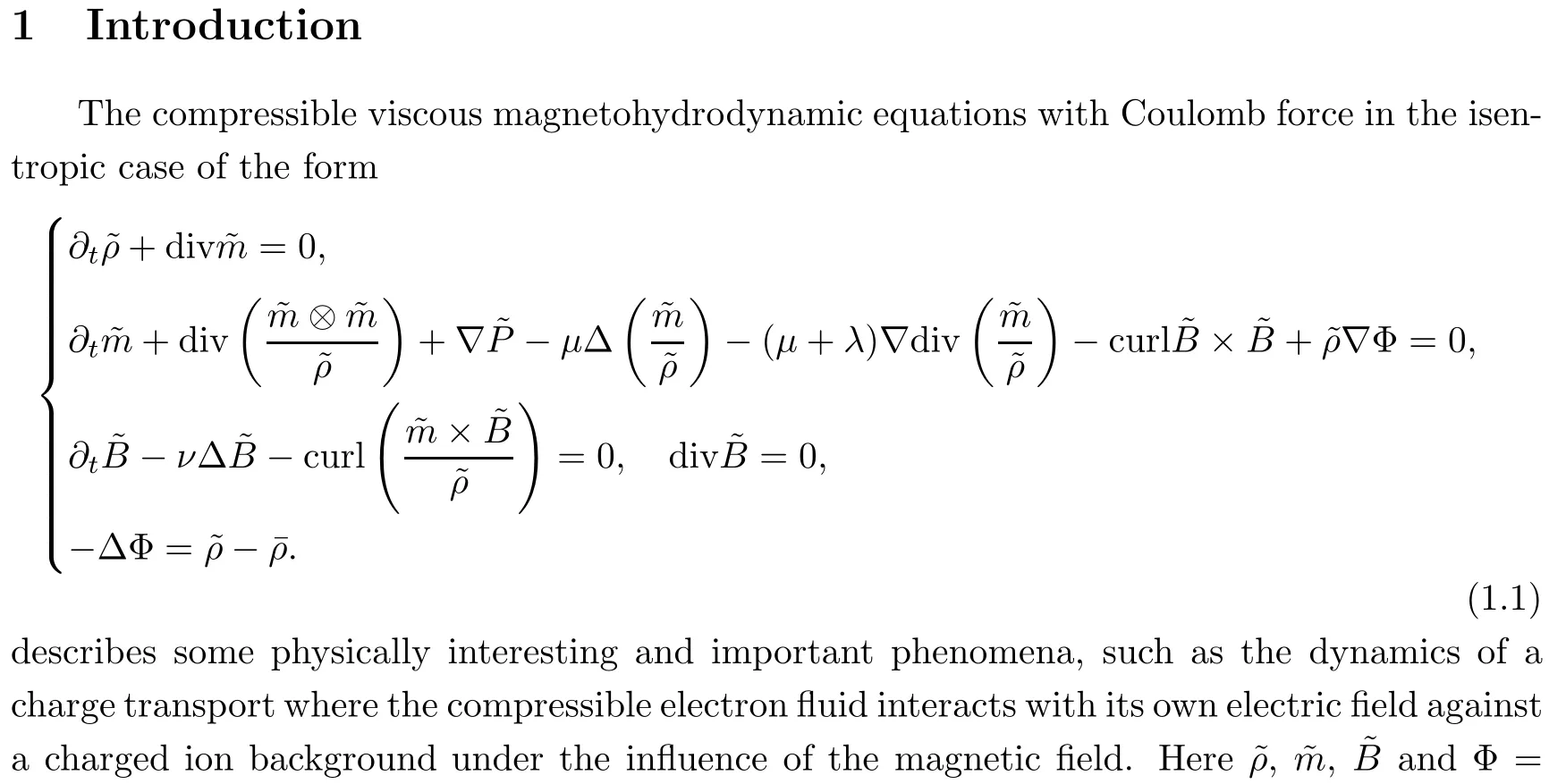
Φ(x,t)are the density,momentum,magnetic field,and electric potential.The pressureP=P(ρ)is a smooth function withP′(ρ)>0 forρ>0.µ,λare the viscosity coefficients of the flow satisfyingµ>0 and 2µ+3λ≥0.The constantν>0 is the magnetic diffusivity acting as the magnetic diffusion coefficient of the magnetic field.is the positive constant background ionic density.We complement(1.1)with the Cauchy data

When we neglect the viscous terms,that is,λ=ν=µ=0,(1.1)will become the compressible ideal MHD-Poisson equations,which can describe some important phenomena in astrophysics;e.g.solar flares(cf.[3,27,35]).For ideal MHD-Poisson equations,Federbush,Luo and Smoller[11]first proved the existence of axi-symmetric stationary solutions whenγ>2 by a variational method.Wang and Liu[31]proved the existence of stationary solutions whenJang,Strauss,and Wu[17]proved the existence of axi-symmetric stationary solutions with a constant rotation speed whenby using an implicit function theorem.Yang[34]showed the local-in-time existence result and quasi-neutral limit result.

When we neglect the Coulomb force,(1.1)will become the compressible viscous MHD equations,and then there are many results that flow from this.For the 1-D or 2-D cases,we refer to[2,4,8,30]and the references cited therein.For the three-dimensional case,for the local or global existence,and the convergence rates of weak,strong,and smooth solutions the we refer to[1,9,10,15,16,21,22]and the references cited therein.

When the magnetic field is not taken into account,one has the compressible Navier-Stokes-Poisson equations to model viscid gaseous stars.For the existence of the strong solution and weak solutions,we refer to[5,13,14,18,25,26]and the references cited therein.Li,Matsumura and Zhang[20]obtained the optimalL2time-decay rate and the optimalL∞time-decay rate in three-dimensions.Comparing with the compressible Navier-Stokes-Poisson equations,we give the decay rate of the magnetic field inL2.For more results about the long time behavior of the global solution of the Navier-Stokes-Poisson equations,we refer to[19,23,32,33]and the references cited therein.

with

1.1 Global Existence result

We now record a Existence result of the solution to(1.1).The proof of the existence theorem has been described in[28];for the sake of convenience,we just state the results in the following theorem:

1.2 Convergence rate

2 Spectral Analysis for Linearized Equations

Without loss of generality,we can assume thatP′(1)=1.Let us consider the linearized system

4 L2-decay for Nonlinear Part

Thus,we obtainN≲δ2+N2;that is,N≲δ.

Appendix

A Analytic tools

Lemma A.1Lettingr1>1,0≤r2≤r1,it holds that