Design of Full-Ocean-Depth Self-Floating Sampler and Analysis of Factors Affecting Core Penetration Depth

REN Yugang, LIU Yanjun, DING Zhongjun, LIU Baohua, and ZHANG Jianhua

Design of Full-Ocean-Depth Self-Floating Sampler and Analysis of Factors Affecting Core Penetration Depth

REN Yugang1), 2), 4), LIU Yanjun1), 3), *, DING Zhongjun2), LIU Baohua2), and ZHANG Jianhua1), *

1),,250061,2),266237,3),,266237,4),,150001,

The hadal zone (ocean depths of 6–11km) is one of the least-understood habitats on Earth because of its extreme conditions such as high pressure, darkness, and low temperature. With the development of deep-sea vehicles such as China’s 7000m manned submersible Jiaolong, abyssal science has received greater attention. For decades, gravity-piston corers have been widely used to collect loose subsea-sediment long-core samples. However, the weight and length of the gravity sampler cables and the operating environment limit sampling capacity at full ocean depths. Therefore, a new self-floating sediment sampler with a spring-loaded auto-trigger release and that incorporates characteristics from traditional gravity-driven samplers is designed.This study analyzes the process by which a gravity-piston corer penetrates the sediment and the factors that affect it. A formula for obtaining the penetration depth is deduced. A method of optimizing the sampling depth is then developed based on structure design and parametric factor modeling. The parameters considered in the modeling include the sampling depth, balance weight, ultimate stress friction coefficient, dimensions of the sampler, and material properties. Thus, a new deep-sea floating parametric sampler designed based on virtual prototyping is proposed. Accurate values for all the design factors are derived from calculations based on the conservation of energy with penetration depth, analyses of the factors affecting the penetration depth, and analyses of the pressure bar stability. Finally, experimental data are used to verify the penetration-depth function and to provide theoretical guidance for the design of sediment samplers.

full-ocean-depth sampling; sediment sampler; factor parameter design; hadal science

1 Introduction

The hadal zone (6–11km) is one of the least-understoodhabitats on Earth due to its extreme conditions such as highpressure, darkness, and low temperature (Wolff, 1970; Cle- mens and Tiedemann, 1997;Zachos., 2001). The zone features rich biodiversity and abundance, but our knowledge of the ecosystem is minimal due to technical limitations.

Recently, there have been new developments in under- water vehicle technology, such as human occupied vehicles (Liu, 2014); remotely operated vehicles (Yoshida, 2007); autonomous underwater vehicles; (Bowen, 2008); and landers (Jamieson, 2009), as shown in Fig.1.

In 2016, China’s submersible and hadal lander comple- ted the 37th Dayang Cruise in the Mariana Trench. The diving area was along the southern slope of the Challenger Deep where the seafloor is approximately 6300–8300mdeep. During this expedition, the submersible was deploy- ed on nine dives during which it made several discoveries (Cui., 2017;Gao., 2017). However, the ability to sample sediment below 11000m is limited, and instru- ments capable of collecting samples at full ocean depths need to be urgently developed (Blankenship-Williams and Levin, 2009; Nunoura, 2018).

Many different systems have been developed to collect continuous samples from a variety of seafloor environments. Mingram. (2007) developed a piston corer that allowed sediment samples to be collected with littledisturbance and intact layering, due to casing in the borehole. Bu. (2000, 2001) in their studies on seabed sampling analyzed the structures and operating principles of non-controllable, controllable, and float-based seabed samplers. They also calculated the ultimate height of the sampling tube when sediment entered the tube to determine the ‘pile effect’ using the Mohr-Coulomb strength criterion and presented a computational method for developing sampler design parameters. Zhang(2009)designed a novel seabed sediment sampler in which seawater pressure was used to compress hydraulic oil in the sealing device to seal the sampler. Qin(2013) investigated low-disturbance sampling and fidelity techniques for seabed surface sampling, developed techniques for maintaining and supplementing the pressure in a pressure- retaining barrel, and designed a fidelity sampler.

Fig.1 Jiaolong manned submersible and Hadal Lander A.

Studies suggest that the most common technique used for deep-sea sediment sampling is ship-borne gravity-dri- ven core sampling. Offshore operations are extremely dif- ficult due to factors such as complicated sea conditions, ship heave, and yaw. For seabed sampling, appropriate gra- vity-driven core sampling devices, 1–5m in length and 200–800kg in weight, have very strict requirements for the winches and cables used for deployment. Complex ocean conditions increase the difficulty of launching such a system over the side and into the water, and stranded cables are often severed due to cable system failures. Despite these challenges, there is the huge demand for scientific open-ocean exploration to understand the deep ocean, and thus, conducting environmental sampling in the hadal zone becomes critical. However, traditional gravity coring is limited by winches, cable lengths, armored cables, sup- port ships, and ocean conditions; hence, it is necessary to develop new seabed samplers to provide technical support for full-ocean-depth scientific research.

The aim of this study is to design a new self-floating sampler that incorporates elements from the traditional gra- vity-driven samplers and addresses the abovementioned issues. We will then examine the factors that affect the penetration depth and optimize them for our design. Finally, we will compare the theoretical results with those obtained using a prototype device and historical data. This work represents a new solution that can be used in the design of future samplers for deep ocean research.

2 Floating Seabed Sampler Design

2.1 Design Principles

Based on the study of the working tools for the Jiaolong manned submersible, we researched small-scale floating seabed sediment samplers that address the issues descri- bed in Introduction. A mechanically triggered release me- chanism allows the proposed sampler to float once sampling is complete, without the need for a launch and recovery system or action by the support ship. A miniaturization design strategy is used to achieve rapid deployment and retrieval by two or three persons. This eli- minates the need to lay and recover cables, which reduces the device weight and removes the limitations associated with ship-borne launch and recovery systems so that full- ocean-depth sampling can be achieved. Hence, multi-tube sampling can meet the demands of scientific research.

The floating seabed sampler developed in this study can operate on the seafloor down to 11000m. It impacts the seabed sediment under gravity, drops its descent weight by mechanical release, and returns to the surface (Fig.2). The device comprises of an upper floating mechanism, lower load-rejection balance weight mechanism, device re-lease cylinder spring, mechanical release mechanism, sam- pling tube gripper mechanism, grounding buffer spring, sampling tube, and section-type pawl spring. Specifically, the upper floating mechanism is composed of a lifting hook,high-intensity deep-sea buoyancy material, and an orientedstabilizer column. The lower load-rejection weight balance mechanism is composed of a balanced weight base and an oriented stabilizer column.

Fig.2 Schematic diagram of full-depth self-floating sampler.

A schematic diagram of the release mechanism is shownin Fig.3. The upper floating and lower load-rejection weight balance mechanisms are assembled using four uniformly distributed oriented stabilizer columns with pre-compres- sed cylindrical springs in the center. The mechanical release mechanism is triggered once the device is grounded. The compression springs then produce large spring forces that eject the upper release floating mechanism. The balanced weight base remains on the seafloor when the springs are released, and the upper release floating mechanism is fitted with the material, providing positive buoyancy.

The sampling tube uses a piston-type design, and there is a section pawl spring at the bottom that allows the se- diment sample to enter the sampling tube unidirectionally. The collected sample is blocked by the pawl spring to pre- vent sample loss during ascent.

Fig.3 Schematic diagram of mechanical release mechanism.

2.2 Operating Principle

A flowchart of the full-depth self-floating sampler pro- cess is illustrated in Fig.4. Before deployment, the upper release and lower load-rejection balance weight mechanisms are assembled and locked in place by hooks at both ends; the balance weight in the lower mechanism is used to pre-compress the sampler’s release springs. Moreover, the system is tested on the deck: its components, including the mechanical trigger-release mechanism, compression springs, and oriented stabilizer columns, are checked.

There is a hoist ring on the upper section of the sampler. During deployment, if conditions permit, the sampler may be lifted and submerged using a small shipboard crane. The sampler is then hoisted over the side and lowered into the water. The sampler may also be lifted by two or three persons using ropes and slowly lowered into the water. The sampler then descends through the water column under its own weight.

Fig.4 Flowchart of full-depth self-floating sampler.

The sampler is provided with a 2mm Kevlar cable, which is not used for deployment. When the upper release floating mechanism reaches the surface, it can be retrieved using this cable. If the sampler grounds and fails to release, it may also be pulled back to the surface using this cable.

3 Factors Contributing to Sampling Depth

3.1 Analytical Calculation of Penetration Depth

The sampler can obtain high velocity, depending on its weight and the conditions in the water column. The sampling tube penetrates the sediment by inertial force. During insertion, the mechanical release mechanism is triggered, and the balanced weight base will remain on the seafloor after release. The sampler ascends due to the positive buoyancy provided by the flotation material. Du- ring ascent, the sampler and sediment in the sampling tube produce relative displacement and negative pressure; this draws in the sediment and improves sample retention. During extraction, the pawl spring mechanism is used to securely block the lower opening of the sampling tube to prevent core sample loss.

We used the conservation of energy principle to analyze the penetration depth of the sampler. When the sampler is falling, its kinetic energy is

whereis the mass of the sampler, andis the velocity of the sampler when it contacts the sediment. The sampler has four sets of annularly distributed sampling tubes. Thus, each set of sampling tubes has 1/4 of the sampler’s initial kinetic energy.

If the penetration depth is the length of sediment within the sample tube, then the potential energy of the sampler on the surface is

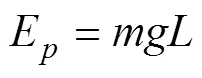
whereis the acceleration due to gravity, andis the penetration depth of the sampling tube.

When the sampler reaches the seabed and penetrates the sediment, due to the mechanical properties of the seafloor, the sampler will encounter various types of resistance, including frictional resistance (occurrence of relative displacement between the sampling tube and the se- diment), vertical water pressure, and slope resistance of the cross section of the sampling tube tip. The dominant resistance is the frictional force, which is generally expressed as

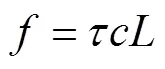
whereis the ultimate frictional coefficient of the sediment (kgcm−2), andis the circumference of the sampling tube (cm).

During penetration, the work done to overcome the frictional force is as follows:

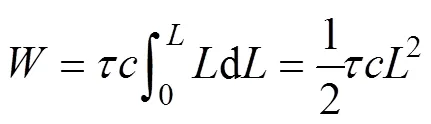
According to the principle of work and energy,

That is,

Therefore, by solving Eq. (5), we can obtain the value of the penetration depth as

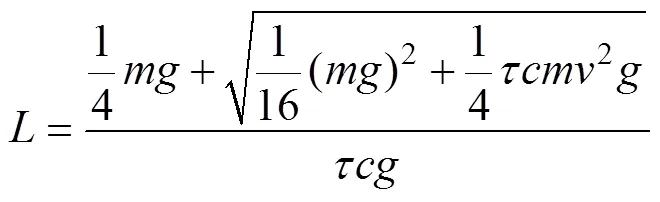
3.2 Factors Contributing to Penetration Depth

According to the conservation of energy principle, the penetration depth of the sampling tube is dependent on its kinetic energy during the descent. The kinetic energy can be expressed as in Eq. (1). Based on our analysis, the kinetic energy is directly proportional to the velocity squared, provided that the mass of the device remains constant (Hoyaux and Ladanyi, 1970). Therefore, the penetration depth canbe improved by increasing the sampler’s initial descent velocity.

According to fluid mechanics, an object falling through a water column is affected by gravity, buoyancy, and motion resistance. The force of gravity is constant and proportional to the object’s weight. Buoyancy is constant and proportional to the object’s volume. The motion resistance is closely related to the object’s relative displacement ve- locity, and higher velocity will lead to greater resistance. When the force due to gravity and the sum of the resistance and buoyancy reach equilibrium, the object achieves a balanced state and falls at a constant velocity. This is the maximum initial penetration velocity of the sampler. Based on the computation and research by scholars in China and other countries, the initial underwater penetration velocity of the sampler can be expressed as follows:

Here,andvare the initial and final descent velocities of the sampler, respectively;ρandρare the densities of the sampler and the sea water, respectively;Cis the coefficient of resistance of the seawater against the sampler;is the maximum cross-sectional area of the sampler; andis the free-sinking distance.

The sinking distance and initial velocity of the sampler have a nonlinear relationship. The sampling tube is constantly affected by the geotechnical resistance of the se- diment during penetration. As the sinking distance increases, the acceleration decreases continuously, and the velocity increases more slowly. Finally, the velocity will become constant when the gravity and resistance reach equilibrium. In other words, the ultimate velocity is re- ached, and it is equivalent to the velocity atX. At this point,

By solving this, we obtain

Then, by substituting Eq. (9) into Eq. (6), we obtain

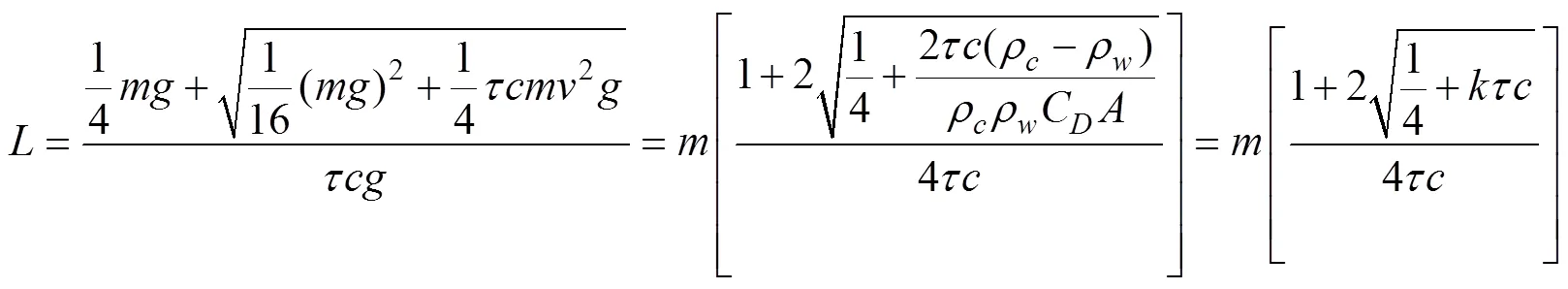
In this study,ρ=11.3×10−3kgcm−3andρ=1.025× 10−3kgcm−3. In addition

As shown in Eq. (10), the penetration depth of the sampler is closely related to the mass and gravity of the sampler, the circumference of the sampling tube, the stress frictional coefficient of the sediment, and the maximum cross- sectional diameter. The acceleration due to gravity is constant, anddepends on the nature of the sediment (., clay ooze,=0.05–0.08kgcm−2; sandy clay ooze,=0.08–0.15kgcm−2; clay sand,=0.08–0.15kgcm−2; sand,=0.20kgcm−2).

3.3 Finite Element Analysis of Sampling Process

To ensure that the self-floating sampler can achieve the structural strength required at full ocean depth across mul-tiple designs, we conducted a finite element analysis of thesampler structure using ANSYS Workbench. The sampling tube was made of PVC, and the rest of the structure was made of 316L stainless steel.

The mechanical module of ANSYS Workbench was usedto provide the mesh for finite element analysis, and a solid entity model was used for the 3D geometric division. To improve the quality and processing speed of the mesh, the patch-independent method with tetrahedral mesh division (based on ICEM CFD) was used. This generated a volume mesh and a mapping to the surface that was used to generate the surface mesh. The global grid fine adjustment relevance value was 65. The same time was set for the dimensional parameters for sizing the global units. To optimize the quality of the grid, ACTS was adopted on the sides and the curvature mode. The area around the support plate was divided into a grid and 10 layers. The minimum face size was 0.002mm, and the maximum face size was 2mm. The skewness function of ANSYS was used for the statistics. The grid contained 960000 cells and 1.62 million nodes with an average separation of 0.24mm (as shown in Fig.5). This was a relatively good grid quality interval. The load capacity of the sampler structure was investigated by applying a load force of 1ton. The finite element analysis showed that the body frame of the sampler was deformed by 0.003mm, a very small de- formation that meets the requirements for the load deformation capacity (as shown in Fig.6).

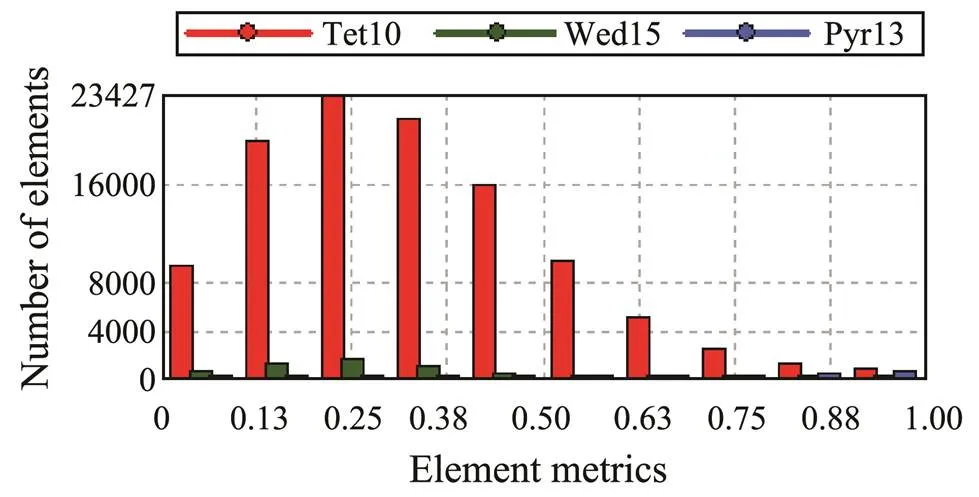
Fig.5 Grid quality statistics base on ANSYS Workbench.

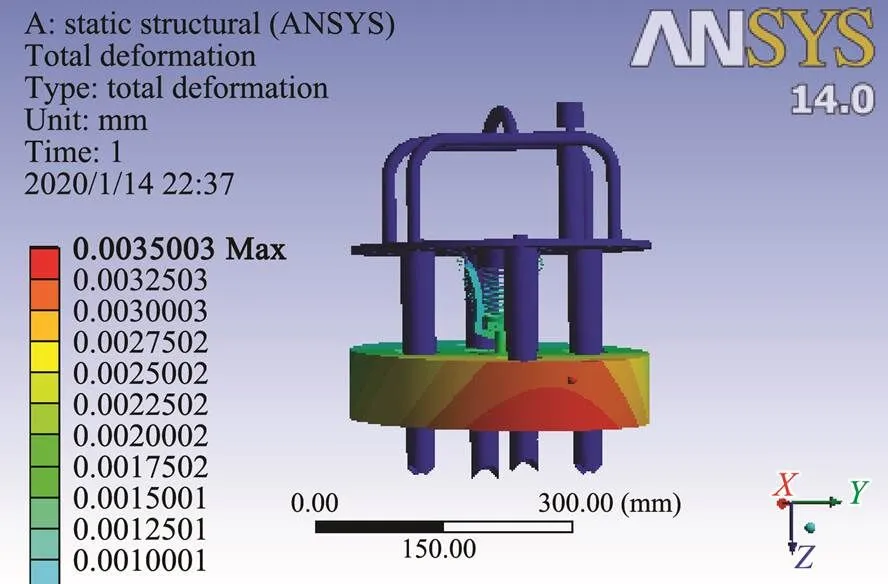
Fig.6 FEM analysis of full-depth self-floating sampler base on ANSYS Workbench.

The inner wall of the sampler pipe was made from PVC. Therefore, the coefficient of friction between the sediment and the inner wall was much smaller than that between the sediment and the steel outer wall. Hence, during the modeling process, we mainly considered the contact between the outer wall and the sediment, as well as the squeezing effect on the outside of the pipe during penetration.

A temporary sampling tube 60mm in diameter and 500mm long was investigated using an elastic-plastic model of soil. To model the penetration process and deformation with rigid body constraints, the displacement penetration method was adopted. The sampling tube parameters for the contact with the soil surface were as follows: the coefficient of friction was 0.45, the entire grid size was 0.02mm, and the overall grid size for the soil was 0.04mm.

According to the finite element analysis data, when the sampler penetrates the sediment to a depth of 500mm, 1123J of external work is required (Table 1).

Table 1 External work during penetration

Meanwhile, according to Eq. (9), the initial penetration velocity of the sampler is 1.26ms−1, and the penetration depth is 500mm. When the sediment and loss of power due to friction with the sampling tube wall are ignored, the kinetic energy is E=1/22=(1/2)×220×1.26×1.26=174.63J. It is assumed that penetration depthis the length of sediment in the penetration tube, the gravitational potential energy isE=220×9.8×0.5=1078J; and the sampler penetration energy is EEE=174.63+1078=1252.63J. Thus, the sampler can provide injection energy, and the finite element analysis of the sampler needs external work to increase. Therefore, the theoretical calculation is more reasonable.

3.4 Sampling Tube Optimization and Sampler Pressure Bar Stability

According to the equation for calculating the sampling depth, the penetration depth of the sampler depends on its mass, the acceleration due to gravity, sediment limit stress, friction coefficient, and the maximum diameter. Consi- dering the miniaturized design, four sampling pipe ring design, flexible cloth and recyclable design goals, sampling weight limit of 180kg (150kg target weight), and sampling depth limit of 500mm, the sampling depth is only limited by the sampling pipe diameter and friction coefficient, which depends on. Therefore, the following actions were taken:

1) A water-lubricated composite material was used to reduce the friction between the sampling tube outer wall and the sediment. The outer wall was coated with a self- lubricating ethylene material so that the limit of the friction coefficient was reduced to≤0.01kgcm−2.

2) Sampling tubes with diameters of 30, 40, 50, and 60 mm were designed for the sampling pipe sections, and the sampling depths were calculated. According to Eqs. (9) and(10), the corresponding final velocities are 3.57, 3.09, 2.77, and 2.53ms−1, respectively. The relationship between the sampling depth and sampling volume for tubes of dif- ferent diameters is illustrated in Fig.7.

Fig.7 Sampling depth and volume under different diameters.

Based on this figure, the optimal sampling diameter was selected as the intersection point of the sampling depth and the sampling volume; hence, the inner diameter of the sampling tube inner diameter was set to 50mm.

The penetration resistanceFand the buckling critical pressureFduring penetration were compared. The safety coefficient of the sampling tube during penetration is

The penetration resistance can be expressed as

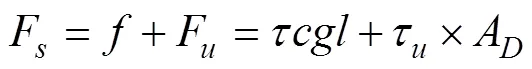
The inertia moment isF=×A, whereFis the end face resistance. According to Euler’s formula and the cri- tical pressure theory for hinged slender bars on both ends, the algorithm for checking the stability of the pressure bars is as follows:

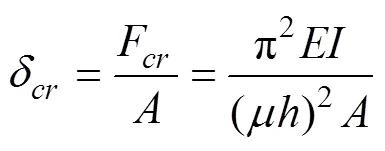
The inertia moment is=2whereis the inertial radius of the cross section; thus,

. (15)

The flexibility value(with dimension 1) is introduced as

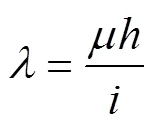
Substituting Eq. (16) into Eq. (15) gives the equation for calculating the critical stress:

The flexibility value has significant parametric effects on the critical stress and reflects factors such as length, cross section, constraint conditions, and shape.

The sampling tube is made from304 stainless steel with an elasticity modulusof 210GPa and proportional limit ofδ=280MPa.

Furthermore, the effect of the sampling tube diameter on the tube stability was investigated. The critical bucklingpressureFand penetration length of 1.5m-long sampling tubes with different pipe diameters were studied (Fig.8), along with the changes in the penetration resistanceFand penetration length (Fig.9).

Fig.8 Curve of instability critical pressure change with pe- netrative length.

Fig.9 Curve of penetration resistance change with penetrative length.

Based on the calculations, the critical buckling load is greater than the penetration resistance, and the safety coefficient is greater than 1.2. Thus, the sampling tube meets the stability requirements of the pressure bars.

4 Experimental Verification

4.1 Experimental and Theoretical Data

Based on the sampler design, a prototype was manufactured with a mechanical release mechanism as shown in Fig.10.

The prototype included a buoyancy material, support for the middle framework, a grounding release mechanism, a sampler orientation mechanism, four sampling tubes, pawlsprings, and a 150kg balance-weight-block. The total mass of the prototype device was 180kg, and the diameter of each sampling tube was 50mm.

The prototype was tested in the gulf of the National Deep Sea Base shown in Fig.11.Considering the geolo- gical environment, an area of sandy clay was selected as the sampling object. The sampler was launched from the back of the ship’s deck (approximately 5 m high above the ship and 6m deep in water). After recovery, the sampling tube was removed, and the sampling length was measured. These steps are demonstrated in Fig.10, and the experimental data are presented in Table 1.

Fig.10 The prototype of full-depth self-floating sampler.

Previous marine geological research has shown that the geomechanical parameters of deep-sea sediments vary with sampling depth and location. The ultimate frictional coefficient varies substantially across different locations and se- diment types. The parameters are summarized in Table 2.

Table 2 Geomechanical parameters of abyssal sediments

Based on historical geological data obtained from the Jiaolong voyage, the seabed conditions in the flat area of the Mariana Trench in the western Pacific Ocea n were used as a reference, and sandy ooze clay was selected for the calculation parameters in this study. According to Eq. (10) and the parameters of the sampler used in this experiment, the sampler penetration depths are presented in Table 3.

Fig.11 Offshore test. a, launch on the ship; b, recovery; c, measure and analysis of the sample.

4.2 Analysis of Experimental Results

By comparing the theoretical and experimental data, we conclude that the equations agree well with the actual results. In addition, analysis of the test results suggests that a functional relationship exists between the ultimate frictional coefficient of the sediment and the sampler penetration depth. To maximize the penetration depth, the dead weight should be increased and the coefficient of friction between the sampling tube and the sediment should be minimized.

Based on calculations using Eq. (10), the penetration depth is closely related to the mass and density of the sampler, the circumference and maximum diameter of the penetrating tube, the ultimate stress frictional coefficient, and the seawater density. Therefore, Eq. (10) may be used as a reference to provide a theoretical basis for sampler design.

5 Conclusions

In this study, we designed a new self-floating seabed sampler that incorporates elements from traditional gravity-driven samplers. We analyzed the properties that affect the penetration depth and developed an equation that can be used for optimization. This equation was applied to the new sampler design, and the results were compared with the experimental results obtained using a prototype sampler and historical data. The comparison revealed that the theoretical results agree well with the experimental results. Therefore, this work can be used as a reference in future sampler designs.

However, there were some limitations that we hope to address in future works:

1) Since the Jiaolong manned submersible is currently undergoing technical upgrades we could only conduct ex- periments in the harbor. Therefore, we plan to carry out sea trials next year during the next voyage.

2)At present, the full-ocean-depth self-floating sampler can be used only on flat areas of the abyss. Hence, we plan to develop a self-adjusting sampler that can operate on sloped areas.

3) In the experiments, we observed that the factors affecting the penetration depth varied with the location, depth, and substrate with a nonlinear relationship. We plan to conduct further research in the future to optimize these nonlinear parameters using the fuzzy control theory.

Nomenclature

ParamaterMeaning AMaximum cross-sectional area of the sampling tube CCircumference of the sampling tube CDCoefficient of friction between seawater and the sampler DMaximum diameter of the sampling tube EElasticity modulus EkKinetic energy of the sampler EpPotential energy of the sample EtSampler penetration energy FFrictional force FcrCritical buckling pressure FsResistance to penetration FuEnd face resistance gAcceleration due to gravity iInertial radius of the cross section IMoment of inertia LPenetration depth of the sampling tube mMass of the sampler n1Safety coefficient of the sampling tube during penetration vFinal descent velocity of the sampler when it contacts the sediment v0Initial descent velocity of the sampler WWork done to overcome friction XFree-sinking distance of the sampler δcrCritical stress δpProportional limit λFlexibility ρcDensity of the sampler ρwDensity of the seawater τUltimate frictional coefficient of the sediment

Acknowledgements

This research was jointly supported by the Stable Supporting Fund of Science and Technology on Underwater Vehicle Technology (No. JCKYS2019604SXJQR-06), the National Natural Science Foundation of China-Marine Sci-ence Research Center of Shandong Provincial GovernmentJoint Funding Project (No. U1606401), the National Na- tural Science Foundation of China (No. 61603108), the Taishan Scholar Project Funding (No. tspd20161007), the National Key Research and Development Plan (Nos. 20 16YFC0300704, 2017YFC030660). We would like to give special thanks to the crew on Leg II of the 37th China Dayang Cruise along the southwestern area of the Ma- riana Trench. The diving area on this leg is on the southern slope of the Challenger Deep between 6300 and 8300 m deep. Valuable sediment data and samples were obtained during this voyage and the data provided were crucial to this work.

Blankenship-Williams, L. E., and Levin, L. A., 2009. Living deep: A synopsis of hadal trench ecology., 43 (5): 137-143, DOI: 10.4031/mtsj.43.5.23.

Bowen, A. D., Yoerger, D. R., and Taylor, C., 2008. The Nereus hybrid underwater robotic vehicle for global ocean science operations to 11000m depth.. Hawaii, 867-873.

Bu, J. W., Yan, T. N., and Bu, S. R., 2000. Structure and working principle of controllable sampler., 19 (4): 100-104 (in Chinese with Eng- lish abstract).

Bu, J. W., Yan, T. N., and Cheng, H. Z., 2001. Structure and working principle of floating-balls sampler., 20 (1): 109-112 (in Chinese with English abstract).

Clemens, S. C., and Tiedemann, R., 1997. Eccentricity forcing ofPliocene–early Pleistocene climate revealed in a marine oxygen-isotope record., 385 (6619): 801-804, DOI: 10.10 38/385801a0.

Cui, W. C., Hu, Y., and Guo, W., 2017. Chinese journey to the Challenger Deep: The development and first phase of sea trial of an 11000-mARV., 51 (3): 23-35, DOI: 10.4031/MTSJ.51.3.2.

Gao, X., Ding, K., and Ren, Y. G., 2017. Target deployment and retrieval using Jiaolong manned submersible in the depth of 6600m in Mariana Trench., 31: 618- 623, DOI: 10.1007/s13344-017-0071-9.

Hoyaux, B., and Ladanyi, B., 1970. Gravitational stress field around a tunnel in soft ground., 7 (1): 54-61, DOI: 10.1139/t70-005.

Jamieson, A. J., Fujii, T., Solan, M., and Priede, I. G., 2009. HADEEP: Free-falling landers to the deepest places on earth.,43 (5): 151-160, DOI: 10. 4031/mtsj.43.5.17.

Liu, F., 2014. Jiaolong manned submersible: A decade’s retrospect from 2002 to 2012., 48 (3): 7-16, DOI: 10.4031/mtsj.48.3.14.

Mingram, J., Negendank, J. F. W., Brauer, A., Berger, D., Hen- drich, A., Köhler, M., and Usinger, H., 2007. Long cores from small lakes–Recovering up to 100m-long lake sediment sequences with a high-precision rod-operated piston corer (Usinger-corer).,37 (4):517-528, DOI: 10.1007/s10933-006-9035-4.

Nunoura, T., Nishizawa, M., and Hirai, M., 2018. Microbial di- versity in sediments from the bottom of the Challenger Deep, the Mariana Trench., 33 (2): 186- 194, DOI: 0.1264/jsme2.ME17194.

Qin, H. W., Chen, J. Q., Wang, J. J., and Chen, Y., 2013. Design and sea trial research of new hydrostatic corer., 24 (7): 942-945 (in Chinese with English abstract).

Wolff, T., 1970. The concept of the hadal or ultra-abyssal fauna., 17: 983-1003, DOI: 10.1016/0011-7471 (70)90049-5.

Yoshida, H., Ishibashi, S., and Watanabe, Y., 2009. The ABISMO mud and water sampling ROV for surveys at 11000m depth.,43 (5): 87-96, DOI: 10. 4031/MTSJ.43.5.31.

Zachos, J., Pagani, M., and Sloan, L., 2001. Trends, rhythms, and aberrations in global climate 65Ma to present., 292 (5517): 686-693, DOI: 10.1126/science.1059412.

Zhang, Q. L., Liu, G. J., and Guo, Y. L., 2009. The structural design and dynamic analysis of collecting process of new type seabed sampler., 28 (4): 20-23 (in Chinese with English abstract).

. E-mail: lyj111ky@163.com

E-mail: jhzhang@sdu.edu.cn

November 22, 2019;

January 14, 2020;

March 4, 2020

(Edited by Chen Wenwen)