Theoretical expressions for soil particle detachment rate due to saltation bombardment in wind erosion
2020-09-24XuYangLiuWenXiaoNingZhenTingWang
XuYang Liu,WenXiao Ning,ZhenTing Wang
1.Key Laboratory of Desert and Desertification, Northwest Institute of Eco-Environment and Resources, Chinese Acade‐my of Sciences,Lanzhou,Gansu 730000,China
2.University of Chinese Academy of Sciences,Beijing 100049,China
ABSTRACT
Keywords:wind erosion;saltation bombardment;cohesive soil;anti-erodibility
1 Introduction
Soil erodibility is a practical concept representing the susceptibility of soils to water or wind erosion(Cook 1937; Bryanet al., 1989; Webb and Strong,2011; Funk, 2016).It is often quantificationally expressed as soil erodibility index,i.e., the potential mass loss per unit area per unit time from a bare,smooth, uncrusted surface, while modeling wind erosion (Chepil and Woodruff, 1963; Woodruff and Siddoway, 1965; Wilson, 1994; Fryrearet al., 1998; Van Peltet al., 2004).Since soils consist of inorganic and organic solids, water, and air (Lal and Shukla, 2004),it is naturally assumed that soil erodibility index is a function of the properties of these four components.Over the past several decades, various empirical expressions, explicitly including particle-size distribution, organic matter content, and moisture content,have been established (Bryan, 1968; Webb and Mc-Gowan, 2009; Wagner, 2013).At the present time,these valuable empirical relations of soil erodibility are used, calibrated or extended worldwide (e.g., Borrelliet al., 2014; Piet al., 2017; Zhanget al., 2017).A major defect of non-process-based wind erosion models is that there exist many empirical parameters required to be determined locally.As pointed out by Webb and Strong (2011), representing soil erodibility dynamics should be regarded as a priority in the ongoing development of wind erosion models.
During wind erosion events, dust particles are emitted from soils through aerodynamic lifting, disaggregation and saltation bombardment (Shao, 2008;Koket al., 2012).The last one is a dominant mecha-nism in respect that most aeolian sand-size grains and aggregations move in saltation.For small particles,aerodynamic lifting is generally unimportant because the inter-particle cohesion is large compared with gravity and aerodynamic force, except a few special cases such as "free" particles (Shao and Klose, 2016;Zhanget al., 2016) or dust devils (Neakraseet al.,2016; Wang, 2016; Kurgansky, 2018).Threshold friction velocity, being an indispensable parameter in most wind erosion models, reflects the capacity of the soil surface to resist aerodynamic lifting.Threshold theories for loose and dry surfaces and the influence of soil moisture are continually developed (e.g., Shao and Lu, 2000; Mckenna Neuman, 2003; Bagnold,2005; Wang, 2006; Bolteet al., 2011).A recent wind tunnel experiment indicates that turbulence effects aerodynamic lifting(Liet al.,2020).The size distribution of emitted particles from dust aggregates or snow crystals owing to disaggregation can be well described by using the power law for brittle material fragmentations (Kok, 2011; Comolaet al., 2017).As one of four crucial sub-processes in the aeolian sediment transport (Anderson and Haff, 1991), sand-bed impact has been studied in detail (e.g., Zheng, 2009).For loose soils,the vertical dust flux generated by saltation bombardment can be successfully predicted by several schemes with respect to air density, friction velocity,threshold friction velocity,and constant coeあicients (Koket al., 2012).However, how to quantify binding strength of dust particles is a challenge(Shao,2008).In fact, the significance of the mechanical properties of bare or crusted soils in the breakdown of soil structures and dust emission has long been noted in studies of wind erosion (Chepil and Woodruff,1963; Smalley, 1970; Wilson, 1994; Riceet al., 1997;Zobecket al., 2003; Wanget al., 2006).They are characterized by rupture modulus (Richards, 1953),surface strength (Riceet al., 1997; Riceet al., 1999),or binding energy (Shaoet al., 1993).A mathematic model of sand grain impact and soil failure indicates that soil erodibility might depend on inter-particle cohesive forces (Smalley, 1970).Early laboratory experiments of water erosion reveals that soil detachment by rainfall is proportional to the ratio of the kinetic energy of raindrops to the shear strength of soils (Al-Durrah and Bradford, 1981; Al-Durrah and Bradford,1982; Nearing and Bradford, 1985;Torriet al., 1987).According to this result, the detachment function in the Texas Tech wind erosion analysis model is constructed by employing the method of dimensional analysis (Wilson, 1994).Another remarkable progress independently achieved by aeolian researchers is the abrasion law,i.e.,the mass removal of target materials per impact is proportional to the kinetic energy of the impacting particle (Greeleyet al., 1982; Anderson,1986; Hagen, 1991).This empirical law is theoretically improved after extracting a non-dimensional parameter containing the Young's modulus and yield stress of the target materials like rocks from a general model of collision and damage(Wanget al.,2011;Wang,2020).
Recently, a large number of direct shear tests for erodible soils have been performed (Li, 2015; Fanget al., 2018; Zhanget al., 2018) and the role of shear strength is still being explored(Zouet al.,2015;Sunamura, 2018).Tensile and shear strengths can be added to the standard spring-dashpot contact model while numerically modeling the saltation developing over cohesive surfaces by the discrete element method(Comola and Lehning 2017; Comolaet al., 2019).From the viewpoint of soil mechanics (Fredlund and Rahardjo, 1993; Terzaghiet al., 1996), the deformation,motion,and failure of topsoils are involved in the process of saltation bombardment, and these behaviors cannot be intuitively and uniquely described by one parameter.In our study, a simple analytical model for the erosion of a bare, smooth, dry, and uncrusted soil surface due to saltation bombardment is built upon the principle and method of classical mechanics.
2 Model
The soil behavior in response to external forces can be described by soil mechanics.Basic differential equations of mass conservation, momentum balance and constitutive relation are referred to Fredlund and Rahardjo (1993) and Terzaghiet al.(1996).An unsaturated soil will reduce to a perfectly elastic body if the terms with respect to pore-air and pore-water pressures in the constitutive relation are neglected.Such an oversimplification offers rough estimations of the quantities of displacement and stress to be predicted(Terzaghiet al., 1996).Different from previous work attempting to establish a general theoretical frame(Wanget al., 2011), here we focus on the special case of erosion caused by the normal impact between a saltating sand grain and a half-space comprised of the soil, as presented in Figure 1, in order to avoid complex numerical computations and meanwhile to capture the physical essence.It is also assumed that the rigid impactor itself rebounds from rather than beds in the topsoil.Given an arbitrary pointNin the soil, our purpose is to assess whether failure occurs or not.The meanings and symbols of the main variables and parameters are listed in Table 1.
The solution to the Boussinesq problem,which describes the elastic deformation of a homogeneous halfspace under a concentrated forcePnormal to its surface, has been obtained (Lurie and Belyaev, 2005;Popov, 2010).In the rectangular coordinate system in Figure 1,the stress field can be expressed by,

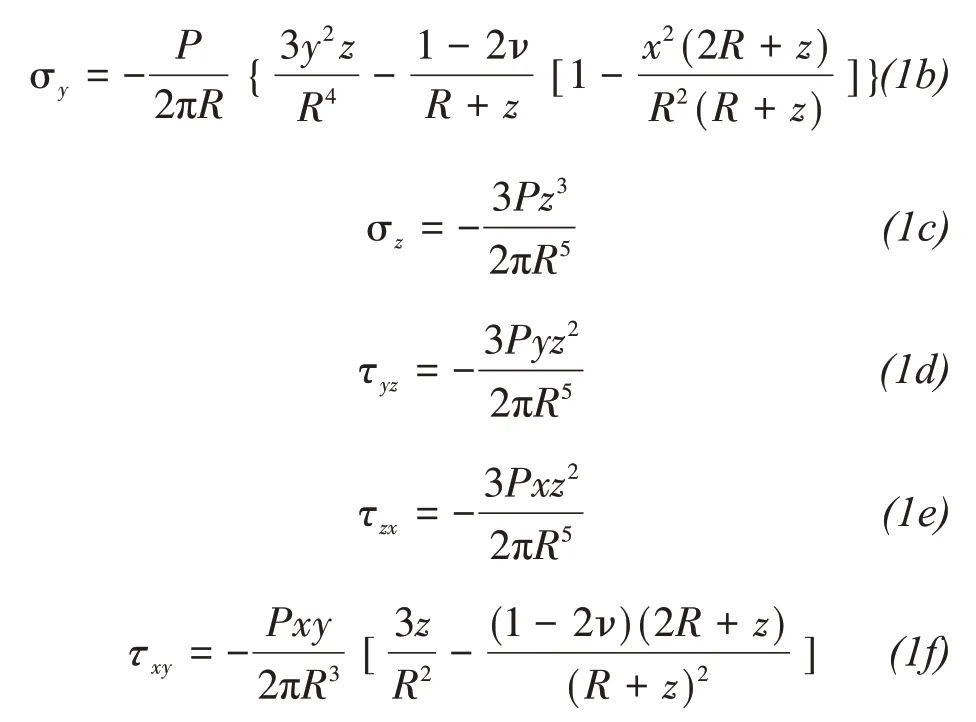
whereis the Poisson's ratio, σ and τ denote normal and shear stress,respectively.
It is assumed that the eroded volume has a semielliptical shape with the equatorial and polar radii ofaandb.Based on the expressions of stress components(1), three principal stresses at (a, 0, 0) are calculated,
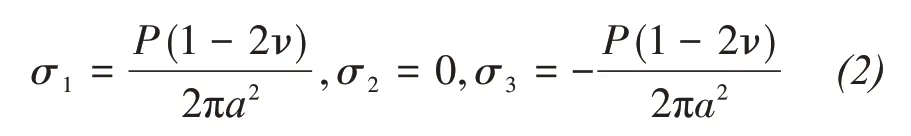
Different from the targets of rocky materials we modeled previously (Wanget al., 2011; Ninget al.,2019), the failure of soils is assessed by the Mohr-Coulomb failure criterion(Shield,1955),

wherecis cohesion,φis friction angle.
From Equations(2)and(3),we have,
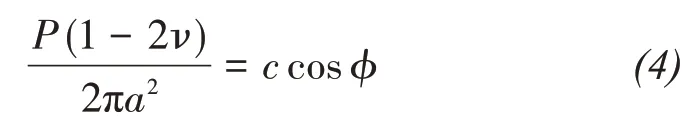
Similarly, the principal stresses at (0, 0,b) and the equation aboutbare,

and
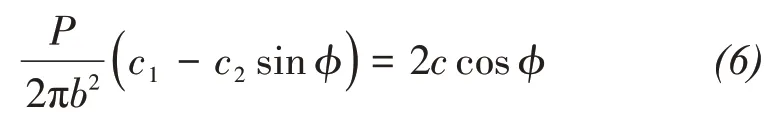
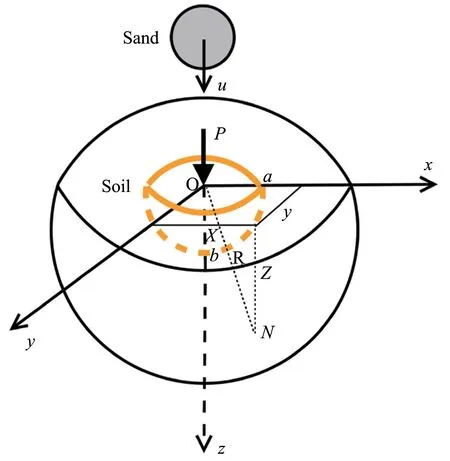
Figure 1 The normal impact between a saltating sand grain and a half-space comprised of the soil.u and P are the vertical speed of the saltating grain and the collision force acting upon the soil.Given an arbitrary point N under the coordinate of(x,y,z),the stress and displacement can be computed.The eroded volume is denoted by a semi-elliptical shape withthe equatorial and polar radii of a and b,respectively

Table 1 Meanings and symbols of the main variables and parameters.The international system of units is applied
There are three unknown quantitiesa,b,Pin Equations(4)and(6).A third relation must be found.The magnitude of time-averaged impact forcePis determined by the momentum theorem,
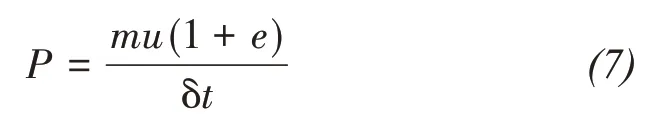
wherem,u,e, and δtare the mass and speed of the saltating grain, the coefficient of restitution, and impact duration, respectively.The Hertzian contact theory reveals that the coefficient of restitutionedepends upon the severity of the impact.For low-speed impacts we studied, a constanteis assumed because it slowly decreases with increasing the impact speed(Johnson,1985).
The impact duration is estimated as,

where thezcomponentwof displacement atz=bhas the form of(Popov,2010),
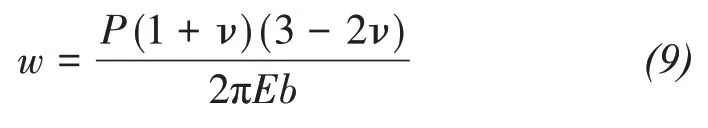
whereEis the Young's modulus.
Combining Equations(7)-(9),the third relation is,
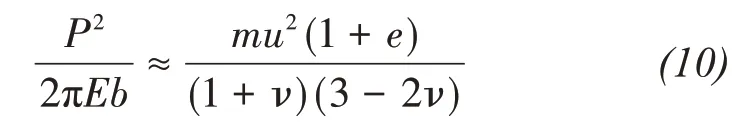
Consequently, the eroded volume per impact can be derived from Equations(4),(6), and(10).Since the physical processes related to wind erosion are often particle-size dependent (Shao, 2008), it is rescaled in terms of the diameter of the saltating grain,

whereVis the detached volume per impact,dandρsare the diameter and density of the saltating sand grain, and the mass expression ofm=ρsπd3/6 has been inserted, and the constantseand ν are implicatively contained in the proportionality coefficient.
Define the incoming mass flux densityqas the mass perpendicularly striking an unit surface area per unit time, the mass transported by wind through the cross sectional area of one grain in the duration ofTis

where the grain numbernis equivalent to impact times.
The length of"sand column"is,

in which a constant volume concentration of sand grains is assumed and will be implicitly contained in the proportionality coefficient of the final expression.
From Equations(12)and(13), the impact timesnand speeduare,

and

The change rate of surface heighth˙ due to soil loss is,

Substituting(11),(14)and(15)into(16),we have,
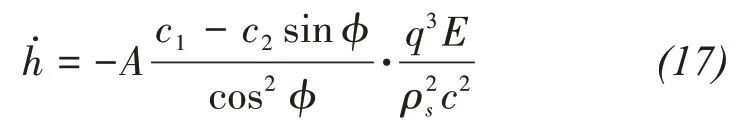
whereAis a positive constant needed to be determined experimentally.
3 Results and discussion
The concise derivation as given above leads to two new analytical detachment Formulas(11)and(17)suitable for the prediction of soil loss caused by individual and continuous impacts of saltating grains respectively.The method we used is similar to the dust production model which attempts to combine an empirical bombardment formula and a saltation model (Alfaro and Gomes, 2001).However, this so-called physically-based model focuses on the mathematic expression of particle size distribution rather than the mechanical processes we insist on investigating(Wanget al., 2011; Ninget al., 2019; Wang, 2020).Different from surface strength, binding energy and shear strength previously used or arbitrarily defined by some aeolian researchers (Shaoet al., 1993; Wilson, 1994; Riceet al., 1997; Riceet al., 1999), four common mechanical parameters in soil engineering are introduced together.Their meanings are clear and obvious.Poisson's ratio ν and Young's modulusEquantify the elastic property of soil.Cohesioncand friction angleφ, originating from the failure criterion,characterize the inter-particle normal stress and internal friction.All of them should be measured for different types of erodible soils.Unfortunately, they have not appeared in the "standard" methods for wind erosion research (Webbet al., 2015).Some values ofcandφcan be estimated from direct shear tests (Li,2015; Zhanget al., 2018).As far as we know, cohesion among them is frequently taken into account to determine the threshold friction velocity.Soil mois-ture has a strong impact on cohesion.Very recently,the effects of soil moisture on erodibilities of several bare soils are experimentally investigated (De Oroet al., 2019).As a macro-phenomenon, soil erodibility dynamics must obey Netwton's laws of motion.Our theoretical work is a small attempt towards the physically-based wind erosion model in light of the first principles.The effects of gravel cover, vegetation vibration, and unsteady airflows on erosion rate should be deduced in the manner of mechanics in the future.At the moment Equations(11)and(17)are not comparable with those empirical wind erosion models which seems more comprehensive (Jarrahet al., 2020).Therefore, the presented model has to be validated indirectly.
The wind tunnel experimental results (Bridgeset al., 2004) were successfully applied to estimate the abrasion coefficient in our previous studies of the abrasion rates of ventifacts (Ninget al., 2019).Fortunately, the abrasions of soil clods by saltating grains are measured in a recent wind tunnel experiment (Daiet al.,2020).Many dry and wet cylinder-and hexagonal prism-shaped clod specimens of four soil types are abraded and the abrasion masses during 300 s under five different saltation mass flux are obtained.We only re-examine their experimental data for dry specimens because water content always changes with time.The abrasion rate can be written as,

whereρbandSare the bulk density and abraded area of the target.For the targets constituted of an identical soil,from Equation(17),we get,

The absolute values of the Pearson correlation coefficients are all larger than 0.60 while fitting the experiment data using Equation(19).For the brown calcic soil,our theoretical model works very well,as presented in Figure 2 where the different values of the fitted coefficient are caused by the specimen shapes.
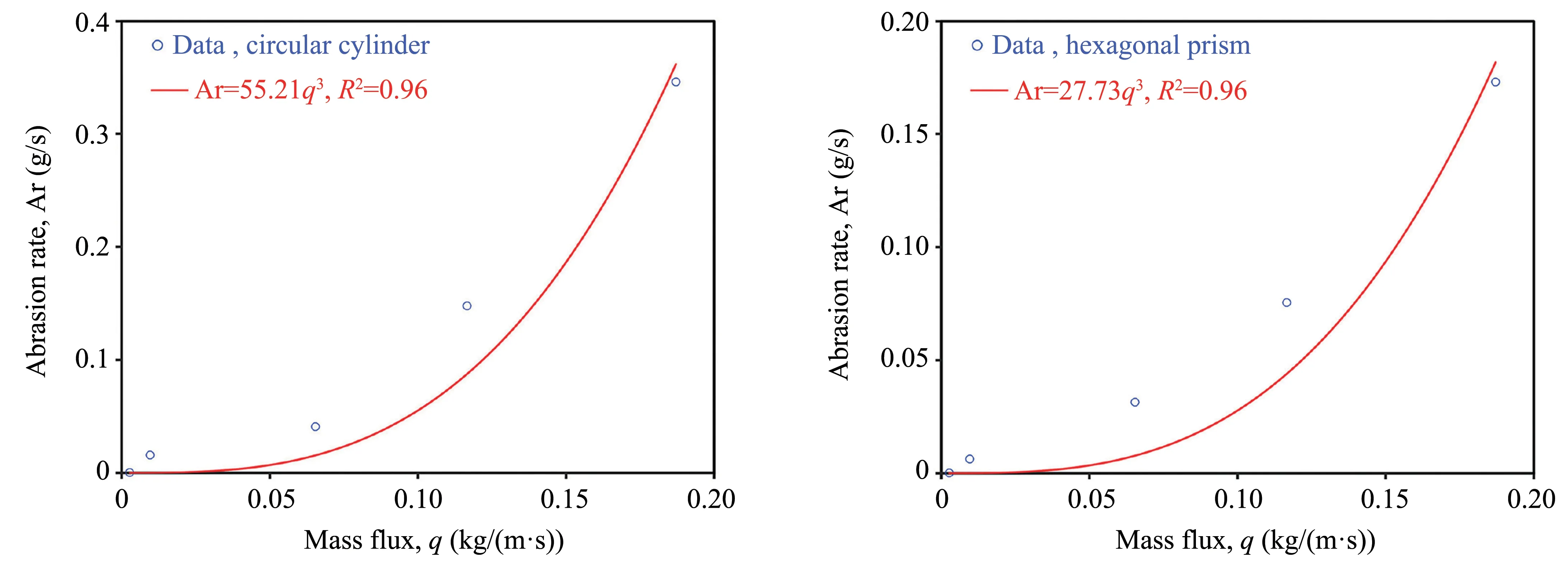
Figure 2 The abrasion rate of brown calcic soil is in direct proportion to the cube of mass flux.The wind tunnel experiment was performed by Dai et al.(2020)
It was once speculated that soil anti-erodibility factors might be closely associated with shear strength (Riceet al., 1997; Riceet al., 1999; Zouet al., 2015; Fanget al., 2018), but the theoretical interpretation is less convictive.The right-hand side of Equation(11)reflects the ratio of the kinetic energy of the impacting grain to the elastic potential energy of the soil per unit volume at the yield point.The latter,with the units of Pa,is,
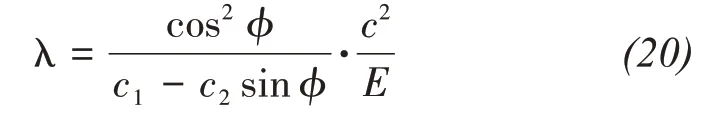
Given the incoming saltating grains,λprovides an evaluation of the anti-erodibilities of different soils.For the four typical erodible soils classified by the unified soil classification system (USCS),λvalues are listed in Table 2,while the other three mechanical parameters,i.e., cohesionc, Young's modulusEand friction angleφ,are sourced from geotechnical information available at http://www.geotechdata.info/parameter/parameter.html.The Poisson's ratio of soils normally ranges from 0.1 to 0.5.A constant value of ν=0.3 is assumed in the computation.The descending order of anti-erodibility (CL, ML, SM, and OL) is similar to that of cohesion.This implies that cohesion is more important than the other parameters.
In the future, delicate experiments should be designed and performed to directly examine the soil particle detachment model we developed here.The mechanical parameters of soils,saltation velocity,and detachment rate can be simultaneously measured in triaxial and wind tunnel experiments.The field measurements of the dust emission rates of differnent soil surfaces are also very helpful.Moreover, it is possible to improve the detachment rate formula based upon the more rigorous contact mechanics theories.

Table 2 Comparison of anti-erodibilities between several erodible soils.The unified soil classification system(USCS)is adopted.The mechanical parameters are sourced from the geotechnical information available at http://www.geotechdata.info/parameter/parameter.html.A constant Poisson's ratio of ν=0.3 is assumed
4 Conclusions
The analytical expressions for the detachment volume per impact and the detachment rate in saltation bombardment are theoretically derived from the principles of classical mechanics.Four common mechanical parameters of soils,i.e., Poisson's ratio,Young's modulus, cohesion and friction angle, are appropriately introduced into wind erosion.The novel expressions can roughly describe the measured dust emission rates over three different cohesive soil surfaces.The concept of anti-erodibility is quantificationally evaluated.It is expected that the presented work will improve the descriptions of the mechanical properties of soils in physically-based wind erosion models.
Acknowledgments:
ZhenTing Wang is grateful to JF Li for providing his Ph.D thesis.This research was supported by Natural Science Foundation of China projects (Nos.41971011 and 41630747).
杂志排行
Sciences in Cold and Arid Regions的其它文章
- Dynamic behavior of the Qinghai-Tibetan railway embankment in permafrost regions under trained-induced vertical loads
- Comparison of sampling schemes for spatial prediction of soil organic carbon in Northern China
- Assessing spatial and temporal variability in water consumption and the maintainability oasis maximum area in an oasis region of Northwestern China
- Validation of AIRS-Retrieved atmospheric temperature data over the Taklimakan Desert
- Variation characteristics of evaporation in the Gulang River Basin during 1959-2013
