热源井填砾抽灌同井流/热贯通及温度锋面运移数值模拟

宋 伟，刘远周，郑湍峰，刘晓秀



热源井填砾抽灌同井流/热贯通及温度锋面运移数值模拟

宋 伟，刘远周，郑湍峰，刘晓秀

（北方工业大学土木工程学院，北京 100144）

针对填砾抽灌同井流贯通、热贯通及温度锋面运移研究的不足，该文在已验证的数值模型中建立监测点、线、面和体对以上问题进行量化分析，并将热源井回水经过回填砾石直接流入抽水管的流量占总回水流量的百分比定义为流贯通强度。研究结果表明，填砾抽灌同井回水进入含水层后的流速可以用某一方向的分速度代替；该模型中的流贯通强度为1.2%，热贯通发生与完全形成的时间分别为模型运行2.5和12 min，抽水温度变化较剧烈的时刻与热贯通形成发展的时刻基本一致。在地下水渗流速度大于1×10-3m/s的区域，速度锋面运移较温度锋面运移快；反之，温度锋面运移快于速度锋面运移。通过对填砾抽灌同井流贯通、热贯通及温度锋面运移的量化分析，为进一步探索填砾抽灌同井最优运行策略和更高换热效率提供了理论基础。

井；温度；速度；填砾抽灌同井；流贯通强度；热贯通；温度锋面；数值模拟

0 引 言

填砾抽灌同井作为单井循环地下换热系统的一种形式[1]，热源井换热效率通常高于循环单井和抽灌同井[2]；由于热源井的结构特点，填砾抽灌同井较抽灌同井更容易发生流/热贯通。流贯通即回灌井水经过热源井内部或与含水层换热后被抽水管抽出；热贯通即回灌井水在热传导与热对流的作用下，其温度锋面运移至抽水区导致抽水温度不同程度的升高或降低。填砾抽灌同井回水经过回水管进入回水区，大部分回水通过渗流进入含水层，并在回水正压、抽水负压和重力的作用下，逐渐朝热源井径向和竖直方向渗流；少量回水在热源井内与回填砾石及热源井壁面换热后流入抽水管。回水向含水层渗流过程中，回水与含水层原水、固体骨架以热传导、热对流和热弥散的方式进行热量交换[3]。热传导发生在地下水与固体骨架、骨架与骨架之间[4]，在确定的水文地质条件下传热系数相对稳定；热对流和热弥散发生在含水层固体骨架孔隙之间，对流传热系数和热弥散系数受地下水流动状态的影响较大[5]。受回填砾石区的孔隙度较含水层的孔隙度大、抽/回水管的压差大以及抽/回水管的间隔短等因素的影响，热源井内较含水层更容易形成流贯通。流贯通引起抽水温度的变化，进而形成热贯通。流/热贯通使得热源井的换热效率及承担负荷的能力降低[6-8]，通常采用排放部分回水以缓减流/热贯通，但长期排放会引起地下水位下降、地面沉降与裂隙、地表植被破坏等问题[9-10]。因此，提高填砾抽灌同井换热效率，探明流/热贯通形成机理显得尤为重要。

随着计算流体力学的迅速发展，数值模拟已成为地源热泵领域科学研究的重要手段之一，并得到了广泛应用。如利用数值模拟对异井循环地下水源热泵进行研究，得出抽水流量是影响其抽水温度的关键[11-12]，减小抽水流量可以有效改善抽水温度[13]；地下水的自然流动可加快抽水井温度的恢复[14]，并增大热响应半径[15]；抽/灌井的合理布局可有效提升系统效率[16-17]。通过建立数学模型，得出循环单井的换热效率通常高于地埋管[18-19]，抽水温度与排放率有关且最佳排放率为12%~13%[20-21]；热传导和热对流是循环单井传热的主要方式[22]，并得出特定条件下其含水层内的温度场及速度场[23-24]，填砾抽灌同井温度和速度场以井呈轴对称分布等[25-26]。根据砂箱试验，获得抽灌同井连续运行的特性[27]，增加抽/回水间距可以有效提高抽灌同井换热效率[28]。目前关于异井循环地下水源热泵的热响应半径[29-30]、地下水渗流对地埋管的影响[31-32]以及含水层储能特性的研究较多[33-34]，而对填砾抽灌同井流/热贯通的研究尚显不足。

本文在已验证的数值模型基础上[35]，对填砾抽灌同井作用下的流/热贯通进行量化分析，进而揭示流/热贯通的形成机理及其影响因素。通过对温度锋面和速度锋面运移的量化分析，得出其运移的速度关系，为进一步提高填砾抽灌同井换热效率提供理论基础。

1 模型建立

1.1 几何模型建立

根据砂箱试验台的几何参数，利用SolidWorks软件1:1建模，图1为几何模型与监测单元的位置及分区。图1a给出了砂箱几何模型及监测点、线、面的位置，在面上，选取过热源井轴心的截面A（=0）上的监测点P1（62, 685, 0）和监测线L1（=235 mm），来研究含水层中点、线、面上速度的大小和方向；建立监测线L2（= 62 mm）和L3（=300 mm）探究含水层温度随时间的变化规律。热贯通初始时间，即抽水管内温度发生变化的时间，为此在热源井内，选取位于抽水管上顶板的监测面S1来观测填砾抽灌同井热贯通形成发展的时间。在含水层内选取正对回水管中心的监测线L4（=685 mm），通过建立监测点获得其温度与速度，以分析含水层温度锋面和速度锋面的运移特性。

如图1b所示，建立包含抽水管的监测体V1（=113 mm）和包含回水管的监测体V2（=113 mm），定量研究各方向汇集到抽水管和从回水管向各方向发散的流量，流过V1和V2的流量总和均与抽/回水流量相等。V1上、下底面为监测面S3、S2，V2上、下底面为监测面S5、S4，空间上分别对应抽/回水管上下壁面。对以上4个监测面进行区域划分，如图1c所示，并假设地下水流出热源井为正，流入热源井为负，向上流动为正，向下流动为负。

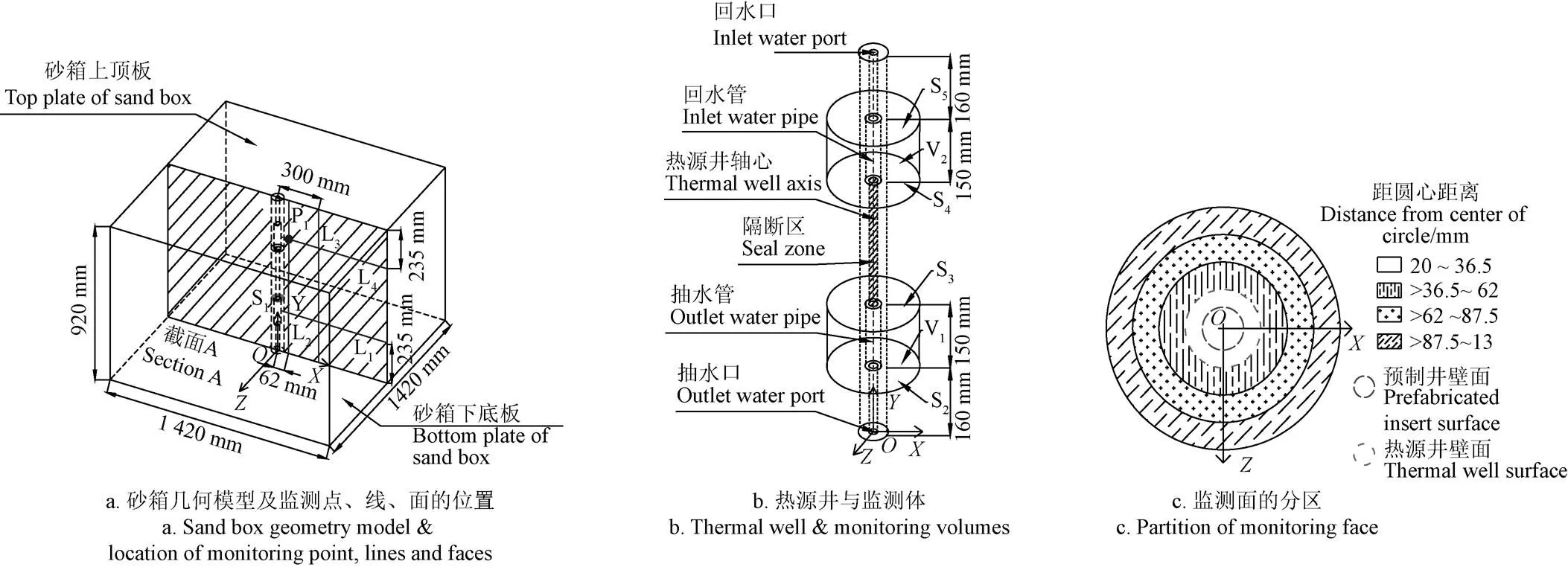
注：P1为监测点; L1~L4为监测线; S1~S5为监测面; V1, V2为监测体.

1.2 网格划分及模拟参数设置

利用ICEM-CFD软件对模型进行结构网格划分，并对局部网格加密。用Fluent14.5软件对所建模型进行模拟计算，砂箱边界条件设置为绝热，热源井内各边界根据实际材料进行设置。回填砾石区的孔隙度设置为0.35，含水层的孔隙度设置为0.25。其他参数如回水口压力，抽水口温度、压力，初始地温等根据实测数据进行设置，主要设置如表1所示。

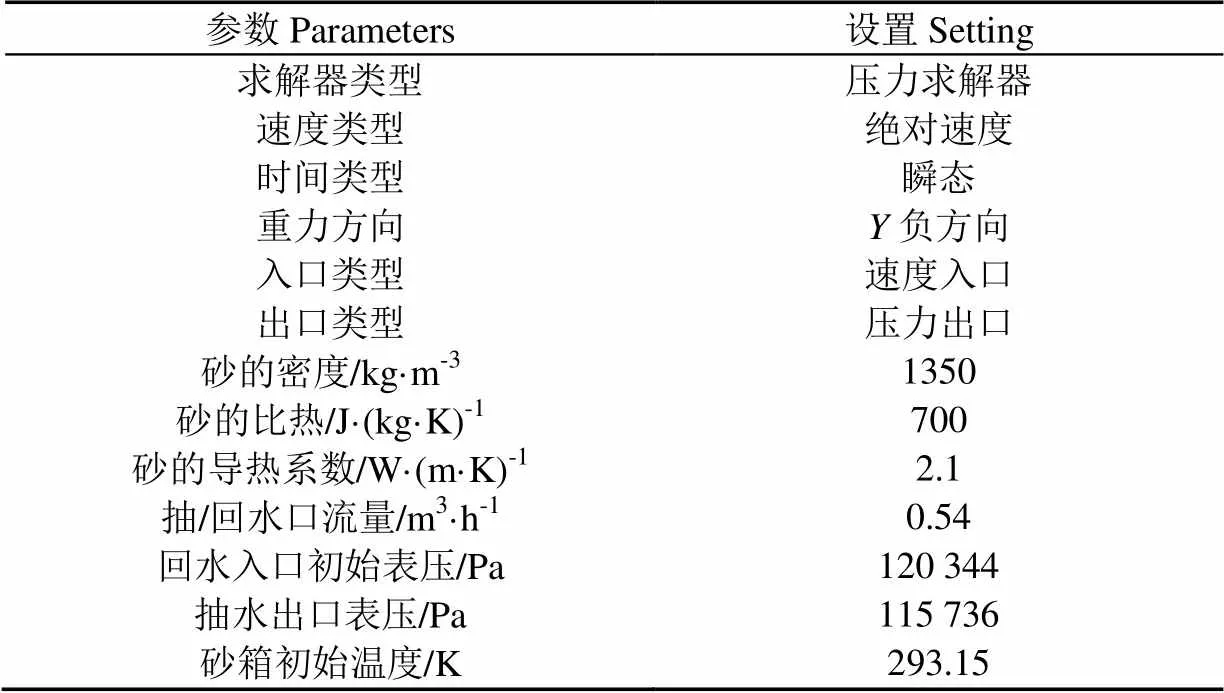
表1 模拟参数设置

2 主导速度与流贯通强度

2.1 监测点/线的主导速度

图2为监测点/线的合速度与3个方向分速度的关系。

注：vx、vy和vz分别为监测点/线X、Y和Z轴向的分速度，m·s-1；v为监测点/线的合速度，m·s-1。

由图2a可知，随着模拟的进行，监测点P1在轴方向的分速度v与该点合速度数值大小基本相等，的平均值为1.8×10-3m/s。v和v与存在数量级上的差异，v为−3.96×10-6m/s，v为5.98×10-5m/s，可见v和v对的影响极小。因此，用v代替该点的合速度，即v为该点的主导速度。图2b为监测线L1的速度随（距热源井轴心距离）的变换规律，该线的主导速度方向为的负方向，且各位置的主导速度与合速度基本重合。在实际工程中，填砾抽灌同井影响下的地下水流动复杂多变，流动方向难以确定。为此，该文就监测点/线3个方向的分速度与其合速度进行对比，由于各方向速度存在数量级的差异，监测点/线的速度可用某一方向分速度代替。

2.2 监测面的速度分布

图3a为截面A右半区域的主导速度分布图，主导速度为和（井深）方向的面积在整个监测面上基本各占一半，而主导速度为正方向只有D1和D2区域。部分回水朝砂箱上顶板流动形成D1区域；砂箱底部的地下水逐渐向抽水管汇集形成D2区域。无论主导速度是方向还是方向，它们都以井中心线呈轴对称分布。因主导速度方向发生改变形成交界线L5和L6，在交界线两侧存在过渡区。过渡区内的速度由“上一方向”的主导速度逐渐向“下一个方向”的主导速度转变，在转变的过程中速度由2个方向的分速度共同决定，但趋向于主导速度的方向。

从图3b、3c速度矢量图可知，热源井内渗流速度远大于含水层的渗流速度，这主要是热源井内回填砾石区的孔隙度大于含水层的孔隙度所致。在热源井内，以回水管为中心，回水经过回填砾石区向上/下流动时速度方向与成一定夹角，驱使热源井中的回水不断向含水层流动。在整个监测面上，地下水流动速度矢量图呈“椭圆型”分布。井中心线以上为回水影响区域，回水以回水管为中心向外发散；井中心线以下为抽水影响区域，地下水逐渐向抽水管汇集。

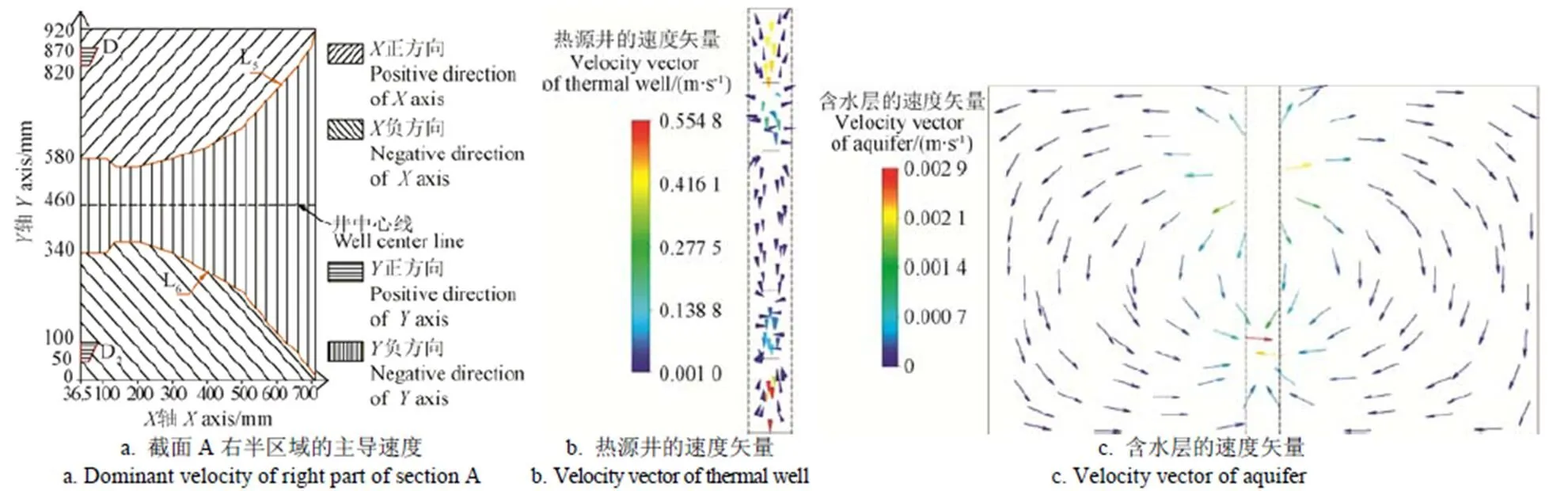
注：D1和D2为主导速度方向为Y正方向的区域；L5和L6为主导速度方向改变而形成的交界线。

2.3 流贯通强度

图4为监测体V1、V2上的监测面及热源井的流量分配情况。图4a显示了不同监测面上各监测区域流量的百分比。通过监测面S2的各区域向抽水管汇集的流量2占抽水流量的39.4%，流过监测面S3向抽水管汇集的总流量3占抽水流量的43.9%。通过V1上下监测面向抽水管汇集的流量占抽水流量的83.3%，从监测体侧面流入抽水管的流量仅占16.7%，可见抽水流量主要来自抽水管上下壁面（=113 mm）以内。V2的各监测面的流量分布与V1相同，回水通过回水管上下壁面（=113 mm）向外发散的流量为83.3%。

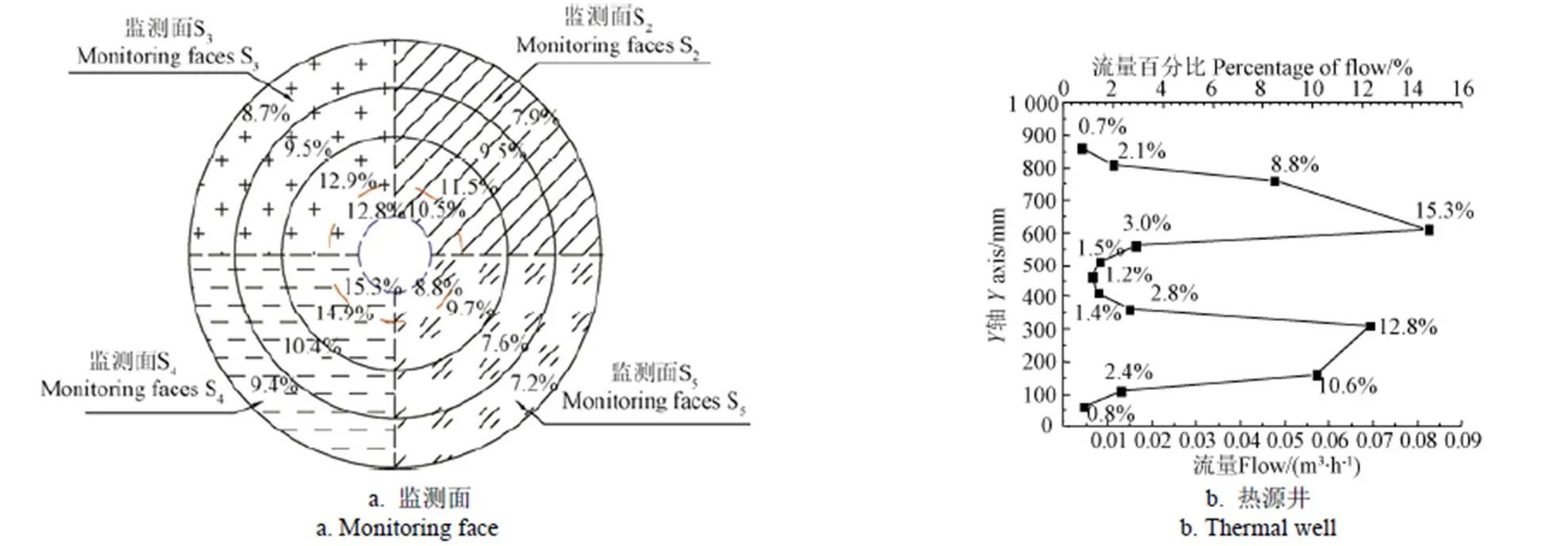
注: 监测面上的数据为流过该区域的流量占监测体流量百分比。

从图4a还可以看出，在同一监测体上，隔断区近侧监测面（S3、S4）所占流量百分比较远侧监测面（S2、S5）大，即3＞2，4＞5。所有监测面中流过S4的流量最大，通过模拟监测得出流经监测面S4各区域（从内到外）单位面积流量依次为28.2、10.2、4.7、3.2 m³/h。由于回灌过程中回水流速较大，回水迅速到达回水管底部，但不能及时流出，只能向上运动通过S5向外渗流，导致2＞5。这种向上流动的过程中出现水头损失，而流过S2的地下水没有这部分水头损失，所以导致流过S5的流量小，两者差值为0.032 94 m³/h。

从图4b热源井内不同井深处的流量占总流量的百分比可以看出，在热源井内，回水流向井中心位置的过程中，热源井内的流量逐渐减小，回水逐渐向含水层流出。从井中心位置到抽水管的过程中，热源井内的流量逐渐增加，说明含水层内的地下水逐渐向热源井内汇集，最终进入抽水管。回水经过热源井回填砾石区直接流入抽水管的流量即为热源井内井中心位置处的流量，因此定义流贯通强度为热源井回水经过回填砾石区直接流入抽水管的流量占总回水流量的百分比。该模型中流过热源井中心位置的流量为0.006 5 m³/h，流贯通强度为1.2%。

3 热贯通与温度/速度锋面运移

3.1 监测线温度随时间的变化

图5给出了监测线温度随时间的变化情况，从图5a监测线L2的温度随时间的变化关系可知，当模拟运行2 min时，正对回水管处的原水已被低温回水占据，温度降至13.5 ℃，基本与回水温度相等。在模拟运行过程中监测线上的温度以正对回水管处为中心向上/下逐渐降低，可明显看出砂箱上顶板阻碍了温度场向上的发展，限制了热源井换热的区域。

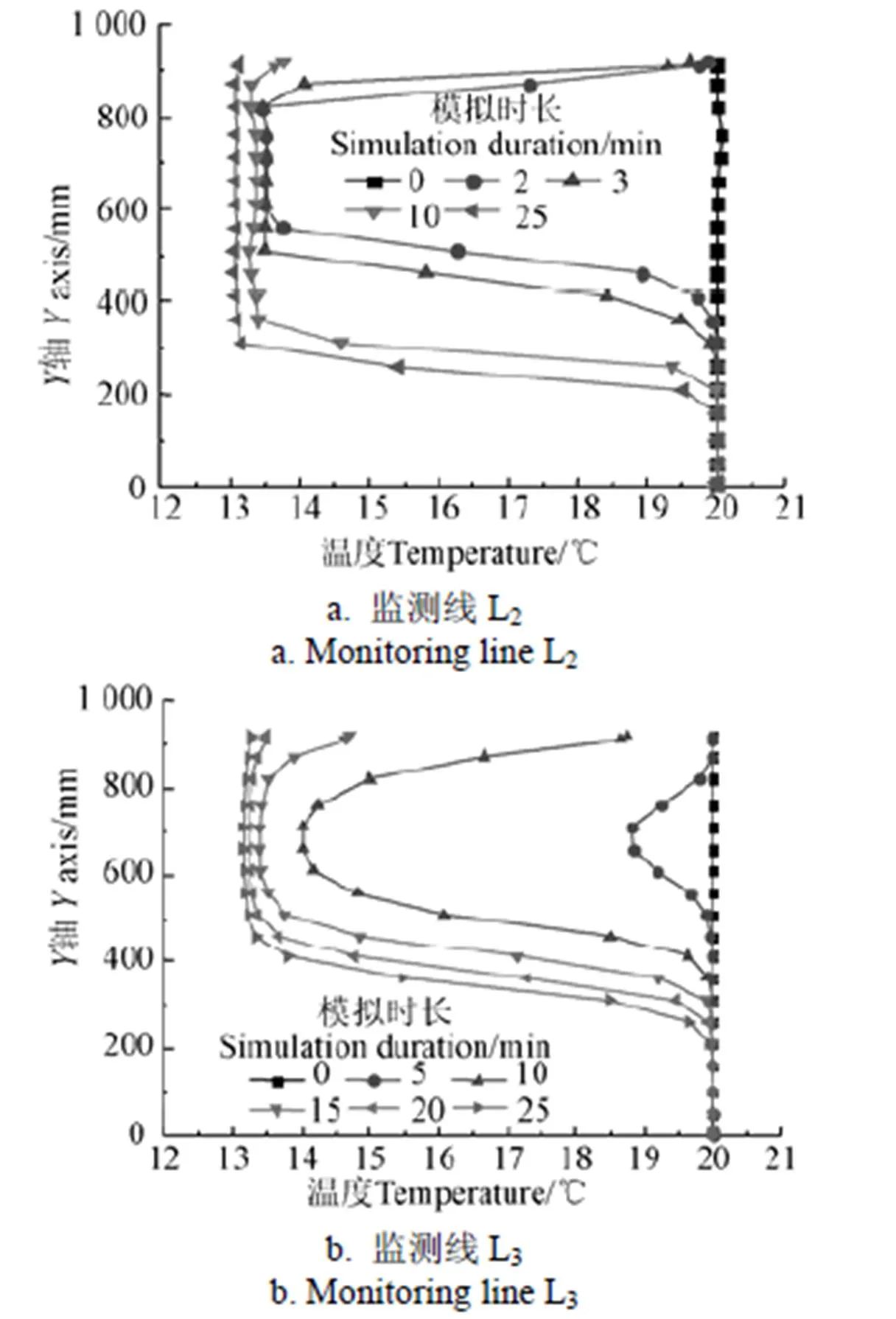
图5 监测线温度随时间的变化

图5b给出了监测线L3的温度随时间的变化情况。当模拟运行5 min时，正对回水管中心位置的温度降低较快；当模拟运行10 min时，L3上温度变化较快的位置同样正对回水管中心，说明在含水层内其他参数相同的情况下，正对回水管中心处温度变化较快。监测线L3上的温度变化幅度在前10 min大于后15 min，这是因为低温回水渗入含水层的速度比较缓慢，前10 min回水与含水层进行热交换的区域较小，致使热交换区域内的温度变化剧烈；10 min后随着热交换区域扩大，含水层温度变化逐渐减缓。

从图5可知，模拟运行25 min之内，砂箱下底板未受到低温回水的影响，受影响的区域仅在距离砂箱下底板200 mm左右的位置。可见，填砾抽灌同井换热的区域主要集中在抽水管以上，因此在实际工程中应适当增大抽/回水管间距以及回水管与隔水顶板之间的距离，从而增加热交换区域，提高填砾抽灌同井承担负荷的能力。

3.2 热贯通与抽水温度的关系

图6为热贯通与抽水温度的变化关系，模拟初始2.5 min监测面S1的温度保持为砂箱的初始温度，2.5 min后S1的温度急剧降低，热贯通开始发生；初始5 min S1的温度从20 ℃降至14 ℃。模拟运行7.5 min后S1的温度下降趋势变缓，并在12 min时稳定在回水温度左右，此时热贯通完全形成。

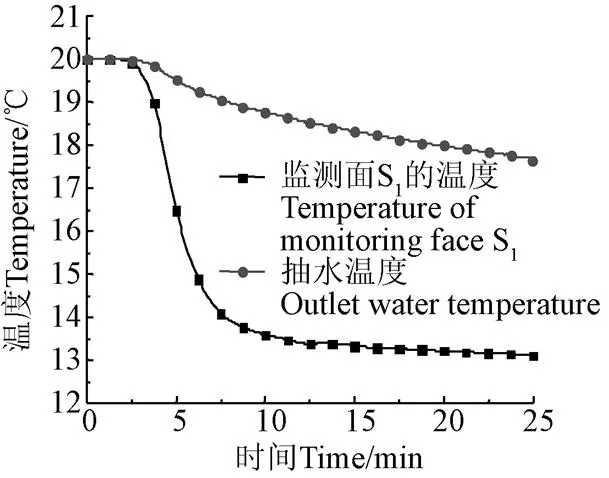
图6 热贯通与抽水温度的变化关系

监测面S1与抽水口相距310 mm，致使热贯通的发生早于抽水温度降低。但对比抽水温度和S1的温度在整个模拟期间随时间变化关系，可认为两者温度开始下降的时间基本一致；热贯通形成发展的时间段恰好是抽水温度变化相对剧烈的时间。可见热贯通对填砾抽灌同井的抽水温度造成直接影响。

3.3 温度/速度锋面运移

图7给出了速度和温度锋面的运移情况。通过对监测线L2与L3温度变化特性发现，在含水层内的同一坐标下，正对回水管中心处的温度最低，并且温度降低的速率也较快。因此选取该处监测线L4来研究温度锋面的运移，定义监测线上各位置的温度从初始温度降低0.01℃所用时间即为温度锋面运移到该位置的时间。通过模拟得出本模型下温度锋面以井为中心向外运移到各位置的所需时间，如图7a所示。

由图7a可知，地下水渗流速度随着远离热源井逐渐降低，且存在数量级差异。距热源井轴心100 mm内的渗流速度变化较快，流速量级为10-3m/s；100到300 mm之间的渗流速度变化较缓，数量级为10-4m/s；300 mm之外，流速量级减小为10-5m/s。拟合得出监测线L4上地下水的渗流速度与的关系，即速度锋面运移到各位置时的速度。

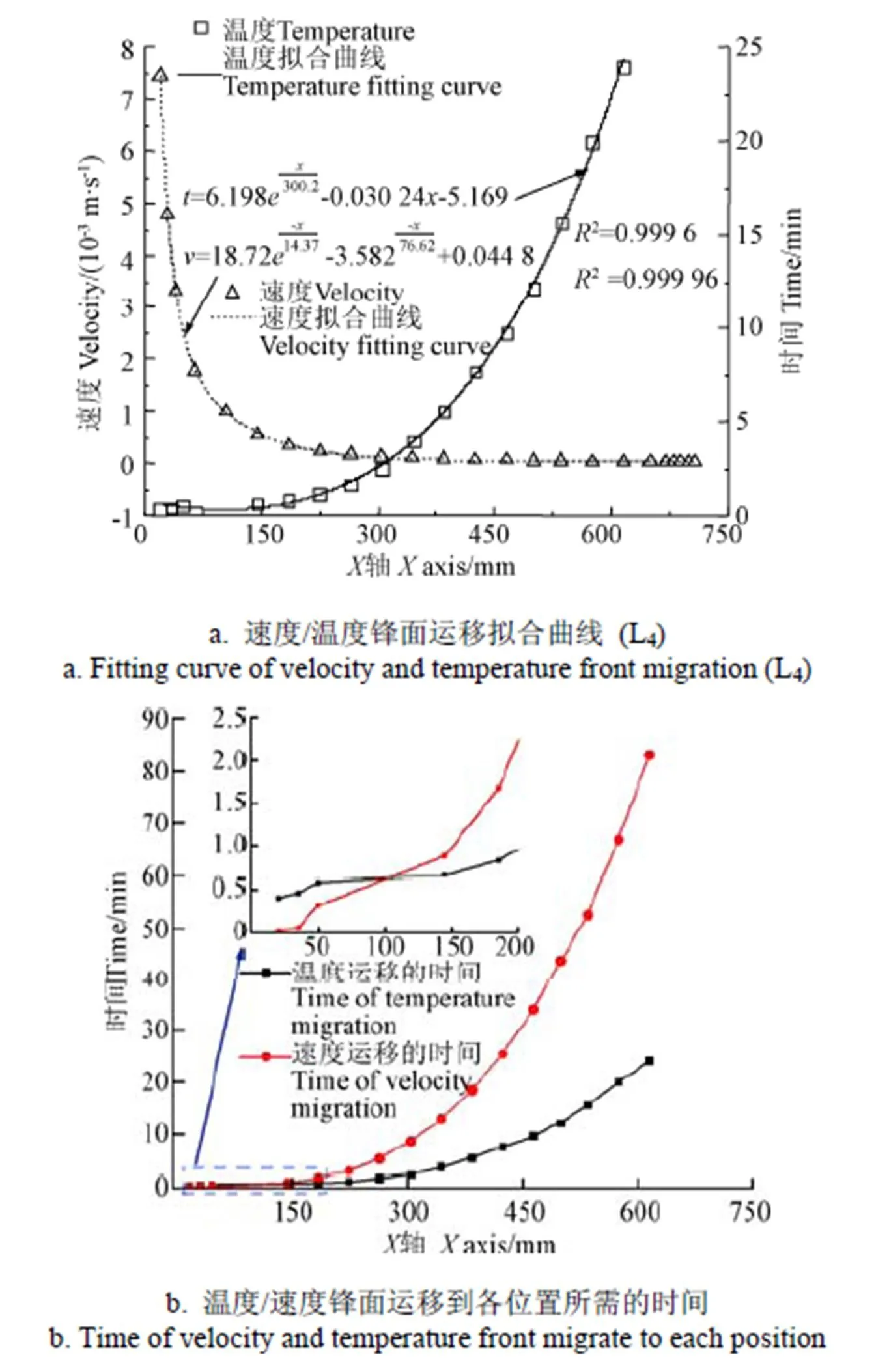
图7 速度和温度锋面的运移

通过温度与速度锋面运移的关系式，计算得出温度与速度锋面运移到各坐标位置所需的时间。由图7可知，距热源井轴心100 mm内，即地下水渗流速度大于1×10-3m/s的区域，速度锋面的运移速度较温度锋面运移速度快；而在100 mm之外，渗流速度小于1×10-3m/s的区域，运移同等距离下温度锋面所需的时间较速度锋面所需的时间短；距热源井轴心越远，地下水的渗流速度越低，运移同等距离，速度锋面所需时间会越长。

4 结 论

该文利用已验证数值模型，在模型中设置监测点、线、面和体分析了填砾抽灌同井的流贯通强度、热贯通形成发展的时间、温度锋面与速度锋面运移的快慢，得到如下结论：

1）在填砾抽灌同井中，确定的点、线和面的速度均可用主导速度代替；地下水在含水层内流动形成的速度矢量图以热源井为中心呈“椭圆型”分布。该模型下的流贯通强度为1.2%，这部分回水从热源井内直接流入抽水管，未与含水层原水和固体骨架进行热交换，它将对填砾抽灌同井抽水温度造成直接影响；

2）热贯通发生与完全形成的时间分别为模型运行2.5和12 min。抽水温度变化相对剧烈的时间与热贯通形成发展的时间基本一致，可见热贯通直接影响填砾抽灌同井的抽水温度；

3）在距热源井轴心100 mm之内，即地下水渗流速度大于1×10-3m/s的区域，速度锋面运移较温度锋面运移快；而在距热源井轴心100 mm之外，渗流速度小于1×10-3m/s的区域，温度锋面运移快于速度锋面运移。

[1] 倪龙，姜益强，姚杨，等. 单井循环地下换热系统健康运行的研究[J]. 流体机械，2009，37(4)：64－68.

Ni Long, Jiang Yiqiang, Yao Yang, et al. Study on healthy operation for standing column well ground heat exchange system[J]. Fluid Machinery, 2009, 37(4): 64－68. (in Chinese with English abstract)

[2] 宋伟，倪龙，姚杨. 单井循环系统在不同初始地温下的特性[J]. 哈尔滨工程大学学报，2014，35(3)：342－346.

Song Wei, Ni Long, Yao Yang. Characteristics of single well systems in different initial aquifer temperatures[J]. Journal of Harbin Engineering University, 2014, 35(3): 342－346. (in Chinese with English abstract)

[3] 倪龙，马最良，孙丽颖. 同井回灌地下水源热泵热力特性分析[J]. 哈尔滨工程大学学报，2006，27(2)：195－199.

Ni Long, Ma Zuiliang, Sun Liying. Thermal characteristics of groundwater heat pump with pumping and recharging in the same well[J]. Journal of Harbin Engineering University, 2006, 27(2): 195－199. (in Chinese with English abstract)

[4] 孙海洲，武强，徐生恒，等. Stokes-Darcy流耦合的单井循环换热地能采集井热物性研究[J]. 太阳能学报，2015，36(11)：2571－2577.

Sun Haizhou, Wu Qiang, Xu Shengheng, et al. Research on thermophysical characteristics with SWGECCHE coupled by Stokes-Darcy flow[J]. Acta Energiae Solaris Sinica, 2015, 36(11): 2571－2577. (in Chinese with English abstract)

[5] 倪龙，马最良. 热弥散对同井回灌地下水源热泵的影响[J].建筑热能通风空调，2005，24(4)：7－10.

Ni Long, Ma Zuiliang. The effect of heat dispersion on groundwater heat pump with pumping &recharging in the same well[J]. Building Energy & Environment, 2005, 24(4): 7－10. (in Chinese with English abstract)

[6] Rees S J, SpitlerJ D, Deng Z, et al. A study of geothermal heat pump and standing column well performance[J]. ASHRAE Transactions, 2004, 110(1): 3－13.

[7] Deng Z, Rees S J, Spitler J D. A model for annual simulation of standing column well ground heat exchangers[J]. HVAC&R Research, 2005,11(4): 637－655.

[8] Song Wei, Ni Long, Yao Yang. Experimental research on bleeding performance of single well ground-water heat pump[J]. Procedia Engineering, 2017, 205: 3162－3169.

[9] Du Xinqiang, Wang Zijia, Ye Xueyan. Potential clogging and dissolution effects during artificial recharge of groundwater using potable water[J]. Water Resources Management, 2013, 27(10): 3573－3583.

[10] Akhmad Azis, Hamzah Yusuf, Zulfiyah Faisal, et al. Water turbidity impact on discharge decrease of groundwater recharge in recharge reservoir[J]. Procedia Engineering, 2015, 125: 199－206.

[11] 胡继华，张延军，于子望，等. 水源热泵系统中地下水流贯通及其对温度场的影响[J]. 吉林大学学报：地球科学版，2008，38(6)：992－998.

Hu Jihua, Zhang Yanjun, Yu Ziwang, et al. Groundwater flow transfixion of groundwater source heat pump system and its influence on temperature field[J]. Journal of Jilin University: Earth Science Edition, 2008, 38(6): 992－998.

[12] 杜丹艳，胡继华，张延军. 水源热泵系统含水层中渗流场模拟及其对温度场的影响分析[J]. 建筑节能，2008，36(8)：8－12.

Du Danyan, Hu Jihua, Zhang Yanjun. Numerical simulation of seepage flow field of the groundwater source heat pump system and its influence on the temperature field[J]. Building Energy Efficiency, 2008, 36(8): 8－12.

[13] 骆祖江，李伟，王琰，等. 地下水源热泵系统热平衡模拟三维数值模型[J]. 农业工程学报，2014，30(2)：198－204.

Luo Zujiang, Li Wei, Wang Yan, et al. Three-dimensional numerical model for heat balance simulation of ground-water heat pump[J]. Transactions of the Chinese Society of Agricultural Engineering (Transactions of the CSAE), 2014, 30(2): 198－204. (in Chinese with English abstract)

[14] 张远东，魏加华，王光谦. 区域流场对含水层采能区地温场的影响[J]. 清华大学学报：自然科学版，2006，46(9)：1518－1521.

Zhang Yuandong, Wei Jiahua, Wang Guangqian. Impact of regional groundwater flow on geological temperature field with energy abstraction from the aquifer[J]. Journal of Tsinghua University:Science and technology, 2006, 46(9): 1518－1521. (in Chinese with English abstract)

[15] 张远东，魏加华，李宇，等. 地下水源热泵采能的水-热耦合数值模拟[J]. 天津大学学报，2006，39(8)：907－912.

Zhang Yuandong, Wei Jiahua, Li Yu, et al. Simulation of changes in geo-temperature field due to energy abstraction from underground aquifers[J]. Journal of Tianjin University, 2006, 39(8): 907－912. (in Chinese with English abstract)

[16] 刘立才，王理许，丁跃元，等. 水源热泵抽灌井布局及其运行过程中地下温度变化[J]. 水文地质工程地质，2007，34(6)：1－5.

Liu Licai, Wang Lixu, Ding Yueyuan, et al. Pumping-injection well distribution of groundwater heat pumps and temperature change in its operation process[J]. Hydrogeology & Engineering Geology, 2007, 34(6): 1－5. (in Chinese with English abstract)

[17] 王慧玲，王峰，孙保卫，等. 地源热泵系统抽灌模式对地下水流场和温度场的影响[J]. 水文地质工程地质，2009，36(5)：133－137.

Wang Huiling, Wang Feng, Sun Baowei, et al. Impact of position and production of injection wells on groundwater flow and temperature field with groundwater source heat pump[J]. Hydrogeology & Engineering Geology, 2009, 36(5): 133－137. (in Chinese with English abstract)

[18] Bobo Mingsum Ng, Chris Underwood, Sara Walker. Numerical modelling of multiple standing column wells for heating and cooling buildings[C]//The 11th International IBPSA Conference, Glasgow, Scotland, 2009: 49－55.

[19] Bobo Mingsum Ng, Chris Underwood, Sara Walker. Standing column wells—Modeling the potential for applications in geothermal heating and cooling[J]. HVAC&R Research, 2011, 17(6): 1089－1100.

[20] Al-Sarkhi A, Abu-Nada E, Nijmeh S, et al. Performance evaluation of standing column well for potential application of ground source heat pump in Jordan[J]. Energy Conversion and Management, 2008, 49(4): 863–872.

[21] Abu-Nada E, Akash B, Al-Hinti I, et al. Modeling of a geothermal standing column well[J]. International Journal of Energy Research, 2010, 32(4): 306－317.

[22] 李旻，刁乃仁，方肇洪. 单井回灌地源热泵地下传热数值模型研究[J]. 太阳能学报，2007，28(12)：1394－1401.

Li Min, Diao Nairen, Fang Zhaohong. Study on numerical heat transfer models of standing column well[J]. Acta Energiae Solaris Sinica, 2007, 28(12): 1394－1401. (in Chinese with English abstract)

[23] 倪龙，余延顺，姜益强，等. 循环单井地下水多流态流动特性[J]. 南京理工大学学报：自然科学版，2010，34(3)：367－371.

Ni Long, Yu Yanshun, Jiang Yiqiang, et al. Characteristics of groundwater multi-pattern flow in standing column well[J]. Journal of Nanjing University of Science and Technology: Nurture Science, 2010, 34(3): 367－371. (in Chinese with English abstract)

[24] Ni Long, Li Haorong, Jiang Yiqiang, et al. A model of groundwater seepage and heat transfer for single-well ground source heat pump systems[J]. Applied Thermal Engineering, 2011, 31(14): 2622－2630.

[25] 武强，孙海洲，徐生恒，等. 基于单井循环浅层地热能开发的数值模拟与分析[J]. 工程勘察，2015，43(1)：45－50.

Wu Qiang, Sun Haizhou, Xu Shengheng, et al. Numerical simulation and result analysis on the development of shallow geothermal energy resources based on single well heat circulation[J]. Geotechnical Investigation & Surveying, 2015, 43(1): 45－50. (in Chinese with English abstract)

[26] 武强，孙海洲，徐生恒，等. 多流场耦合的单井循环换热地能采集井物理场分析[J]. 太阳能学报，2015，36(12)：3015－3022.

Wu Qiang, Sun Haizhou, Xu Shengheng, et al. Analysis of physical fields on SWGECCHE coupled with multi-flow field[J]. Acta Energiae Solaris Sinica, 2015, 36(12): 3015－3022. (in Chinese with English abstract)

[27] 宋伟，倪龙，姚杨. 不同抽回间距的单井循环地下水源热泵系统试验[J]. 农业工程学报，2014，30(2)：205－211.

Song Wei, Ni Long, Yao Yang. Experiment on single well groundwater heat pump systems in different distances between pumping and injection screens[J]. Transactions of the Chinese Society of Agricultural Engineering(Transactions of the CSAE), 2014, 30(2): 205－211. (in Chinese with English abstract)

[28] 宋伟，倪龙，姚杨. 热源井抽灌同井连续取/放热特性试验[J].农业工程学报，2017，33(11)：248－253.

Song Wei, Ni Long, Yao Yang. Experiment on characteristics of continuous heat absorption/release for pumping and recharging in same thermal well[J]. Transactions of the Chinese Society of Agricultural Engineering(Transactions of the CSAE), 2017, 33(11): 248－253. (in Chinese with English abstract)

[29] Viola Somogyi, Viktor Sebestyén, Endre Domokos, et al. Thermal impact assessment with hydrodynamics and transport modeling[J]. Energy Conversion and Management, 2015, 104(10): 127－134.

[30] Zhou Yanzhang, Zhou Zhifang. Simulation of thermal transport in aquifer: A GWHP system in Chengdu, China[J]. Journal of Hydrodynamics, 2009, 21(5): 647－657.

[31] Antonio Capozza, Michele De Carli, Angelo Zarrella. Investigations on the influence of aquifers on the ground temperature in ground-source heat pump operation[J]. Applied Energy, 2013, 107: 350－363.

[32] Zhang Wenke, Yang Hongxing, Guo Xiaoqiang, et al. Investigation on groundwater velocity based on the finite line heat source seepage model[J]. International Journal of Heat and Mass Transfer, 2016, 99: 391－401.

[33] Kastner O, Norden B, Klapperer S, et al. Thermal solar energy storage in Jurassic aquifers in Northeastern Germany: A simulation study[J]. Renewable Energy, 2017, 104: 290－306.

[34] Anibas C, Kukral J, Possemiers M, et al. Assessment of seasonal aquifer thermal energy storage as a groundwater ecosystem service for the Brussels-Capital Region: Combining groundwater flow, and heat and reactive transport modeling[J]. Energy Procedia, 2016, 97: 179－185.

[35] 宋伟，朱柯，刘远周. 基于CFD的填砾抽灌同井数值模拟[J].可再生能源，2018，36(4)：626－632.

Song Wei, Zhu Ke, Liu Yuanzhou. Numerical simulation of forced external circulation standing column well based on CFD[J]. Renewable Energy Resources, 2018, 36(4): 626－632. (in Chinese with English abstract)

Numerical simulation on flow/thermal transfixion and temperature front migration of thermal well of pumping & recharging well filled with gravel

Song Wei, Liu Yuanzhou, Zheng Tuanfeng, Liu Xiaoxiu

(100144,)

The system of pumping & recharging well filled with gravel (PRWFG) is a specific type of the single thermal well used as ground heat exchanger. The heat efficiency of PRWFG is higher than that of the standing column well (SCW) or the traditional pumping & recharging well (PRW). The inlet water of the PRWFG converges and seeps toward the thermal well both horizontally and longitudinally as combined impacts of the positive recharging pressure, the negative pumping pressure and the gravity. During the procedure of seepage, the heat transfers between the inlet water and the water/solids in the aquifer via conduction, convection and thermal dispersion. The amount of heat transferred directly affects the efficiency and the capacity of the thermal well. Due to the low velocity of underground water, heat conduction occurs between the fluid and the solids, as well as between the solids. The heat transfer coefficient is relatively stable if there are certain hydrogeological conditions. Heat convection and thermal dispersion occur in the pores among the solids and the heat transfer coefficients depend on the flow rate of groundwater. A small amount of inlet water enters the outlet water pipe after heat exchanged with the gravel zone and the wall of the thermal well. Considering the facts that normally the porosity of the gravel zone is larger than that of the aquifer, the pressure difference between inlet and outlet water pipes is high and the distance between them is short, flow transfixion is more commonly happened in the thermal well than in the aquifer. Flow transfixion is a process that the inlet water in the thermal well enters the outlet water pipe through the gravel zone and the zone near the wellbore. The flow transfixion leads to a thermal transfixion by mixing water at different temperatures and mixed flow moves to the outlet water pipe. The formation of flow/thermal transfixion reduces the heat transfer efficiency and load-carrying capacity of thermal well. Currently, the bleeding strategy is usually used for decreasing flow/thermal transfixion. With the rapid development of computational fluid dynamics, numerical simulation is widely applied to the field of ground source heat pump. However, lots of researches focus on the aspects such as the thermal response radius of the pumping and recharging wells, the effect of groundwater seepage on the ground heat exchangers, and the characteristic of aquifer thermal energy storage. Considering the fact that there is insufficient research to predict the flow/thermal transfixion and the temperature migration of PRWFG, this paper provides a fundamental study in order to better understand the operation mechanism. The dynamic characteristics of PRWFG are quantitatively studied by establishing observation point, lines, surfaces and volumes based on the validated numerical model. The strength of flow transfixion is defined as the portion of the inlet water flow from the thermal well directly enters the outlet-water pipe through the gravel zone. Results show that the resultant velocity of PRWFG inlet water which flows back to the aquifer can be replaced by the component velocity in a specific direction. In this model, the strength of flow transfixion strength is 1.2 %. The time for the thermal transfixion to start and completely developed is 2.5 and 12 minutes respectively for the model running. It is also observed that during the same period when there are severe variations of the outlet water temperature, the thermal transfixion occurs and develops. In the area within 100 mm from the axis of thermal well, where the seepage velocity of groundwater is higher than 1×10-3m/s, the velocity front migration is faster than the temperature front migration. On the contrary, the temperature front migration is greater than the velocity front migration. The quantitative analysis of flow transfixion, thermal transfixion and temperature front migration of PRWFG will provide a theoretical basis for further exploration of the optimized operation of the PRWFG system with higher efficiency.

well; temperature; velocity; pumping & recharging well filled with gravel; flow transfixion strength; thermal transfixion; temperature front; numerical simulation

宋 伟，刘远周，郑湍峰，刘晓秀. 热源井填砾抽灌同井流/热贯通及温度锋面运移数值模拟[J]. 农业工程学报，2018，34(24)：210－216. doi：10.11975/j.issn.1002-6819.2018.24.025 http://www.tcsae.org

Song Wei, Liu Yuanzhou, Zheng Tuanfeng, Liu Xiaoxiu. Numerical simulation on flow/thermal transfixion and temperature front migration of thermal well of pumping & recharging well filled with gravel[J]. Transactions of the Chinese Society of Agricultural Engineering (Transactions of the CSAE), 2018, 34(24): 210－216. (in Chinese with English abstract) doi：10.11975/j.issn.1002-6819.2018.24.025 http://www.tcsae.org

2018-04-08

2018-10-23

国家自然科学基金资助项目（41602278）；中国博士后科学基金资助项目（2016M601129）；供热供燃气通风及空调工程北京市重点实验室研究基金资助课题（NR2016K03）；北方工业大学青年拔尖人才培育计划资助项目（XN018032）；北方工业大学青年毓杰人才计划资助项目（18XN154-006）

宋 伟，博士，副教授，长期从事浅层地热能领域的研究。Email：stillwater2013@163.com

10.11975/j.issn.1002-6819.2018.24.025

TK529

A

1002-6819(2018)-24-0210-07