Scheme for Asymmetric and Deterministic Controlled Bidirectional Joint Remote State Preparation∗
2018-11-19JinShi施锦andYouBangZhan詹佑邦
Jin Shi(施锦) and You-Bang Zhan(詹佑邦)
School of Physics and Electronic Electrical Engineering,Jiangsu Province Key Laboratory of Modern Measurement Technology and Intellige,Huaiyin Normal University,Huaian 223300,China
AbstractThe scheme for asymmetric and deterministic controlled bidirectional joint remote state preparation by using one ten-qubit entangled state as the quantum channel is proposed.In this scheme,Alice and David want to remotely prepare an arbitrary single-qubit state at Bob’s site,at the same time,Bob and Eve wish to help Alice remotely prepare an arbitrary two-qubit entangled state.Alice and Bob can simultaneously prepare the desired states with the cooperation of David and Eve under the control of Charlie.
Key words:joint remote state preparation,entangled state,unitary transformation
1 Introduction
As the carrier of information,quantum states are easily intercepted or replaced by eavesdroppers when direct transmission.Quantum teleportation(QT)[1]and remote state preparation(RSP)[2−4]are two important techniques for transferring information in quantum communication.Based on quantum entanglement,many QT and RSP schemes can be realized via local operations and classical communication.Different from QT protocols,the prepared state is completely known to the sender in RSP schemes.RSP can consume less resources than QT,especially for reconstructing large quantum states.RSP protocols are widely used in quantum information.Various theoretical and experimental RSP schemes[5−16]have been proposed.
In order to avoid the combination of information concentrated on one person,joint remote state preparation(JRSP)schemes[17−37]have been put forward.Each of senders knows partly the state to be prepared in JRSP,which is a secure method for transmitting quantum state.Deterministic joint remote state preparation is that the total successful probability reaches 100%.[32−37]Recently,as the new type of quantum communication schemes,bidirectional RSP schemes include symmetric bidirectional RSP[38−41]and asymmetric bidirectional RSP[42−46]have been proposed.The schemes of controlled bidirectional RSP(CBRSP)were presented.[47−48]The distant collaborators can simultaneously prepare the desired states with permission of the controller in CBRSP protocols.
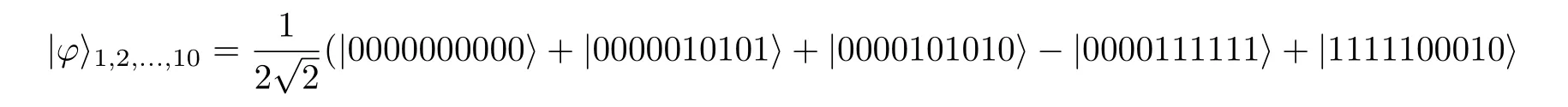
In this paper,a novel scheme for asymmetric and deterministic controlled bidirectional JRSP(CBJRSP)is proposed by using one ten-qubit entangled state as the quantum channel.In our asymmetric and deterministic CBJRSP scheme,Alice and David wish to remotely prepare an arbitrary single-qubit state at distant Bob’s site,meanwhile,Bob and Eve want to remotely prepare an arbitrary two-qubit state at distant Alice’s site under the control of the supervisor Charlie.It is shown that,only if the controller Charlie agrees to collaborate with four senders,they can remotely prepare the desired states by means of some appropriate basis vectors measurement and unitary operations.
2 Asymmetric and Deterministic CBJRSP by Using One Ten-Qubit Entangled State
Since bidirectional QT schemes and bidirectional JRSP schemes by using multi-qubit entangled state have been reported in many papers,we try to propose the scheme for asymmetric and deterministic CBJRSP by using one ten-qubit entangled state as the quantum channel in this section.Suppose Alice and David wish to help Bob remotely prepare an arbitrary one-qubit state|ϕ⟩,at the same time,Bob and Eve wish to help Alice remotely prepare an arbitrary two-qubit stateunder the control of Charlie.
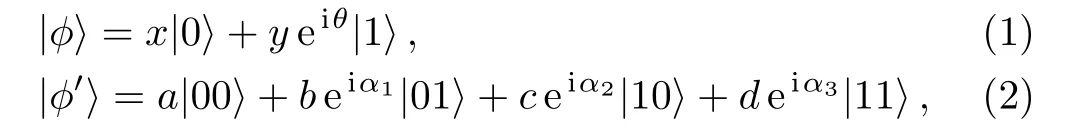
where modulus coefficients(x,y,a,b,c and d)are real and x2+y2=1,a2+b2+c2+d2=1.Alice knows modulus coefficients(x and y).David knows phase coefficient θ.Bob knows modulus coefficients(a,b,c and d).Eve knows phase coefficients αj,(j=1,2,3).Alice,Bob,Charlie,David,and Eve share one ten-qubit entangled state as the quantum channel.
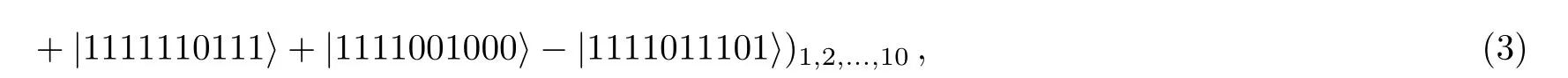
where the qubits 1,9,and 10 belong to Alice,the qubits 3,5,and 6 belong to Bob,the qubit 4 belongs to Charlie,the qubit 2 belongs to David,the qubits 7 and 8 belong to Eve.As shown in Fig.1,we have presented the sketch of the protocol for CBJRSP via a ten-qubit entangled state as the quantum channel.
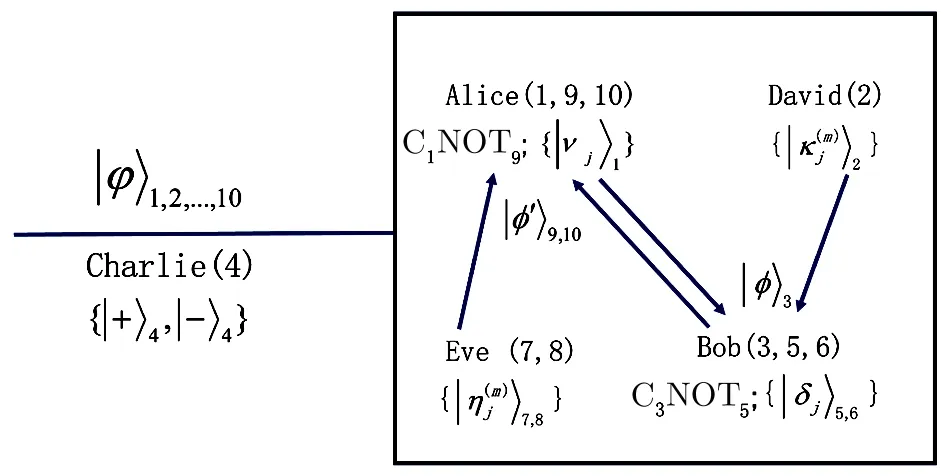
Fig.1 The sketch of our CBJRSP protocol.

Asymmetric and deterministic CBJRSP can be realized under control of Charlie.The controller Charlie performs a one-qubit measurement on the qubit 4 under the measuring basis{|+⟩,|−⟩}.If the measuring result is|+⟩4,then the statecan be transformed into

Alice operates CNOT on her qubits 1 and 9.The qubit 1 as controlled qubit and the qubit 9 as target qubit(C1NOT9).Bob also operates CNOT on his qubits 3 and 5.The qubit 3 as controlled qubit and the qubit 5 as target qubit(C3NOT5).Then the statecan be transformed into

The state can be rewritten as
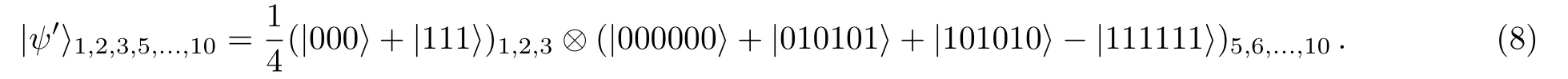

Alice operates C1NOT9on her qubits 1 and 9.Bob also operates C3NOT5on his qubits 3 and 5.Then the statecan be transformed into

The state can be rewritten as

In order to complete the asymmetric and deterministic CBJRSP,Alice chooses a set of mutually orthogonal basis vectorsas the measuring basis of the qubit 1,which are given by
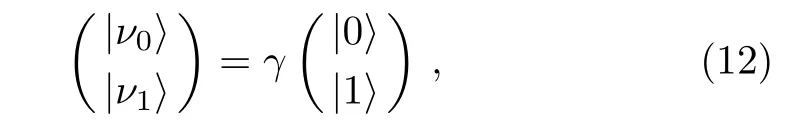
where γ is given
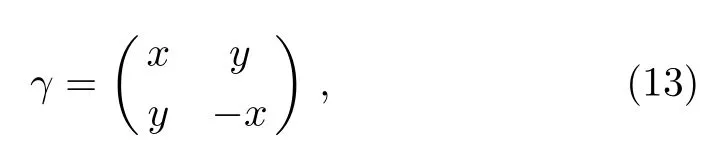
David chooses a set of mutually orthogonal basis vectorsas the measuring basis of the qubit 2,which are given by
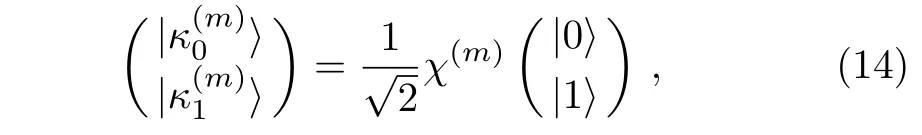
where
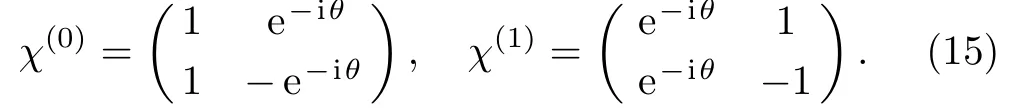
Bob chooses a set of mutually orthogonal basis vectorsas the measuring basis of the qubits 5 and 6,which are given by

where B is given
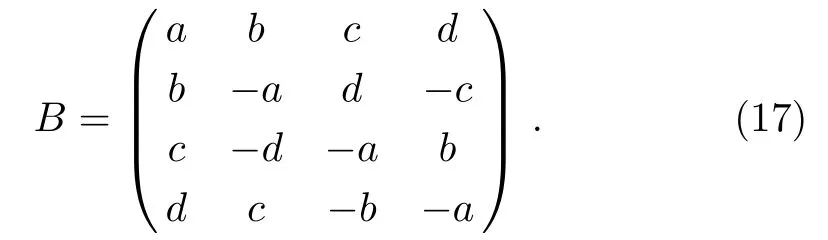
Eve chooses a set of mutually orthogonal basis vectorsas the measuring basis of the qubits 7 and 8,which are given by

where

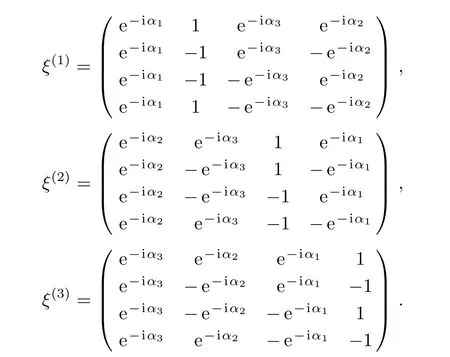
Suppose the measuring results areandthe state can be expressed as
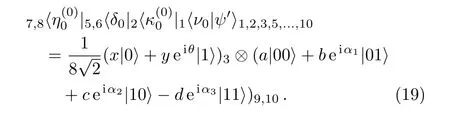
Bob makes unitary transformation(I)3.Alice operates controlled σzgate.The qubit 9 as controlled qubit and qubit 10 as target qubitThen Alice and David help Bob remotely prepare the one-qubit stateBob and Eve help Alice remotely prepare the two-qubit state
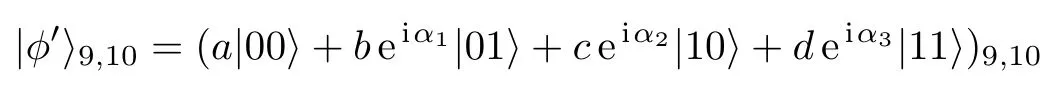
simultaneously. If Charlie’s measuring result isBob makes unitary transformation(σz)3.Alice operates.Then Alice and David help Bob remotely prepareAt the same time,Bob and Eve help Alice remotely prepare the two-qubit stateAll measuring results and operations of Alice and Bob,the measuring results of David and Eve are listed in Table 1.As we can see from Table 1,the measuring results are,then Bob makes unitary transformation(I)3.Bob can obtain one-qubit stateAlice makes unitary transformation,and(σx)9.Alice can obtain two-qubit state
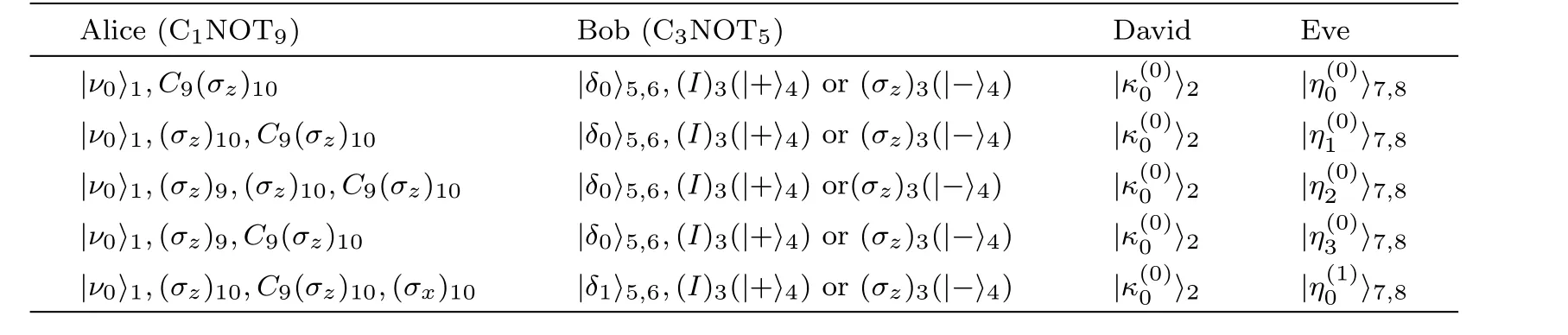
Table 1 The measuring results and operations of Alice and Bob,the measuring results of David and Eve.

Table 1(continued)

Table 1(continued)
3 Conclusion
In summary,by using basis vectors measurement,controlled not gate operation,controlled σzgate operation,and appropriate unitary transformations,the new scheme for asymmetric and deterministic CBJRSP is proposed.In this scheme,Alice and David remotely prepare an arbitrary single-qubit state at distant Bob’s site.Meanwhile,Bob and Eve remotely prepare an arbitrary twoqubit state at distant Alice’s site under the control of Charlie.Our CBJRSP process can be divided into two JRSP processes with only one controller.Different from former controlled JRSP schemes,the asymmetric and deterministic CBJRSP scheme proposed in this paper has higher efficiency and lower resource costs.The asymmetric and deterministic CBJRSP scheme herein has many potential applications in the field of quantum information.For example,in our asymmetric and deterministic CBJRSP scheme,Alice and Bob can exchange their quantum states simultaneously with the cooperators under the controller Charlie,which can realize long-distance safe transmission of information.Similarly,the asymmetric and deterministic CBJRSP scheme can be extended to CBJRSP of N(N>2)different M-qubit(M>2)quantum states.Multi-party exchange their quantum states at the same time with the cooperators under the controller,which can be applied in the quantum internet.Compared with other bidirectional JRSP protocols,our scheme is more secret.We have provided a new way to construct safer quantum channel for quantum secure communication.
杂志排行
Communications in Theoretical Physics的其它文章
- Numerical Study of Unsteady MHD Flow and Entropy Generation in a Rotating Permeable Channel with Slip and Hall Effects∗
- Hierarchical Human Action Recognition with Self-Selection Classifiers via Skeleton Data∗
- Spin Thermoelectric Effects in a Three-Terminal Double-Dot Interferometer∗
- Third-Order Magnetic Susceptibility of an Ideal Fermi Gas∗
- Coherent Control of the Hartman Effect through a Photonic Crystal with Four-Level Defect Layer∗
- Linear Analyses of Langmuir and EM Waves in Relativistic Hot Plasmas∗
