冰盖下梯形及抛物线形输水明渠正常水深显式迭代算法
2018-08-10韩延成初萍萍梁梦媛高学平
韩延成,初萍萍,梁梦媛,唐 伟,高学平
冰盖下梯形及抛物线形输水明渠正常水深显式迭代算法
韩延成1,初萍萍1,梁梦媛1,唐 伟1,高学平2
(1. 济南大学资源与环境学院,济南 250022;2. 天津大学水利工程仿真与安全国家重点实验室,天津 300072)
随着冬季用水量的增加,越来越多的输水工程在冬季冰盖下输水,冰盖下输水已经成为一种常态化输水方式,但目前对明渠正常水深的显式计算方法的研究主要针对不结冰渠道的,缺少对冰盖下输水时正常水深的显式计算方面的研究。该文推导了梯形断面冰盖下输水时正常水深和流量关系,提出了正常水深的简易显式迭代算法,并经过证明,此迭代算法是收敛的。用同样的方法,推导了抛物线形断面冰盖下输水时正常水深和流量关系,提出了计算正常水深的简易显式迭代算法。算例表明,该文提出的冰盖下梯形断面和抛物线形断面的显式迭代算法具有形式简单、计算量小、精度高,收敛性好的特点,一般需要3~5次迭代就可使误差小于0.01 m,当增大迭代次数时,误差进一步减小。研究为冰盖下输水渠道正常水深计算提供了便捷的计算方法,对冰期输水渠道的设计及运行管理具有理论和实践意义。
渠道;水力学; 设计; 明渠; 冰盖下输水;正常水深;显式迭代算法
0 引 言
中国北方受蒙古高压寒冷气流的控制,冬季寒冷,北方10月以后大部分地区气温都会降到0℃以下,水体表面会发生结冰现象。随着冬季反季节农业种植规模的扩大以及城市的发展,冬季需水量大幅增加。越来越多的工程采用常年输水或冬季输水。例如引黄济青工程为冬季输水渠道,输水期为10月至翌年3月。南水北调东线山东段输水期为10月至翌年5月。另外南水北调中线、引滦入津、引黄济津、万家寨引黄调水、新疆乌什水水库引水工程等在结冰后也采用冰盖下输水的模式。除此之外,东北、西北、华北等地许多引水发电工程也采用冰盖下输水。冬季冰盖下输水已经成为一种重要的渠道输水方式[1-2]。
正常水深的计算是渠道水力学计算的重要内容之一,在渠道设计、运行管理、输水调度过程中大量使用[3]。但是正常水深与流量之间为复杂的非线性函数关系,不能直接求解,不方便工程应用[4-6]。学者们对正常水深的显式求解方法进行了大量的研究,赵延风等[4,7]提出了梯形断面的迭代算法,Bijankhan等[8]给出了蛋形断面正常水深的迭代算法,Liu等[9]研究了马蹄形断面的正常水深的迭代算法,Li等[10]提出了抛物线形断面正常水深的迭代算法。张新燕等[11]研究了抛物线形断面的正常水深计算公式,张宽地等[12]研究了圆形隧洞正常水深的牛顿迭代算法,张新燕等[13-14]采用SAS软件研究了U形渠道和圆形隧洞无压流正常水深直接求解公式,武周虎[15]提出了蛋形断面明渠正常水深的简化算法。
这些显式或直接计算方法,极大地方便了渠道设计者和运行管理者。但是已有的正常水深计算方法和公式是针对不结冰渠道的,对冰期输水渠道,冰盖的形成导致过流能力下降[16],其正常水深计算需要考虑冰盖阻力的影响;因此那些不结冰条件下正常水深的显式计算公式是不适应的。目前还缺少对冰盖下输水时正常水深的显式计算方法研究,对冰盖下输水渠道的运行和管理带来了不便,研究冰盖下正常水深的计算对冰期输水渠道设计、运行具有重要意义。本文以梯形断面、抛物线形断面为研究对象,推导冰盖下输水时正常水深和流量之间的关系,提出梯形断面、抛物线形断面正常水深的简易显式迭代算法,对方便冰期输水渠道在运行、设计中正常水深的计算具有现实意义。
1 梯形断面冰盖下输水均匀流计算
由于冰盖密度较水小,且柔性较大,冰盖一般浮在水面。冰盖下均匀流一般用曼宁公式表示为[17]


将式(2)代入式(1)得到冰盖下输水正常水深计算公式为

2 梯形断面的正常水深的显式迭代法
2.1 显式迭代算法


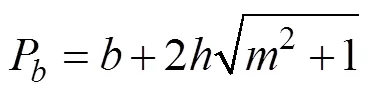



注:为水深,m;为底宽,m;为边坡系数;下同。
Note:is water depth, m;is bottom width, m;is side slope; Same as below.
图1 冰盖下梯形渠道断面示意图
Fig.1 Schematic of cross section of trapezoid channel under ice cover
由式(9)可以看出,冰盖下输水糙率不是常数[2],其与水深有关。将式(4)、(8)、(9)代入式(1),得到冰盖下均匀流输水流量的计算公式为




因此迭代公式可表示为
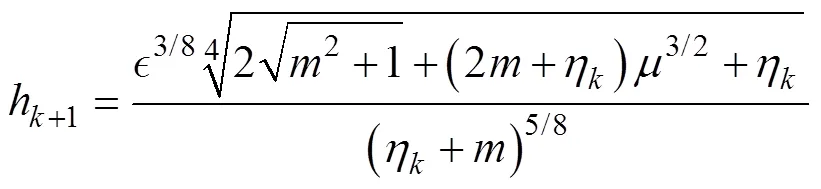

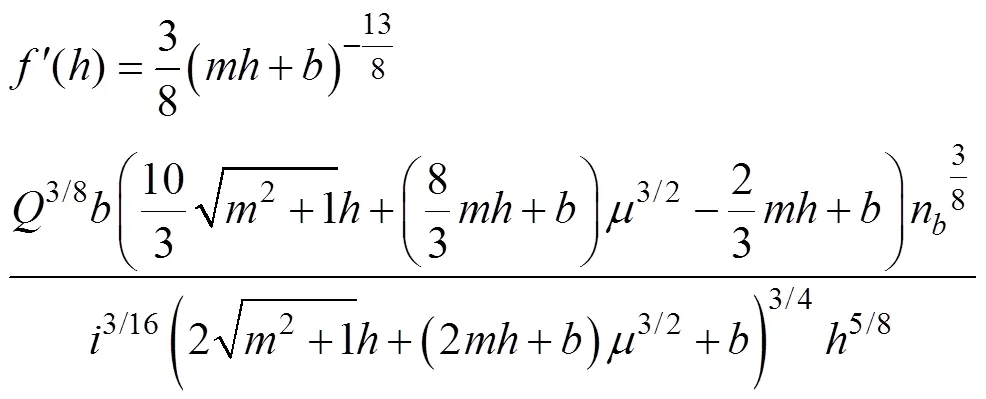
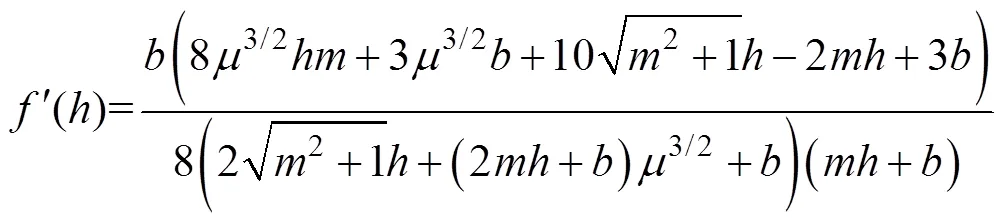


2.2 梯形断面简易迭代公式的应用

编制数值求解程序,采用埃特金迭代法或拟牛顿法[23],求解式(10),得到正常水深h=3.745 138 7 m。将其作为理论参考值。
为了检验迭代公式的收敛性,采用不同初值0=0.01、0.1、1.0、2.0、5.294 2、10、100 m(其中,=5.294 2 m为根据水力最优断面得到的正常水深),代入式(13)迭代得到结果如表1所示。可以看出,本迭代公式具有很好的收敛性,一般需要3~5次就可使误差小于0.01 m。取水力最优断面为初值时,只需要3次可使误差小于0.01 m。除了上述初值,也用其他初值经过检验,不论初值多少,公式均具有良好的收敛效果。当增大迭代次数时,不论初值为多少,均能收敛于理论值。

表1 梯形断面不同初值时正常水深的迭代结果
注:0为初始值,h为第次迭代结果,h理论参考值。下同。
Note:0is the initial value;his the value of thethiteration for normal depth,his the theoretical value of normal depth. Same as below.
3 冰盖下抛物线形断面的正常水深显式迭代算法
3.1 冰盖下抛物线形渠道均匀流计算
学者们普遍认为抛物线形断面(见图2)具有拐点少、应力集中点少、裂缝少、渗漏小,另外具有稳定性好、过流能力大,水力学特性优良的特点[24-27]。巴基斯坦的High Level渠,西班牙Genil-Cabra渠等采用了抛物线形断面[28]。
抛物线形断面形状可表示为[17,29-31]


注:为水面宽度;为水深,m。
Note:is width of water surface, m;is water depth, m.
图2 冰盖下抛物线形渠道断面特性
Fig.2 Cross section characteristics of parabolic channel under ice cover

湿周包括渠床湿周和冰盖造成的湿周,分别表示为

将式(22)代入式(2),可以得到综合糙率为

将式(21)~式(23)代入曼宁公式(1),简化后得到流量和水深的关系式为

3.2 显式迭代法公式构建

根据式(25),构造的迭代公式为

为了减小迭代次数,初值可取非冰盖下抛物线形最优断面得到的水深[26-27]

用同样的方法,可以证明式(26)是收敛的,在此不在赘述。
3.3 显式迭代法公式应用
编制数值求解程序,采用埃特金迭代法或拟牛顿 法[23],均可得到正常水深h=3.846 841 14 m。将其作为理论参考值。
根据水力最优断面公式(27),得到水力最优断面条件下0=3.040 4 m。取不同初值(0=0.01、0.1、1.0、5.0、10.0、100、3.040 4 m),根据迭代公式(26)得到结果如表2所示。
可以看出,本迭代公式具有很快的收敛速度,一般需要3~5次就可使误差小于0.01 m。取最优断面为初值时,只需要3次可使误差小于0.01 m。当增大迭代次数时,不同初值,均能收敛于理论值。

表2 抛物线形断面不同初值正常水深迭代结果
4 结 论
本文推导了冰盖下输水时梯形断面和抛物线形断面综合糙率及流量计算公式,根据理论推导提出了冰盖下梯形断面和抛物线形断面正常水深显式迭代算法,得到如下结论:
1)提出了冰盖下梯形断面和抛物线形断面的新的迭代公式,具有形式简单,计算方便的特点,采用不同初值,均能收敛于理论值。
2)通过理论证明,本文提出的冰盖下梯形断面和抛物线形断面正常水深的迭代公式是收敛的。
3)实例表明,本文提出的迭代公式具有很好的收敛性,一般需要3~5次就可使误差小于0.01 m。增加迭代次数后都能收敛到理论值。
[1] 穆祥鹏,陈文学,郭晓晨,等. 高纬度地区渠道无冰盖输水的冰情控制研究[J]. 水利学报,2013,44(9):1071-1079. Mu Xiangpeng, Chen Wenxue, Guo Xiaochen et al. Study on long-distance water transfer channel with thermal insulation cover in winter[J]. Journal of Hydraulic Engineering, 2013, 44(9): 1071-1079. (in Chinese with English abstract)
[2] Han Yancheng, Xu Zhenghe, Easa S M, et al. Optimal hydraulic section of ice-covered open trapezoidal channel[J]. Journal of Cold Regions Engineering, 2017, 31 (3): 06017001. DOI: 10.1061/(ASCE)CR.1943-5495.0000128
[3] 韩延成,徐征和,高学平,等. 二分之五次方抛物线形明渠设计及提高水力特性效果[J]. 农业工程学报,2017,33(4):131-136. Han Yancheng, Xu Zhenghe, Gao Xueping, et al. Design of two and a half parabola-shaped canal and its effect in improving hydraulic property[J]. Transactions of the Chinese Society of Agricultural Engineering (Transactions of the CSAE), 2017, 33(4): 131-136. (in Chinese with English abstract)
[4] 赵延风,祝晗英,王正中,等. 梯形明渠正常水深的直接计算方法[J]. 西北农林科技大学学报:自然科学版,2009,37(4):220-224. Zhao Yanfeng, Zhu Hanying, Wang ZhengZhong, et al. A direct formula for normal depth in trapezoidal open channel[J]. Journal of Northwest A &F University: Nat Sci Ed, 2009, 37(4): 220-224. (in Chinese with English abstract)
[5] 张宽地,吕宏兴,赵延风. 明流条件下圆形隧洞正常水深与临界水深的直接计算[J]. 农业工程学报,2009,25(3):1-5.Zhang Kuandi, Lü Hongxing, Zhao Yanfeng. Direct calculation for normal depth and critical depth of circular section tunnel under free flow[J]. Transactions of the Chinese Society of Agricultural Engineering (Transactions of the CSAE), 2009, 25(3): 1-5. (in Chinese with English abstract)
[6] 张新燕,吕宏兴. 抛物线形断面渠道正常水深的显式计算[J]. 农业工程学报,2012,28(21):121-125. Zhang Xinyan, Lü Hongxing. Explicit solution for normal depth in parabolic-shape channel[J]. Transactions of the Chinese Society of Agricultural Engineering (Transactions of the CSAE), 2012, 28(21): 121-125. (in Chinese with English abstracts)
[7] 王正中,席跟战,宋松柏,等. 梯形明渠正常水深直接计算公式[J]. 长江科学院院报,1998,15(6):1-7. Wang Zhengzhong, Xi Genzhan, Song Songbai, et al. A direct calculation formula for normal depth in open trapezoidal channel[J]. Journal of Yangtze River Scientific Research Institute, 1998, 15(6): 1-7. (in Chinese with English abstract)
[8] Bijankhan M and Kouchakzadeh S. Egg-shaped cross
section: Uniform flow direct solution and stability identification[J]. Flow Measurement and Instrumentation, 2011, 22(6), 511-516.
[9] Liu Jiliang, Wang Zhengzhong, and Fang Xing. Iterative formulas and estimation formulas for computing normal depth of horseshoe cross-section tunnel[J]. Journal of Irrigation and Drainage Engineering, 2010, 36(11): 786-790.
[10] Li Yonghong, Gao Zhaoliang. Explicit solution for normal depth of parabolic section of open channels[J]. Flow Measurement and Instrumentation, 2014, 38: 36-39.
[11] 张新燕,吕宏兴. 抛物线形断面渠道正常水深的显式计算[J]. 农业工程学报,2012,28(21):121-125. Zhang Xinyan, Lü Hongxing. Explicit solution for normal depth in parabolic-shape channel[J]. Transactions of the Chinese Society of Agricultural Engineering (Transactions of the CSAE), 2012, 28(21): 121-125. (in Chinese with English abstracts)
[12] 张宽地,吕宏兴,赵延风. 明流条件下圆形隧洞正常水深与临界水深的直接计算[J]. 农业工程学报,2009,25(3):1-5.Zhang Kuandi, Lü Hongxing, Zhao Yanfeng. Direct calculation for normal depth and critical depth of circular section tunnel under free flow[J]. Transactions of the Chinese Society of Agricultural Engineering (Transactions of the CSAE), 2009, 25(3): 1-5. (in Chinese with English abstract)
[13] 张新燕,吕宏兴,朱德兰. U形渠道正常水深的直接水力计算公式[J]. 农业工程学报,2013,29(14):115-119. Zhang Xinyan, Lü Hongxing, Zhu Delan. Direct calculation formula for normal depth of U-shaped channel[J]. Transactions of the Chinese Society of Agricultural Engineering (Transactions of the CSAE), 2013, 29(14): 115-119. (in Chinese with English abstract)
[14] 张新燕,吕宏兴. 无压圆形隧洞正常水深直接算法[J]. 水力发电学报,2014,33(1):127-131. Zhang Xinyan, Lü Hongxing. Direct calculation method for normal depth in non-pressure circular tunnel[J]. Journal of Hydroelectric Engineering, 2014, 33(1): 127-131. (in Chinese with English abstract)
[15] 武周虎.蛋形断面明渠正常水深和临界水深的简化算法[J]. 人民长江,2014(4):73-76.Wu Zhouhu. Simplified algorithm of normal depth and critical depth of egg-shape cross-section open channel[J]. Yangtze River, 2014(4): 73-76. (in Chinese with English abstract)
[16] 杨开林. 明渠冰盖下流动的综合糙率[J]. 水利学报,2014,45(11):1310-1317. Yang Kailin. Composite roughness of ice-covered flows in open channels[J]. Journal of Hydraulic Engineering, 2014, 45(11): 1310-1317. (in Chinese with English abstract)
[17] Chow V T. Open Channel Hydraulics[M]. New York: McGraw-Hill, 1959.
[18] Uzuner M S. The composite roughness of ice covered streams[J]. Journal of Hydraulic Research, 1975, 13(1): 79-102.
[19] 颜丙池. 南水北调冰期输水原型观测与研究[J]. 河北水利水电技术,2002(2):41-42. Yan Bingchi. Prototype observation and research for South- to-North Water Diversion Project during freezing period[J]. Water Conservancy and Hydropower Technology of Hebei, 2002(2): 41-42. (in Chinese with English abstract)
[20] 马喜祥,白世录,袁学安,等. 中国河流冰情[M]. 郑州:黄河水利出版社,2009
[21] 穆祥鹏,陈文学,崔巍,等. 南水北调中线干渠冰期输水能力研究[J]. 南水北调与水利科技,2009,7(6):118-122. Mu Xiangpeng, Chen Wenxue, Cui Wei et al. Study on water diversion capacity in the middle route of the south- to-north water transfer project in ice period[J], South- to-North Water Transfers and Water Science & Technology, 2009, 7(6): 118-122. (in Chinese with English abstract)
[22] 颜庆津. 数值分析[M]. 北京:北京航天航空大学出版社,2006.
[23] 周长发. 科学与工程数值算法:Visual Basic 版[M]. 北京:清华大学出版社,2002.
[24] Mironenko A P, Willardson L S, Jenab S A. Parabolic canal
design and analysis[J]. Journal of Irrigation and Drainage Engineering, 1984, 110(2): 241-246.
[25] Han Yancheng. Horizontal bottomed semi-cubic parabolic channel and best hydraulic section[J]. Flow Measurement and Instrumentation, 2015, 45: 56-61.
[26] Han Yancheng, Easa S M. Superior cubic channel section and analytical solution of best hydraulic properties[J]. Flow Measurement and Instrumentation, 2016, 50: 169-177.
[27] Han Yancheng, Easa S M. New and improved three and one-third parabolic channel and most efficient hydraulic section[J]. Canadian Journal of Civil Engineering, 2017, 44(5): 387-391.
[28] Anwar A A, de Vries T T. Hydraulically efficient power-law channels[J]. Journal of Irrigation and Drainage Engineering, 2003, 129(1): 18-26.
[29] Hu Han, Huang Jiesheng, Qian Zhongdong, et al. Hydraulic analysis of parabolic flume for flow measurement[J]. Flow Measurement and Instrumentation, 2014, 37: 54-64.
[30] Han Yancheng, Gao Xueping., Xu Zhenghe. The best hydraulic section of horizontal-bottomed parabolic channel section[J]. Journal of Hydrodynamics, 2017, 29(2): 305-313.
[31] Loganathan G V. Optimal design of parabolic canals[J]. Journal of Irrigation and Drainage Engineering, 1991, 117(5): 716-735.
Explicit iterative algorithm of normal water depth for trapezoid and parabolic open channels under ice cover
Han Yancheng1, Chu Pingping1, Liang Mengyuan1, Tang Wei1, Gao Xueping2
(1.250022,; 2.300072,)
With the increase of water demand in winter in northern China, more and more open-channel water diversion projects transport water under the ice cover in winter such as the Middle Route of South-to-North Water Diversion Project. The method of transporting water using open channel under the ice cover has become increasingly common to delivery water from reservoirs, rivers and lakes to cities. The normal water depth is an important parameter in channel design, operation, flood control, and flow measurement and maintenance of the open channel or sewage systems. The explicit calculation algorithms of the normal water depth for the open channel are mainly for free-ice channels. This paper proposed algorithm of normal water depth of open channel under ice cover. The expression of the synthesis roughness for the flow of the channel under the ice cover was determined based on the studies of Sabaneev. The relationship between the normal water depth and the flow rate of the trapezoid section under the ice cover was derived. A simple explicit iterative algorithm to compute the normal water depth was then proposed. It proved that the new iterative algorithm was convergent using the convergence theory of iteration. The formula to getting the initial value was proposed using the best hydraulic section. The application examples were given to compute the normal depth under the ice cover by using the new iterative algorithm. The results showed that the explicit iterative algorithm proposed had a fast convergence speed. In general, the error would be less than 0.01 m with only 3-5 times iterations. The number of the iteration decreased when using the initial value from the best hydraulic section. When the number of iterations was increased, the iterative value would be closer to the theoretical value of the normal depth. By using the same method, the relationship between the normal water depth and the flow rate under the ice cover was derived for the parabolic section, and a simple explicit iterative algorithm for calculating the normal depth of water was proposed too. The examples showed that the explicit iterative formula of the parabolic section proposed was simple and had a fast convergence speed too. In general, the error would be less than 0.01 m with only 3-5 times iterations as well as trapezoid section. The study of this paper provides a convenient method for the calculation of the normal water depth of the water conveyance channel under the ice cover. The research has theoretical and practical significance for the design, operation and management of the water conveyance channels under the ice cover because the normal depth is most widely used in the water conservancy project.
canals; hydraulic; design; open channel; water transport under ice cover; normal depth of water; explicit iterative algorithm
10.11975/j.issn.1002-6819.2018.14.013
TV 131.4
A
1002-6819(2018)-14-0101-06
2018-01-31
2018-05-10
国家“十二五”科技支撑计划(2015BAB07B02-6);山东省自然科学基金(ZR2017LEE028); 山东省重点研发计划(2016GSF117038)
韩延成,甘肃武威人,副教授,主要从事水力学及河流动力学方面的研究。Email:stu_hanyc@ujn.edu.cn
韩延成,初萍萍,梁梦媛,唐 伟,高学平.冰盖下梯形及抛物线形输水明渠正常水深显式迭代算法[J]. 农业工程学报,2018,34(14):101-106. doi:10.11975/j.issn.1002-6819.2018.14.013 http://www.tcsae.org
Han Yancheng, Chu Pingping, Liang Mengyuan, Tang Wei, Gao Xueping. Explicit iterative algorithm of normal water depth for trapezoid and parabolic open channels under ice cover[J]. Transactions of the Chinese Society of Agricultural Engineering (Transactions of the CSAE), 2018, 34(14): 101-106. (in Chinese with English abstract) doi:10.11975/j.issn.1002-6819.2018.14.013 http://www.tcsae.org
