Residual Symmetry Reduction and Consistent Riccati Expansion of the Generalized Kaup-Kupershmidt Equation∗
2018-06-15XiZhongLiu刘希忠JunYu俞军ZhiMeiLou楼智美andQiaoJunCao曹巧君
Xi-Zhong Liu(刘希忠),Jun Yu(俞军),Zhi-Mei Lou(楼智美),and Qiao-Jun Cao(曹巧君)
Institute of Nonlinear Science,Shaoxing University,Shaoxing 312000,China
1 Introduction
Nonlinear wave equations in mathematical physics play a major role in various fields,such as plasma physics,lf uid mechanics,optical fibers,solid state physics,chemical kinetics,geochemistry,and so on.[1]It is well known that the symmetry method is one of the most powerful tools in differential equations.The Lie point symmetry groups and associated reduction solutions can be obtained by using the classical or nonclassical Lie group method.[2−3]On the other hand,for integrable systems,nonlocal symmetries can be obtained via Lie-Baklund symmetries,[4]potential symmetries,[5]inverse recursion operators,[6]the conformal-invariant form,[7]Darboux transformation,[8]Baklund transformation(BT)and Lax pair.[9]However,nonlocal symmetries cannot be used directly to construct finite transformations and symmetry reduction solutions.
Recently,nonlocal residual symmetry related with the truncated Painleve expansion has been investigated and many interesting results were obtained by localizing it to a Lie point symmetry in a new system.[10−14]Furthermore,by generalizing the truncated Painleve expansion,Lou de fined a new integrability for many nonlinear systems in the sense of possessing a consistent Riccati expansion(CRE).[15]For CRE integrable systems,interaction solutions between soliton and nonlinear waves can be easily obtained.[16−18]
In this paper,we investigate the generalized Kaup-Kupershmidt(gKK)equation by applying residual symmetry localization procedure and CRE method,respectively.The gKK equation is a fifth-order nonlinear evolution equation,which takes the form

with arbitrary constantsaandb.In particular,by takinga=1/10,b=−5 in Eq.(1),we get the Kaup-Kupershmidt(KK)equation
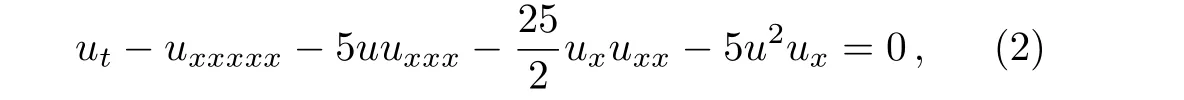
which is one of the solitonic equations related to the integrable cases of the Henon-Heiles system.Although the KK equation resembled with the Sawada-Kotera(SK)equation

they are fundamentally different,since there is no scaling which reduces Eq.(2)to Eq.(3).N-soliton solutions of the KK equation have been found by making use of Hirota’s bilinear transformation method.[19]In Ref.[20],the authors gave explicit formulas for the in finitesimal generators of symmetries by using a bi-Hamiltonian formulation.Moreover,the KK equation has in finite sets of conservation laws.[21−24]
This paper is organized as follows. In Sec.2,the residual symmetry of the gKK system is obtained from the truncated Painleve expansion and localized into a Lie point symmetry in a prolonged system,then the corresponding finite transformation group is given by using Lie’s first principle.In Sec.3,the general form of Lie point symmetry group of the prolonged gKK system is obtained and the corresponding symmetry reduction solutions are constructed by using the standard Lie symmetry method,from which the interaction solutions between solitons and nonlinear waves for the original gKK equation(1)could be derived out.In Sec.4,the gKK equation is proved to be CRE integrable and some new Baklund tansformations are given based on this property,from which new interaction solutions of the gKK equation are obtained.The last section contains discussion and a summary.
2 Localization of Residual Symmetry and the Related Backlund Transformation
By means of leading-order analysis,the truncated Painleve expansion of Eq.(1)reads
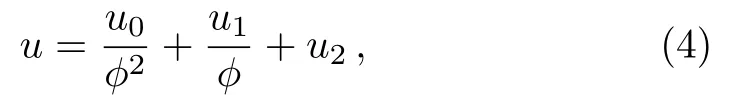
whereϕis the singular manifold,andu0,u1,v2are functions ofx,tto be determined later.Substituting Eq.(4)into Eq.(1)and setting zero the coefficients of all the same powers of 1/ϕwe obtain

whereϕsatis fies Schwartzian form of Eq.(1)
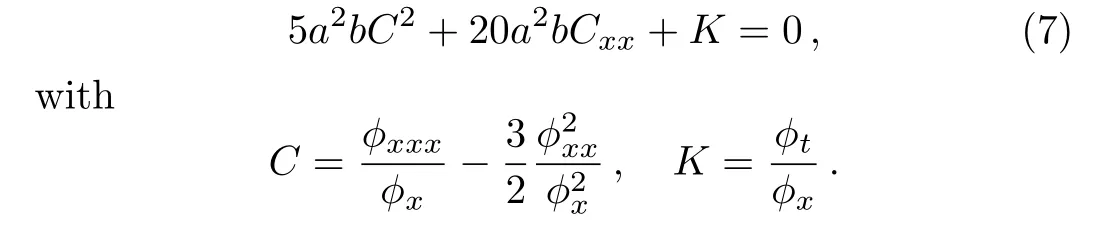
It is obviously that Eq.(7)is form invariant under the M¨obious transformation
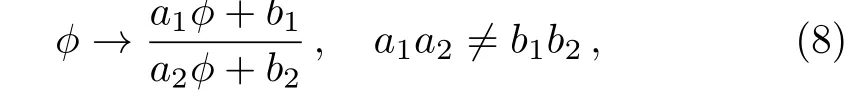
which means Eq.(7)possess three symmetriesσϕ=d1,σϕ=d2ϕand

with arbitrary constantsd1,d2,andd3.
By substituting Eq.(5)into Eq.(4),we get the following BT:
Theorem1Ifϕis a solution of the Schwartzian equation(7),then the solutionuof Eq.(1)is expressed by
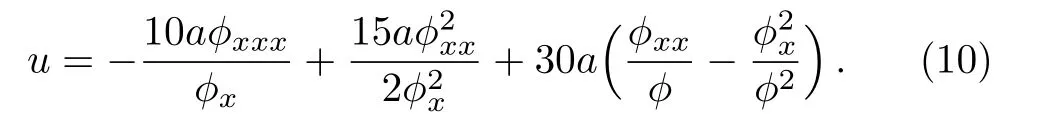
It is interesting that the residueu1of the truncated Painleve expansion(4)with respect to the singular manifoldϕis just a nonlocal symmetry of Eq.(1)with the solutionu2,which can be verified by substituting it into the linearized form of the gKK equation with Eqs.(5)and(7),for this reason it is called residual symmetry.Actually,the residual symmetryσu=u1is just the generator of the Backlund transformation(4),which relates to the M¨obious transformation symmetry(9)by the linearized equation of Eq.(6).
Due to the nonlocal property of a residual symmetry,it is hard to construct the corresponding finite transformation.To overcome this obstacle,a direct way is to localize it in a new prolonged system by introducing two new dependent variables,i.e.,

All the symmetries of the different variables are related with each other by the linearized equations of Eqs.(1),(7),(11),and(12)
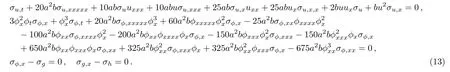
It can be easily verified that the solutions of Eq.(13)have the form

ifd3=−1/30aandd1=d2=0 is fixed forσϕ.In other words,the residual symmetryσu=30aϕxxis localized in the properly prolonged system(1),(7),(11),and(12)with the Lie point symmetry vector
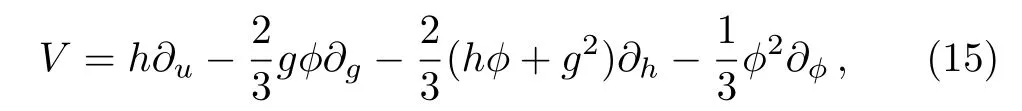
which means that symmetries related to the truncated Painleve expansion is just a special Lie point symmetry of the prolonged system.
The finite transformation group corresponding to the Lie point symmetry(15)can be found by solving the following initial problem
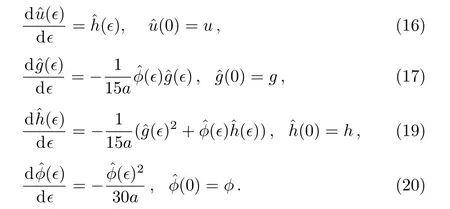
Solvingouttheseequationsleadsto thefollowing Backlund transformation:

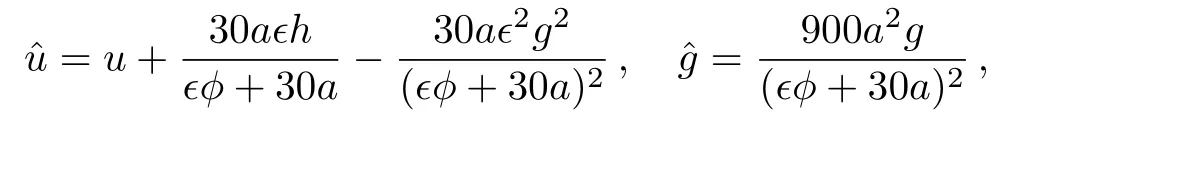
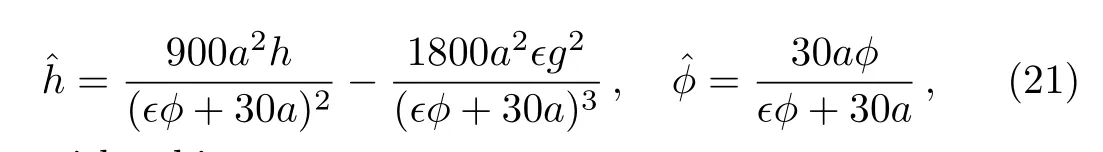
with arbitrary group parameterϵ.
3 New Symmetry Reductions of the Generalized Kaup-Kupershmidt Equation
We seek the Lie point symmetry of the prolonged gKK system(1),(7),(11),and(12)in the general form
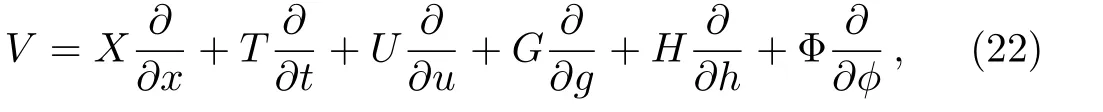
which means that the prolonged gKK system is invariant under the following transformation

with the in finitesimal parameterϵ.Equivalently,the symmetry in the form(22)can be written as a function form

Substituting Eq.(24)into Eq.(13)and vanishing all the coefficients of the independent partial derivatives of dependent variablesu,g,handϕ.Then we obtain a system of over determined linear equations for the in finitesimalsX,T,U,G,H,Φ.Calculated by computer algebra,wefinally get the desired result
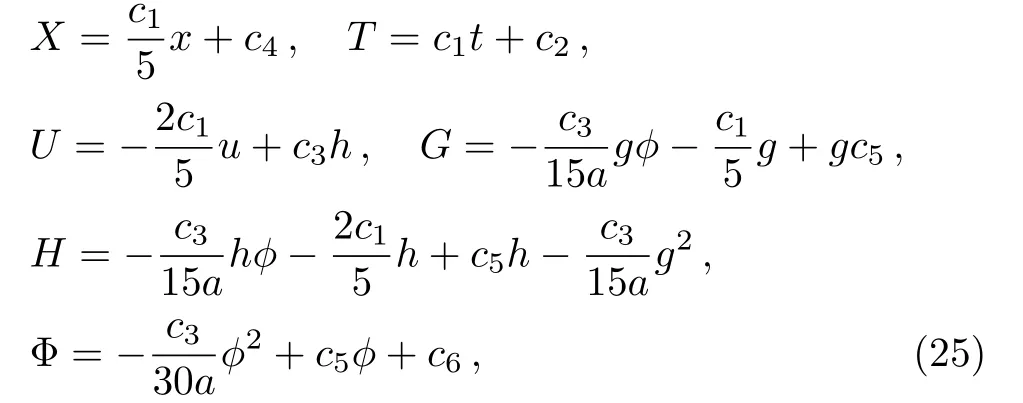
with arbitrary constantsc1,c2,c3,c4,c5,c6.
When settingc1=c5=c6=0 andc3=1 in Eq.(25),the general symmetry degenerated into the special form of Eq.(15),which includes residual symmetry of the gKK equation.
Consequently,the symmetries in Eq.(24)can be written as
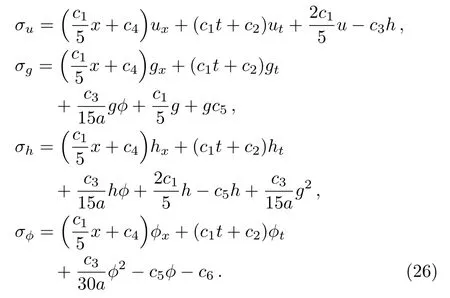
To give out the group invariant solutions of the prolonged gKK system,we have to solve Eq.(26)under symmetry constraintsσu=σg=σh=σϕ=0,which is equivalent to solving the corresponding characteristic equation
Without loss of generality,we consider the symmetry reduction solutions of the prolonged gKK system in the following two subcases.
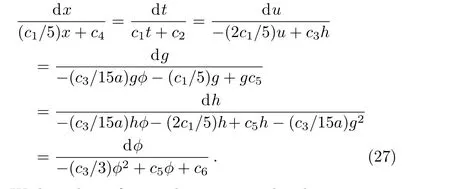
In this case,by solving Eq.(27),we get
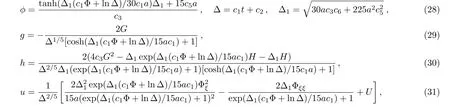
where the group invariant variable is
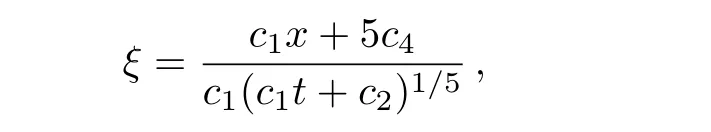
whileU,G,H,and Φ are all group invariant functions ofξ.
Substituting Eqs.(28)–(31)into the prolonged system(2),(7),(11),and(12)yields

where Φ satis fies the following symmetry reduction equation
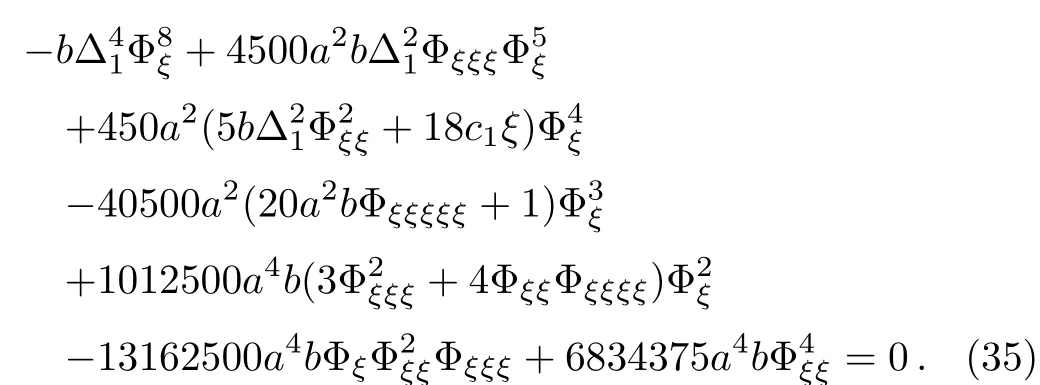
One can see that once Φ is solved out from Eq.(35),thenU,G,andHcan be solved out directly from Eqs.(32),(33),and(34),respectively.The explicit solutions of the gKK equation(1)is immediately obtained by substitutingU,H,G,and Φ into Eq.(31).
To give out a concrete example,we derive a simple solution for Eq.(35)under the condition∆1=3c1a

with arbitrary constantsd1andd2,which leads to a simple solution for the gKK equation(1)

In this case,after solving out Eq.(27),we get the similarity reduction solutions of the prolonged gKK system as follows
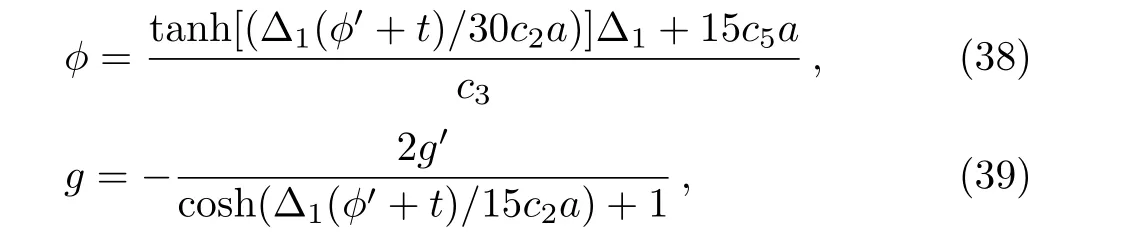
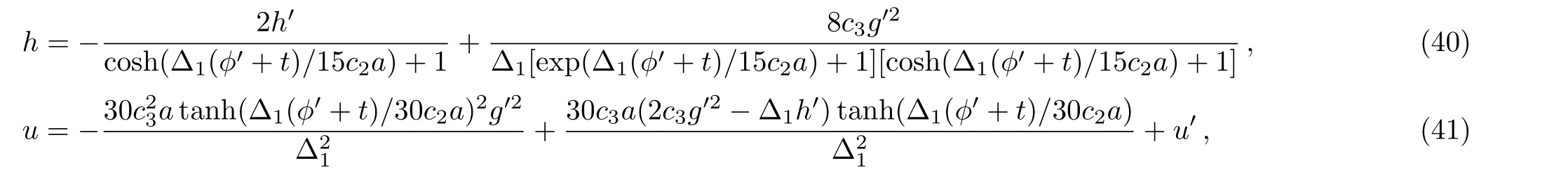
where the group invariant variable isx′=(c2x−c4t)/c2andu′,g′,h′,ϕ′are all group invariant functions ofx′.
Substituting Eqs.(38),(39),(40)and(41)into the prolonged gKK system(1),(7),(11),and(12)yields

whereϕ′satis fies the following symmetry reduction equation
From the symmetry reduction equations(42),(43),(44),and(45),one can easily get exact solutions of gKK equation by solving outu′,g′,h′,ϕ′and substitute them into Eq.(41).
4 CRE Solvability and New Exact Solutions
4.1 CER Integrable
To investigate the consistent Riccati expansion(CRE)integrability for the gKK equation(1),by using leading order analysis,we give the following truncated expansion solution
u=v0+v1R(w)+v2R(w)2,(w=w(x,y,t)),(46)wherev0,v1,v2are arbitrary functions of(x,t)andR(w)is a solution of the Riccati equation
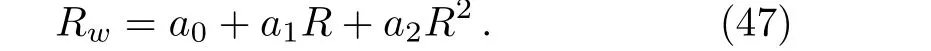
Substituting Eq.(46)with Eq.(47)into Eq.(2)and vanishing all the coefficients of different powers ofR(w),we get seven over-determined equations for only four undetermined functionsv0,v1,v2,andw.It is fortunate that the over determined equations are consistent with each other and the coefficients are

From above discussion,it is shown that gKK equation(1)really has the truncated Painleve expansion solution related to the Riccati equation(47).At this point,we call the expansion(46)a CRE expansion and the gKK equation is CRE integrable.[25]
In summary,we have the following theorem:
Theorem 3Ifwis a solution of
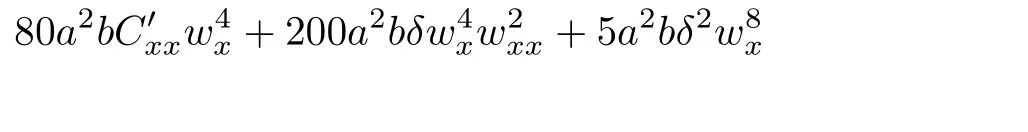
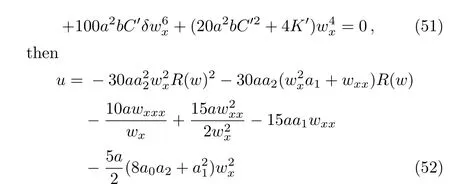
is a solution of the gKK equation(1),withR=R(w)being a solution of the Riccati equation(47).
4.2 Consistent tanh-Function Expansion
When the Riccati equation(47)takes the special solutionR=tanh(w),the truncated Painleve expansion solution(46)becomes
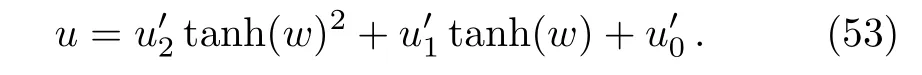
It is quite clear that a CRE solvable system must be CTE(consistent tanh expansion)solvable,and vice versa.If a system is CTE solvable,some important explicit solutions,especially the interactions between soliton and other nonlinear waves,may be directly constructed.To this end,we give out the following non-auto BT.
Theorem 4Ifwsatis fies the following equation

is a solution of the gKK equation(1).
In order to obtain the explicit solution of Eq.(1),we considerwof Eq.(54)in the form

wheregis an arbitrary function ofxandt.It will lead to interaction solutions between solitons and background nonlinear waves.By means of Theorem 4,some nontrivial solutions of the gKK equation can be obtained from some quite trivial solutions of Eq.(54),which are listed as follows.
Case 1In Eq.(54),we take a trivial seed solution
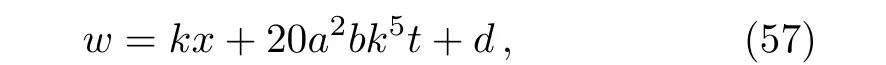
withk,dbeing arbitrary constants.Then substituting Eq.(57)into Theorem 4 yields the following soliton solution for the gKK equation(1)

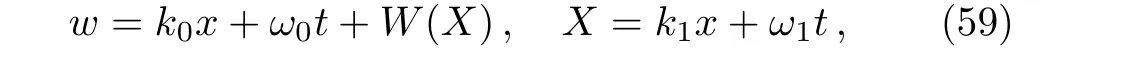
Case 2We consider a special solution of Eq.(54)with the form wherek0,ω0,k1,ω1are arbitrary constants.Substituting Eq.(59)into Eq.(54),we find thatW1(X)≡W(X)Xsatis fies the following elliptic function equation:

whereC2andC3are arbitrary constants.Then the solution of the gKK equation(1)has the form
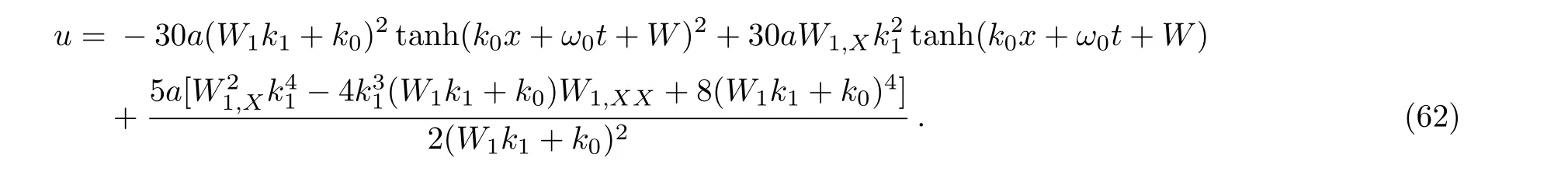
The form of the solution(62)with Eq.(60)indicates that it describes the interaction mode between soliton and periodic waves.It can be verified that there is no simple cnodial solutions likeµ0+µ1cn(mX,n)for Eq.(54)under the condition(61),however,the more complex cnoidal solutions are hard to get.Instead,we give a tanh form solution

with arbitrary constantsµ0,µ1,andm. Substituting Eq.(63)with Eq.(61)into Eq.(60)and setting the coefficients of tanh(mX)equal zero,yields


with Eq.(64)into Eq.(62),we get a new solution for the gKK equation


5 Conclusion and Discussion
In summary,the gKK equation is investigated by using residual symmetry and CRE method,respectively.By introducing new variables,the residual symmetry is localized to a Lie point symmetry in a prolonged system and the corresponding finite transformation group is obtained by using Lie’s first theorem.For the prolonged gKK system,the general form of Lie point symmetry is found and symmetry reduction solutions are obtained in two subcases.From these symmetry reduction solutions,various interaction solutions of the gKK equation could be obtained.Moreover,the gKK equation is proved to be integrable in the CRE sense and some new Backlund transformations are obtained,from which some special special solutions including interaction solution between soliton and periodic waves are obtained.
Besides the residual symmetry discussed in this paper,there are other kinds of nonlocal symmetries,which can be obtained from Backlund transformation,negative hierarchies[26−27]and the self-consistent sources,[28]etc.The relation between these nonlocal symmetries in obtaining interaction solutions is an interesting topic,which will be discussed in our future research work.
[1]A.M.Wazwaz,Commun.Nonl.Sci.Numer.Simulat.10(2005)451.
[2]P.J.Olver,Application of Lie Groups to Differential Equations,Springer-Verlag,New York(1993)
[3]G.W.Bluman and S.Kumei,Symmetries and Differential Equation,Springer-Verlag,Berlin(1989)
[4]F.Galas,J.Phys.A:Math.Gen.25(1992)L981.
[5]G.W.Bluman,A.F.Cheviakov,and S.C.Anco,Applications of Symmetry Methods to Partial Differential Equations,Springer,New York(2010).
[6]S.Y.Lou,Phys.Lett.B 302(1993)261.
[7]S.Y.Lou,J.Phys.A:Math.Gen.30(1997)4803.
[8]S.Y.Lou and X.B.Hu,J.Phys.A:Math.Gen.30(1997)L95.
[9]X.P.Xin and Y.Chen,Chin.Phys.Lett.30(2013)100202.
[10]X.N.Gao,S.Y.Lou,and X.Y.Tang,J.High Energy Phys.05(2013)29.
[11]S.Y.Lou,Residual Symmetries and Baklund Transformations,arXiv:1308.1140v1(2013).
[12]X.R.Hu,S.Y.Lou,and Y.Chen,Phys.Rev.E 85 056607-056615(2012)
[13]W.G.Cheng,B.Li,and Y.Chen,Commun.Nonlinear Sci.Numer.Simulat.29(2015)198.
[14]X.Z.Liu,J.Yu,B.Ren,and J.R.Yang,Chin.Phys.B 24(2015)010203.
[15]S.Y.Lou,Consistent Riccati Expansion and Solvability,arXiv:1308.5891v2(2013).
[16]L.L.Huang and Y.Chen,Chin.Phys.B 25(2016)060201.
[17]W.G.Cheng,B.Li,and Y.Chen,Commun.Theor.Phys.63(2015)549.
[18]Y.H.Wang and H.Wang,Nonlinear Dyn.89(2017)235.
[19]A.Parker,Physica D 137(2000)34.
[20]B.Fuchssteiner and W.Oevel,J.Math.Phys.23(1982)358.
[21]B.A.Kupershmidt,Phys.Lett.A 102(1984)213.
[22]M.Musette and C.Verhoeven,Physica D 144(2000)211.
[23]U.Goktas and W.Hereman,J.Symb.Comput.24(1997)591.
[24]D.Baldwin,U.Goktas,W.Hereman,et al.,J.Symb.Comput.37(2004)699.
[25]S.Y.Lou,Stud.Appl.Math.134(2015)372.
[26]C.W.Cao and X.G.Geng,J.Phys.A:Math.Gen.23(1990)4117.
[27]Y.Cheng and Y.S.Li,Phys.Lett.A 157(1991)22.
[28]Y.B.Zeng,W.X.Ma,and R.L.Lin,J.Math.Phys.41(2000)5453.
杂志排行
Communications in Theoretical Physics的其它文章
- Thermodynamic Nonequilibrium Features in Binary Diffusion∗
- Ordinary Mode Instability in a Cairns Distributed Electron Plasma
- Thermal Conductivity of Complex Plasmas Using Novel Evan-Gillan Approach
- Spontaneous Emission Originating from Atomic BEC Interacting with a Single-Mode Quantized Field
- Molecular Dynamics Simulations of the Elastic Anisotropy of Pd at Extreme Conditions∗
- In fluences of Crystal-Field and Interlayer Coupling Interactions on Dynamic Phase Diagrams of a Mixed-Spin(3/2,2)Bilayer System∗
