带梯度项的广义复Monge-Ampère型方程的梯度估计

袁 日 荣

(厦门大学数学科学学院,福建 厦门 361005)

(1)

(2)

若c0=1且其他的常数为零,则方程(1)就是著名的复Monge-Ampère型方程.带梯度项的复Monge-Ampère型方程的一个特殊且重要的例子是Sasakian度量空间的测地线方程[1].本研究的目的之一是将他们的估计推广到更一般的情形.当c0=c1=…=cn-3=0时,笔者得到了方程(1)的C2,α-估计[2].

对于标准的方程而言,即χ为流形M上一个光滑的实 (1,1)-形式,这类形如式(1) 的方程的研究可追溯到文献[3-6]，这些工作研究了复 Monge-Ampère 方程.从那以后,这类方程引起了很多有趣且重要的研究,可参考文献[7-14]及其引用的文献.

在陈述本文中主要结果之前,需要介绍一些符号.在局部坐标(z1,…,zn)下,记

(3)

同时需假设χ满足如下结构条件：

(4)

本文中的主要结果可如下表述:

(5)

那么对于方程(1)满足χu>0的解u∈C3(M),存在一个依赖|ψ|C0,1(M)及其他已知信息的常数C,使得

(6)

注1定理 1推广了Guan等[1]的梯度估计.条件(5)可看成一种锥条件[13],有兴趣的读者可参考文献 [9-10，12，22].

1 预备知识

记λ=λ(χu)为χu关于Kähler形式ω的特征根.令σk为一个初等对称函数,其定义为

(7)

方程(1)可等价地写成

(8)

本文中主要结果的关键是下面的引理1.Fang等[9]首先对反σk方程证明这个引理1.Guan[15-16]和Székelyhidi[22]将它推广到更一般的Hessian方程.

引理1假设条件(5)成立,那么存在两个正常数R0,ε>0,使得当|χu|≥R0时,有

(9)

(10)

(11)

2 定理的证明

定理1的证明考虑如下闸函数

φ=Aeη,

其中A和B为两个待定的正常数.

(12)

由假设可知,在p点处有

(13)

约定下文中的计算均在p点处进行.通过计算可知

(14)

由此

(15)

对方程 (8) 进行求导可得

(16)

现在,利用前文中的假设 (4) 得到

(17)

式中的L为方程 (8) 的线性化算子

v∈C2(M).

(18)

简单计算可得

φi=φηi,

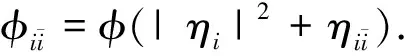
(19)

使用 Cauchy-Schwarz 不等式和假设 (4),得到

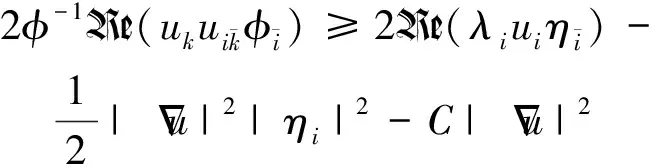
(20)

和

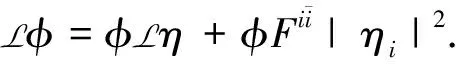
(21)

再由式(13),(15)～(21),有

(22)

(23)

下面,依据引理1来进行讨论.假设 |λ|≤R0,其中R0,ε均为引理 1中的常数,那么存在常数K1>0,使得

是平凡的.

若|λ|≥R0,则由式(4)和引理 1可知

因此,

(24)

不失一般性,可假设λ1≥…≥λn.如果A,B≫1,并且

那么

(25)

为证明式(25),仅需要验证存在某个正数δ>0,使得λn≥δ.

综上可知，如果λi≤λj，则

fjλj≤fiλi,

因而,

(26)

由Cauchy-Schwarz 不等式

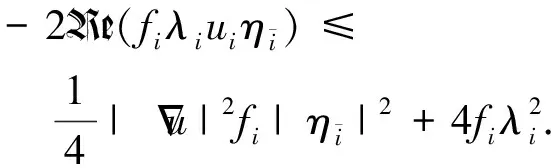
(27)

由式(22),(25),(27) 和引理1可得到定理1梯度估计的证明.

[1] GUAN P F,ZHANG X.Regularity of the geodesic equation in the space of Sasake metrics[J].Adv Math,2012,230:321-371.

[2] YUAN R R.On a class of fully nonlinear elliptic equations containing gradient terms on compact Hermitian manifolds[EB/OL].[2017-03-12].https:∥cms.math.ca/10.4153/CJM-2017-015-9.

[3] AUBIN T.Equations du type Monge-Ampère sur les varietes Kahleriennes compactes[J].Bull Sci Math,1978,102:63-95.

[4] BEDFORD E,TAYLOR B.The Dirichlet problem for a complex Monge-Ampère equation[J].Invent Math,1976,37:1-44.

[5] CAARELLI L A,KOHN J,NIRENBERG L,et al.The Dirichlet problem for nonlinear second-order elliptic equations,Ⅱ.Complex Monge-Ampère,and uniformaly elliptic,equations[J].Comm Pure Appl Math,1985,38:209-252.

[6] YAU S T.On the Ricci curvature of a compact Kahler manifold and the complex Monge-Ampère equation Ⅰ[J].Comm Pure Appl Math,1978,31:339-411.

[7] CHEN X X.A new parabolic ow in Kahler manifolds[J].Comm Anal Geom,2004,12:837-852.

[8] DONALDSON S K.Moment maps and dieomorphisms[J].Asian J Math,1999,3:1-16.

[9] FANG H,LAI M J,MA X N.On a class of fully nonlinear ow in Kahler geometry[J].J Reine Angew Math,2011,653:189-220.

[10] GUAN B,LI Q.The Dirichlet problem for a complex Monge-Ampère type equation on Hermitian manifolds[J].Adv Math,2013,246:351-367.

[11] GUAN B,SUN W.On a class of fully nonlinear elliptic equations on Hermitian manifolds[J].Calc Var Partial Dierential Equations,2015,54:901-916.

[12] SUN W.On a class of fully nonlinear elliptic equations on closed Hermitian manifolds[J].J Geom Anal,2016,26:2459-2473.

[13] SONG J,WEINKOVE B.On the convergence and singularities of the J-flow with applications to the Mabuchi energy[J].Comm Pure Appl Math,2008,61:210-229.

[14] KOMZSIK L.Implicit computational solution of generalized quadratic eigenvalue problems[J].Elsevier Science Publishers B V,2001,37(10):799-810.

[15] SMITH H A,SINGH R K,SORENSEN D C.Formulation and solution of the non-linear,damped eigenvalue problem for skeletal systems[J].International Journal for Numerical Methods in Engineering,1995,38(18):3071-3085.

[16] GUAN B.The Dirichlet problem for fully nonlinear elliptic equations on Riemannian manifolds[EB/OL].[2017-03-12].https:∥arxiv.org/abs/1403.2133.

[17] LI Y Y.Some existence results of fully nonlinear elliptic equations of Monge-Ampère type[J].Comm Pure Appl Math,1990,43:233-271.

[18] URBAS J.Hessian equations on compact Riemannian manifolds[C]∥Nonlinear Problems in Math-ematical Physics and Related Topics Ⅱ.New York:Kluwer/Plenum,2002:367-377.

[19] BLOCKIV.A gradient estimate in the Calabi-Yau theorem[J].Math Ann,2009,344:317-327.

[20] HANANI A.Equations du type de Monge-Ampère sur les varietes hermitiennes compactes[J].J Funct Anal,1996,137:49-75.

[21] HOU Z.Complex Hessian equation on Kahler manifold[J].Int Math Res Not,2009,16:3098-3111.

[22] SZEKELYHIDI G.Fully non-linear elliptic equations on compact Hermitian manifolds[EB/OL].[2017-03-12].https:∥arxiv.org/abs/1501.02762.