Particle image velocimetry measurement of velocity distribution at inlet duct of waterjet self-propelled ship model ＊

Jie Gong (龚杰), Chun-yu Guo(郭春雨), Tie-cheng Wu (吴铁成), Da-gang Zhao (赵大刚)

College of Shipbuilding Engineering, Harbin Engineering University, Harbin 150001, China,

E-mail: gongjie13@hrbeu.edu.cn

Particle image velocimetry measurement of velocity distribution at inlet duct of waterjet self-propelled ship model＊

Jie Gong (龚杰), Chun-yu Guo(郭春雨), Tie-cheng Wu (吴铁成), Da-gang Zhao (赵大刚)

College of Shipbuilding Engineering, Harbin Engineering University, Harbin 150001, China,

E-mail: gongjie13@hrbeu.edu.cn

A vehicle-mounted three-dimensional underwater particle image velocimetry (PIV) device is used in a towing tank to measure the velocity distribution of the inlet duct of a waterjet ship model in a self-propulsion test. The following points are shown through a comparison of the influences of the stationary and free states of the ship model on the measured results: (1) during the test, the ship attitude will change, specifically, the ship model will heave and trim, (2) the degree of freedom disturbs the processing of the pixel images enough to distort the subsequent image processing, (3) the stationary state of the ship model is the optimal mode for measuring the velocity distribution using the PIV device, and (4) if the changes must be considered, the man-made heaving and trimming may be pre-applied, and be made a corrected stationary mode. In addition, the momentum effect coefficient and the energy effect coefficient are calculated in a non-uniform inflowing state, and the related factors affecting the two coefficients are analyzed. The test results show that the pumping action of the waterjet creates a transverse vector in the cross-sectional speed, which increases the non-uniformity of the inflow. These results could help to establish the design requirements for a waterjet-propelled ship type.

Particle image velocimetry (PIV), velocity distribution profile, degree of freedom, transverse vector

Introduction

The waterjet propulsion is used for high-speed ships with an increasing frequency. Compared with a traditional propeller system, the waterjet propulsion is characterized by the high resistance to cavitation, the low appendage resistance, the low noise, the good operability, and the adaptability to a wide range of working conditions[1]. The common types of waterjetpropelled ships include the high-speed catamarans, the hydrofoil craft, the sidewall hoverships, and the trimarans.

In its 20th general meeting, the International Towing Tank Conference (ITTC) organization formed the Specialist Committee on Waterjets, which is committed to the experimental research of waterjetpropelled ships. After research and development for a period of more than a decade, during its 24th generalmeeting, the ITTC standardized a test procedure for the waterjet propulsion, that is, the testing and extrapolation methods for predicting the waterjet propulsive performance of a high-speed marine vehicle[2]. In this test procedure, the momentum flux method is used to determine the propulsive performance of a waterjet propulsor in a ship model. When the waterjet propulsor is in operation, a portion of the inflow is the water flow close to the ship's hull. According to the boundary-layer theory[3], the velocity of the water flow has a large gradient and is lower than the ship's speed[4]. The proportion of the low-speed flow pumped into the propulsor has a great effect on the propulsion system[5]. Therefore, the waterjet propulsion theory introduces the momentum effect and energy effect coefficients, to be used to describe the effects of the non-uniform inflow caused by the ship's hull on the propulsive performance and to analyze the conversion of the system efficiency more accurately in a later stage.

The research results show that the accurate measurement of the velocity distribution at each station in the test procedure is the key to the determination of the propulsive efficiency and the performance of the waterjet propulsion system[6]. Therefore, this is the most important and challenging part of the experimental research. Over the past few decades, there has been continuous development work done on selfpropulsion tests for waterjet propelled ship models[7,8].There are three test methods for measuring the velocity distribution of each station: (1) using a differential pressure transducer, (DPT), (2) the laser Doppler velocimetry (LDV), and (3) the particle image velocimetry (PIV). The DPT is essentially a mechanical measurement technology, whose sensitivity and resolving ability will affect the accuracy of the measurement. The LDV is targeted at a single point in space, and its applications are very limited.The PIV can be used to measure a complex flow field,and to provide rich spatial information and flow characteristics about the flow fields of an entire plane.

These three test methods have been used in a large number of studies in both China and abroad. In its 23rd general meeting, the ITTC selected the scientific survey ship “Athena” of the United States Navy as the prototype for research on a waterjet propulsor and ship hull system[9,10]. In its 24th general meeting, the participant institutions submitted their research results on the self-propulsion test. Park et al.[11,12]conducted experimental research of the pressure distribution of the flow channels of a waterjet propulsor in a wind tunnel without using the water medium, to provide a detail understanding of complicated flow phenomena of a flush type intake duct of waterjet. Kim and Chun[13]conducted experimental research of an axial-flow waterjet propulsor model.During this test, the DPT technology was used to measure the velocity distribution at each station of the waterjet propulsor with the water medium, In addition,the effect of the gap between the blade tip and the tube wall on the propulsive performance of the waterjet propulsor was studied, and results showed that the efficiency difference according to the gap variation(1.5% of diameter and 0.7% of diameter) was about 25% in their overall efficiency. Chun et al.[14]and Kim et al.[15]conducted numerical and experimental researches of a waterjet propulsion system, and applied the waterjet propulsion system to an amphibious tracked vehicle, to analyze the effect of different impeller diameters on its propulsive performance,with the conclusions that the waterjet size should be optimized according to the weight and the size of amphibious military vehicles. Jessup et al.[16]conducted research of the hydrodynamic performance of a joint high-speed sealift (JHSS) ship model with four axial-flow waterjet propulsors. The LDV technology was used to measure the velocity distribution at stations 1, 3, and 6 on the starboard side of the ship model, and together with the wall static pressure waterhead, the technology was also used to measure the pressure distribution at each station. Meanwhile,Jessup conducted research of the power-performance differences between axial and mixed flow waterjets for different ship types, and the results showed that the axial flow waterjet is better suited for high speed ships. The LDV measurement method that he used was subsequently integrated into the test procedure of the ITTC[17]. Wu et al.[18,19]used the two-dimensional PIV and the three-dimensional PIV, respectively, to conduct experimental research of the tip leakage vortex of an axial-flow waterjet propulsion pump blade. Although their research focused on the performance of the impeller blade, it also involved a few important applications of the PIV technology to a waterjet propulsor.

In China, the Marine Design and Research Institute of China (MARIC) has built a comprehensive performance test bench for the waterjet propulsion[20],which can be used to conduct the pump performance tests, the internal characteristics tests, and the reliability tests for both model pumps and real pumps.Chang et al.[21]conducted a bench performance test and a self-propulsion research for a new type of waterjet propulsor. In their self-propulsion research, a numerical simulation was conducted by using the computational fluid dynamics (CFD). With the DTMB5415 ship model as a prototype ship in full scale, Yang et al.[22]conducted comparative research of the noise source distributions and the hydrodynamic performances of the waterjet propulsion and a traditional propeller system, and analyzed the effects of waterjet propulsion on the free surface of the ship's stern. In their studies, numerical computations were used without any actual experiment. In China, selfpropulsion tests for waterjet-propelled ship models also started. However, in view of the rigorous requirements placed on the testing device and the complexity of the analysis and processing of selfpropulsion test data, most of the research results of self-propulsion tests in China were limited to numerical computations.

Judging by the above mentioned research history,a great progress has been made on the self-propulsion tests for waterjet-propelled ship models. Due to the constraints of the PIV equipment and experiment conditions[23,24], experiment researches based on the applications of the PIV equipment were few in the velocity distribution measurement for a waterjet self-propelled ship model in a towing tank. The accurate measurement of the velocity distribution at each station in the test procedure is the key to the calculation of the inlet duct loss coefficient, the outlet nozzle loss coefficient, the pump efficiency and the volume flow rate through the waterjet system.Therefore, this is the most important and challenging part of the experimental research. As one of the most advanced test method, the successful application of the PIV will contribute to the methods for measurements of the velocity distribution. The flow details in the PI V results will g reatly pr omo te the de velopment oftheself-propulsiontestsofthewaterjetpropulsion ship model and the research of the waterjet and hull interactions.

Fig.1 Body plan and side profile of ship model

By applying a vehicle-mounted three-dimensional underwater PIV device in a self-propulsion test of a waterjet self-propelled ship model, this study focuses on the PIV measurements of the velocity distributions at the specified stations, the comparison of the effects of different test states on the measurement results, and the analyses of the mechanism of the influence factors during the measurements. In addition,the momentum effect and energy effect coefficients are calculated in a non-uniform inflow state, the relevant influencing factors are analyzed for the calculated values of the two coefficients, with the flow details of the refined flow field to determine a highreliability measurement methods to complete the first application of the PIV technology to a self-propulsion test of a waterjet self-propelled ship model. The experimental procedure and the relevant conclusions can be applied for similar experiments.

1. Model design and testing device

1.1 Ship hull model

As shown in Figs.1 and 2, the ship hull model is the separate midbody of a high-speed trimaran. Figure 1 shows the body plan and the side profile of the ship model. The midbody is a long and thin ship hull. The ship's bow is fitted with a blade bulbous bow, with torrent threads to generate turbulent flows around the ship's hull. The tail of the ship model has a transom stern, not only to increase the virtual length of the hull to reduce the resistance during a high-speed voyage,but also to facilitate the installation of the waterjet propulsor with a flush inlet duct. The ship's hull is a wooden skeleton, and the shell is made of fiberglass.Table 1 lists the principal dimensions and the KPIs of the ship model.

Fig.2 (Co lor online) Actual pic ture of ship mo del. Surfaces coa- tedwith blackmatte inkcould preventthegeneration of extremely bright reflected light

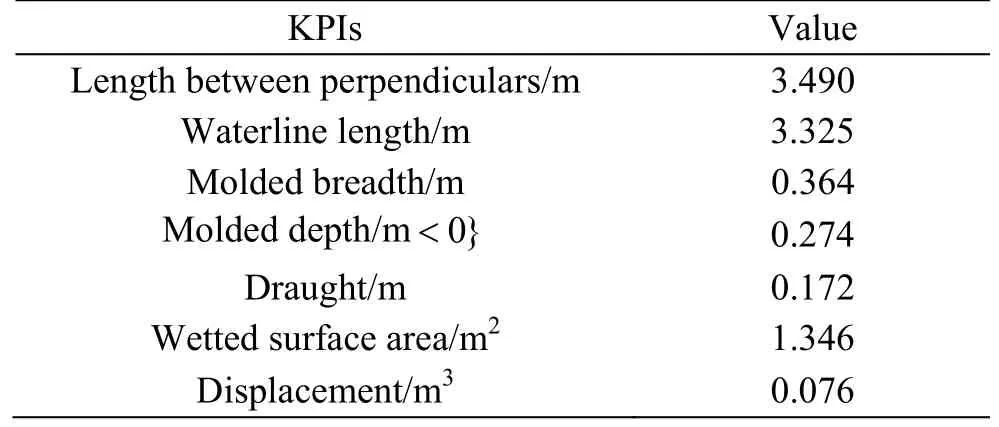
Table 1 KPIs for ship hull model

In the normal operating state, the PIV device emits laser beams to create particle images. To prevent the generation of extremely bright reflected light on the underwater surface of the ship's hull during the data acquisition, the ship's hull area within the view range of the camera is coated with black matte ink.The coating areas for this test include: the area from station 10 to station 20 in the length direction of the ship, the whole stern transom plate, and the nozzle of the waterjet propulsor. For details, refer to the actual picture of the ship model(Fig.2).

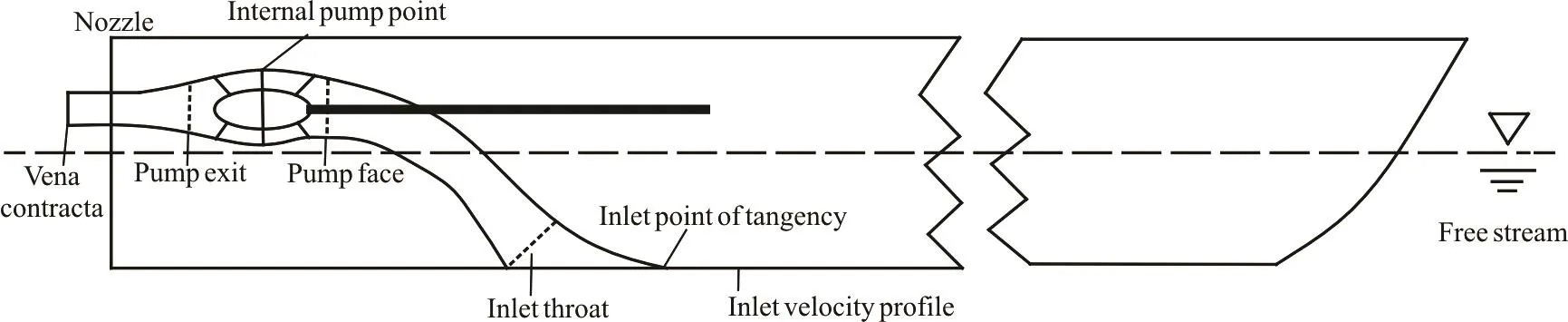
Fig.3 Positions of stations specified in ITTC

1.2Waterjet propulsor model

The whole waterjet propulsor is installed at the tail of the ship model. The tail of the ship model is fitted with a mouth with the same size as the inlet duct.The inlet duct of the waterjet propulsor is connected to the mouth in a watertight way, and the stern transom plate is also fitted with a mouth used for the traverse of the nozzle pipe. As previously mentioned, an accurate measurement of the velocity distribution at each station is vital in the momentum flux method. In accordance with the test regulations of the ITTC[1], the different stations are positioned as shown in Fig.3.

The waterjet propulsor model for the selfpropulsion test has to meet the requirement of the geometric similarity. The inlet duct of the waterjet propulsor is flushed with the surrounding sides. In the manufacturing process, the inlet lip region and the sides of the inlet have to be precisely machined, and the pressure casting is used for the pump flow channel model(Fig.4, Fig.5). The other parts are made with a numerically controlled machine tool[25]. Because the diameters of the impeller and the stator of the waterjet propulsor are less than 150 mm, no rigorous requirements are placed on the machining precision. The propeller shaft is made of stainless steel, and all other parts are made of aluminum. Table 2 lists the main parameters of the waterjet propulsor.

Fig.4(a) Front view of impeller

Fig.4(b) Side view of impeller

Table 2 Main parameters of waterjet propulsor

Fig.4(c) Front view of stator

Fig.4(d) Side view of stator

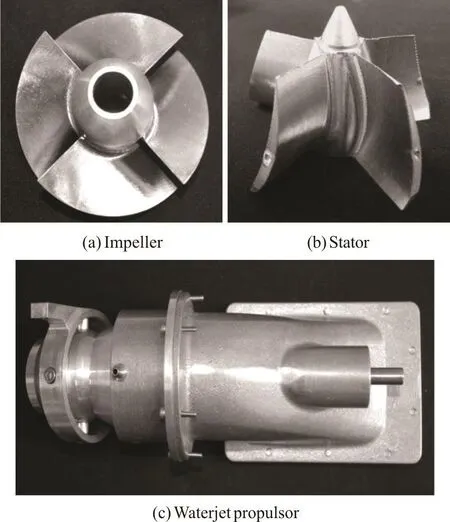
Fig.5 Real pictures of waterjet

1.3PIV device

A vehicle-mounted underwater PIV measurement system is used for the experimental of the waterjet self-propelled ship model. The technical specifications of this system are as follows:

(1) Resolution ratio of CCD camera: 2 048 ×2 048 pixels.

(2) Maximum pulse energy of laser device:1 200 mJ.

(3) Duration of laser beam: 4 ns.

(4) Laser wavelength: 532 nm-1 064 nm.

(5) Thickness of laser film: 0.6 mm.

(6) Measurement area: 400 × 400 mm.

(7) PIV tracing particle: polyamide tracing particle (PSP-50).

Figure 6 shows the system layout of the PIV device.

Fig.6 (Color online) Layout of measuring system

2. Experimental methods

According to the requirements of the flow flux method, the cross section is positioned in the length direction of the ship as follows: 10% forward from the front edge of the inlet duct of the waterjet propulsor(250 mm away from the stern transom plate). Figure 7 shows the specified station 1a and the PIV capture area.

Fig.7 Position of station 1a and PIV capture area

2.1PIV calibration and particle broadcast sowing

Before the measurement process, the PIV device first has to be calibrated. Through the calibration process, the pixel information of the particle images acquired by the CCD cameras is related to the particle-displacement information. After the calibration process is completed, the polyamide tracing particles (PSP-50) are broadcast-sowed in the towing tank. Figure 8 shows the calibration target spot array and the calibrated coordinate system.

Fig.8 (Color online) Calibration target spot array and calibrated coordinate system

2.2 Test procedure

The self-propulsion test is conducted in a towing tank, and the ship model is connected to a trial voyage instrument as shown in Fig.9. In order to evaluate the effects of the degree of freedom of the ship model on the PIV measurement, two test models are used in this experiment, namely a free model (in the conventional test state) and a fixed model (with the degrees of freedom locked in the heaving and trimming directions). The test conditions are as follows. The navigational speedmV is 1.722 m/s (the navigational speed of the corresponding real ship is 20 kn), and the rotating speed of the impeller is 2 000 rpm. The design scheme of the free model is the same as that used for the self-propulsion test of a conventional propeller ship model. Specifically, the degrees of freedom are not restricted in the heaving and trimming directions. The design scheme of the fixed model consists of mounting a bidirectional locking clasp on each connecting rod of the ship bow and the midship.In both states, a force-measuring balance is used to acquire the data on heaving and trimming, and the acquired data are used to analyze the test process.During the test, the run in each state is repeated thrice to verify the repeatability.

2.3 Comparison of experiment data

As previously mentioned, during the entire data acquisition process, cameras 1 and 2 in the underground torpedo body acquire 90 groups of associated double frames of pixel images. The 66th and 70th groups (acquired by camera 2) of pixel images acquired at the moments of A =39.720s and B=40.300s in the free model are selected to compare the heaving and trimming variation amplitudes.As shown in Fig.10, two acquired images are transparently overlapped at the same coordinate to determine the changes in the viewable area (reflection lines of ship's hull). Figure 11 shows a partial enlarged drawing of the transparent overlapping results in the same coordinate. There is an obvious displacement generated by the reflection lines.Actually, the underwater part of the ship's hull undergoes motion changes.

Fig.10 Diagrams of transparent overlapping of 66th and 70th groups of images from acquisition results

According to the heaving and trimming data acquired through the force-measuring balance, the following comparisons are made: (1) effects of heaving value (PIV measurement duration) and heaving variation amplitude on the acquisition process for the two models, (2) effects of trimming value (PIV measurement duration) and trimming variation amplitude on the tests for the two models. Both the trim and heave values are zero as the ship is in hydrostatic condition.

Fig.11 Partial enlarged dra wings of transparent overlapping of the same coordinate

Fig.12 Comparison of heaving values and variation amplitudes between two models

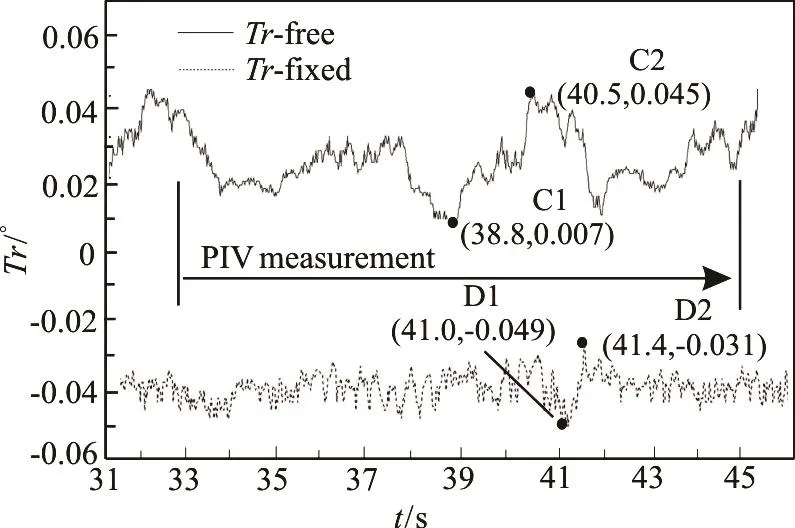
Fig.13 Comparison of trim values and variation amplitudes be- tween two models

Under a test condition, the total duration of the data acquisition is about 50 s. The total duration of the PIV measurement is 12.15 s, therefore, the duration of the PIV measurement is about in the range of the 33rd second to the 45th second in the whole test procedure.In the figure below, the time area indicated by the unidirectional arrow is the duration of the PIV measurement. The total trigger delay is less than 0.5 s.TheX/Ycoordinates in Fig.12 and Fig.13 mean time/heave (t/He) and time/trim (t/Tr) respectively.

For the effects of the heaving value (He) and the heaving variation amplitude, in the fixed model,the average heaving value during the PIV measurement is 0.101 mm, and the peak-valley difference of the heaving value in the total duration of the data acquisition is in the range from -0.077 mm to 0.094 mm.In the free model, the average heaving value during the PIV measurement is -5.141 mm, and the peak-valley difference of the heaving value in the total duration of the data acquisition is in the range from-0.84 mm to 0.722 mm. Evidently, the design scheme of the fixed model has a significant effect on the acquisition process, and the heaving changes are very slight during the PIV measurement. During the test procedure of the free model, the ship's hull obviously sinks, and the heaving value changes periodically.

Figure 13 shows the comparison of the trim values (Tr) and the variation amplitudes between the two models. For the effects of the trimming value and the trimming variation amplitude, in the fixed model, the peak-valley difference of the trimming value in the total duration of the data acquisition is in the range from - 0 .008oto 0 .0 10o. In the free model,theaveragetrimming valueduring thePIV measurement is 0 .029o,and the peak-valley difference of the trimming value in the total duration of the data acquisition is in the range from - 0 .022oto 0 .0 16o. The peak values of the two models appear at the moments of C1, C2, D1, and D2 in the above figure. If the difference between the draught forward and the draught aft is used here to describe the state of the ship model, it is equal to 10 mm according to the formuladr=tanθ·L. There is no evident changes of the ship posture in view of the fact that the heave value is about 0 mm at the same time, so it is sufficient to affirm the fixed state of the ship model.The trimming value of the free mode indicates that the ship model is in a slight trim-by-bow state, and the trimming variation amplitude is in a relatively large range. The heaving and trimming data acquired through the force-measuring balance could provide a secondary proof for the changes in the ship attitude.

3. Results and discussions

3.1Mechanism of the effect of Ship's DOF

According to the degrees of freedom of the ship model, the free model contains certain heaving and trimming changes within the period of data acquisition.The variations in the ship's attitude during the test could have caused changes in the reflective positions of the ship's hull, which is supposed to remain constant during the whole process. As a result, the common portion of the 90 groups of images during the background image calculations would be scattered,and the reflection area of the ship's hull would be remarkably enlarged. In the subsequent process of noise reduction, a subtraction operation is also applied to the real moving particles around the ship's hull.Therefore, the moving particles around the ship hull would disappear, the subtraction operation for the area to be measured would fail to achieve its goal, and the noise-reduction process would distort the picture,making it meaningless to conduct the subsequent processing and analysis.

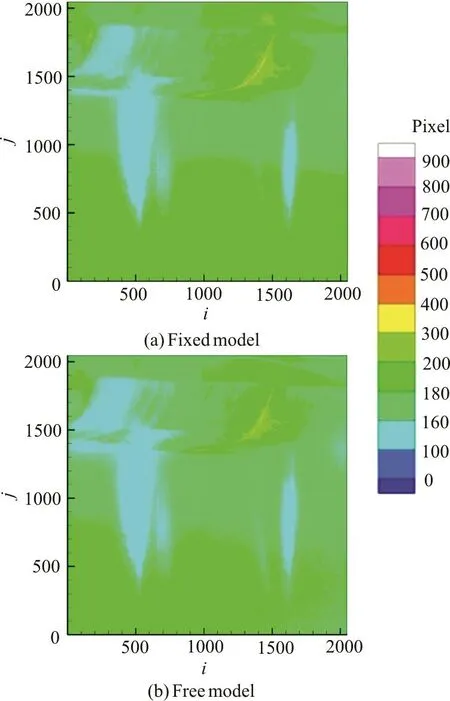
Fig.14 (Color onlinr) Original pixel maps of background ima- ges. i and j mean the selected amount in horizontal and vertical directions, (,)ij means the coordinates of the selected point and the pixel is the reading value of that point

Fig.15 (Color online) Ship model positioning in pixel maps and analysis of pixel values

For the fixed model, the ship model is stationed in a fixed position throughout the test process, and the reflected light generated by the ship's hull throughout the test process appear to be at the same position.Thus, the common portion of the 90 groups of images during the background image calculations only contains the diffraction signals of the reflected light generated by the ship's hull. The real particles around the ship's hull are retained, and subsequent calculations for the particle images give the information of the moving particles around the ship's hull. The following section shows the results of a comparative analysis of the calculation results of the double-frame background images acquired by camera 2 in the two test states. The underwater part of the ship's hull is coated with black matte ink. Thus, the reflected signals could be clearly displayed in a high-resolution map through the color gradation. Figure 14 shows the original pixel maps of the background images for the two models. Figure 15 shows the ship model positioned in the pixel maps, the analysis of the pixel values, and the grey level histograms of the double frames of images.

For the fixed model, the borders of the ship model are clear. The pixel values of 160-180 faithfully reflect the reflected light generated by the underwater part of the ship's hull, and the pixel values up to approximately 600 reflect the real reflective strength after a laser beam has illuminated the ship's hull. For the free model, the ship's attitude varies during the test process. The pixel values of 160-180 reflect the scattering generated by the underwater part of the ship's hull. Thus, the flow field in the dotted-line area around the ship's hull is regarded as the reflected light ge nera ted by it. T he intensity of th e illum ina ted area oftheship'shullisscatteredasaresultofmotions and changes. Thus, the real reflected light is not concen trated, and there are very few pixel points with a pixelvalueof about 600. Figure 16 and Fig.17show the grey-level histograms of two frames of the pixel maps. TheXaxis and theYaxis represent the gray values (Gv) and the number of pixels (N1andN2) for corresponding gray value, respectively.

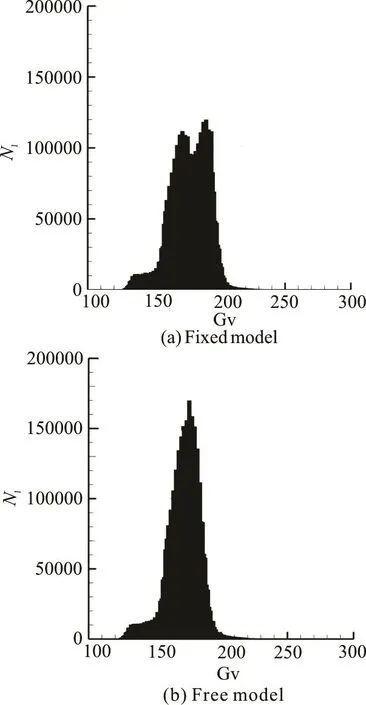
Fig.16 Comparisons of grey level histograms of first frames of pictures

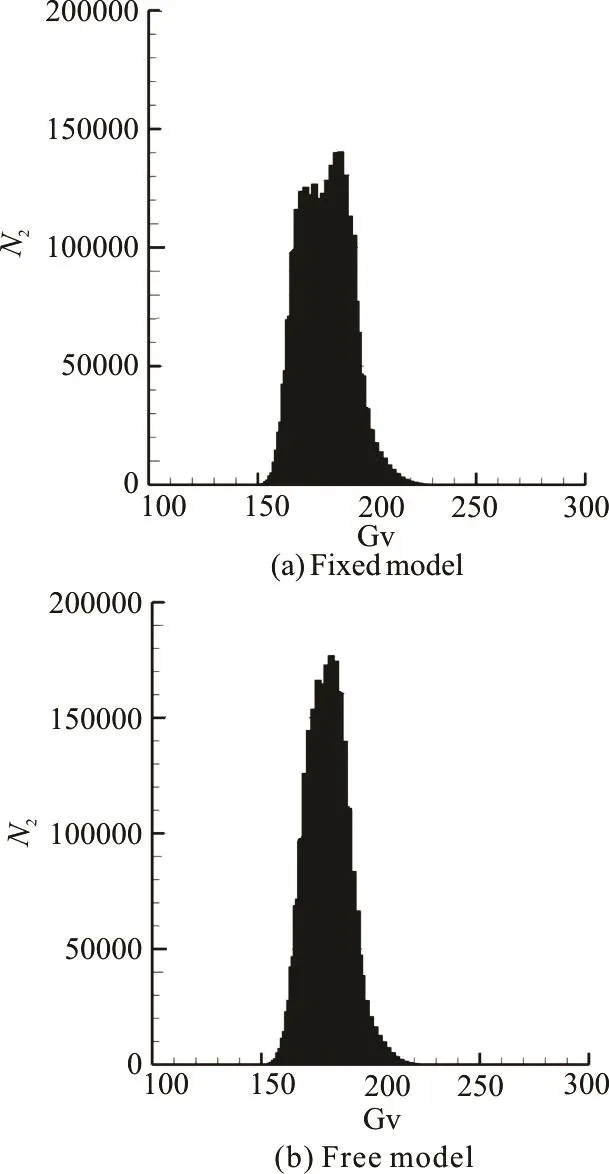
Fig.17 Comparisons of grey level histograms of second framesof pictures

In the first and second frames of the images: (1)when the grey value is in the range of 0-160, the distribution of the pixel points and the distribution pattern in the histogram in the fixed model are basically consistent with those in the free model, (2)when the grey value is in the range of 160-180, the number of pixel points in the fixed model is significantly smaller than that in the free model, an obvious peak clipping occurs in the distribution of the pixel points in the fixed model, and there is a difference of about 40 000 in the peak number of pixel points between the fixed and free models, and (3)when the grey value is in the range of 180-300, the histograms of the pixel values tend to be consistent between the fixed and free models. Overally, the pixel value histogram in the fixed model has a smaller peak value than that in the free model. The basic distribution pattern of the pixel values in the fixed model is basically consistent with that in the free model, and the differential area between the two models is obvious.

To sum up, the degrees of freedom of the ship model during the test procedure affect the test results,and the changes in the ship attitude in the free model are an immediate cause of the expansion of the grey range in the reflection area of the ship's hull in the background images. For the PIV measurements of the velocity distribution at the inlet duct of a waterjet self-propelled ship model, the fixed model will provide more accurate and reliable test results.

In practice, a real navigating ship inevitably faces heaving and trimming variations. In order to ensure that the test conditions are as close to actual conditions as possible, in the test, the variations in the ship attitude must be taken into account. Therefore, manmade heaving and trimming may be pre-applied to the ship model, and then based on these pre-applied values, a corrected fixed model may be used for the experimental research, thus ensuring the reliability of the test results.

3.2Analysis of axial velocity

Among the three measurements for the fixed model, the optimal result is selected for the data analysis. As both the ship model and the waterjet propulsor are installed symmetrically, the test results for the right-half axial field are selected for the data analysis. Th e coordi nate dire ctions are define d as follows: thedirectionfrom theship's sternto itsbow is the positive direction of the axisZ. The direction from the ship's bottom to its deck is the positive direction of the axisY, and the direction from the midship to the starboard is the positive direction of the axisX(as shown in Fig.6). The origin of the coordinates is determined by the position of the cross section.

In the vector diagram of station 1a, the positioning coordinates of the ship's hull are as follows: the lowest point of the ship's bottom in the ship section is at the coordinates (0 mm, 10 mm). The width of the inlet duct of the waterjet propulsor is determined to be from =10 mm to 70 mm. Its vertical position is determined to bey= 10mm , and the central axis of the waterjet propulsor is in the sectionx= 40mm . The profile of the ship's hull in station 1a is obtained from a three-dimensional model.

In Fig.18, the isolines indicate the non-dimensionalized distribution of the axial velocity,Windicates the test value of the axial velocity,Vmindicates the navigational speed of the ship model, and the region of interest is located between the ship's hull and the dot-dash lines. During the motion of the ship's hull, the water flowing around it generates a boundary layer in certain areas on the hull surface due to the viscous effect. As shown in the figure, the interval between the iso-velocity lines in a section with the samexvalue reflects the thickness distribution of the boundary layer in that section. The boundary layer thickness of a turbulent flow with the same speed difference as thexvalue increases from 0 mm to 80 mm.The axial velocity is increased with the distance along the ship's hull, and amounts to about 90% ofVmwhen the vertical position is aty= - 2 0mm . The axial velocity beyond this range is approximately the navigational speed of the ship model.

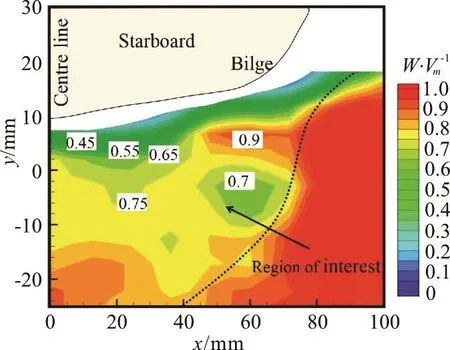
Fig.18 (Color online) Diagram of velocity distribution of inlet duct. The isolines indicate the non-dimensionalized dis- tribution of the axial velocity, the region of interest is located between the ship's hull and the dot-dash lines

The distribution of specific velocity points may be obtained from the test results. Eight subordinate stations (A, B, ··, H) are selected according to the following rules: (1) in the horizontal direction: based on the distance from the central axis, select eight stations (=0 mm, 10 mm, 20 mm, 30 mm, 40 mm,50mm,60mm and 70mm),respectively,corresponding to the dash dot line A, B, C, D, E, F, G,H, (2) in the vertical direction: in the range fromy= 10mm toy=- 2 5mm, select spatial points at intervals of 1 mm and determine their velocity values.Figure 19 shows the horizontal stations selected in the velocity diagram.

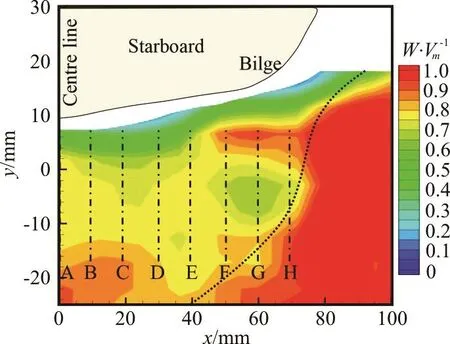
Fig.19 (Color o nlin e) Ei g ht ho rizo ntal s tati ons se lec te d in the velocitydiagram.DashdotlinesA,B,C,D,E, F, G, H correspondto 20 mm,30 mm, 40 mm, 50 mm, 60 mm and 70 mm, respectively

Fig.20 Distribution curves of axial velocity

Figure 20 shows the results for the velocity points. According to the distributions of the axial velocity at the coordinates from =0 mm to 30 mm,the overall trends are approximately the same. In the depth range fromy= 10 mm to 0 mm, the axial velocity rapidly increases to about 75% of the navigational speed. When the depth is less thany=0mm, the increase in the axial velocity slows down,when the depth is in the range fromy= -5 mm to-10 mm, the axial velocity decreases to a certain extent (the following section will analyze this phenomenon). When the depth is lower thany= - 10 m m , the axial velocity slowly increases,when the depth isy= -2 5mm , the axial velocity is equal to about 85% ofVmand becomes stable.

In the range from =40 mm to 70 mm, one sees differences in the trends of the four distribution curves for the axial velocity. At the depthy= 10mm ,the axial velocity is very high, specifically, more than 60% ofVm. This indicates that the draught of the ship shell in this area gradually decreases, and the low-velocity flow area is above the depthy= 10mm ,providing a corroborative evidence for the positioning of the ship's hull in the measurement results. In the depth range fromy= 10 mm to 5 mm, high-velocity areas appear in three sections: =50 mm, 60 mm,and 70 mm. In the depth range fromy= 0 mm to-10 mm, one sees a certain decrease in the axial velocity in four sections. Finally, the axial velocity of the four sections is approximately 90% ofVmat the depthy= -2 5mm .

3.3Analysis of vectors and streamlines

In the measurement section, within the width range from =0 mm to 70 mm of the inlet duct of the waterjet propulsor, most vectors are in the upward direction and tend to approach the central axis of the inlet duct. The water flows outside the shipboard move to the ship bottom due to the pumping effect. In the distribution curve of the axial velocity, there is an area in which the axial velocity decreases. This explains why the axial velocity in Section 3.2 is lower.Meanwhile, the data show that a more obvious trend for the decrease in the axial velocity is associated with a larger axial vector component.

The streamline results are based on the vector analysis. Because of the pumping effect of the waterjet propulsor, the water flows within a certain depth range generate an obvious flowing in the oblique upward direction. The generation of a transverse vector proves that the waterjet propulsor affects the axial flows around the ship's hull. The streamlines inside the round frame in Fig.21 show that an obvious flow separation phenomenon is produced in the outside area of the ship's hull at the widthx= 80mm .This determines the influence area of the pumping effect from an experimental viewpoint.

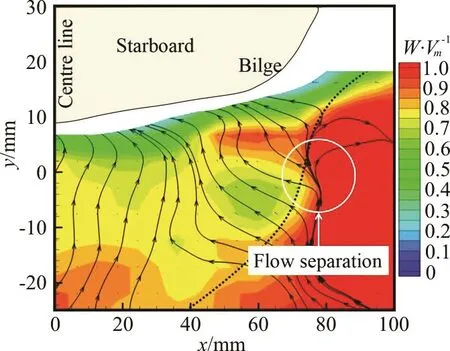
Fig.21 (Color online) Velocity vector and streamline chart. Wa- ter flows within a certain depth range generate obvious flowing in the oblique upward direction, and an obvious flow separation phenomenon is shown with white circle

3.4Momentum effect coefficient and kinetic ener- gy effect coefficient β

The above test results may be used to calculate and . The calculation process is as follows:Vmis equal to 1.722 m/s, and the rotating speed of the impeller is 2 000 rpm. According to the waterjet propulsion theory, the effective flow layer thicknessY0and the effective inflow widthW0must be determined before calculating and (Fig.22).

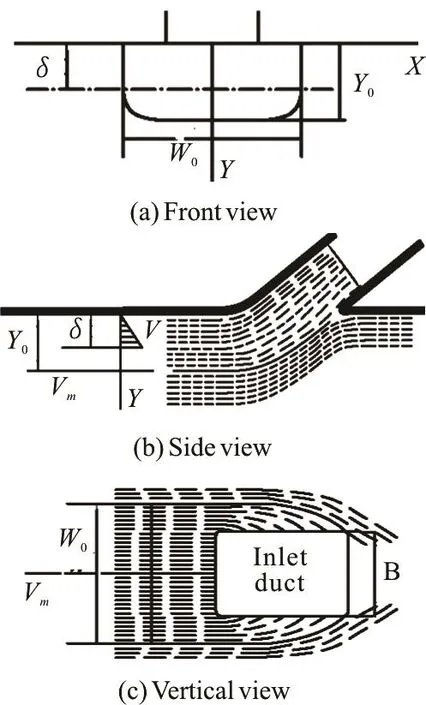
Fig. 22 Diagrams of inflow at inlet duct. Three pictures are, res- pectively, (a) Front view, (b) Side view and (c) Vertical view of the capture area in station 1a. δ stands for the boundary layer thickness calculated. stands for the effective flow layer thickness. stands for the effec- tive inflow width

The determination processcombinesthe empiricalformula and theinstallation of an experiment model. On the determination ofY0,previous studies pointed out that high accuracy is not necessary for the capture area, and the region of station 1a is insensitive to a minor variation in the area shape. So the selection of the empirical formula is both reasonable and in accordance with the calculation accuracy requirements. As the inlet geometry is a flush type intake duct, the corresponding empirical formulaY0=(1/3)for the flush type inlet is selected in the calculation.A1stands for the throat area of the waterjet propulsor. If the diameter of the inlet duct throatD1=0.06m, the throat area of the waterjet propulsor isA1=(π/4)=2.83× 1 0-3m2,and the effective flow layer thickness isY=1.77× 1 0-2m .

0

On the determination ofW0, the effective inflow widthW0should be 120%-150% of the intake duct width according to the experimental experience.However, due to the installed waterjet model, the distance from the inner edge of the inlet duct to the ship center is equal to 10 mm, so for the inner side of the capture area, the ship center line is selected in view of the symmetry of the flow field. There is no doubt that the width center line should be overlapped with the center axis of the inlet duct, naturally, the outer line should be in the positionx= 80mm. The inflow half-width of the inlet duct in the ship bottom is 0.04 m, and the capture area calculated is a semi-ellipse.

As the capture area calculated is a semi-ellipse,the capture area could be described with

The full width of the inlet duct is divided into six equal parts, so theYvalue of each equal part is determined by this formula

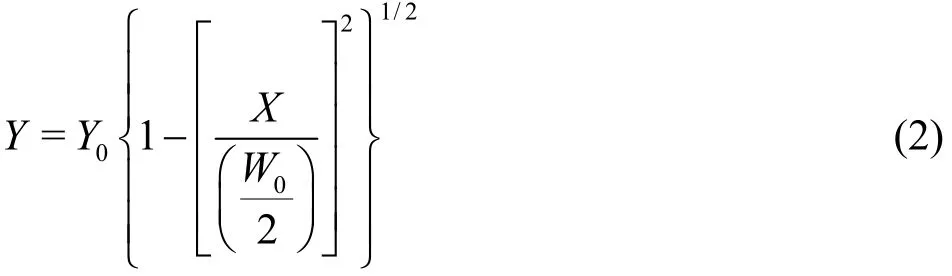
The calculation of the boundary layer thicknessδis based on the plate boundary layer theory[4]. The distance from the front end of the inlet duct to the bow waterline =3.2ml. Then

so the water flows around the ship hull in the inlet duct are turbulent flows asRe＞Reec=5×105, and the boundary layer thickness is calculated according to the plate turbulent boundary layer formula. Before solving the momentum integral equation, the velocity distribution is supposed to obey an exponential distribution

and the formulaδ=0.382lRe-1/5is used in the calculation of the boundary layer thickness. Finally the boundary layer thickness is 0.054 m.

Figure 23 shows the measured velocity profile and the assuming velocity distribution. Comparing the measurement results and the theory distribution, it is seen that most measured points are lower than the theoretical distribution line, however, their dispersion tendencies are in agreements.

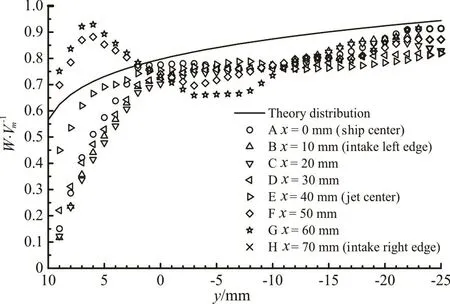
Fig.23 Comparison between the measurement velocity profiles and the assuming theory distribution

In order to evaluate the simulation accuracy of the exponential distribution, the exponential curve fitting process is applied to the discrete velocity in different positions. Meanwhile, the coefficient of determinationR2is calculated for the evaluation of fitting. Generally,0≤R2≤1, the best fitting effect is achieved whenR2=1. It can be noticed from Fig.24 thatR2of the theoretical distribution is equal to unity. Figure 24 shows theR2values calculated from the velocity data in eight positions. The velocity distribution fits well in regions near the jet center, but poorly in a small part around the bilge of the ship model. It implies that the assumption of the exponential distribution is reasonable for the velocity distri-bution in the capture area, but not completely. Meanwhile, this process could offer a proof for the nonuniform velocity distribution in the capture area quantitatively.

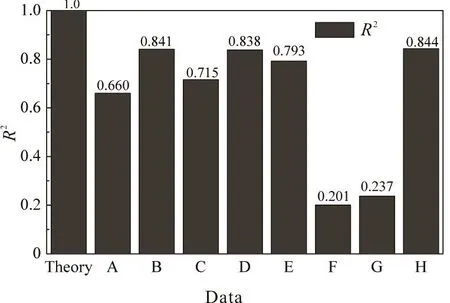
Fig.24 Histogram of R2 after exponential curve fitting pro- cess in eight stations

Evidently,0Y＜ , indicating that the effective inflow areas are all within the turbulent boundary layer of the interior zone. It is shown that the more the boundary-layer low-velocity flows are pumped, the lower the propulsion coefficient[5,13]. Evidently, the boundary-layer effect calculated under those conditions has a great effect upon the propulsive performance. The baseline of the elliptical area is positioned at the positiony= 10mm , and the trapezoidal method is used to conduct the numerical integration for each equal part of the inlet duct. After the numerical integration of each cross section is complete, the Simpson method is used for the numerical integration along the width direction of the inlet duct.Figure 25 shows that the area in the semi-ellipse line is the capture area calculated.

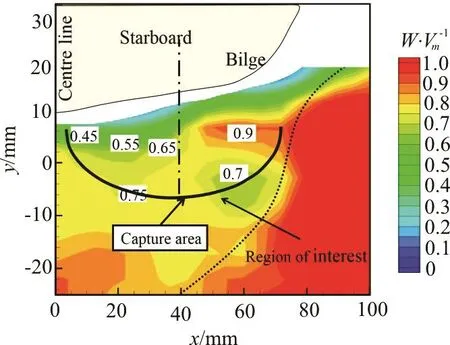
Fig.25 (Color online) Capture area outline at inlet duct

Finally, and are calculated as follows:

According to the above data processing procedures, the other two groups of the data are processed.Figure 26 is the comparison of the velocity distribution in 3 positions at 3 instants (T1, T2 and T3).There is a difference between the results shown in 3 reading positions but it is acceptable as far as the calculation results are concerned. and of three groups are 0.716, 0.725, 0.733 and 0.532, 0.535,0.523 in 3 instants, respectively. The above calculation results show favourable repeatability for the experiment.

Fig.26 Comparisons of velocity distribution in 3 instants (T1, T2 and T3)

It is obviously that the two parameter values do not meet the design requirements for a conventional waterjet-propelled ship, but they do basically show the experiential relationship2βα≈. In addition, the calculation results are consistent with the actual conditions. The and values of a normal waterjetpropelled high-performance ship under the design condition are about 0.90 and 0.80, respectively[13]. The and β values calculated in this test are a little different from these values, but are within a reasonable range. As for the waterjet and hull matching: the waterjet propulsor does not match the ship's hull well.The inlet duct size, the area of the inflow throat and the inflow segment could affect these two values. The waterjet propulsor is installed at a relatively rear position, which affects the distribution of the boundary layer thickness of the measurement station.On the other hand, the molded lines from the midship to the ship stern are changed significantly (in the initial design, the propulsion type is a traditional propeller). In an ideal design, the propeller disk surface has a good wake area at the ship stern, located below the inlet duct of the waterjet propulsor. As for the operating conditons: in the calculation of and β values , the rotating speed should reach the corresponding speed self-propelled point,and the rotating speed 2 000 rpm could not satisfy the demand asmV is equal to 1.722 m/s, the standard rotating speed should be larger than 2 000 rpm, so it is reasonal that and values are smaller than those under the design condition. To sum up, the inlet duct velocity distribution is successfully measured for the fixed model. Because of the pumping effect at the inlet duct, a transverse vector is generated in the measurement station. The non-uniform inflows around the ship's hull are more significant, and the boundary layer becomes thinner. The accuracy of the measured results could be confirmed by the calculation of the momentum effect coefficients and . This paper provides some ideal experiment results.

4. Discussions

A reasonable match between the propulsor and the ship hull and a scientific installation position for the propulsor are of vital importance to reduce the influences of the ship's hull on the propulsive performance and improve the efficiency of the propulsion system. The proposed waterjet-propelled ship type should be designed to have a stern that allows a more uniform distribution of the inflow velocity in the inflowing section, which should serve as an evaluation index for the design quality.

The way to verify the accuracy of the measured results by the calculation of the momentum effect coefficient and the kinetic energy effect coefficient cannot provide an absolute guarantee for the accuracy or the validity of the measured results. As the first application of such tests and the ship model, there is no similar test results in China and abroad for comparison. But from the experiment repeatability of the test results, a certain guarantee precision is secured.The current PIV testing methods have no way to complete all specified stations' velocity distribution measurement in the ITTC procedures, including several internal stations. In-depth development is the measurement of the velocity distribution for these internal stations.

5. Conclusions

By first applying a PIV device to the measurement of the velocity distribution at the inlet duct of a waterjet self-propelled ship model, this study investigates the mechanism of how the changes in the ship attitude affect the measurement results during the measurement process. The conclusion is that a fixed model is in the optimal test state for the experimental research based on the pixel information of pixel maps combined with the data monitored in the force-measuring balance. This conclusion gives some insight for similar experiments.

Experimental methods have successfully obtained some ideal test results. If the changes of the ship attitude are considered in a similar experiment,the man-made heaving and trimming may be preapplied to the ship model. Based on the pre-applied values, a corrected fixed model may be used for the experimental research.

The test has obtained ideal experiment results.The axial velocity distribution, the details of the flow,and the calculated critical parameters can be used as references for further researches. Finally, the effects of the ship type and the capture area shape are analyzed on the propulsive efficiency of a waterjet propulsor, and the proposed design requirements for a waterjet-propelled ship type. These points will also be of certain reference value.

Acknowledgements

This work was supported by the Basic Research Project Titled “Multihull Ship Technology Key Laboratory of Fundamental Science for National Defense” carried out by Harbin Engineering University, the author conveys sincere gratitude to the participant members of the towing tank laboratory.

[1] Van Terwisga T., Hoyt J. G., Zangeneh M. et al. Report of specialist committee on validation of waterjet test procedures [C]. Proceedings 23rd International Towing Tank Conference. Venice, Italy, 2002.

[2] Takai T., Kandasamy M., Stern F. Verification and validation study of URANS simulations for an axial waterjet propelled large high-speed ship [J]. Journal of Marine Science and Technology, 2011, 16(4): 434-447.

[3] Newman J. N. Marine hydrodynamics [M]. Boston, USA:MIT Press, 1977, 175-193.

[4] Pope S. B. Turbulent flows [M]. Cambridge, UK: Cambridge University Press, 2010, 121-150.

[5] Allison J. L. Marine waterjet propulsion [J]. SNAME Transactions, 1993, 101: 275-335.

[6] Van Terwisga T. Waterjet-hull interaction [D]. Doctoral Thesis, Delft, The Netherlands: Delft Technical University,1996.

[7] Falchi M., Felli M., Grizzi S. et al. SPIV measurements around the DELFT 372 catamaran in steady drift [J].Experiments in Fluids, 2014, 55(11):1-20.

[8] Kim W. J., Van S. H., Kim D. H. Measurement of flows around modern commercial ship models [J]. Experiments in Fluids, 2001, 31: 567-578.

[9] ITTC 2008. Propulsion committee, final report and recommendation to the 25th [C]. Proceedings of the 25th ITTC.Fukuoka, Japan, 2008.

[10] Gao Z. L., Vassalos D. The dynamics of the floodwater and the damaged ship in waves [J]. Journal of Hydrodynamics, 2015, 27(5): 689-695.

[11] Park W. G., Janga J. H., Chunb H. H. et al. Numerical flow and performance analysis of waterjet propulsion system [J].OceanEngineering,2005,32(14-15):1740-1761.

[12] Park W. G., Yun H. S., Chun H. H. et al. Numerical flow simulation of flush type intake duct of waterjet [J]. Ocean Engineering, 2005, 32(17-18): 2107-2120.

[13] Kim M. C., Chun H. H. Experimental investigation into the performance of the axial-flow-type waterjet according to the variation of impeller tip clearance [J]. Ocean Engineering, 2007, 34(2): 275-283.

[14] Chun H. H, Ahn B. H., Cha S. M. Experiment and analysis on the waterjet propulsion system of the tracked vehicle[C]. Proceedings of Spring Meeting of Society of Naval Architect of Korea. Seoul, Korea, 2001.

[15] Kim M. C., Chun H. H., Kim H. Y. et al. Comparison of waterjet performance in tracked vehicles by impeller diameter [J]. Ocean Engineering, 2009, 36(17-18):1438-1445.

[16] Jessup S., Donnelly M., Fry D. et al. Performance analysis of a four waterjet propulsion system for large sealift ship[C]. 27th Symposium on Naval Hydrodynamics. Seoul,Korea, 2008.

[17] Lu L., Pan G., Wei J. et al. Numerical simulation of tip clearance impact on a pumpjet propulsor [J]. International Journal of Naval Architecture and Ocean Engineering,2016 , 8(3): 219-227.

[18] Wu H., Tan D., Miorini R. L. et al. Three-dimensional flow structures and associated turbulence in the tip region of a waterjet pump rotor blade [J]. Experiments in Fluids,2011, 51(6): 1721-1737.

[19] Wu H., Miorini R. L., Katz J. Measurements of the tip leakage vortex structures and turbulence in the meridional plane of an axial water-jet pump [J]. Experiments in Fluids, 2011, 50(4): 989-1003.

[20] Li G. Q. Design on auto-measure system for the compositive test station of the propulsive water-jet pump [D].Master Thesis, Shanghai, China: Shanghai Jiao Tong University, 2007(in Chinese).

[21] Chang S. P., Wang Y. S., Ding J. M. Performance test and numerical calculation of a mixed-flow waterjet [J].Journal of Harbin Engineering University, 2012, 33(5):660-664(in Chinese).

[22] Yang Q., Wang Y., Zhang Z. Numerical prediction of the fluctuating noise source of waterjet in full scale [J].Journal of Marine Science and Technology, 2014, 19(4):510-527.

[23] Theunissen R. Adaptive image interrogation for PIV [D].Doctoral Thesis, Brussel, Belgium: Vrije Universiteit Brussel, 2009.

[24] Scarano F., Riethmuller M. L. Iterative multigrid approach in PIV image processing with discrete window offset [J].Experiments in Fluids, 1991, 26(6): 513-523.

[25] Sun Y., Su Y., Wang X. et al. Experimental and numerical analyses of hydrodynamic performance of propeller boss cap fins in propeller-rudder system [J]. Engineering Applications of Computational Fluid Mechanics, 2016, 10(1):145-159.

July 8, 2015, Revised March 7, 2016)

* Project supported by the National Natural Science Foundation of China (Grant Nos. 51379043, 51209048 and 51409063).

Biography: Jie Gong (1991-), Male, Ph. D. Candidate

Chun-yu Guo,

E-mail: guochunyu_heu@outlook.com