不动点和一类非线性随机动力系统的稳定性

王春生，丁 红

(1.广州大学华软软件学院 管理系，广东 广州 510990;2.广州大学 公共管理学院，广东 广州 510006)

不动点和一类非线性随机动力系统的稳定性

王春生1，丁 红2

(1.广州大学华软软件学院 管理系，广东 广州 510990;2.广州大学 公共管理学院，广东 广州 510006)

考虑了一类变时滞非线性中立型随机动力系统，给出了确保其零解均方渐近稳定性条件.这些条件不需要时滞有界，也不要求系统的系数函数符号固定.给出的均方渐进稳定性定理一定程度上推广和改进了相关文献的结果.

非线性中立型随机动力系统；不动点; 变时滞;均方渐近稳定

目前很多专家和学者都选择采用不动点方法研究随机动力系统的稳定性，得到了很优异的结果.如文献[1-6]利用不动点方法研究过随机动力系统零解的存在性、周期性、有界性和稳定性，文献[7-12]也采用不动点方法研究过多种类型的随机动力系统的稳定性.作为此项研究的推广，本文将研究具有变时滞非线性中立型随机动力系统的均方渐近稳定性，以期推广和改进相关文献结果.

1 主要结果

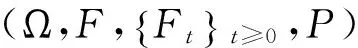
考虑以下变时滞非线性中立型随机系统

d[x(t)-G(t,x(t-τ(t))]=[a(t)x(t)+f(t,x(t-τ(t)))]dt+g(t,x(t),x(t-δ(t)))dw(t)

(1)

其中当s∈[m(0),0]时，x(s)=φ(s)，φ∈C([m(0),0],R),a(t)∈C(R+,R),τ(t),δ(t)∈C(R+,R+) 满足：当t→时，t-τ(t)→和t-δ(t)→.m(0)=min{inf(s-τ(s),s≥0), inf(s-δ(s),s≥0)}.

同时，G(t,x)∈C(R+×R,R)，f(x)∈C(R+×R,R)，g(x,y)∈C(R+×R×R,R)和存在一个q(t),c(t),e(t)∈C(R+,R) 使得

定理1 假设τ(t) 可导且存在常数α∈(0,1)和连续函数h(t):[0,)→R,使得当t≥0时,

(i) liminft→h(s)ds>-;

则，系统(1) 的零解均方渐进稳定当且仅当t→时,

证明 定义S为所有F-适应过程φ(t,ω):[m(0),)×Ω→R所构成的Banach 空间，且对固定的ω∈Ω，φ(t,ω)关于t几乎处处连续，其初值ψ(s,ω)=φ(s).我们定义当t→时,

定义算子π:S→S满足：当t∈[m(0),0]时，(πx)(t)=φ(t).当t≥0时

(1)π在[0,)上均方连续.

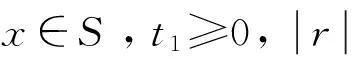
易证当

(2)π(S)⊂ S.

当t→时，t-δ(t)→且E|x(t)|2→0，E|x(t-δ(t))|2→0.对任意ε>0，存在T1>0使得当t≥T1时，E|x(s)|2<ε和E|x(s-δ(s))|2<ε.所以有

类似可证当t→时所以有π(S)⊂S.

(3)π是压缩映射.

由条件(ii)可知，存在常数L>0和β满足α2<β<1使得

(3)

对任意x,y∈S有

为了证明渐近稳定性，需要证明方程(1)的零解是均方稳定的.假设任意的ε>0 和δ>0 (δ<ε) 满足

其中L来自公式(3).如果x(t)=x(t,0,φ(0))是方程(1)的解，满足： E|φ|2<δ且x(t)=(πx)(t).下面证明对所有的t≥0有E|x(t)|2<ε.

这与t*的定义相违背.这说明，如果条件(iii)成立，方程 (1)的零解均方渐近稳定.

由公式 (2) 和x(t)=(πx)(t)知，对所有的t≥tk,

然而，

(4)

另一方面，如果方程(1)的零解均方渐近稳定，则当t→时，E|x(t)|2=E|x(t,tk,φ)|2→0.

因为当n→时，tn-τ(tn)→，由条件(ii)知，当n→时，

这与公式(4)相违背.所以条件(iii)是方程(1)零解均方渐近稳定的必要条件.故得证.

2 实例

d[x(t)-0.1x(t-τ)]=-x(t)dt+ex(t-τ)dω(t)

(5)

很显然，在定理1中可取q(t)=0.1,a(t)=-1,b(t)=0,e(t)=1，如果选择h(t)≡1，

则由定理1可知，系统 (5) 的零解均方渐进稳定.

[1]BURTON T A. Fixed points and stability of a nonconvolution equation[J].Proceedings of the American Mathematical Society,2004,132:3 679-3 687.

[2] BURTON T A , FURUMOCHI T. A note on stability by Schauder's theorem[J].Funkcialaj Ekvacioj,2001,44:73-82.

[3] BURTON T A , FURUMOCHI T. Fixed points and problems in stability theory[J].Dynamical Systems and Applications, 2001,10:89-116.

[4] RAFFOUL Y N. Stability in neutral nonlinear differential equations with functional delays using fixed point theory[J].Mathematical and Computer Modelling,2004,40:691-700.

[5] LUO J W. Fixed points and stability of neutral stochastic delay differential equations[J].Journal of Mathematical Analysis and Applications,2007,334:431-440.

[6] MAO X R. Exponential stability for stochastic differential delay equations in Hilbert spaces[J]. Quarterly J.Math, Oxford(2), 1991,42：77-85.

[7] 王春生. Fixed points and stability of stochastic integro-differential equations(英文版)[J]. 广州大学学报(自然科学版),2009,8(2):49-52.

[8] 王春生，李永明.中立型多变时滞随机微分方程的稳定性[J].山东大学学报(理学版),2015, 50(5):82-87.

[9]王春生. 中立型随机积分微分方程的稳定性[J].四川理工学院学报(自然科学版),2011，24(1)：81-84.

[10]王春生.随机微分方程稳定性的两种不动点方法的比较[J].四川理工学院学报(自然科学版),2012，25(4)：81-84.

[11] 王春生. 不动点与非卷积型随机Vollterra微分方程的稳定性[J].荆楚理工学院学报,2011，26(2)：30-33.

[12]王春生. Volterra型积分微分动力系统的稳定性 [J].湖北文理学院学报,2016，37(8)：5-7.

(编辑：郝秀清)

Fixed point and stability of a kind of stochastic nonlinear system

WANG Chun-sheng1,DING Hong2

(1.Management Department,South China Institute of Software Engineering, Guangzhou University, Guangzhou 510990,China;2.School of Public Administration,Guangzhou University,Guangzhou 510006,China)

In this paper，we consider a nonlinear neutral stochastic system with variable delays and give conditions to ensure that the zero solution is asymptotically mean square stable by means of fixed point theory. These conditions do not require time delays, and do not require the system to be fixed. An asymptotically mean square stable theorem with necessary and sufficient condition is proved. Some well-known results are improved and generalized.

nonlinear neutral stochastic system; fixed point; variable delays; mean square stable

2016-07-02

广东省自然科学基金项目(2016A030313542)；广东省普通高校青年创新人才项目(自然科学)(2015KQNCX200)；广州大学华软软件学院教学、科学研究项目(ky201402)

王春生,男，paperspring@163.com； 通信作者: 丁红，女，182377757@163.com

1672-6197(2017)05-0024-05

O231.3

A