一类捕食者染病的捕食者-食饵系统的随机动力学行为

冯 涛，孟新柱

(山东科技大学 数学与系统科学学院，山东 青岛 266590)

一类捕食者染病的捕食者-食饵系统的随机动力学行为

冯 涛，孟新柱

(山东科技大学 数学与系统科学学院，山东 青岛 266590)

考虑了一类具有Beddington-DeAngelis功能性反应和Lévy跳的捕食者染病的捕食者-食饵系统的动力学行为。利用Lyapunov方法和伊藤公式，本文讨论了系统全局正解的存在唯一性；研究了随机系统在其确定性模型的平衡点周围的长时间行为。研究结果表明，在一定条件下，随机系统的解会在其确定性系统的平衡点周围波动，且波动的幅度与随机系统所受干扰的强度呈正相关。最后，本文运用Matlab数值模拟对前述理论进行了验证。

捕食者-食饵系统；Beddington-DeAngelis功能性反应；渐近行为；随机扰动；Lévy跳

近年来，随机干扰对生态系统的影响逐渐成为生物数学领域的研究热点[1-6]。在生态系统中，捕食者和食饵之间的相互作用经常受到一些环境因素的影响，例如海啸、水源、地震、火山、疾病等。许多学者研究了传染病对捕食者-食饵种群的影响[7-13]和环境干扰对捕食者-食饵种群的影响[14-17]。研究传染病和环境噪声对捕食者-食饵种群的影响具有重要的生物学意义。本文结合传染病与环境噪声对生物种群的影响，研究了一类带有Beddington-DeAngelis功能性反应和Lévy跳的捕食者染病的捕食者-食饵系统的动力学行为。

1 数学模型

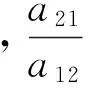
文献[13]研究了如下具有Beddington-DeAngelis功能性反应和捕食者染病的捕食者-食饵系统：

(1)

令

实际上，生态系统中的物种可能会遭受到一些较大的不确定因素的干扰，比如火山喷发、地震、海啸和气候骤变等，一般把这类较大的干扰因素称为有色噪声干扰，数学上使用Lévy跳表示。考虑这类有色噪声干扰后，模型(1)变为：

(2)

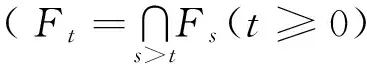
2 全局正解的存在唯一性

本文的研究对象为生物种群，由于负数解不存在生物学意义，因此本节首先证明模型(2)存在全局唯一正解。

假设 2.1 假定以下条件成立[15]：

(i) 1+γi(u)>0，

其中，i=1,2,3，这两个假设意味着Lévy噪声的强度不会无限大。

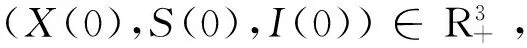
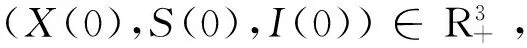
证明由引理2.1可知，只需要证明τe=∞。定义一个充分大的正常数k0，使得

定义停时

(3)

V(X,S,I)=X-1-lnX+S-1-lnS+I-1-lnI。

(4)

由伊藤公式得

(5)

其中

(6)

其中，H0>0是常数。

对方程(5)的两端从0到τk∧N积分并取期望，得

(7)

(8)

结合方程(7)和方程(8)，得

其中1nk 是Ωk的指标函数。令k→+∞，得

+∞≥V(X(0),S(0),I(0))+H0N≥+∞。

显然与假设矛盾。故τ∞=∞。

3 模型(2)解的渐近性质

其中

证明定义

由伊藤公式得

(9)

其中

(10)

对方程(9)两端由0到t积分并取期望，得

(11)

对方程(11)两端同时除以t，令t→∞并取上界，得

证毕。

推论 3.1当σ1,γ1=0时，模型(2)等同于模型(1)。由定理3.1中(10)可知，此时

所以模型(1)的平衡点E1(K,0,0)是全局渐近稳定的。

注 3.1由定理3.1可知，如果干扰强度σ1,γ1足够小，模型(2)的解会在模型(1)的边界平衡点E1(K,0,0)周围震荡，且震荡的幅度与干扰的强度呈正相关。

其中

定义

由伊藤公式得

(12)

其中

同理可得

(13)

其中

同理

(14)

结合方程(12)～(14)，得

(15)

其中

(16)

对方程(15)两端由0到t积分并取期望，得

(17)

对方程(17)两端同时除以t，令t→∞并取上界，得

证明完毕。

推论3.2当σi,γi=0(i=1,2)时，模型(2)等同于模型(1)。由定理3.2中方程(16)知，此时

其中

证明由于(X*,S*,I*)是系统(1)的正平衡点，所以

定义

由伊藤公式得

(18)

其中

同理可得

(19)

其中

同理

(20)

其中

结合方程(18)～(20)，得

(21)

其中

对方程(21)两端由0到t积分并取期望，得

(22)

对方程(22)两端同时除以t，令t→∞并取上界，得

证明完毕。

推论 3.3当σi,γi=0(i=1,2,3)时，模型(2)等同于模型(1)。由定理3.3知，此时

所以模型(1)的正平衡点E3(X*,S*,I*)是全局渐近稳定的。

注 3.3由定理3.3知，如果干扰强度σi,γi=0(i=1,2,3)足够小，模型(2)的解会在模型(1)的正平衡点E3(X*,S*,I*)周围震荡，且震荡的幅度与干扰的强度呈正相关。

4 数值仿真

利用欧拉法和Matlab2014b[13]，本文进行了数值模拟以支持获得的结果。数值仿真中，使用以下参数：

初始值X(0)=3,S(0)=2,I(0)=2，p=q=1，Ζ=(0,+∞)，λ(Ζ)=1，步长Δt=0.001。

在图1中，b1=0.6，b2=0.4，b3=0.2，a11=0.5，a12=0.6，a21=0.3，a22=0.1，a33=0.1，β=0.2。图1(a)为确定性模型(1)的时间序列图，(b)-(d)为随机模型(2)的时间序列图，干扰强度分别取:(b)σi=0.05,γi=0.1，(c)σi=0.05,γi=0.15，(d)σi=0.05,γi=0.2，其中i=1,2,3。

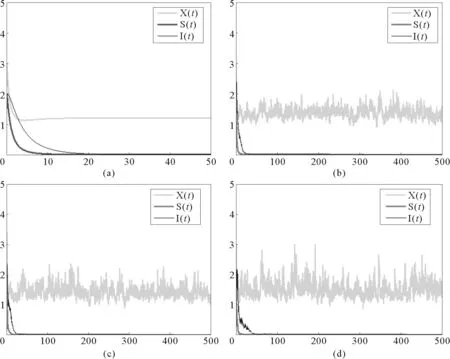
(a) 确定性模型(1)；(b)-(d)为随机模型(2)，干扰强度分别取值:(b)σi=0.05,ri=0.1，(c)σi=0.05,ri=0.15，(d)σi=0.05,ri=0.2，其中i=1,2,3。

在图2中，b1=0.6，b2=0.12，b3=0.5，a11=0.5，a12=0.6，a21=0.8，a22=0.1，a33=0.4，β=0.1。图2(a)为确定性模型(1)的时间序列图，(b)～(d)为随机模型(2)的时间序列图，干扰强度分别取：(b)σi=0.05,γi=0.1，(c)σi=0.05,γi=0.15，(d)σi=0.05,γi=0.2，其中i=1,2,3。

在图3中，b1=1，b2=0.1，b3=0.1，a11=0.2，a12=0.6，a21=0.1，a22=0.1，a33=0.2，β=0.5。图3(a)为确定性模型(1)的时间序列图，(b)-(d)为随机模型(2)的时间序列图，干扰强度分别取：(b)σi=0.05,γi=0.1，(c)σi=0.05,γi=0.15，(d)σi=0.05,γi=0.2，其中i=1,2,3。

在这种情况下，a11=0.2>p(b1-a11X*)=0.062 24，平衡E3(X*,S*,I*)=(4.69,0.66,1.15)R1=6.72>1。图3显示系统(2)的解在系统(1)的平衡点E3周围浮动，且浮动的幅度与干扰的强度σi,γi(i=1，2，3)的取值呈正相关关系。这与定理3.3中的结论一致。

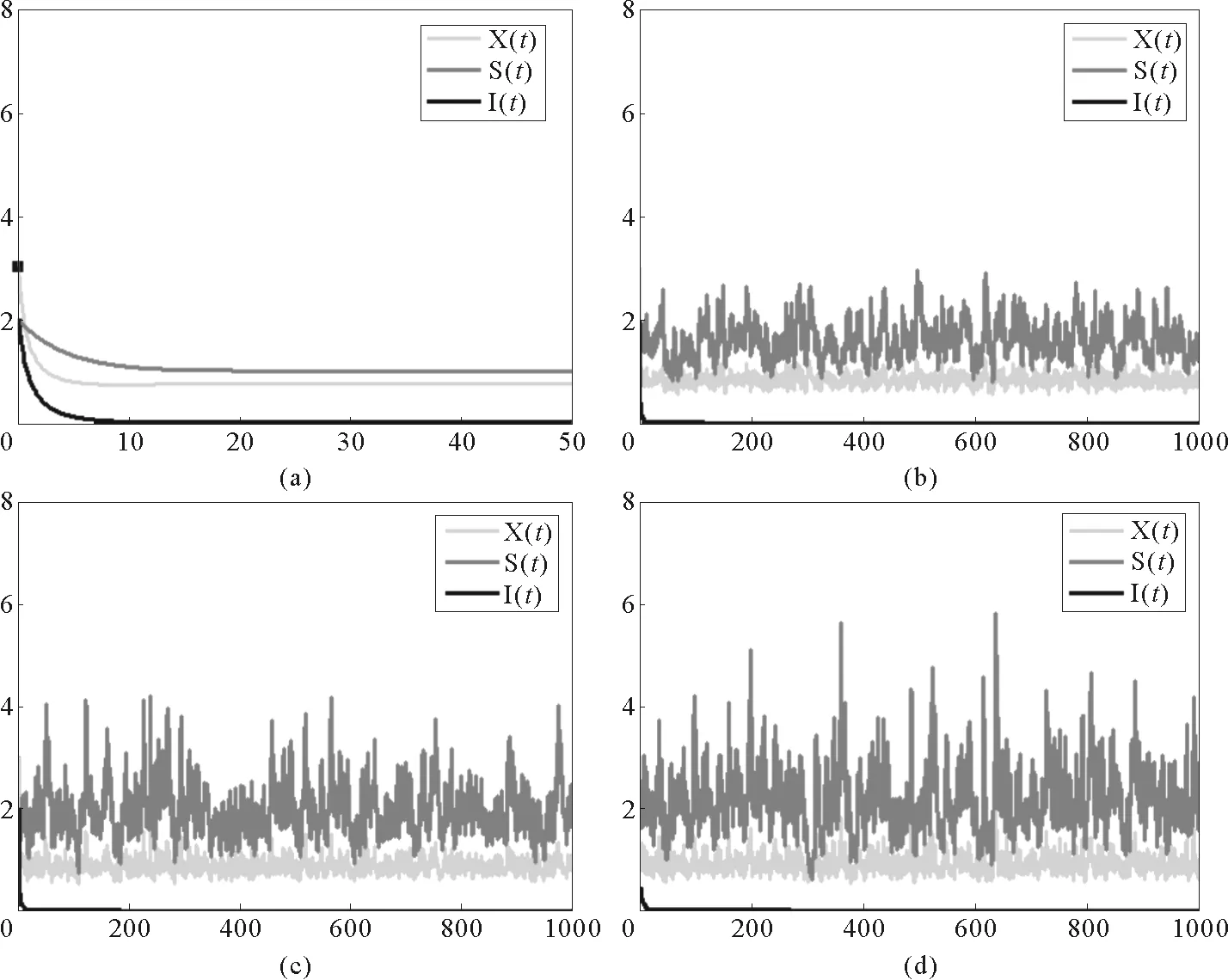
(a) 确定性模型(1)；(b)-(d)为随机模型(2)，干扰强度分别取值:(b)σi=0.05,ri=0.1，(c)σi=0.05,ri=0.15，(d)σi=0.05,ri=0.2，其中i=1,2,3。

(a) 确定性模型(1)；(b)-(d)为随机模型(2)，干扰强度分别取值:(b)σi=0.05,ri=0.1，(c)σi=0.05,ri=0.15，(d)σi=0.05,ri=0.2，其中i=1,2,3。

5 总结

本文研究了一类带有Beddington-DeAngelis功能性反应和Lévy跳的捕食者染病的捕食者-食饵系统。运用李雅普诺夫方法和推广的伊藤公式，本文首先证明了系统(2)全局正解的存在唯一性，然后讨论了模型(2)的解在其确定性模型的平衡点周围的渐近行为。Lévy跳在生物学上表示一些大的环境干扰，比如地震、火山、海啸等。当这类噪声干扰发生的时候，会对生物种群的稳定性产生一定的影响。文中干扰强度参数分别表示三个种群所受自然环境干扰的强度，比如地震、火山、暴雨等自然灾害的强度。定理3.1～3.3的理论结果表明，当这类噪声干扰不是特别大的时候，并不会使物种灭绝，但会使种群的数量在平衡状态周边浮动，且这种浮动的强度与噪声干扰的强度呈正相关关系。也就是说，种群对环境的变化具有一定的适应能力，当环境变化的强度很小的时候，它们并不会导致物种的灭亡，而是使物种密度在一定范围内波动。并且，当这种干扰强度不断增大时，它们对物种密度产生的影响也会越大。

[1]MENG X Z,ZHAO S N,FENG T,et al.Dynamics of a novel nonlinear stochastic SIS epidemic model with double epidemic hypothesis[J].Journal of Mathematical Analysis and Applications,2015,433:227-242.

[3]YAGI A,TON T V.Dynamic of a stochastic predator-prey population[J].Applied mathematics and Computation,2011,218(7):3100-3109.

[4]刘文昌,孟新柱.具有脉冲毒素输入的随机收获模型最优捕获策略[J].山东科技大学学报(自然科学版),2015,34(5):98-103. LIU Wenchang,MENG Xinzhu.Optimal harvesting strategies for stochastic harvest model with impulsive toxicant input[J].Journal of Shandong University of Science and Technology(Natural Science),2015,34(5):98-103.

[5]ZHANG Q M,JIANG D Q,LIU Z W,et al.Asymptotic behavior of a three species eco-epidemiological model perturbed by white noise[J].Journal of Mathematical Analysis and Applications,2016,433:121-148.

[6]LIU M,WANG K.Persistence,extinction and global asymptotical stability of a non-autonomous predator-prey model with random perturbation[J].Applied Mathematical Modelling,2012,36:5344-5353.

[7]HAQUE M,JIN Z,EZIO V.An ecoepidemiological predator-prey model with standard disease incidence [J].Mathematical Methods in the Applied Sciences,2009,32:875-898.

[8]CHATTOPADHYAY J,ARINO O.A predator-prey model with disease in the prey[J].Nonlinear Analysis-Series A Theory and Methods and Series B Real World Applications,1999,36:747-766.

[9]XIAO Y N,CHEN L S.Modeling and analysis of a predator-prey model with disease in the prey[J].Mathematical Biosciences,2001,171:59-82.

[10]HADELER K P,FREEDMAN H I.Predator-prey populations with parasitic infection[J].Journal of mathematical biology,1989,27:609-631.

[11]HAN L T,MA Z E,HETHCOTE H W.Four predator prey models with infectious diseases[J].Mathematical and Computer Modelling,2001,34:849-858.

[12]HETHCOTE H W,WANG W D,HAN L T,et al.A predator-prey model with infected prey[J].Theoretical Population Biology,2004,66:259-268.

[13]LI S,WANG X P.Analysis of stochastic predator-prey models with disease in the predator and Beddington-DeAngelis functional response[J].Advances in Difference Equations,2015,(1):1-21.

[14]ZU L,JIANG D Q,O'REGAN D.Conditions for persistence and ergodicity of a stochastic Lotka-Volterra predator-prey model with regime switching[J].Communications Nonlinear Science and Numerical Simulation,2015,29:1-11.

[15]LI D,CUI J A,SONG G H.Permanence and extinction for a single-species system with jump-diffusion[J].Journal of Mathematical Analysis and Applications,2015,430:438-464.

[16]LIU M,WANG K.Stochastic Lotka-Volterra systems with Levy noise[J].Journal of Mathematical Analysis and Applications,2014,410:750-763.

[17]ZOU X L,WANG K,Numerical simulations and modeling for stochastic biological systems with jumps[J].Communications Nonlinear Science and Numerical Simulation,2014,19:1557-1568.

(责任编辑：傅 游)

Stochastic Dynamics of a Predator-prey System with Disease in Predator

FENG Tao，MENG Xinzhu

(College of Mathematics and Systems Science，Shandong University of Science and Technology，Qingdao，Shandong 266590，China)

This paper investigated the stochastic dynamics of an infected predator-prey model with Beddington-DeAngelis functional response and Lévy jump. By using Lyapunov methods and It’s formula,this paper first discussed the existence and uniqueness of the global positive solution of the stochastic system,and then studied the asymptotic behaviors around the equilibrium points of its deterministic model. Results show that the solutions of the stochastic system fluctuate around the equilibrium points of its deterministic model under certain conditions,and the fluctuation intensity is positively correlated with the intensity of interference. Finally,numerical simulations were carried out to verify the theoretical findings.

predator-prey system; Beddington-DeAngelis functional response; asymptotic behaviors; random disturbance; Lévy jump

2016-05-16

国家自然科学基金项目(11371230,11501331)；山东省自然科学基金项目(ZR2015AQ001,BS2015SF002)；山东科技大学科研创新团队项目(2014TDJH102)

孟新柱(1972—)，男，山东菏泽人，教授，博士生导师，主要从事生物数学方面的研究，本文通信作者. E-mail:mxz721106@sdust.edu.cn

O175

A

1672-3767(2017)01-0099-12