关于4阶极小渐近基的一个结果

李静文,凌灯荣

(安徽师范大学数学计算机科学学院,安徽 芜湖 241003)

关于4阶极小渐近基的一个结果

李静文,凌灯荣

(安徽师范大学数学计算机科学学院,安徽 芜湖 241003)

设h≥2,若h阶渐近基A的任一真子集均不是h阶渐近基，则称集合A是自然数集N的h阶极小渐近基.为进一步刻画渐近基与极小渐近基之间的关系,本文综合运用自然数的b进制表示理论及分类讨论的方法,证明了存在一个集合是4阶渐近基且其任何子集均不是4阶极小渐近基.

极小渐近基;5进制表示

1 引言

令集合N是全体非负整数所组成的集合,A⊆N.对任意整数h≥2,令

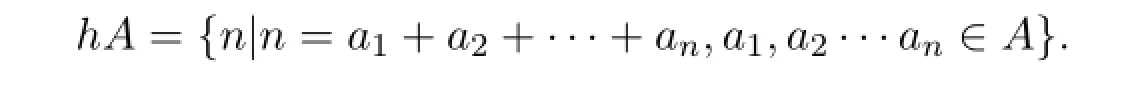
若对任意充分大的整数n均有n∈hA,则称集合A为h阶渐近基.若集合A的任意子集均不是h阶渐近基，则称集合A是h阶极小渐近基.对于任意元素a∈A,记Ea=hAh(A{a}),则集合A是h阶极小渐近基等价于Ea是无限集.设S⊆N,若对称差(S2A)∪(2AS)是有限集,则称这两个集合是渐近等价的.若对称差(S2A)∪(2AS)是无限集,则称这两个集合是渐近不等价的.1955年,文献[1]引入了极小渐近基的概念.1956年,文献[2]给出了极小基存在的非构造性证明.

1974年,文献[3]构造了首个极小渐近基且构造了一个2阶渐近基满足任意子集均不是2阶极小渐近基.

定理1.1[3]设

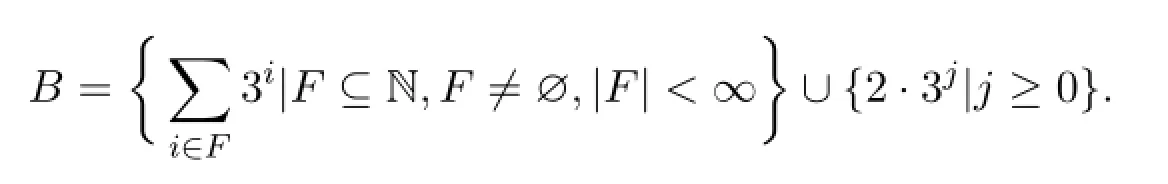
则B是2阶渐近基且B不包含任何2阶极小渐近基.

1978年,文献[4]构造了一类有无限多渐近基,但没有极小渐近基的集合.

定理1.2[4]设p1=2＜p2＜p3＜···是素数序列,m2＜m3＜···是递增的正整数序列且满足对于k≥2有mk+1＞2mk+pkpk+3和pkpk-1|mk.设

记

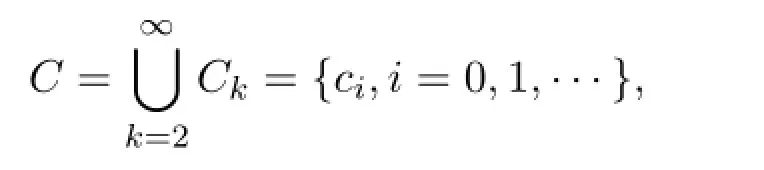
其中c0＜c1＜c2＜···,则C是N的渐近基且满足

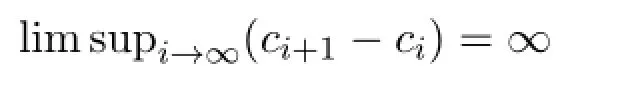
和对于任意有限集F，CF是N的渐近基.

定理1.3[4]存在一类集合S,具有无穷多渐进不等价的渐近基且没有极小渐近基.

最近凌灯荣和汤敏已构造一个3阶渐近基B满足任意子集均不是3阶极小渐近基.本文主要构造了一个4阶渐近基B满足任意子集均不是4阶极小渐近基.关于极小渐近基的见相关文献[5-7].

2 主要结果和证明

定理2.1设

则B是满足任意子集均不是4阶极小渐近基的4阶渐近基.

引理2.1若

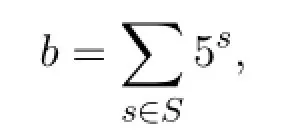
其中S是N的有限子集且card(S)＞1,则b是必需的.

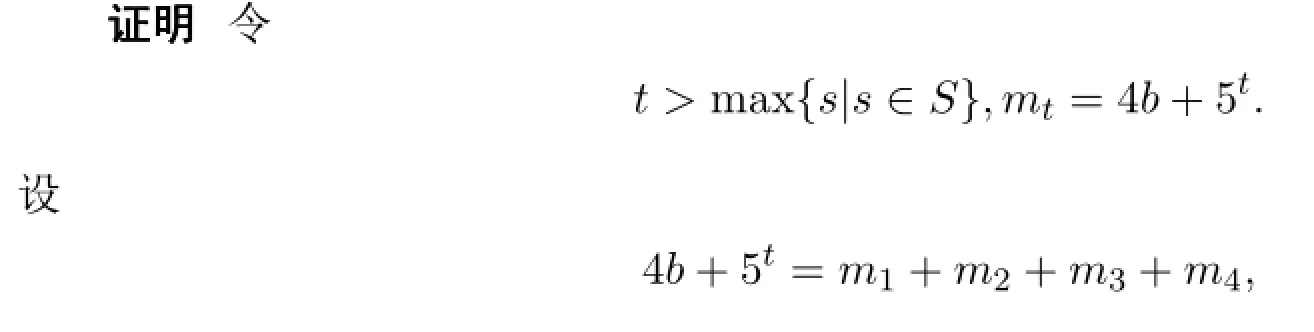
引理2.2若s＞0,则5s∈4B的惟一解是5s=5s-1+5s-1+5s-1+2·5s-1.

引理2.3B的任意无限子集是必需的.

引理 2.4B中元素1和B的子集 {5s,2·5s-1},{5s,2·5s},{5s,5s-1}都是必需的,其中s＞0.

引理2.5设U和V是N的有限子集,其中

[1]Stöhr A.Gelöste und ungelöste fragenüber basen der natürlichen zahlenreihe II[J].J.Renie Angew.Math., 1955,194：111-140.

[2]Härtter E.Ein beitrag zur theorie der minimalbasen[J].J.Renie Angew.Math.,1956,196：170-204.

[3]Nathanson M B.Minimal bases and maximal nonbases in additive number theory[J].J.Number theory, 1974,6：324-333.

[4]Erdös P,Nathanson M B.Sets of natural numbers with no minimal asymptotic bases[J].Proc.Amer.Math.Soc.,1978,70：100-102.

[5]Jia X D,Nathanson M B.A simple construction of minimal asymptotic bases[J].Acta Arith.,1989,51：95-101.

[6]Lee J B.A construction of minimal asymptotic bases[J].Period.Math.Hungar.,1993,26：211-218.

[7]Ling Deng Rong,Tang Min.On minimal asymptotic g-adic bases[J].Bull.Aust.Math.Soc.,2015,92：374-379.

A note minimal asymptotic basis of order 4

Li Jingwen,Ling Dengrong
(School of Mathematics and Computer Science,Anhui Normal University,Wuhu 241003,China)

Let h≥2,an asymptotic basis A of order h is minimal if no proper subset of A is an asymptotic basis of order h.In order to further describe the relationship between asymptotic basis and minimal asymptotic basis,this paper coordinates the theory of the b-adic representation of positive integers and the classification method.We prove that an asypmtotic basis of order 4 containing no subset which is a minimal asymptotic basis of order 4.

minimal asymptotic basis,5-adic representation

O156

A

1008-5513(2016)06-0606-12

10.3969/j.issn.1008-5513.2016.06.006

2016-06-18.

国家自然科学基金(11471017).

李静文(1992-),硕士生,研究方向：计算数论.

2010 MSC:11B13