具可变时滞的二阶非线性中立型泛函微分方程的振动性
2016-06-01杨甲山
杨 甲 山
(梧州学院 信息与电子工程学院, 广西 梧州 543002)
具可变时滞的二阶非线性中立型泛函微分方程的振动性
杨 甲 山
(梧州学院 信息与电子工程学院, 广西 梧州 543002)
摘要:研究一类非线性的具有可变时滞的二阶中立型泛函微分方程的振动性,利用Riccati变换技术及不等式分析技巧,获得了该方程振动的2个新的判别准则,所举例子说明这些准则是方程振动的“sharp”条件.
关键词:振动性;变时滞;泛函微分方程;Riccati变换
微分方程在自然科学及工程技术等领域有着非常广泛的应用,如著名的二阶Emden-Fowler型微分方程x″(t)+at-1x′(t)+btm-1xn(t)=0已广泛应用于数学物理、理论物理(特别是核物理)、生物工程、信息技术及工程机械等领域.近年来具变时滞的中立型泛函微分方程的振动性研究引起了国内外学者的广泛兴趣[1-24].笔者考虑如下形式的具变时滞的二阶非线性中立型微分方程
{a(t)[(x(t)+p(t)x(τ(t)))′]γ}′+
q(t)f(x(δ(t)))=0,t≥t0
(1)
的振动性,其中,函数a,p,q∈C([t0,+∞),R);常数γ为2个正奇数之商;函数f∈C(R,R)并且uf(u)>0(u≠0),本文假设下列条件成立:
(H1) a∈C1([t0,+∞),(0,+∞)),q(t)>0,p(t)≥0.
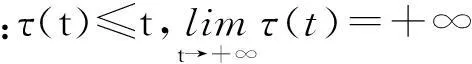
(H3)当u≠0时,f(u)/u≥L(这里常数L>0).
如果x(t)满足a(t)[(x(t)+p(t)x(τ(t)))′]γ∈C1([Tx,+∞),R),且在区间[Tx,+∞)满足式(1),则称函数x(t)∈C1([Tx,+∞),R)(Tx≥t0)是方程(1)的解,本文只关注方程(1)的非平凡解.如果方程(1)的解x(t)既不最终为正也不最终为负,则称解x(t)是振动的,否则是非振动的;如果方程(1)的所有解都是振动的,则称其是振动的.本文将分别在条件
(2)
和
(3)
成立的情况下建立方程(1)的振动性判别准则,改善对方程(1)的中立项系数函数的限制条件:0≤p(t)<1,得到这些准则的特殊情形,推广并改进了最近文献中的一系列结果.
引理1设A>0,B>0和λ>0均为常数,则

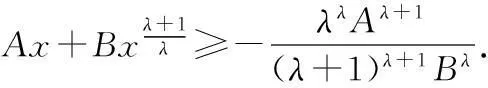
可由数学分析法证明之.
1主要结果及其证明
引入记号
z(t)=x(t)+p(t)x(τ(t)),
Q(t)=min{q(t),q(τ(t))},
φ+(t)=max{φ(t),0}.
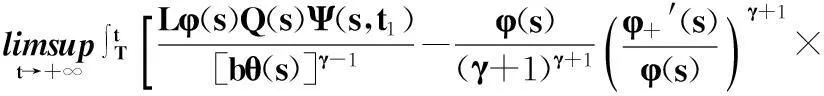
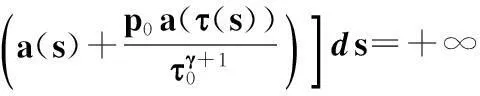
(5)
其中常数T≥t0足够大,b>0,函数
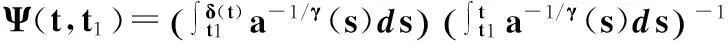
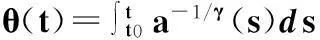
则方程(1)是振动的.
证明用反证法:设方程(1)有一个最终正解x(t)(当x(t)为最终负解时类似可证),则∃t1≥t0,当t≥t1时,有x(t)>0,x(τ(t))>0,x(δ(t))>0.由z(t)的定义,有z(t)>0,z(t)≥x(t)(t≥t1).由方程(1),
[a(t)(z′(t))γ]′=-q(t)f(x(δ(t)))≤
-Lq(t)x(δ(t))<0,
(6)
由此式容易证得z′(t)>0(t≥t1).应用式(6),
当t≥t1时,有

(7)
综合式(6)与(7),当t≥t1时,得
[a(t)(z′(t))γ]′+Lq(t)x(δ(t))+
p0Lq(τ(t))x(δ(τ(t)))+

-LQ(t)[x(δ(t))+p0x(δ(τ(t)))]≤
-LQ(t)z(δ(t))≤0,
(8)
令

(9)
则w(t)>0(t≥t1),利用式(9)及引理1(i),可得
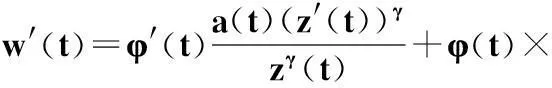
(10)
再令
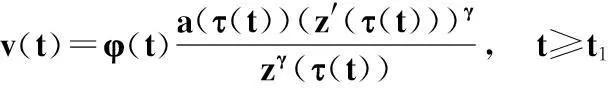
(11)
则v(t)>0(t≥t1).由于τ′(t)≥τ0>0,z′(t)>0,由引理1(i),类似地可得
(12)
于是,综合式(10),(12),并注意到式(8),可得
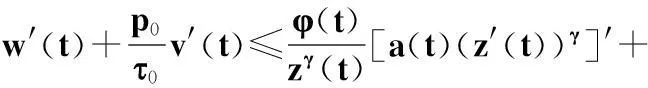
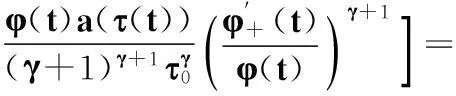
(13)
由式(6)知,a(t)[z′(t)]γ(t≥t1)是单调减少的,因此有

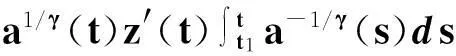
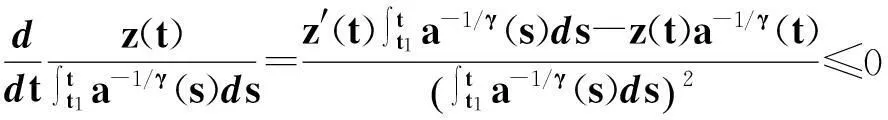
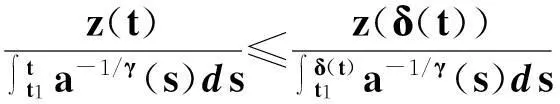
(14)

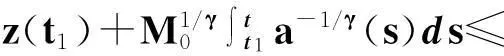
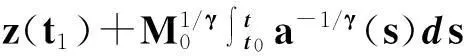
所以,存在充分大的t2≥t1及常数b>0,当t≥t2时,就有
(15)
将式(14),(15)代入式(13),得

所以
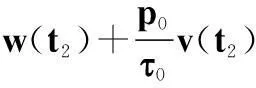
这与式(5)矛盾.定理1证毕.
定理2设式(3)成立,且0≤p(t)≤p0<+∞(p0为常数),若存在函数φ∈C1([t0,+∞),(0,+∞))使得式(5)成立,且

(16)
其中常数T≥t0足够大,函数
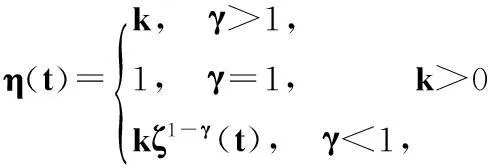
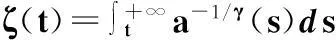
证明用反证法:设方程(1)有一个最终正解x(t)(当x(t)为最终负解时类似可证),则∃t1≥t0,当t≥t1时,有x(t)>0,x(τ(t))>0,x(δ(t))>0,根据定理1的证明,a(t)[z′(t)]γ严格单调递减且最终定号,进而z′(t)最终为正或为负.因此只需考虑如下2种情形:
情形iz′(t)>0(t≥t1).证明同定理1,可得到一个与式(5)矛盾的结果.
情形iiz′(t)<0(t≥t1).定义函数w(t)如下:

(17)
则w(t)<0(t≥t1),并且
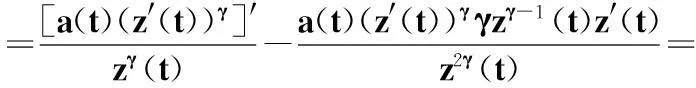
(18)
利用a(t)[z′(t)]γ的单调递减性,当s≥t≥t1时,有a(s)[z′(s)]γ≤a(t)[z′(t)]γ,进而
z′(s)≤a1/γ(t)z′(t)a-1/γ(s),
此式两边从t到u(u≥t)对s积分,可得
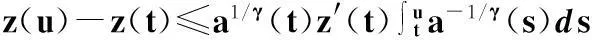
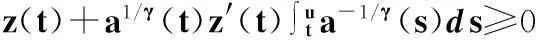
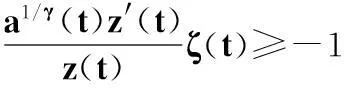
-1≤w1/γ(t)ζ(t)≤0,t≥t1.
(19)
定义函数v(t)如下:
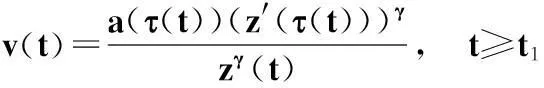
(20)
则v(t)<0(t≥t1).再利用a(t)[z′(t)]γ的单调递减性,有
a(τ(t))[z′(τ(t))]γ≥a(t)[z′(t)]γ,
进一步,
于是,由式(20),并利用z′(t)<0,可得

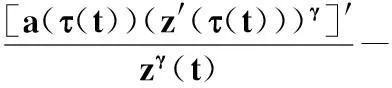
(21)
由a(τ(t))[z′(τ(t))]γ≥a(t)[z′(t)]γ,得v(t)≥w(t),利用式(19),同样有
-1≤v1/γ(t)ζ(t)≤0,t≥t1.
(22)
由式(18)和(21),并应用式(8)(由定理1的证明知,此时式(8)仍然成立)及z(δ(t))≥z(t),可得
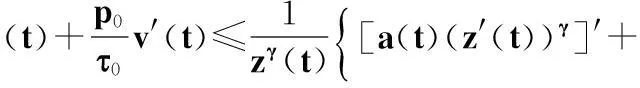

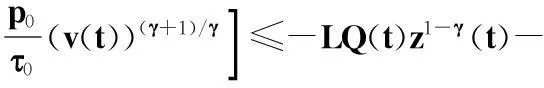
(23)
当γ>1时,因为z(t)>0,z′(t)<0(t≥t1),所以z(t)≤z(t1),即z1-γ(t)≥z1-γ(t1)=k.
当γ=1时,显然有z1-γ(t)=1.
当γ<1时,由于a(t)[z′(t)]γ是单调减小的,所以当s≥t≥t1时,有
a(s)[z′(s)]γ≤a(t)[z′(t)]γ,
即
z′(s)≤{a(t)[z′(t)]γ}1/γa-1/γ(s),
因此
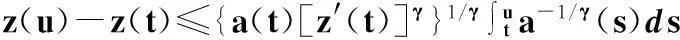
有
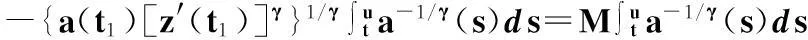
这里M=-{a(t1)[z′(t1)]γ}1/γ=-a1/γ(t1)z′(t1)>0为常数,令u→+∞,则由上式得

即z1-γ(t)≥M1-γζ1-γ(t).
综合上述3种情形及函数η的定义,由式(23),得
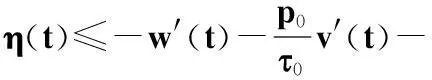
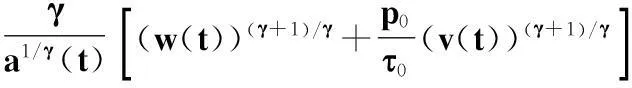
上式两边乘以ζγ(t)并从t1到t(t≥t1)积分,采用分部积分法,ζ′(t)=-a-1/γ(t),由式(19)和(22),以及引理1(ii),得
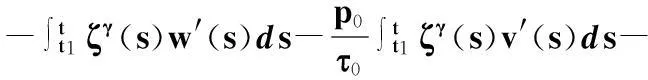
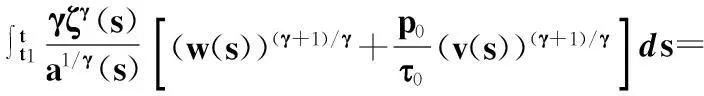
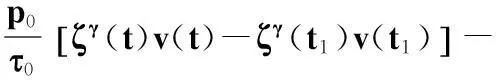

所以,
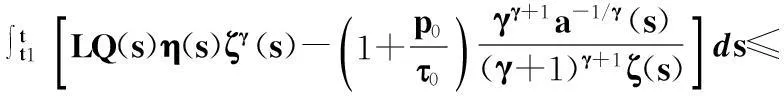

这与式(16)矛盾.定理2证毕.
2实例分析
例1考虑如下二阶微分方程(α>0为常数):
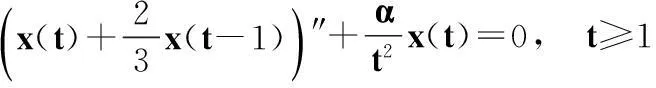
(24)
这里γ=1,a(t)≡1,p(t)=2/3,q(t)=α/t2,τ(t)=t-1,δ(t)=t,f(u)=u.容易验证,条件(H1)~(H3)均满足.现取φ(t)=t,注意到L=1,p0=2/3,τ0=1,Ψ(s,t1)=1,则
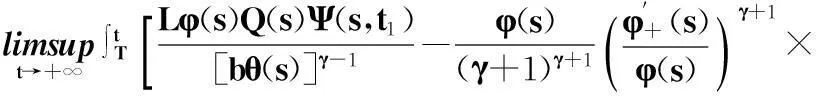

所以由定理1知,当α>5/12≈0.416 67时,方程(24)是振动的.
注2现改用文献[12]中的定理2.1来判定:因为
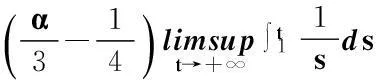
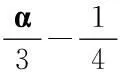
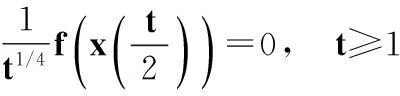
(25)
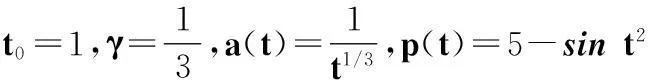
取f(u)=u[1+ln(1+u4)],因为
0 且 所以条件(H1)~(H3)全部满足.又因为 Q(t)=min{q(t),q(τ(t))}=t-1/4, 且 为简化计算,取φ(t)=1,T=3,则 所以定理1的条件全部满足,于是由定理1知方程(25)是振动的. 注3笔者注意到,由于方程(25)的中立项系数函数p(t)>1,因此文献[1-3,5-8,11-16,19-22]等中的定理对方程(25)均不适用. 参考文献(References): [1]HASANBULLI M, ROGOVCHENKO Y V. Oscillation criteria for second order nonlinear neutral differential equations[J]. Applied Mathematics and Computation,2010,215(12):4392-4399. [2]LI Tongxing, AGARWAL R P, BOHNER M. Some oscillation results for second-order neutral differential equations[J]. The Journal of the Indian Mathematical Society,2012,79(1-4):97-106. [3]LI Tongxing, AGARWAL R P, BOHNER M. Some oscillation results for second-order neutral dynamic equations[J]. Hacettepe Journal of Mathematics and Statistics,2012,41(5):715-721. [4]LI Tongxing, ROGOVCHENKO Y V. Asymptotic behavior of higher-order quasilinear neutral differential equations[J]. Abstract and Applied Analysis,2014,doi:org/10.1155/2014/395368. [5]LI Tongxing, ROGOVCHENKO Y V, ZHANG Chenghui. Oscillation of second-order neutral differential equations[J]. Funkcialaj Ekvacioj,2013,56(1):111-120. [6]AGARWAL R P, BOHNER M, LI Tongxing, et al. Oscillation of second-order Emden-Fowler neutral delay differential equations[J]. Annali di Matematica Pura ed Applicata,2014,193(6):1861-1875. [7]ZHANG Chenghui, AGARWAL R P, BOHNER M, et al. New oscillation results for second-order neutral delay dynamic equations[J]. Advances in Difference Equations,2012,2012:227.dol:10.1186/1687-1847-2012-227. [8]AGARWAL R P, BOHNER M, LI Tongxing, et al. A new approach in the study of oscillatory behavior of even-order neutral delay differential equations[J]. Appl Math Comput, 2013,225:787-794. [9]YANG Jiashan, QIN Xuewen. Oscillation criteria for certain second-order Emden-Fowler delay functional dynamic equations with damping on time scales[J]. Advances in Difference Equations, 2015(1):1-16. [10]YANG Jiashan, QIN Xuewen, ZHANG Xiaojian. Oscillation criteria for certain second-order nonlinear neutral delay dynamic equations with damping on time scales [J]. Mathematica Applicata, 2015,28(2):439-448. [11]SUN Shurong, LI Tongxing, HAN Zhenlai, et al. On oscillation of second-order nonlinear neutral functional differential equations[J]. Bulletin of the Malaysian Mathematical Society,2013,36(3):541-554. [12]YE L, XU Z. Oscillation criteria for second order quasilinear neutral delay differential equations[J]. Applied Mathematics and Computation,2009,207(2):388-396. [13]SUN Shurong, LI Tongxing,HAN Zhenlai,et al. Oscillation theorems for second-order quasilinear neutral functional differential equations[J]. Abstract and Applied Analysis,2012,2012:1-17.dol:10.1155/2012/819342. [14]杨甲山,方彬.一类二阶中立型微分方程的振动性[J].数学的实践与认识,2013,43(23):193-197. YANG Jiashan, FANG Bin. Oscillation of a class of second order neutral differential equations[J]. Mathematics in Practice and Theory, 2013,43(23):193-197. [15]杨甲山.具阻尼项的高阶中立型泛函微分方程的振荡性[J].中山大学学报:自然科学版,2014,53(3):67-72. YANG Jiashan. Oscillation of higher order neutral functional differential equations with damping[J]. Acta Scientiarum Naturalium Universitatis Sunyatseni,2014,53(3):67-72. [16]杨甲山.具正负系数和阻尼项的高阶泛函微分方程的振动性[J].华东师范大学学报:自然科学版,2014(6):25-34. YANG Jiashan. Oscillation of higher order functional differential equations with positive and negative coefficients and damping term[J].Journal of East China Normal University: Natural Science,2014(6):25-34. [17]杨甲山,张晓建.具阻尼项的二阶拟线性泛函差分方程的振荡性判别准则[J].浙江大学学报:理学版,2015,42(3):276-281. YANG Jiashan, ZHANG Xiaojian. Oscillation criteria for a class of second order quasilinear functional difference equation with damping[J].Journal of Zhejiang University:Science Edition,2015,42(3):276-281. [18]杨甲山.时标上一类具阻尼项的二阶动态方程的振荡性[J].系统科学与数学,2014,34(6):734-751. YANG Jiashan. Oscillation for a class of second-order dynamic equations with damping on time scales[J]. Journal of Systems Science and Mathematical Sciences,2014,34(6):734-751. [19]LI Tongxing, ROGOVCHENKO Y V, TANG S. Oscillation of second-order nonlinear differential equations with damping[J]. Mathematica Slovaca,2014,64(5):1227-1236. [20]杨甲山,覃学文.具阻尼项的高阶Emden-Fowler型泛函微分方程的振荡性[J].中山大学学报:自然科学版,2015,54(4):63-68. YANG Jiashan, QIN Xuewen. Oscillation of higher order Emden-Fowler functional differential equations with damping[J]. Acta Scientiarum Naturalium Universitatis Sunyatseni,2015,54(4):63-68. [21]杨甲山,张晓建.具正负系数的二阶阻尼微分方程的振动性[J].高校应用数学学报:A辑,2011,26(4):399-406. YANG Jiashan, ZHANG Xiaojian. Oscillation of second order damped differential equation with positive and negative coefficients[J]. Applied Mathematics A Journal of Chinese Universities:Ser A,2011,26(4):399-406. [22]杨甲山.具正负系数和阻尼项的高阶微分方程的振动定理[J].中山大学学报:自然科学版,2012,51(1):30-34. YANG Jiashan. Oscillation theorems of higher order differential equations with positive and negative coefficients and damping term[J]. Acta Scientiarum Naturalium Universitatis Sunyatseni,2012,51(1):30-34. [23]杨甲山.一类二阶非线性变时滞差分方程解的振动性[J].烟台大学学报:自然科学与工程版,2012,25(2):90-94. YANG Jiashan. Oscillation of solutions for a class of second-order nonlinear variable delay difference equation[J]. Journal of Yantai University: Natural Science and Engineering Edition,2012,25(2):90-94. [24]杨甲山.一类二阶非线性变时滞差分方程的振动准则[J].中央民族大学学报:自然科学版,2012,21(1):37-41. YANG Jiashan. Oscillation criteria for a class of second order nonlinear difference equation with variable delay[J]. Journal of Minzu University of China: NaturalSciences Edition,2012,21(1):37-41. YANG Jiashan Abstract:We study the oscillatory behavior of a class of second-order nonlinear neutral functional differential equations with variable delay. By using the generalized Riccati transformation and the inequality technique, we establish two new oscillation criteria for the oscillation of the equations. The examples are provided to illustrate that our result gives a sharper estimate for the oscillation of the equations. Key Words:oscillation; variable delay; functional differential equation; Riccati transformation 中图分类号:O 175.7 文献标志码:A 文章编号:1008-9497(2016)03-257-07 作者简介:杨甲山(1963-),ORCID:http://orcid.org/0000-0002-0340-097X,男,教授,主要从事微分方程的理论与应用研究,E-mail:syxyyjs@163.com. 基金项目:广西壮族自治区教育厅科研项目(2013YB223);国家青年科研基金资助项目(61503171);梧州学院2014年校级科研重大项目(2014A003);硕士学位授予单位立项建设项目(桂学位[2013]4号). 收稿日期:2015-08-16. DOI:10.3785/j.issn.1008-9497.2016.03.002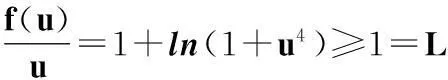

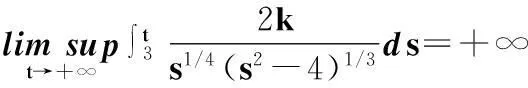

(SchoolofInformationandElectronicEngineering,WuzhouUniversity,Wuzhou543002,GuangxiZhuangAutonomousRegion,China)
Oscillation of certain second-order nonlinear neutral functional differential equations with variable delay.Journal of Zhejiang University(Science Edition), 2016,43(3):257-263
