Automobile magnetorheological damper multi-objective optimization analysis
2015-10-29GuohongDENGFeiLIchuanYANGJianOUYongZHANG
Guo-hong DENG, Fei LI*, E-chuan YANG, Jian OU, Yong ZHANG
(1College of Vehicle Engineering, Chongqing University of Technology, Chongqing 400054, China)(2College of Mechanical Engineering, Chongqing University of Technology, Chongqing 400054, China)
Automobile magnetorheological damper multi-objective optimization analysis
Guo-hong DENG1, Fei LI1*, E-chuan YANG2, Jian OU1, Yong ZHANG1
(1CollegeofVehicleEngineering,ChongqingUniversityofTechnology,Chongqing400054,China)(2CollegeofMechanicalEngineering,ChongqingUniversityofTechnology,Chongqing400054,China)
In order to improve automotive passive safety performance, a single rod cylinder magnetorheological damper that applied to the front energy-absorbing structure is proposed and optimized. By means of Bingham -plastic nonlinear flow modified model (BPM), taking the maximum damping force and the damping dynamic range as the optimization goals to avoid the design limitation under the high impact condition, the NSGA-II algorithm of modeFRONTIER is used to design the structural parameters of magnetorheological damper for multi-objective optimization. Optimization results showed that the maximum damping force and the damping dynamic range are inversely proportional, the optimization algorithm that used made it impossible for two optimization objectives to achieve the optimal at the same time, only to choose the optimized solutions that meet the conditions from all the Pareto solutions, the magnetic field distribution of optimized magnetorheological damper is more concentrated and reasonable.
Magnetorheological damper, Multi-objective optimization, BPM model, The NSGA - II algorithm
1 Introduction
With the rapid development of automobile industry and the rapid increasing of car ownership, car collision accidents happened under various traffic conditions also show a trend of raising, among them, the low-speed collision is one of the highest frequency of traffic accidents. But automobile parts of energy absorption play an important role during a low-speed collision, so, improving the energy absorption characteristics of components in low-speed collision can effectively improve the passive safety performance of car. Compared with energy absorption mechanism and responsiveness of traditional bumpers, magnetorheological damper can better play its cushioning effect with fast response time, continuous adjustable damping force. However, at present the design of magnetorheological dampers mainly bases on the quasi static models[1-3], which are only applicable to low speed environment, when magnetorheological dampers are applied to high speed impact occasions(such as car crash), it is necessary to adopt a new design theory and method. So, on the basis of Bingham-plastic nonlinear flow modified model(BPM)[4], regarding the maximum damping force and the damping dynamic range as the optimization goals, the NSGA-II algorithm of modeFRONTIER was used to design the structural parameters of the magnetorheological damper for multi-objective optimization, and the optimization results were analyzed.
2 The working principle of magnetorheological damper
Magnetorheological dampers make mainly use of magnetorheological fluid flowing properties to control the adjustable damping force. Under the action of magnetic field, MRF can transform quickly and reversibly Newtonian fluid with good liquidity into Bingham plastic solid with high yield strength, low liquidity. When the current size of excitation coil changes, applied magnetic field changes gradually, magnetorheological fluid viscosity also changes gradually. Magnetorheological fluid’s shear yield stress changes, as magnetic induction intensity of applied magnetic field changes, so as to adjust the damping force of damper continuously and reversible. According to the different forms of stress and flow of MRF applied to dampers magnetorheological damper mainly consists of valve type, shear, extrusion and shear valve type four basic patterns. According to the background requirements of magnetorheological damper used in the front energy absorption structure in car , based on the single rod cylinder monocular lever shear valve type magnetorheological damper as the research object, the piston structure diagram is shown in Fig.1.
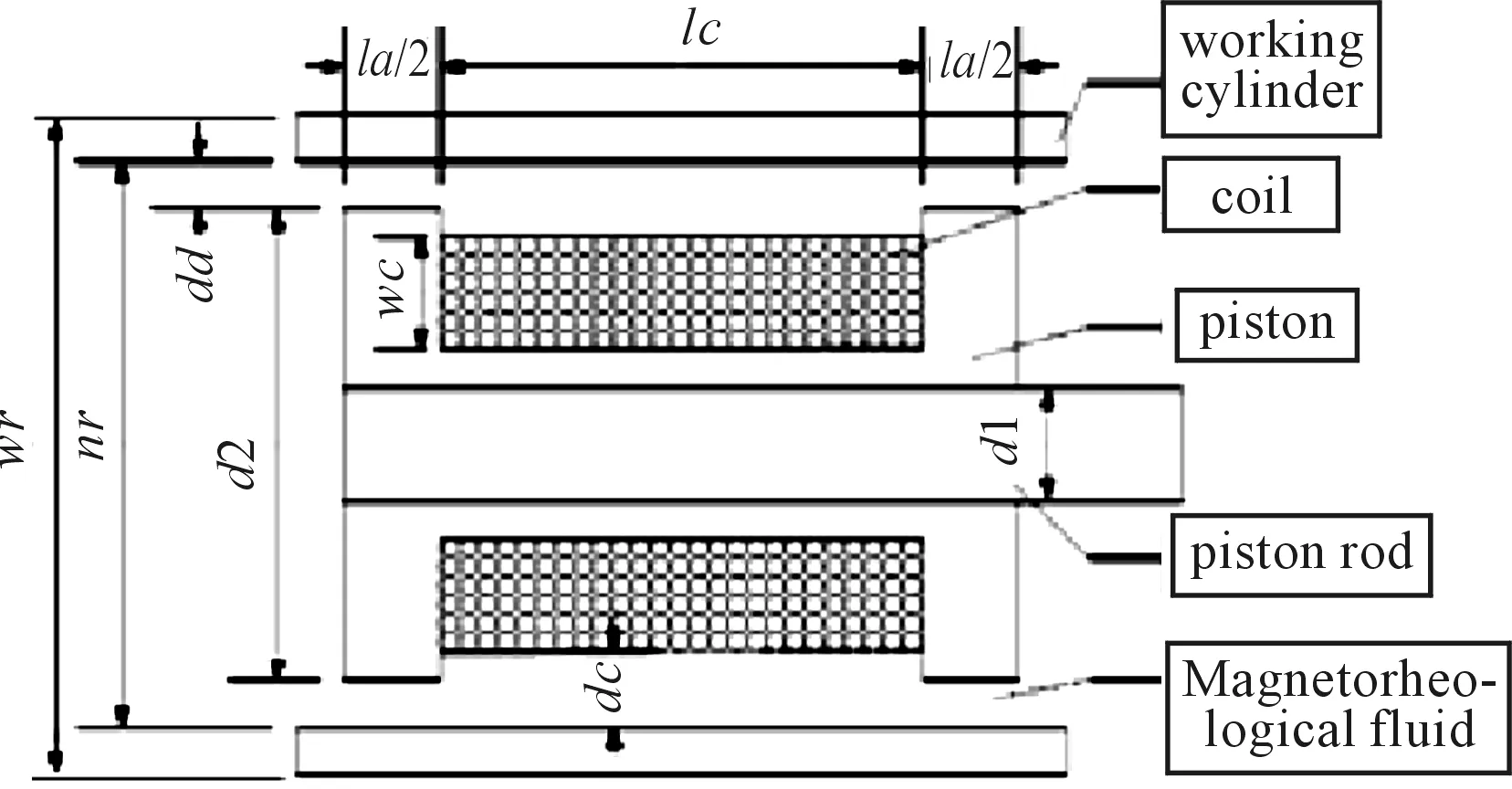
Fig.1 The piston structure diagram
Where,nrmeans cylinder diameter,d1 means piston rod diameter,d2 means piston diameter,lameans piston effective length,lcmeans coil width,dcmeans the clearance of the coil and damping channel,wcmeans the depth of coil,ddmeans damping clearance.
3 Bingham-plastic nonlinear flow modified model(BPM) features
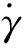
(1)
When speed is low, the flow of MRF can be approximate to laminar flow, the design of the damper adopts BP model can meet the engineering requirements, but as more and more wide engineering application of magnetorheological damper, its running speed has been improved, gradually develops to the impact, collision, drop and other fields of the high speed, at this moment, magnetorheological fluid flow of activation regional of the magnetorheological damper transforms layer flow into turbulent flow[6], BP model applied to low speed condition becomes no longer accurate under high speed, it will produce great error when design, so, in this paper, adopting Bingham-plastic nonlinear flow modified model(BPM)[7-9]based on Bingham plastic model. According to the fluid mechanics knowledge, the maximum damping force can be obtained when magnetorheological fluid flows through the damping channel.
(2)
(3)
Among them:
Foff=PoffAp
Poff=Pη+Pml+Pcoil+Pee
Where,Fmaxmeans maximum damping force produced by piston structure;Foffmeans the viscous force of piston compression stroke without magnetic field;Fsmeans the damping force of the shear mode without magnetic field;Fτmeans the coulomb damping force with magnetic field;Dmeans the damping dynamic range;Poffmeans pressure loss caused by the viscous damping;Apmeans the piston cross-sectional area;ηmeans MRF viscosity;V1means MRF average flow velocity in work gap;V2means MRF average flow velocity in coil and work cylinder clearance;τymeans MRF shear yield stress with magnetic field;Pηmeans the pressure loss of MRF flows through the piston outside diameter and the inner wall of the work cylinder;Pmlmeans the expansion and compression pressure loss of MRF flows through the coil and the inner wall of the work cylinder ;Pcoilmeans the pressure loss of MRF flows through the coil and the inner wall of the work cylinder;Peemeans the expansion and compression pressure loss of MRF flows through the piston outside diameter and working cylinder inner diameter;ρmeans the density of magnetorheological fluid;f1andf2mean respectively Darcy friction coefficient related to the Reynolds number of the piston outside diameter and working cylinder inner diameter, the coil and working cylinder inner diameter;KentryandKexitmean respectively inlet pressure loss coefficient and the outlet pressure loss coefficient, respectively 0.5 and 1;KscandKsemean respectively inlet pressure loss coefficient and outlet pressure loss coefficient,Admeans the sectional area of the piston outside diameter and working cylinder inner diameter;Acmeans the sectional area of the coil and working cylinder inner diameter.
4 Multi-objective optimization of magnetorheological damper
4.1Damperdesignrequirements
In order to design reasonable magnetorheological damper, it is necessary to estimate the range of control damper damping force and stroke, it is assumed that the vehicle collides with fixed rigid wall at a certain speed, the collision process does not occur secondary collision, all the kinetic energy is dissipated by two magnetorheological dampers, ignoring the friction between the tires and the ground, car is 1 421 kg, the initial velocity of the collision is 3 m/s(10.8 km/h), collision time is approximate 56 ms, the required average damping force of magnetorheological damper is 38 kN calculated by the impulse theorem in head-on collision, other dimensions are estimated according to the limiting conditions of damper replaces crash box: working cylinder outside diameter is 60 mm, inner diameter is 54 mm, piston length is 50 mm, the damper’s biggest length is 320 mm. The proposed design of this paper is that the magnetorheological damper’s biggest damping force is greater than 38.5 kN, the damping dynamic range is greater than 1.2 when the maximum current is 2 A at 3 m/s.
Considering the requirements of the magnetorheological damper applied to frontal crash. Setting the maximum speed of the piston as 3 m/s. The adopted magnetorheological fluid is SG-MRF2035 developed by a company, the fitting relationship of the shear yield stressτyand the magnetic flux densityBis that:
τy=93B5+355B4-480B3+233B2+51B-3
The materials of piston and cylinder are industrial pure iron, the piston rod is 45# steel, the wire is enamelled copper withφ0.8 mm, the relative permeability is 1.
4.2Optimizationdesignvariables,constraintsandobjectivefunctions
When the performance parameters of magnetorheological fluid are confirmed, the size of the magnetorheological damper damping channel and the size of the excitation magnetic field will have a decisive effect on the magnetorheological damper performance. The size of excitation magnetic field is related to coil loading current and the size of piston, loading current can be controlled through constant current power, but the piston sizes need to be optimized. So, settingla,lc,dc,wc,ddas optimization variables. Optimization variables and scope is shown Table 1.
Table 1 Optimization variables and scope
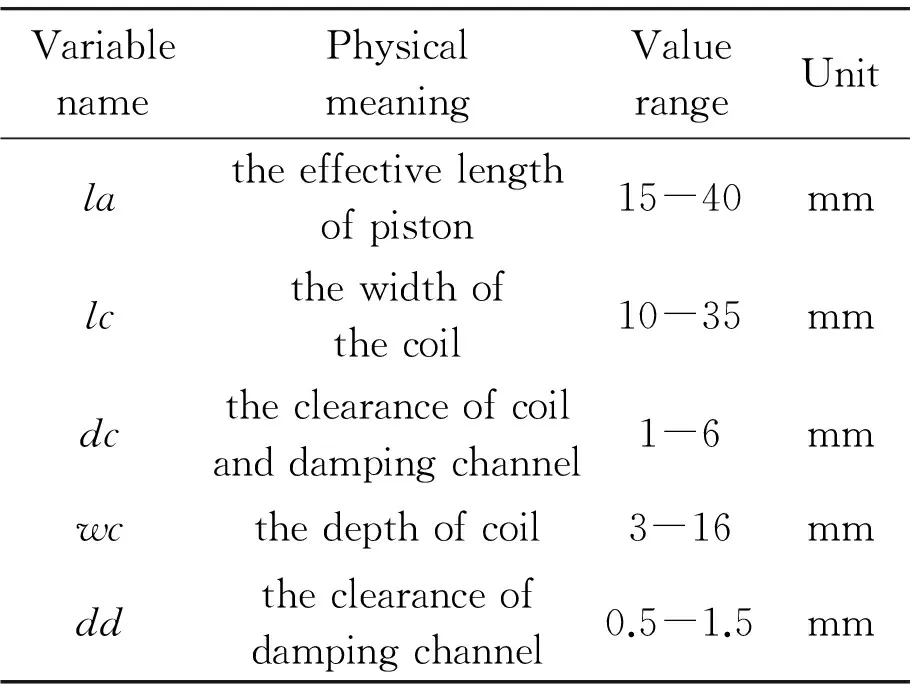
VariablenamePhysicalmeaningValuerangeUnitlatheeffectivelengthofpiston15-40mmlcthewidthofthecoil10-35mmdctheclearanceofcoilanddampingchannel1-6mmwcthedepthofcoil3-16mmddtheclearanceofdampingchannel0.5-1.5mm
The initial value for the initial variables are:dd=1 mm,dc=2 mm,wc=5 mm,la=30 mm,lc=20 mm.
Other parameters values arewr=60 mm,nr=54 mm,d1=24 mm.
The objective functions: to makeFmaxandDthe largest.
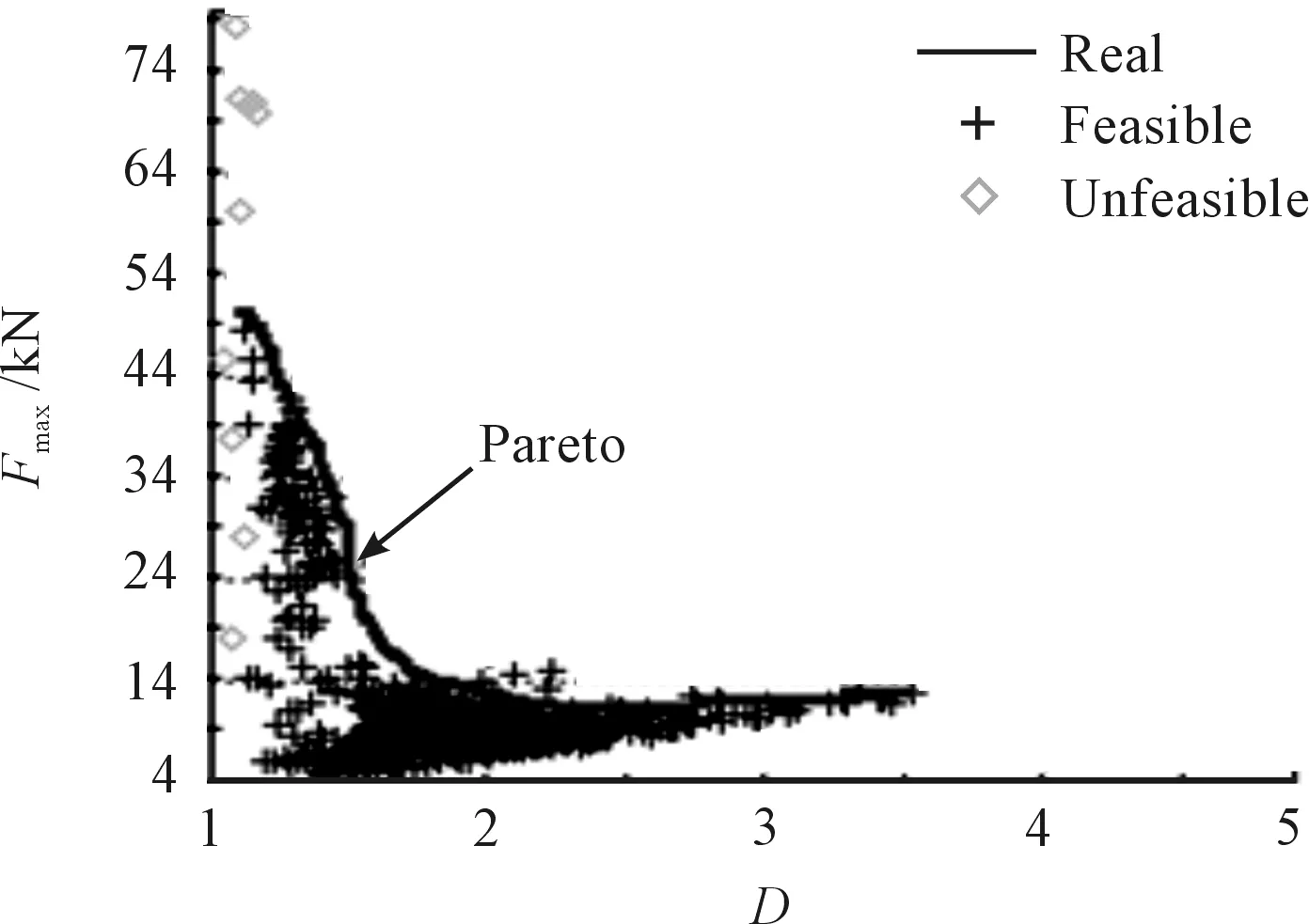
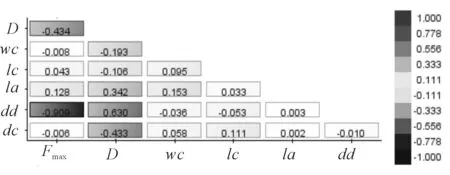
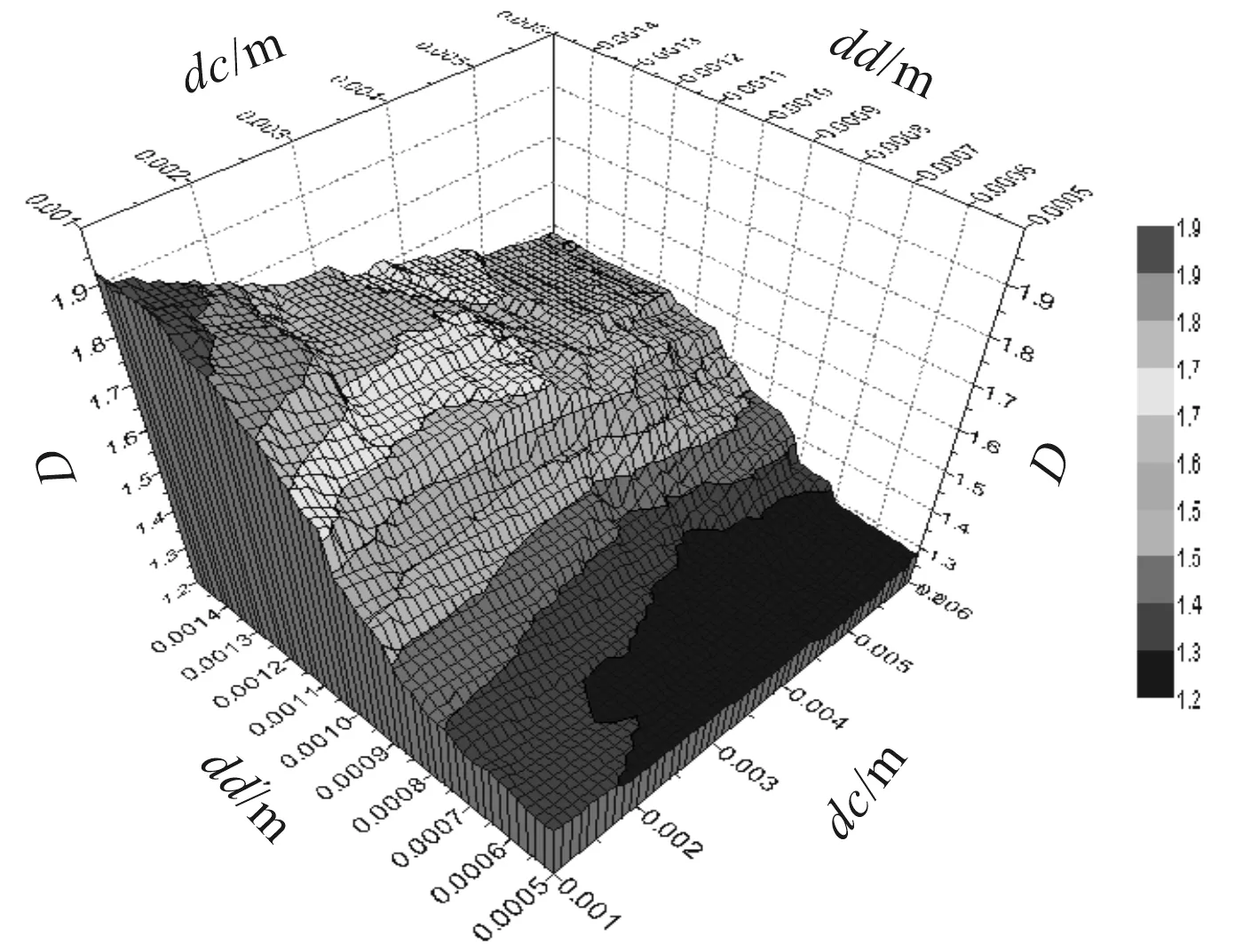
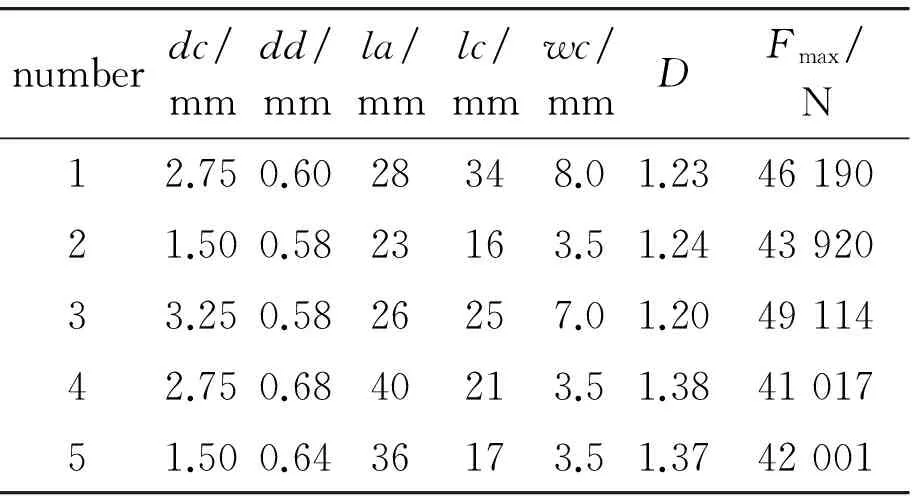
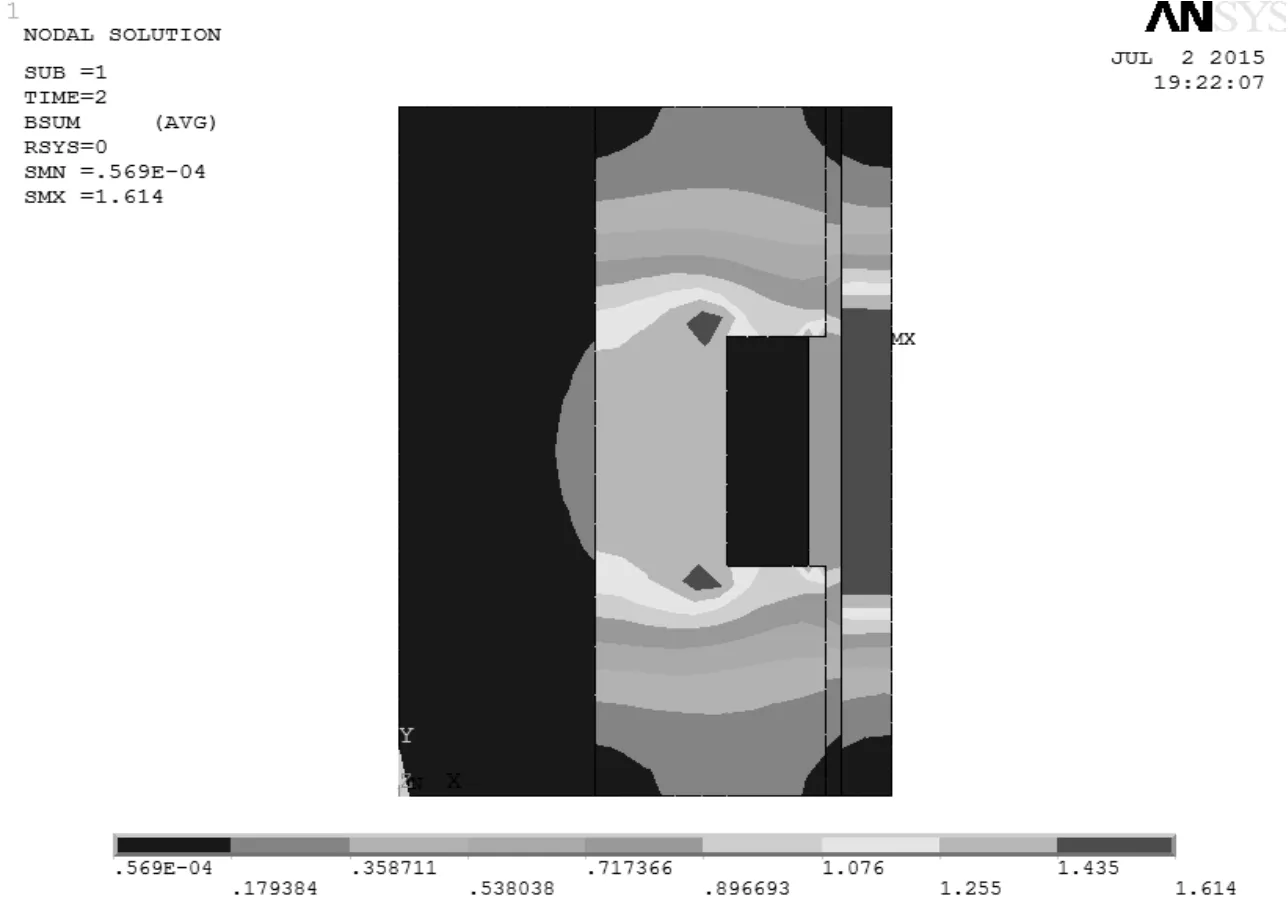
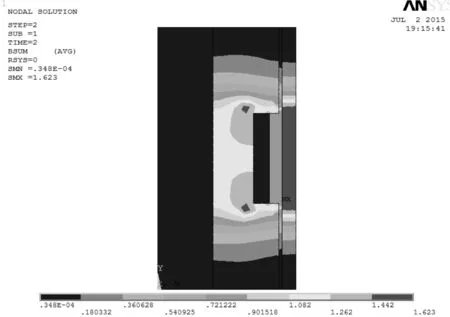
Constraints: 5 000 N D>1.2 dc+wc<13 mm 4.3Establishingoptimizationmodel This optimization model is established using multi-objective optimization analysis software modeFRONTIER, first simulating the magnetic flux density produced by piston and coil by using magnetic field analysis module of ANSYS and optimization variables[10], inputting the magnetic flux density into the Matlab, and then Matlab brings the optimization variables and the magnetic flux density into the mechanical model(BPM)for magnetorheological damper to calculate the maximum damping force and the damping dynamic range. According to the need to select 30 groups optimization variables, setting optimization variables according to the scope of table 1, setting optimization initial conditions combined with the constraints. Choosing NSGA-II algorithm for the multi-objective optimization, the maximum generation is 200, crossover rate is 0.9, mutation rate is 0.1, multi-objective optimization model is set up as shown in Fig.2. Fig.2 The optimization model Seeing optimized Pareto frontier is very clear(as shown in Fig.3), it’s impossible for two optimization objectivesDandFmaxto achieve the optimal at the same time, the relationship of them is inversely proportional, so only to choose eligible optimized solutions in many frontier solutions. Through the correlation coefficient matrix analysis of five optimization variables and two optimization targets(as shown in Fig.4), seeing that the two main factors of influencing the maximum damping force are the clearance of damping channelddand the piston effective lengthla, the correlation coefficient are respectively -0.909 and 0.128; The two main factors of influencing the damping dynamic range are the clearance of damping channelddand the clearance of coil and damping channeldc, the correlation coefficient are respectively 0.630 and -0.433. Fig.3 Pareto solutions for the chart Fig.4 The correlation coefficient matrix When analyzing the relationship of multiple independent variables and dependent variables, it’s more intuitive to observe the effect of each variable on the dependent variables through the response surface analysis. Fig.5 is the response surface analysis of the clearance of damping channelddand the piston effective lengthlaabout the maximum damping force. Fig.6 is the response surface analysis of the clearance of damping channelddand the clearance of coil and damping channeldcabout the damping dynamic range. Fig.5 The influence ofdd,lato theFmax Fig.6 The influence ofdd,dctoD As can be seen from the Fig.5: the change of the maximum damping force along the clearance of damping channeldddirection is faster than the piston effective lengthladirection, i.e, the influence of the clearance of damping channel on the maximum damping force is greater than the piston effective length, the damping clearance is more smaller and the maximum damping force is more bigger, but the piston effective length makes the maximum damping force decrease within a certain scope. From Fig.6 can be obtained: the change of the damping dynamic range along the clearance of damping channeldddirection is faster than the clearance of coil and damping channeldcdirection, i.e, the influence of the clearance of damping channel on the damping dynamic range is greater than the clearance of coil and damping channel, the clearance of damping channel is more bigger and the damping dynamic range is more bigger, but the clearance of coil and damping channel is more bigger, the damping dynamic range is more smaller. Considering the application background of automotive magnetorheological damper, table 2 lists the five groups typical Pareto solutions which can satisfy the maximum damping force and the damping dynamic range, making the effective length of pistonlaas big as possible when it’s possible for the two optimization goals to obtain the maximum value, at the same time, according to the design requirements of vehicle under low-speed collision (it is high speed for magnetorheological damper), choosing the fourth group solution as the final optimization solution. Simulating the magnetic induction intensity produced by piston coil for before optimization and optimized structure parameters of magnetorheological damper in magnetic field analysis module of ANSYS, as shown in Figs.7,8, the simulation results showed that the optimized magnetic field distribution is more concentrated and reasonable. Table 2 Typical Pareto solutions numberdc/mmdd/mmla/mmlc/mmwc/mmDFmax/N12.750.6028348.01.234619021.500.5823163.51.244392033.250.5826257.01.204911442.750.6840213.51.384101751.500.6436173.51.3742001 Fig.7 Magnetic induction intensity before optimization Fig.8 The optimized magnetic induction intensity The method of using the non dominated sorting genetic algorithm (NSGA II) to optimize the structure parameters of the magnetorheological damper for multi-objective is feasible. Optimization results showed that it’s impossible for two optimization goals to achieve the optimal at the same time, only to choose the optimized solutions that meet the conditions from all the Pareto solutions, the magnetic field distribution of optimized magnetorheological damper is more concentrated and reasonable. The NSGA-II algorithm can well solve the magnetorheological damper structure parameters optimization problem. Applying the optimized magnetorheological damper to the automobile front energy-absorbing structure to study its energy absorption characteristics will be the work that next step need to be done. Acknowledgement This paper is supported by Graduate Student Innovation fund of Chongqing University of Technology (YCX2014201). [1]Zang Chuanxiang. The mechanical properties of magnetorheological damper and its application in artillery Reverse recoil[D]. Nanjing: Nanjing University of Science and Technology, 2011:33-38. [2]Guan Xinchun, Guo Pengfei, Ou Jinping. The multi-objective optimization design and analysis of the magnetorheological damper[J]. Engineering Mechanics, 2009, 26(9): 30-35. [3]Metered H. Application of Nonparametric Magnetorheological Damper Model in Vehicle Semi-active Suspension System[J]. SAE Int. J. Passeng. Cars - Mech. Syst. 2012, 5(1):715-726. doi:10.4271/2012-01-0977. [4]Min Mao, Wei Hu, Norman M W. A nonlinear analtical model for magnetorheological energy absorbers under impact conditions[C]// Proceedings of ASME 2009 Conference on Smart Materials, Adaptive Structures and Intelligent Systems. USA: 2009, 1447: 393-404. [5]Shames I H, Cozzarelli F A. Elastic and inelastic stress Analysis[M]. Prentice Hall, Englewood Cliffs, New Jersey, 1992. [6]Yang Guangqiang, SPENCER B F, CARLSON J D, et a1. Large-scale MR fluid dampers: Modeling and dynamic performance considerations[J]. Engineering Structures, 2002, 24(3):309-323. [7]Mao Min. Adaptive Magnetorheologocial Sliding Seat System for Ground Vehicles [D]. USA: University of Maryland, 2011. [8]Gu Xiaoke. Magnetorheological damper multi-objective optimization design and semi-active suspension simulation research[D]. Chongqing: Chongqing University, 2014: 13-17. [9]Guan Zhi. The multi-objective optimization design and experimental study of automobile magnetorheological collision buffer[D]. Chongqing: Chongqing University, 2012. [10] Wang Lingling, Song Aiguo, Kuang Yinghui, et al. The design of new magnetorheological damper and magnetic field finite element analysis[J]. Machinery Design and Manufacture, 2011 (4): 10-12. 10.3969/j.issn.1001-3881.2015.24.007 Document code: A U463 汽车磁流变阻尼器多目标优化分析 邓国红1,李飞1*,杨鄂川2,欧健1,张勇1 1.重庆理工大学 车辆工程学院, 重庆400054 2.重庆理工大学 机械工程学院, 重庆400054 为了改善汽车的被动安全性能,提出将一种单杆单筒式磁流变阻尼器应用于汽车前部吸能结构中。提出以修正Bingham塑性模型(BPM模型)为理论基础,以最大阻尼力和可调范围为优化目标,运用modeFRONTIER自带的非支配排序遗传算法(NSGA-II)对所采用的磁流变阻尼器结构参数进行多目标优化分析。优化结果表明:最大阻尼力和可调范围成反比,所用的优化算法不可能使两个优化目标同时达到最优,只能在众多前沿解中选择符合条件的优化解。优化后的磁流变阻力器磁场分布更加集中合理。 磁流变阻尼器;多目标优化;BPM模型;非支配排序遗传算法(NSGA-II) 25 May 2015; revised 18 July 2015; Fei LI, graduate student for master degree. E-mail:378714208@qq.com accepted 15 October 2015 Guo-hong DENG, Professor. E-mail: dengguohong@cqut.edu.cn Hydromechatronics Engineering http://jdy.qks.cqut.edu.cn E-mail: jdygcyw@126.com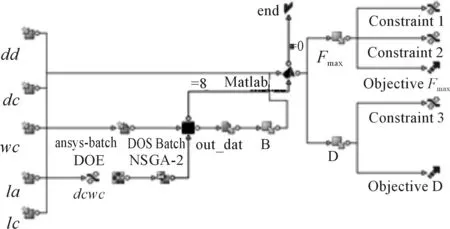
5 Optimization results

6 Conclusions
猜你喜欢
杂志排行
机床与液压的其它文章
- Molecular dynamics study of the effects of tool geometric parameters on titanium nanometric cutting
- Research on active suspension control strategy based on fuzzy PID control
- The design of control system applied in artificial grass tufting machine adopting servomotors
- Co-simulation and consumption analysis of wheel loader on negative loading condition
- Influence of the underlap in the poppet valve on its performance
- Comprehensive reliability evaluation of foreign high-end gantry machining Center
