混合AKNS-CLL方程的N-孤子解

潘红飞，夏铁成

（上海大学理学院，上海 200444）

混合AKNS-CLL方程的N-孤子解

潘红飞，夏铁成

（上海大学理学院，上海 200444）

由Hirota方法推导出混合AKNS-CLL方程的双线性导数方程和N-孤子解，并比较混合AKNS-CLL方程、AKNS方程和CLL方程的单孤子解|q|和|r|的图像，可以发现混合AKNS-CLL方程的特征形状不同于经典AKNS和CLL方程解.最后，通过约化，得到混合非线性Schr¨odinger方程的N-孤子解.

混合AKNS-CLL方程；混合非线性Schr¨odinger方程；N-孤子解；Hirota方法

非线性Schr¨odinger方程是非线性波理论研究所关注的一个问题.这一类方程的物理背景来源于非线性光学、非线性水波和等离子物理学等［1-4］.许多Schr¨odinger方程的精确解可以通过Hirota双线性法、反散射变换法、Jacobi椭圆法、tanh函数法等方法获得［5-8］，Schr¨odinger方程的多分量和超可积情形也是当前研究的热点［9-11］.

本工作考虑如下混合AKNS-CLL方程：

式中，q=q（x，t），r=r（x，t）是关于x和t的复函数，a，b∈C.当a=1，b=0和a=0，b=1时，方程（1）分别对应于二阶等谱AKNS方程［12-13］和带导数的非线性Schr¨odinger方程［14-15］.当r=q∗时，令t为-it，x为ix，则方程（1）约化为混合非线性Schr¨odinger方程（combined nonlinear Schr¨odinger equation，CNSE），即

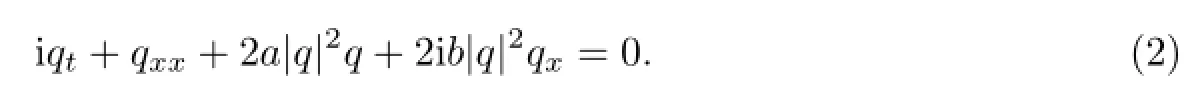
1　双线性形式和N-孤子解

令

并利用恒等式

则方程（1）转化为双线性导数方程：

式中，D是Hirota双线性算子，定义为

为求出混合AKNS-CLL方程（1）的N-孤子解，将f，g，s，h按参数ε展成级数，则有

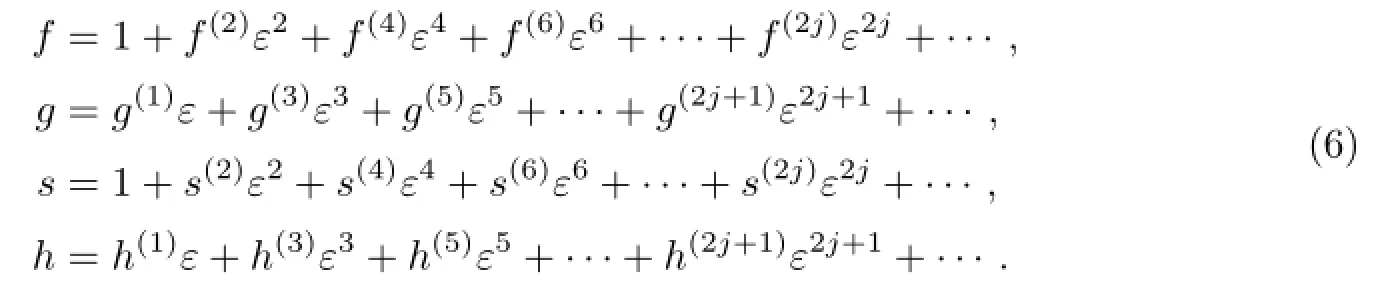
将式（6）代入方程（5），并比较ε的同次幂系数，可得

由式（7）和（8）可知，g（1）和h（1）有如下线性指数函数形式的解：

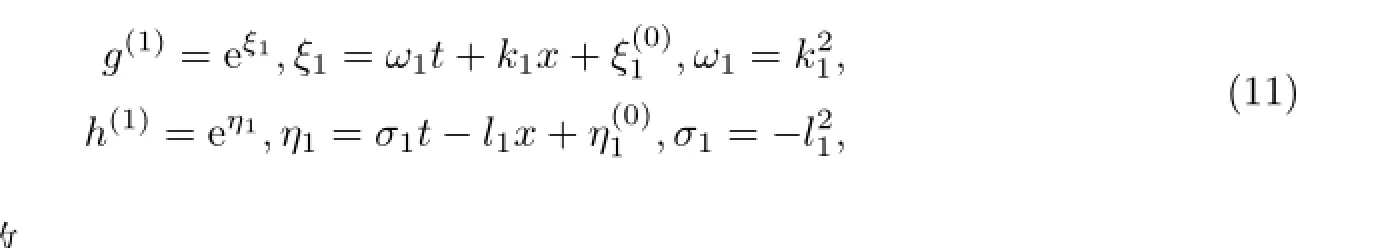
由式（3），可推得当ε=1时方程（1）的单孤子解为

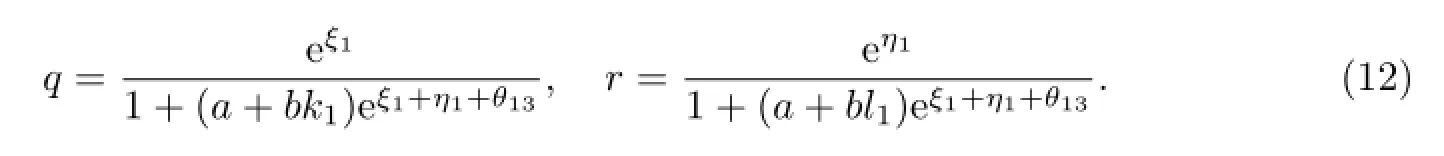
特别地，当（a，b）=（1，0）时，式（12）为二阶等谱AKNS方程的单孤子解［13］；当（a，b）=（0，1）时，式（15）为带导数的非线性Schr¨odinger方程的单孤子解［16］.作为对比，本工作给出由式（12）决定的|q|和|r|在4种情形时的图像（见图1）.通过选择不同的参数k1，l1，可以发现混合AKNS-CLL方程有类似于尖孤子解（peakon soliton）的性态（见图1（d）），这种现象是单一AKNS方程或CLL方程所不具有的一类特性.AKNS-CLL方程与AKNS方程、CLL方程存在本质的区别.

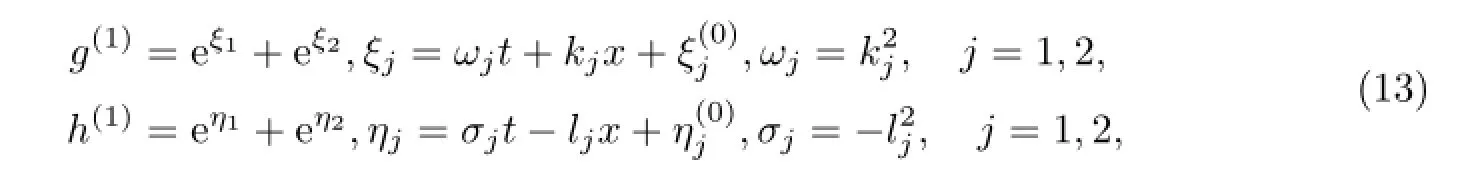
图1　方程（1）的单孤子解Fig.1 One soliton solutions of Eq.（1）

将式（13）代入式（9）和（10），可得

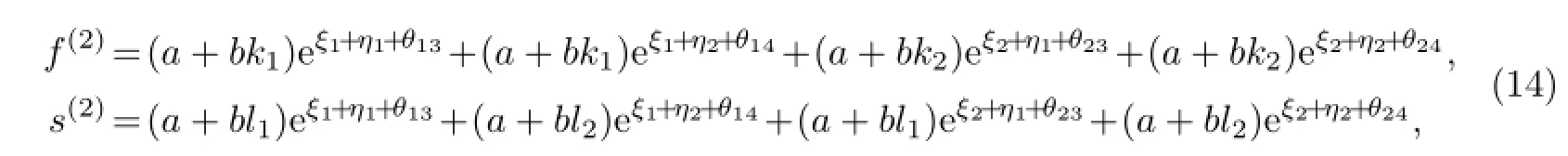
将式（13）和（14）代入式（7）和（8）的第二式，有

将式（13）～（15）代入式（9）和（10）的第二式，又可得到

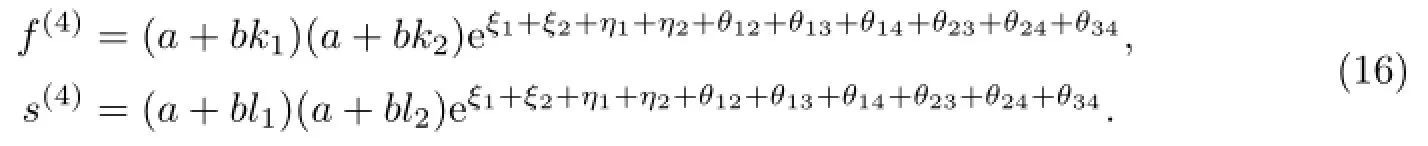
根据式（7）～（10），可取

所以，有

因此，令ε=1，即可求得方程（1）的双孤子解（见式（3））.特别地，当（a，b）=（1，0）时，可引出二阶等谱AKNS方程的双孤子解［13］；当（a，b）=（0，1）时，可求得带导数非线性Schr¨odinger方程的双孤子解［16］.

一般地，方程（1）的关于f，g，h和s的N-孤子解（N=1，2，···）为

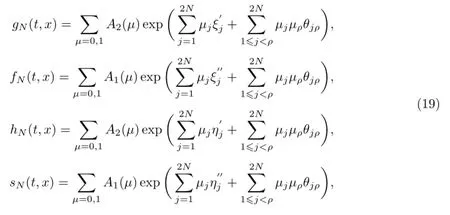
式中，

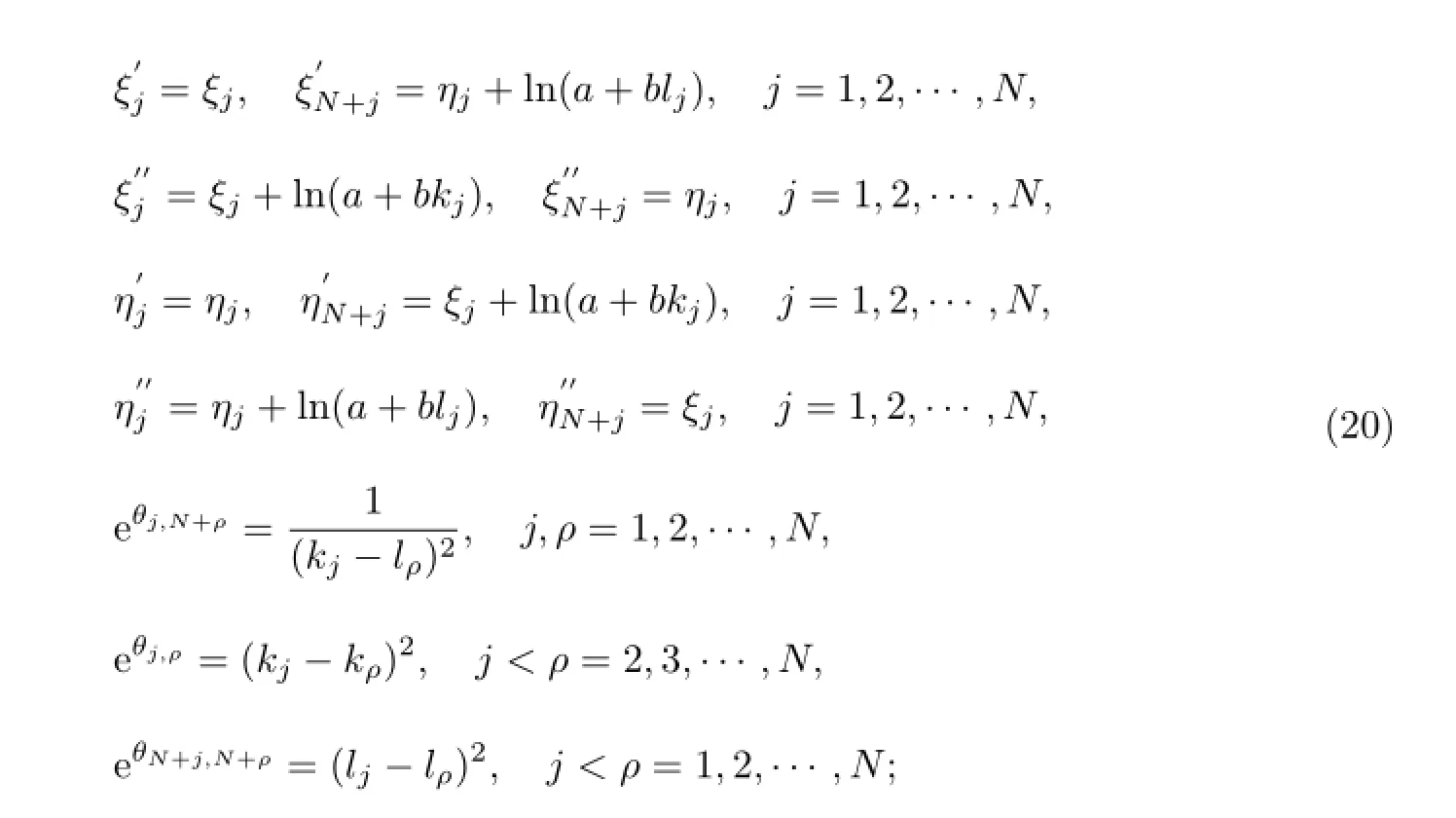
A1（µ），A2（µ）表示当µj（j=1，2，···，2N）取所有可能的0或1时，还需分别满足

2　约化

作为应用，考虑混合非线性Schr¨odinger方程（式（2）），并通过方程（1）的约化给出双线性导数方程和N-孤子解.取s=f∗，h=g∗，并令t为-it，x为ix，则方程（4）约化为式（2）的双线性导数方程，即

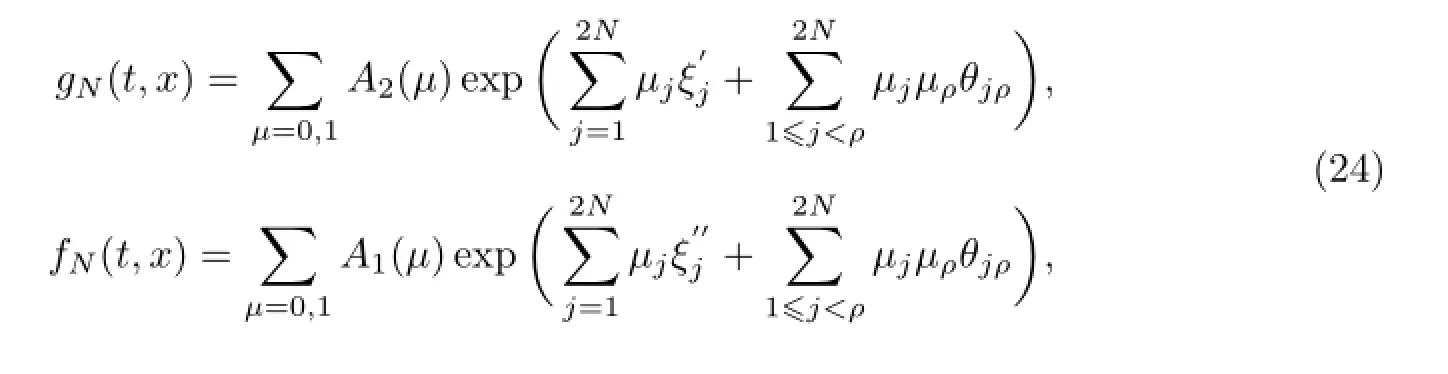
式中，

致谢感谢上海大学数学系陈登远教授的有益指导.

［1］Johnson R S.A modern introduction to the mathematical theory of water waves［M］.New York：Cambridge University Press，1997：298-345.

［2］Belashov V Y，Vladimirov S V.Solitary wave in dispersive complex media［M］.Berlin：Springer-Verlag，2005.

［3］Kivshar Y S，Agrawal G P.Optical solitons from fibers to photonic crystals［M］.New York：Academic Press，2003：3-25.

［4］Maimistov A I.Solitons in nonlinear optics［J］.Quantum Electronics，2010，40：756-781.

［5］Zayed E M E，Zedan H A，Gepreel K A.On the solitary wave solutions for nonlinear Hirota-Satsuma coupled KdV of equations［J］.Chaos，Solitons and Fractals，2004，22（2）：285-303.

［6］Khuri S A.A complex tanh-function method applied to nonlinear equations of Schr¨odinger type［J］.Chaos，Solitons and Fractals，2004，20（5）：1037-1040.

［7］Ablowitz M J，Clarkson P A.Solitons，nonlinear evolution equations and inverse scattering［M］.Cambridge：Cambridge University Press，1991：70-100.

［8］Hirota R.The direct methods in soliton theory［M］.Cambridge：Cambridge University Press，2004：157-168.

［9］Tsuchida T，Wadati M.New integrable systems of derivative nonlinear Schr¨odinger equations with multiple components［J］.Physics Letter A，1999，257：53-64.

［10］Roelofs G H M，Kersten P H M.Supersymmetric extensions of the nonlinear Schr¨odinger equation：symmetries and coverings［J］.Journal of Mathematical Physics，1992，33（6）：2185-2206.

［11］He G L，Zhai Y Y，Geng X G.A super hierarchy of coupled derivative nonlinear Schr¨odinger equations and conservation laws［J］.Communications in Nonlinear Science and Numerical Simulation，2014，19（7）：2228-2233.

［12］Chen D Y，Zhang D J，Bi J B.New double Wronskian solutions of the AKNS equation［J］. Science China Mathematics，2008，51（1）：55-69.

［13］Ablowitz M J，Kaup D J，Newell A C，et al.The inverse scattering transform-Fourier analysis for nonlinear problem［J］.Studies in Applied Mathematics，1974：53（4）：249-315.

［14］Chen H H，Lee Y C，Liu C S.Integrability of nonlinear Hamiltonian systems by inverse scattering method［J］.Physica Scripta，1979，20：490-492.

［15］Chen D Y.k-constraint for the modified Kadomtsev-Petviashvili system［J］.Journal of Mathematical Physics，2002，43（4）：1956-1965.

［16］Zhai W，Chen D Y.N-soliton solutions of general nonlinear Schr¨odinger equation with derivative［J］.Communications in Theoretical Physics，2008，49（5）：1101-1104.

N-soliton solutions of combined AKNS-CLL equation

PAN Hong-fei，XIA Tie-cheng
（College of Sciences，Shanghai University，Shanghai 200444，China）

The bilinear form and N-soliton solutions are derived for a combined AKNSCLL equation using the Hirota approach.These solutions are novel in general.The one-soliton solutions of the combined AKNS-CLL equation，AKNS equation and CLL equation were drawn.Also，the combined AKNS-CLL equation is given a different characteristic from the classical AKNS and CLL equations.The bilinear form and N-soliton solutions of combined nonlinear Schr¨odinger equation are obtained by reduction.

combined AKNS-CLL equation；combined nonlinear Schr¨odinger equation；N-soliton solutions；Hirota approach

O 175.2

A

1007-2861（2015）06-0709-08

10.3969/j.issn.1007-2861.2014.04.002

2014-06-23

国家自然科学基金资助项目（11271008）

夏铁成（1960—），男，教授，博士生导师，研究方向为孤子与可积系统.E-mail：xiatc@shu.edu.cn