Dirichlet空间上Toeplitz算子的乘积

冯丽霞

(1.西北大学数学与科学史研究中心，陕西 西安 710127；2.山西师范大学数学与计算机科学学院，山西 临汾 041004)

Dirichlet空间上Toeplitz算子的乘积

冯丽霞1，2

(1.西北大学数学与科学史研究中心，陕西 西安 710127；2.山西师范大学数学与计算机科学学院，山西 临汾 041004)

给出了Dirichlet空间上一个Toeplitz算子的共轭算子与另一个Toeplitz算子的乘积仍为Toeplitz算子的刻画.并得到了Dirichlet空间上一个Toeplitz算子的共轭算子与另一个Toeplitz算子的乘积为零算子的充要条件.

Dirichlet空间；Toeplitz算子；共轭算子；乘积

1 预备知识

设D是复平面C上的单位开圆盘，dA表示D上正规化的面积测度.称D上光滑函数f在范数

下，取闭包所得到的空间为Sobolev空间，记为S，则S是一个Hilbert空间，定义其上内积为

由S中所有满足f(0)=0的解析函数构成的闭子空间称为Dirichlet空间，记为D.

D上的一个非负测度μ称为D-Carleson测度，如果存在非负常数c，使得

∫D|f|2dμ≤c‖f‖2，f∈D.

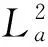
令

设φ∈M，则φ定义的D上Toeplitz算子Tφ为

Tφ(f)=P(φf)，f∈D，

其中P是从S到D上的正交投影.容易验证Tφ是D上的有界算子.一直以来，各种积分算子的有界性也是函数空间上算子理论的研究课题之一[1].

(1)φ=0，且ψ=0；

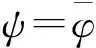
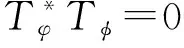
2 定理证明

下面引理表明，由S中的调和函数φ定义的Toeplitz算子Tφ在D上有界，当且仅当φ∈M.

引理1 设φ是S中的调和函数.如果Tφ在D上是有界的，则φ∈M.

证明 令

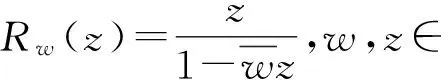
容易验证：

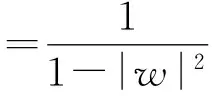
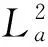
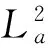
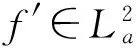
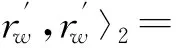
〈φ′rw，kw〉2+〈φkw，kw〉2=(1-|w|2)φ′(w)rw(w)+φ(w)=

w(1-|w|2)φ′(w)+φ(w)≤ω〈φ′，kw〉2+φ(w).

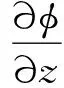
对任意f，ɡ∈D，

〈Tφf，ɡ〉=〈φf，ɡ〉=〈φ′f+φf′，ɡ′〉2=〈φ′f，ɡ′〉2+〈φf′，ɡ′〉2.

因为φ有界且Tφ有界，所以

|〈φ′f，ɡ′〉2|≤|〈Tφf，ɡ〉|+|〈φf′，ɡ′〉2|≤(‖Tφ‖+‖φ‖∞)‖f‖‖ɡ‖，

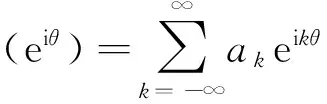
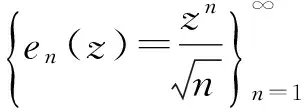
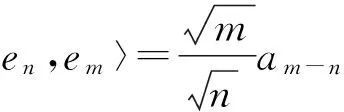
(1)

定理1的证明 充分性易验证，我们只给出必要性的证明.

设

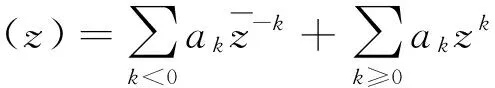
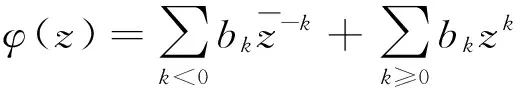
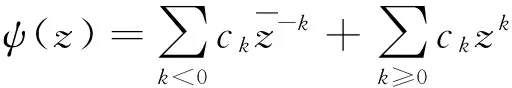
由(1)式，对于i，j≥1，

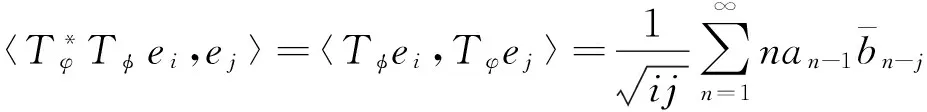
(2)

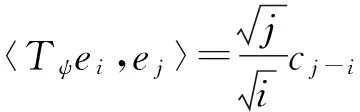
(3)

(4)

同理可得

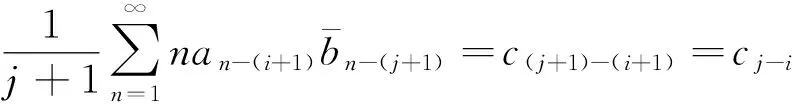
即

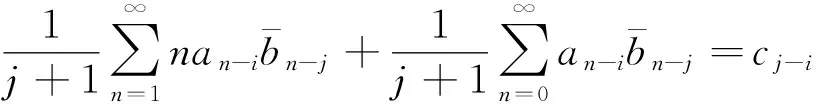
(5)

利用(4)和(5)式可知

即

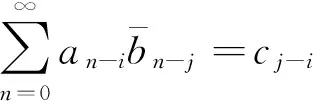
(6)

同时也有

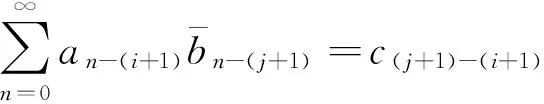
即

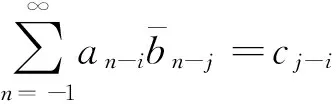
(7)

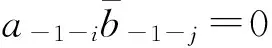
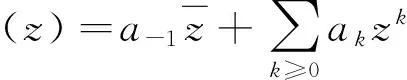
或

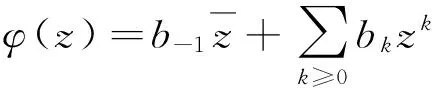
利用(4)与(6)式计算得

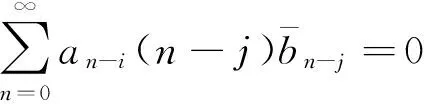
(8)

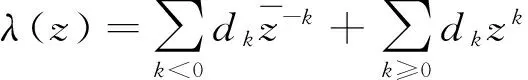
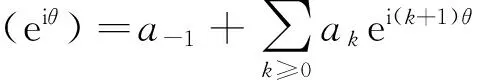
或

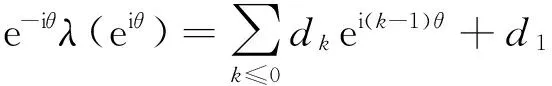
再由(8)式，

这表明eiθφ(eiθ)λ(eiθ)=0或e-iθφ(eiθ)λ(eiθ)=0，对几乎处处的θ∈[0，2π]成立.由F.Riesz和M.Riesz定理[7]得φ=0或λ=0.

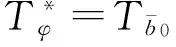
[1] 周淑娟.Marcinkiewicz积分在加权Hardy空间的有界性[J].东北师大学报(自然科学版)，2015，47(2)：21-24.

[2] CHEN Y，NGUYEN Q D.Toeplitz and Hankel operators with symbols on Dirichlet space[J].J Math Anal Appl，2010，369(1)：368-376.

[3] LEE Y.Algebraic properties of Toeplitz operators on the Dirichlet space[J].J Math Anal Appl，2007，329(2)：1316-1329.

[4] LEE Y.Finite sums of Toeplitz products on the Dirichlet space[J].J Math Anal Appl，2009，357(2)：504-515.

[5] LEE Y，ZHU K.Sums of products of Toeplitz and Hankel operators on the Dirichlet space[J].Intergr Equ Oper Theory，2011，71(2)：275-302.

[6] YU T.Toeplitz operators on the Dirichlet space[J].Integr Equ Oper Theory，2010，67(2)：163-170.

[7] DOUGLAS R G.Banach algebra techniques in operator theory.2nd ed[M].New York：Springer-Verlag，1998：137.

(责任编辑：李亚军)

Product of Toeplitz operators on the Dirichlet space

FENG Li-xia1，2

(1.Center for the History of Mathematics and Science，Northwest University，Xi’an 710127，China；2.School of Mathematics and Computer Sciences，Shanxi Normal University，Linfen 041004，China)

It is studied that the product of the adjoint of a Toeplitz operator and another Toeplitz operator can be a Toeplitz operator on the Dirichlet space under some conditions.As a corollary，we show that on the Dirichlet space，the zero product of the adjoint of a Toeplitz operator and another Toeplitz operator holds only for trivial symbols.

Dirichlet space；Toeplitz operators；conjugate operator；product

1000-1832(2015)04-0042-04

10.16163/j.cnki.22-1123/n.2015.04.009

2014-03-08

国家自然科学基金资助项目(11201274).

冯丽霞(1978—)，女，博士，讲师，主要从事泛函分析，数学史与数学教育研究.

O 177.1 [学科代码] 110·57

A