Measurement and Modeling of Vapor-Liquid Equilibrium for Ternary System Water + 2-Propanol + 1-Butyl-3-methylimidazolium Chloride*

(邓东顺)(乔玉珍)(姬登祥)(葛筠)(章连众)**

Zhejiang Province Key Laboratory of Biofuel, College of Chemical Engineering and Material Science, Zhejiang University of Technology, Hangzhou 310014, China

Measurement and Modeling of Vapor-Liquid Equilibrium for Ternary System Water + 2-Propanol + 1-Butyl-3-methylimidazolium Chloride*

DENG Dongshun(邓东顺), QIAO Yuzhen(乔玉珍), JI Dengxiang(姬登祥), GE Yun(葛筠)and ZHANG Lianzhong(章连众)**

Zhejiang Province Key Laboratory of Biofuel, College of Chemical Engineering and Material Science, Zhejiang University of Technology, Hangzhou 310014, China

Vapor-liquid equilibrium (VLE) data for water + 2-propanol + 1-butyl-3-methylimidazolium chloride ([bmim]Cl) were measured. Six sets of complete T, χ, y data are reported, in which the 2-propanol mole fraction on IL-free basis is fixed separately at 0.1, 0.2, 0.4, 0.6, 0.8, and approximate 0.98, while the IL mass fraction is varied from 0.1 to 0.8, in an interval of 0.1. The non-random-two-liquid (NRTL) and electrolyte non-random-two-liquid (eNRTL) equations are used to correlate the experimental data with satisfactory results. The ternary VLE behavior is also modeled with the parameters obtained by correlating two data sets, in which the mole fraction of 2-propanol on IL-free basis is approximately 0.1 and 0.98. In this way, the six sets of data are reproduced satisfactorily. With the eNRTL model, the root-mean-square deviation for temperature is 0.82 K and that for vapor-phase mole fraction is 0.0078. The influences of IL on activity coefficients and relative volatility of the volatile components are also graphically illustrated.

vapor-liquid equilibrium, eNRTL equation, ionic liquid, 2-propanol, activity coefficient, relative volatility

1 INTRODUCTION

In recent years, the utilization of ionic liquids (ILs) as entrainers in the extractive distillation of azeotropic or close boiling mixtures has gained considerable attention [1, 2]. Compared with conventional entrainers, ILs possess some special merits suitable for distillation, such as non-volatility, large liquidus range, high compatibility with many organic solvents. Usually, when an IL is introduced into an azeotropic mixture to be separated, the components change their non-ideality and activity coefficients to a different extent because the interactions between IL and each component are discriminating. It is desirable that the changes may enhance the relative volatility to break the azeotrope. The influences of IL on relative volatility depend mainly on the composition dependence of activity coefficients in the IL-containing mixture. Therefore, it is necessary to understand the changes of vaporliquid phase behavior arising from the addition of IL. At the present stage, such thermodynamic information is mainly obtained from experimental measurement of vapor-liquid equilibrium (VLE) data and by correlation using a model of excess Gibbs energy [3-10].

In our previous work [11], we developed a procedure for the experimental measurement and modeling of vapor-liquid equilibrium data for IL-containing ternary systems, taking the system water + ethanol + 1-hexyl-3-methylimidazolium chloride as a case study. Six sets of complete T, χ, y data were measured, in which two data sets were used for correlation of the non-random-two-liquid (NRTL) model [12] and the other four data sets were used for checking the model. Based on detailed discussion, the modeling of VLE behavior of a ternary system containing IL has been generally recommended by correlation of two ternary data sets, measured at a relatively wide range of IL mass fractions, while the mole fractions of the volatile quasibinary pair are distributed separately in the two diluted ends. In the following work, we have extended the procedure to several water-alcohol-IL systems [13-15].

As a continuation of our study, we will extend the experimental measurement and modeling to the system water (1) + 2-propanol (2) + [bmim]Cl (3) in present work. The modeling is based on T, χ, y data in a relatively wide range of IL mass fractions up to 0.8 and in a relatively complete composition range for the volatile binary pair. The effect of ILs on the mixture of water and 2-propanol was studied by Westerholt et al. for [HMIM][BTI] and [BMPYR][BTI] [6], and by Li et al. for [EMIM][BF4] [8]. In our previous work [16], we also studied the same ternary system, but the measurements were performed only in the 2-propanol-rich region and the highest IL mass faction was 0.65. To the best of our knowledge, there are no other VLE data for the ternary system.

2 EXPERIMENTAL

2.1 Materials

Water was doubly distilled. 2-propanol (analytical reagent grade, Sinopharm Chemical reagent Co. Ltd, with purity 99.9% checked by GC and a water mass fraction of 3.6×10−4indicated by Karl-Fischer analysis), 1-methylimidazole (99.5%, Yancheng MedicalChemical Factory), and 1-chlorobutane (chemical pure grade, Sinopharm Chemical reagent Co. Ltd) were used without further purification. [bmim][Cl] was prepared by simple reaction of 1-methylimidazole with 1-chlorobutane under a nitrogen atmosphere and purified by recrystallization in a mixture of ethyl acetate and acetonitrile. Before use, the IL was dried for 24 h under vacuum at 340 K to remove volatile impurities. Karl Fischer analysis showed typically a water mass fraction of 1.2×10−3in the IL. The IL was also checked by electrospray ionization mass spectrometry (ESI-MS), showing a single positive ion, m/z=139 ([bmim]).

2.2 Experimental apparatus

VLE data were measured by an ebulliometer described in detail previously [17]. Pressure was measured by a precision pressure gauge with an uncertainty of ±0.04 kPa. A 20 dm3glass container was used as pressure buffer connected to the ebulliometer. Temperature was measured by a standard platinum thermometer and a 6-1/2-digit multimeter. Uncertainty of the resistance measurement was ±8 mΩ, which is equivalent to ±0.08 K for temperature measurement.

2.3 Experimental procedure

The VLE data were determined in such a way that the IL mass fraction, w3, changed from high to low, while the mole fraction of 2-propanol on IL-free basis, χ2′, remained approximately unchanged. At IL mass fractions w3=0.6, 0.7, and 0.8, the measurements were performed at p=30 kPa in order to decrease the boiling point. It is commonly recognized that the activity coefficients in a liquid mixture depend strongly on composition, only weakly on temperature and very weakly on pressure. Therefore, the present measurements mainly reflect the composition dependence of activity coefficients.

At the beginning of measurement, samples of water, 2-propanol, and the IL were introduced into the ebulliometer. The water contents of 2-propanol and the IL were determined by Karl-Fischer analysis. Every sample added in or taken out of the ebulliometer was weighed with an electronic balance (Mettler-Toledo AL204) with an uncertainty of ±0.0002 g. Masses of each component added in the ebulliometer were calculated, so we have the overall synthetic masses for the first measurement. When the equilibrium was established, the vapor condensate was sampled and analyzed. As the IL is nonvolatile, the vapor phase is composed of water and 2-propanol. Vapor-phase composition was determined by analyzing the water content using the Karl-Fischer method. The uncertainty of the vapor-phase composition was estimated to be 0.0001 in water mole fraction or relatively 1%, whichever is the greater. Liquid-phase compositions were calculated according to the procedure presented in previous work [17]. The next measurement was carried out by replacement of certain amount of the mixture in the boiler with IL-free mixture of water and 2-propanol to keep χ2′ approximately unchanged. The measurement was repeated until w3was close to 0.1.

3 RESULTS and DISCUSSION

The experimental VLE data for the ternary system water (1) + 2-propanol (2) + [bmim]Cl (3) are listed in Table 1, with w3varied from 0.8 to 0.1 and χ2′ remained approximately unchanged. The pressure isabout 30 kPa for w3=0.8, 0.7, and 0.6, and 100 kPa for w3=0.5 to 0.1. The experimental points are regularly distributed at eight w3and six χ2′ levels. The liquid-phase compositions are reported in χ2′ and w3. Activity coefficients of water (γ1) and 2-propanol (γ2) and the relative volatility of 2-propanol to water (α2,1) are also reported. In the calculation of activity coefficients the vapor phase is regarded as an ideal gas and the saturated vapor pressures are calculated using parameters from literature [18].

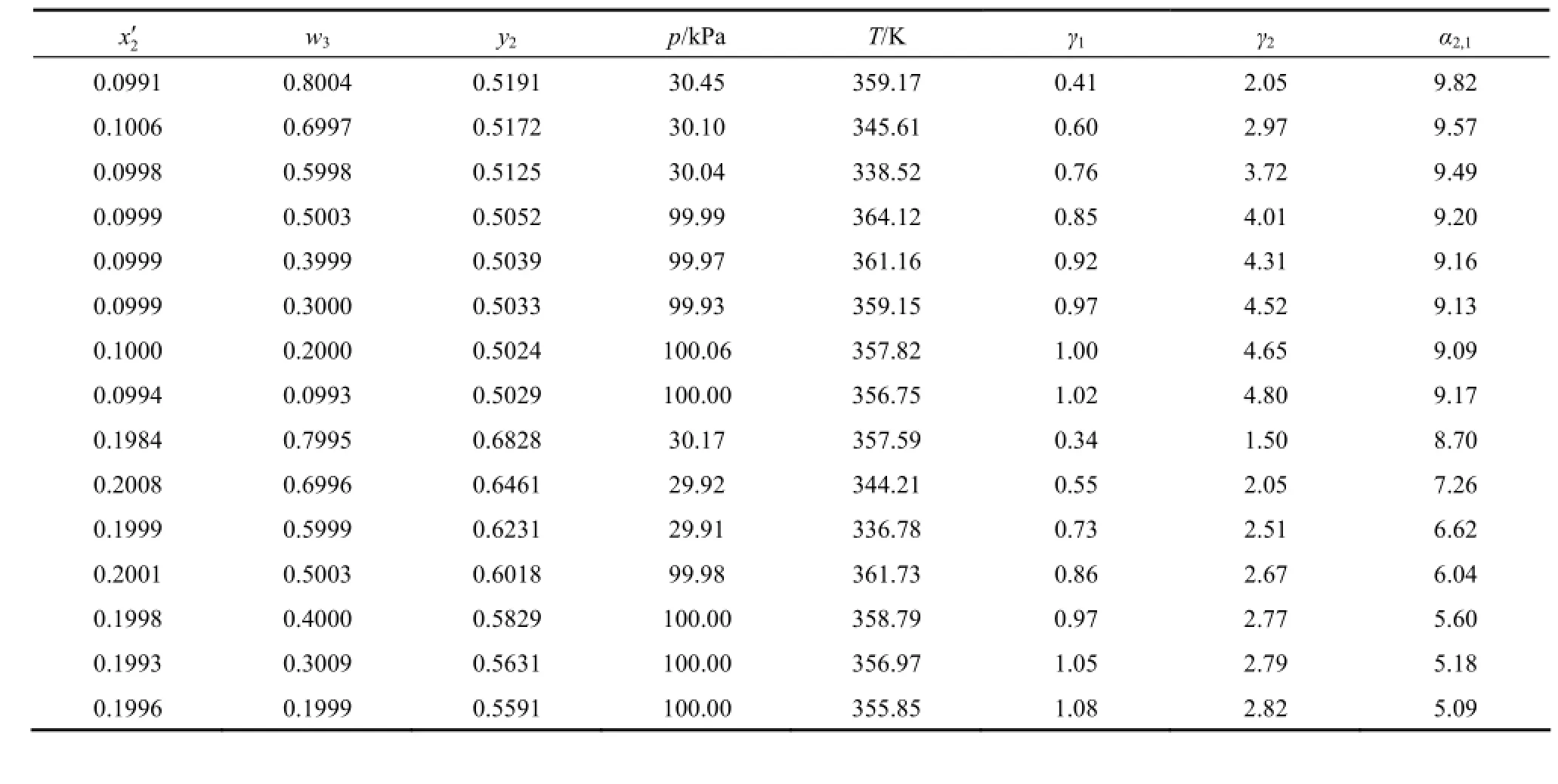
Table 1 Vapor-liquid equilibrium data for the ternary system water (1) + 2-propanol (2) + [bmim]Cl (3)

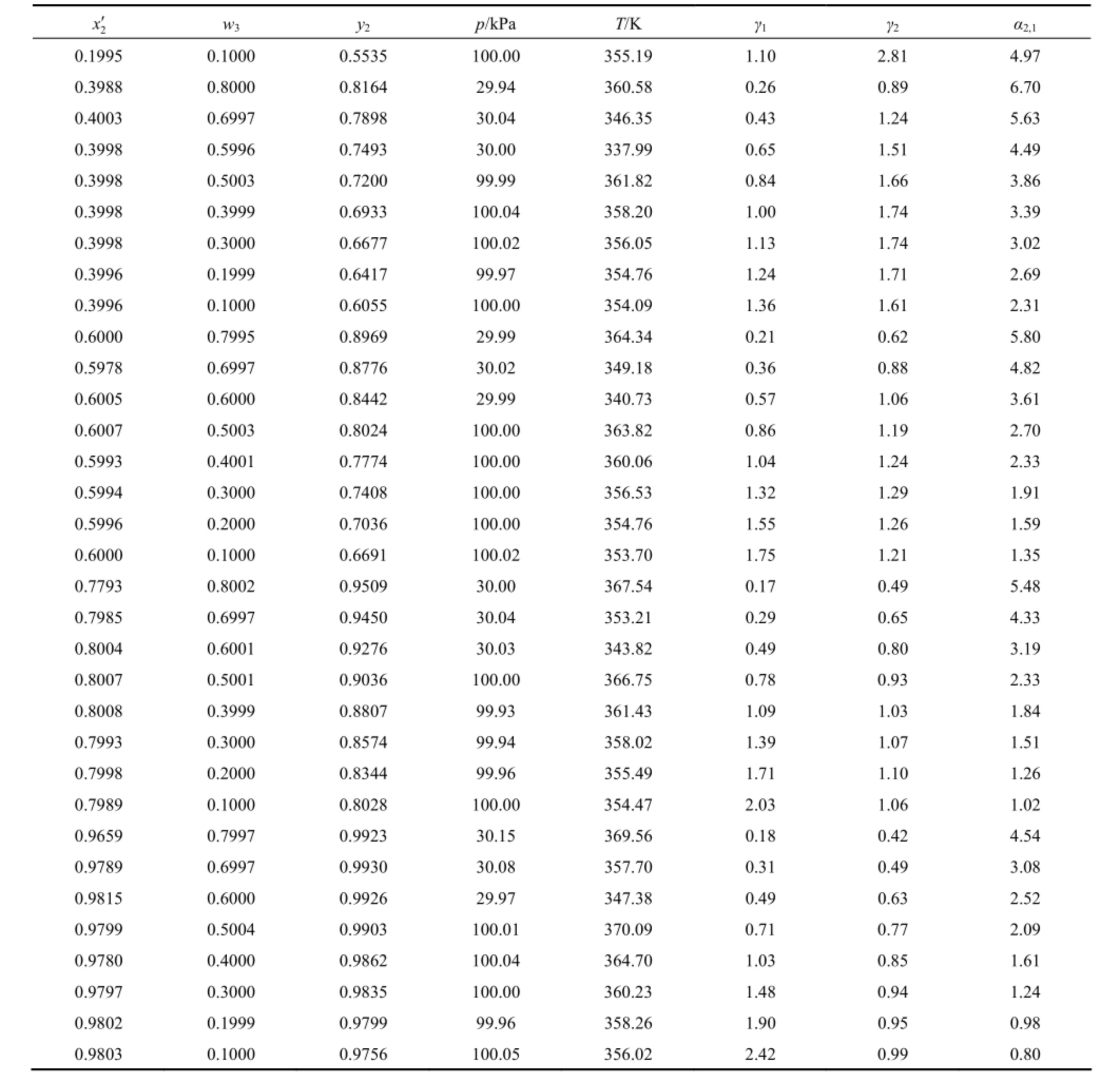
Table 1 (Continued)

In the measurements, temperature uncertainty was less than 0.04 K. As discussed in previous work [11, 16], the uncertainty for w3was estimated to be ±0.003. The uncertainty for molar composition of water or 2-propanol was estimated to be less than 1%.

NRTL [12] and eNRTL [19] equations are used to correlate the six data sets. For the eNRTL equation, expressions for the liquid-phase activity coefficients of volatile components in a ternary system containing a salt have been presented by Vercher and Rojo [20]. In the correlations, the binary parameters for water + 2-propanol are taken from literature [21]. While the non-randomness factors α13and α23are chosen at the most common value of 0.3 for the NRTL equation and 0.2 for the eNRTL equation, the binary parameters for water + [bmim]Cl and 2-propanol + [bmim]Cl areobtained by minimization of the following objective function:

in which N is the number of data points. The obtained parameters are used to calculate the ternary VLE data in comparison with the experimental values. Results are illustrated in Table 2, in which δTand δyare respectively the root mean square deviations of temperature and vapor-phase composition. It is obvious that eNRTL equation provides better results, with δT=0.77 K and δy=0.0074.

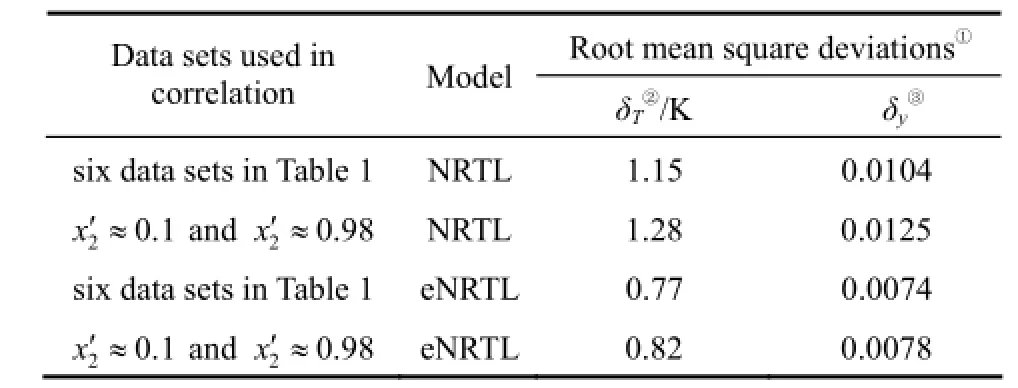
Table 2 Root mean square deviations δTand δyin calculation of VLE data of water (1) + 2-propanol (2) + [bmim]Cl (3) based on the NRTL and eNRTL equations

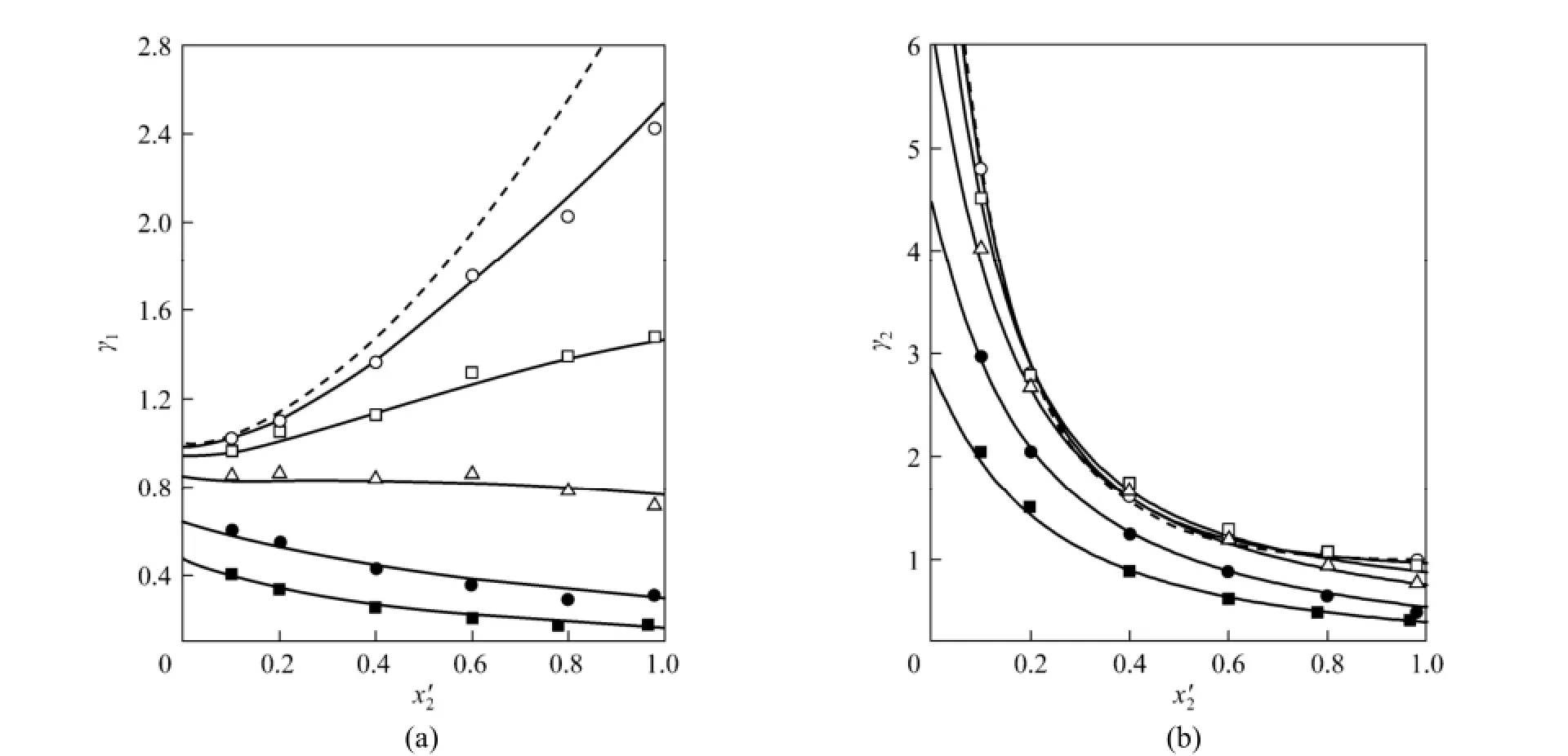
Figure 1 Experimental and calculated activity coefficients of water γ1(a) and 2-propanol γ2(b) in relation with mole fraction of 2-propanol on IL-free basis χ′2for the saturated mixture water (1) + 2-propanol (2) + [bmim]Cl (3)○ w3=0.1, p≈100 kPa; □ w3=0.3, p≈100 kPa; △ w3=0.5, p≈100 kPa; ● w3=0.7, p≈30 kPa; ■ w3=0.8, p≈30 kPa; lines: calculated by the eNRTL equation using parameters in Table 3; solid lines: calculated values at w3=0.1, 0.3, 0.5, 0.7, and 0.8 at relevant pressures; dashed lines: calculated values for the system water (1) + 2-propanol (2) at p=100 kPa

Following the procedure developed in previous work [11] for modeling ternary VLE behavior, the parameters obtained by correlation of two data sets at χ2′≈0.1 and χ2′ ≈ 0.98 are used to reproduce ternary VLE data and compared with the six sets of experimental data. Results are also shown in Table 2. The eNRTL equation provides better results, with δT=0.82 K and δy=0.0078. These deviations are almost the same as those by direct correlation of the six data sets. Therefore, the two data sets at χ2′≈0.1 and χ2′ ≈0.98 appear to be adequate for modeling the VLE behavior in the experimental composition range. The optimized binary parameters for the eNRTL equation are given in Table 3.

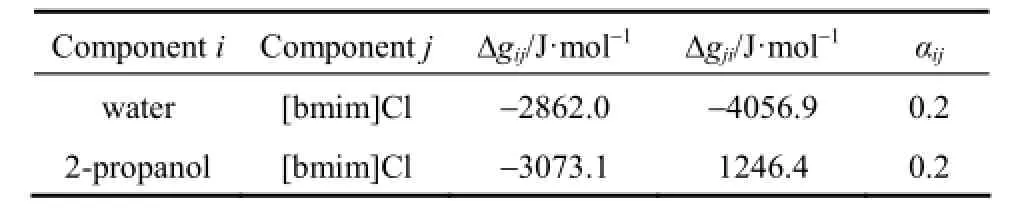
Table 3 Energy parameters Δgijand Δgjiand nonrandomness factors αijfor the eNRTL model obtained from correlation of ternary VLE data of water (1) + 2-propanol (2) + [bmim]Cl (3) using data sets at χ′2≈0.1 and χ′2≈0.98

VLE results calculated by using parameters in Table 3 and experimental data are also graphically compared in Figs. 1-3. Fig. 1 shows the relationship of γ1and γ2with χ2′. As the experimental data are distributed regularly at different w3, it is expedient to compare the calculated activity coefficients with experimental values at several fixed IL mass fractions, inrelation with χ2′. For the best illustration, typical results at w3=0 (no IL), 0.1, 0.3, 0.5, 0.7, and 0.8 are presented. While γ2decreases with increasing χ2′ at all given w3, γ1increases with increasing χ2′ at w3=0.1 and 0.3, and decreases with increasing χ2′ at higher IL mass fractions, namely at w3=0.5, 0.7 and 0.8. These trends are similar to those presented for the system water + 2-propanol + [bmim][OAc] [14]. On the other hand, the effect of IL mass fraction on the activity coefficients can be also observed in Fig. 1. Generally, both γ1and γ2decrease with increasing w3at the same χ2′.

The relative volatility of 2-propanol to water,is expressed asin which the ratiodepends weakly on temperature and has small change from 1.939 to 1.955 in the experimental temperature range. Therefore, the effect of IL on α2,1is mainly embodied in the ration of the activity coefficients. Although the decrease of γ1is beneficial for enhancement of the relative volatility, the decrease of γ2is undesirable. Effect of the IL on α2,1is shown in Fig. 2. While the addition of IL increases the relative volatility in the 2-propanol-rich region, showing a salting-out effect, there is a salting-in effect in the water-rich region. In Fig. 3, y2is shown in relation with χ2′. The addition of IL to water (1) + 2-propanol (2) azeotropic mixture leads to noticeable increase of vapor phase molar fraction of 2-propanol, and the azeotrope can be easily broken with small content of IL.

Figure 2 Experimental and calculated relative volatility of 2-propanol to water α2,1in relation with mole fraction of 2-propanol on IL-free basis χ′2for the saturated mixture water (1) + 2-propanol (2) + [bmim]Cl (3)○ w3=0.1, p≈100 kPa; □ w3=0.3, p≈100 kPa; △ w3=0.5, p≈100 kPa; ● w3=0.7, p≈30 kPa; ■ w3=0.8, p ≈ 30 kPa; lines: calculated by the eNRTL equation using parameters in Table 3; solid lines: calculated values at w3=0.1, 0.3, 0.5, 0.7, and 0.8 at relevant pressures; dashed lines: calculated values for the system water (1) + 2-propanol (2) at p=100 kPa

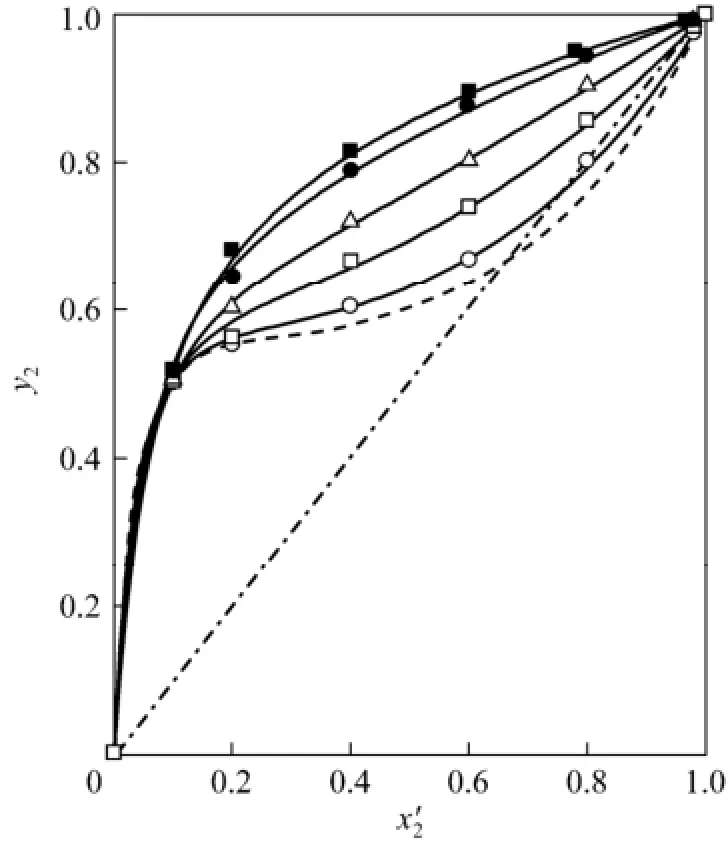
Figure 3 Experimental and calculated vapor phase mole fraction of 2-propanol, y2, in relation with mole fraction of 2-propanol on IL-free basis, χ′2, for the saturated mixture water (1) + 2-propanol (2) + [bmim]Cl(3)○ w3=0.1, p≈100 kPa; □ w3=0.3, p≈100 kPa; △ w3=0.5, p≈100 kPa; ● w3=0.7, p≈30 kPa; ■ w3=0.8, p≈30 kPa; lines: calculated by the eNRTL equation using parameters in Table 3; solid lines: calculated values at w3=0.1, 0.3, 0.5, 0.7, and 0.8 at relevant pressures; dashed lines: calculated values for the system water (1) + 2-propanol (2) at p=100 kPa

4 CONCLUSIONS

T, χ, y data are reported for the ternary system water + 2-propanol + [bmim]Cl. Six data sets are obtained in a relatively wide range of IL mass fractions up to 0.8 and at χ2′=0.1, 0.2, 0.4, 0.6, 0.8, and approximately 0.98. The NRTL and eNRTL equations are used to correlate the experimental data with satisfactory results. By correlating the two data sets using eNRTL equation, respectively, at χ2′=0.1 and χ2′ ≈ 0.98, all of the six data sets are well reproduced, with δT=0.82 K and δy=0.0078. Owing to the regular distribution of the experimental data, good agreement between experiment and calculation is graphically illustrated. Influence of IL on the VLE behavior of the volatile components is also presented.

NOMENCLATURE

Δg NRTL parameters

p pressure, kPa

T temperature, K

w mass fraction

χ mole fraction of liquid phase

y mole fraction of vapor phase

α12, α13, α23NRTL parameters

α2,1relative volatility of component 2 to component 1

γ activity coefficient

δ root mean square deviations

Superscripts

sat saturated vapor pressure of a pure component

′ prime symbol, indicating the quantity on IL-free basis

Subscripts

1, 2, 3 volatile (1, 2) or nonvolatile (3) component

REFERENCES

1 Seiler, M., Jork, C., Karvanou, A., Arlt, W., Hirsch, R., “Separation of azeotropic mixtures using hyperbranched polymers or ionic liquids”, AIChE J., 50 (10), 2439-2454 (2004).

2 Li, Q.S., Zhang, J.G., Lei, Z.G., Zhu, J.Q., Zhu, J.J., Huang, X.Q.,“Selection of ionic liquids as entrainers for the separation of ethyl acetate and ethanol”, Ind. Eng. Chem. Res., 48 (19), 9006-9012 (2009).

3 Shen, C., Li, X.M., Lu, Y.Z., Li, C.X., “Effect of ionic liquid 1-methylimidazolium chloride on the vapour liquid equilibrium of water, methanol, ethanol, and {water + ethanol} mixture”, J. Chem. Thermodyn., 43 (11), 1748-1753 (2011).

4 Lei, Z.G., Arlt, W., Wasserscheid, P., “Separation of 1-hexene and n-hexane with ionic liquids”, Fluid Phase Equilib., 241, 290-299 (2006).

5 Wang, J.F., Li, C.X., Wang, Z.H., Li, Z.J., Jiang, Y.B., “Vapor pressure measurement for water, 2-propanol, and their binary mixtures in the presence of an ionic liquid 1-ethyl-3-methylimidazolium dimethylphosphate”, Fluid Phase Equilib., 255 (2), 186-192 (2007).

6 Westerholt, A., Liebert, V., Gmehling. J., “Influence of ionic liquids on the separation factor of three standard separation problems”, Fluid Phase Equilib., 280, 56-60 (2009).

7 Zhao, J., Dong, C.C., Li, C.X., Meng, D., Wang, Z.H., “Isobaric vapor-liquid equilibria for ethanol-water system containing different ionic liquids at atmospheric pressure”, Fluid Phase Equilib., 242 (2), 147-153 (2006).

8 Li, Q.S., Xing, F.Y., Lei, Z.G., Wang, B.H., Chang, Q.L., “Isobaric vapor-liquid equilibrium for isopropanol + water + 1-ethyl-3-methylimidazolium tetrafluoroborate”, J. Chem. Eng. Data, 53 (1), 275-279 (2008).

9 Cai, J.L., Cui, X.B., Zhang, Y., Li, R., Feng, T.Y., “Isobaric vapor liquid equilibrium for methanol + methyl acetate + 1-octyl-3-methylimidazolium hexafluorophosphate at 101.3 kPa”, J. Chem. Eng. Data, 56 (6), 2884-2888 (2011).

10 Li, Q.S., Zhu, W., Wang, H.C., Ran, X.M., Fu, Y.Q., Wang, B.H.,“Isobaric vapor-liquid equilibrium for the ethanol + water + 1,3-dimethylimidazolium dimethylphosphate system at 101.3 kPa”, J. Chem. Eng. Data, 57 (3), 696-700 (2012).

11 Zhang, L.Z., Ge, Y., Ji, D.X., Ji, J.B., “Experimental measurement and modeling of vapor-liquid equilibrium for ternary systems containing ionic liquids: a case study for the system water + ethanol + 1-hexyl-3-methylimidazolium chloride”, J. Chem. Eng. Data, 54 (8), 2322-2329 (2009).

12 Renon, H., Prausnitz, J. M., “Local compositions in thermodynamic excess functions for liquid mixtures”, AIChE J., 14 (1), 135-144 (1968).

13 Geng, W., Zhang, L.Z., Deng, D.S., Ge, Y., Ji, J.B., “Experimental measurement and modeling of vapor-liquid equilibrium for the ternary system water + ethanol + 1-butyl-3-methylimidazolium chloride”, J. Chem. Eng. Data, 55 (4), 1679-1683 (2010).

14 Deng, D.S., Wang, R.F., Zhang, L.Z., Ge, Y., Ji, J.B., “Vapor-liquid equilibrium measurements and modeling for the ternary system (water + 2-propanol + 1-butyl-3-methylimidazolium acetate)”, Physics & Chemistry of Liquids, 50 (4), 504-512 (2012).

15 Deng, D.S., Wang, R.F., Zhang, L.Z., Ge, Y., Ji, J.B., “Vapor- liquid equilibrium measurements and modeling for ternary system water + ethanol + 1-butyl-3-methylimidazolium acetate”, Chin. J. Chem. Eng., 19 (4), 703-708 (2011).

16 Zhang, L.Z., Han, J.Z., Deng, D.S., Ji, J.B., “Selection of ionic liquids as entrainers for separation of water and 2-propanol”, Fluid Phase Equilib., 255 (2), 179-185 (2007).

17 Zhang, L.Z., Deng, D.S., Han, J.Z., Ji, D.X., Ji, J.B., “Isobaric vaporliquid equilibria for water + 2-propanol + 1-butyl-3-methylimidazolium tetrafluoroborate”, J. Chem. Eng. Data, 52 (1), 99-205 (2007).

18 Reid, R.C., Prausnitz J.M., Poling, B.E., The Properties of Gases and Liquids, 4th ed., McGraw-Hill, New York (1987).

19 Mock, B., Evans, L.B., Chen, C.C., “Thermodynamic representation of phase equilibria of mixed-solvent electrolyte”, AIChE J., 32 (10), 1655-1664 (1986).

20 Vercher, E., Rojo, F.J., Martínez-Andreu, A., “Isobaric vapor-liquid equilibria for 1-propanol + water + calcium nitrate”, J. Chem. Eng. Data, 44 (6), 1216-1221 (1999).

21 Marzal, P., MontÓn, J.B., Rodrigo, M.A., “Isobaric vapor-liquid equilibria of the water + 2-propanol system at 30, 60, and 100 kPa”, J. Chem. Eng. Data, 41(3), 608-611 (1996).

Received 2012-07-02, accepted 2013-01-28.

* Supported by the National Natural Science Foundation of China (20776132) and the Natural Science Foundation of Zhejiang Province (Y4100699).

** To whom correspondence should be addressed. E-mail: zhanglz@zju.edu.cn