两两可换的对合矩阵的群逆和可逆性

李虹晔, 崔小琴

(湖北师范学院 数学与统计学院， 湖北 黄石 435002)

1 引言及预备引理

幂等矩阵和对合矩阵是矩阵分析和算子理论中,两类最基本的矩阵,它们在矩阵分析、算子理论、统计学、经济学等中起着很重要的作用.在[1]中Graybill F A发现了两个幂等矩阵的线性组合在统计学中的作用.人们对于这两类矩阵组合的问题有不少的研究成果.例如:秩、可逆性、群逆、Drain逆等.在[2]和[3]中,Tian研究了两个幂等阵的线性组合及块为幂等阵的组合的分块矩阵的秩.在[4-7]中,Zuo和Gro J ,Trenkle G,以及Koliha J J,Rakocevic V研究了两个幂等矩阵的线性组合可逆性.在[8-11]中Deng和Zhang研究了幂等矩阵的和与差的Drain逆.本文先研究了两个幂等(对合)矩阵之差的可逆性和群逆的充要条件，并给出了三个两两可换的对合矩阵组合的群逆和可逆性的几个充要条件.

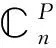
设P∈n×n,令,则P∈⟺A=I-2P∈,从而A∈⟺∈

设A∈n×n,如果存在唯一的X∈n×n使得AXA=A,XAX=X,AX=XA成立.则称A是一个群逆阵,且X是A的 群逆.用A#来表示A的群逆.容易证明,若A的群逆存在,则群逆一定唯一.且A是群逆阵⟺r(A)=r(A2) .用表示所有的n阶群逆阵组成的集合,即:

由文献[12]，我们有

引理1 设P是幂等矩阵,则存在n阶可逆阵S,使得

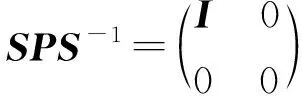
a)M#存在⟺A#与C#都存在,并且AπBCπ=0 .其中Aπ=I-AA#,Cπ=I-CC#.

b)如果M#存在,则

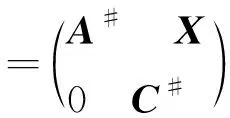
其中X=(A#)2BCπ+BCπ+AπB(C#)2-A#BC#.

2 主要结果及其证明

定理1 若P,Q是两个幂等矩阵,则有

1)P-Q可逆当且仅当I-PQ和P+Q-PQ可逆.

2)P-Q是群逆阵,则I-PQ和P+Q-PQ是群逆阵.

证明1):⟹ 设P-Q可逆，则(P-Q)2是可逆的. ∀x∈N(I-PQ),有

x=Px=PQx,QPx=QPPQx=QPQx=Qx,(P-Q)2x=(P+Q-PQ-QP)x=0

可得出x=0 .从而I-PQ是可逆的.

又因为∀x∈N(P+Q-PQ), 则有Px+(Q-PQ)x=0.两边同乘P,可得出Px=0,有

(Q-PQ)x=0=(Q2-PQ)x=(Q-P)Qx=0

又因为P-Q可逆,则

Qx=0,Px=Qx=0=(P-Q)x=0

P-Q可逆,则x=0.从而P+Q-PQ是可逆的.

⟸ 设I-PQ与P+Q-PQ都可逆,对∀x∈N(P-Q) ,则有

Px=Qx=PQx=QPx,PQPQx=PQPx

而

(I-PQ)(P+Q-PQ)x=(P+Q-PQ-PQP-PQ+PQPQ)x=

Px+Qx-2PQx-PQPx+PQPQx=0

因为I-PQ与P+Q-PQ都可逆,有x=0.从而P-Q可逆.

2)P-Q是群逆阵,由引理1，设

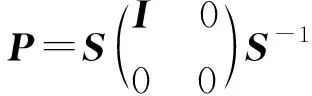
定理2 设A,B是两个对合矩阵，有

1)A-B可逆当且仅当3I+A+B-AB, 3I-A-B-AB可逆.

2)A-B是群逆阵,则3I+A+B-AB,3I-A-B-AB是群逆阵.

证明 设

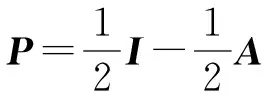
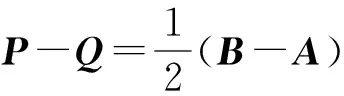
由定理1,我们得到A-B可逆，当且仅当 3I+A+B-AB和 3I-A-B-AB都可逆.同时由A-B是群逆阵，则3I+A+B-AB和3I-A-B-AB是群逆阵.

定理3 设A,B,C满足A2=B2=C2=I,且A,B,C两两可换

T=aI+bA+cB+dC+eAB+fBC+gAC+hABC

则有

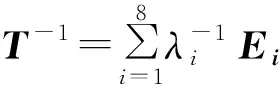
其中Ei和λi，i=1,2,…,8 定义如下:

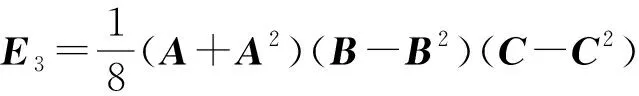
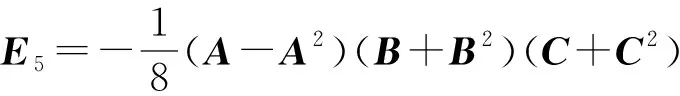
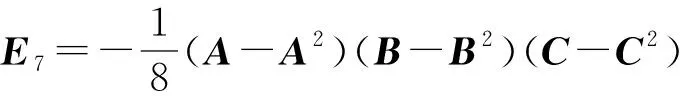
λ1=a+b+c+d+e+f+g+h,λ2=a+b+c-d+e-f-g-h

λ3=a+b-c-d-e+f-g+h,λ4=a+b-c+d-e-f+g-h

λ5=a-b+c+d-e+f-g-h,λ6=a-b+c-d-e-f+g+h

λ7=a-b-c-d+e+f+g-h,λ8=a-b-c+d+e-f-g+h

证明 由于A,B,C可对角化且它们两两可换,所以我们可以对A,B,C作如下的分解

A=S(I⊕I⊕I⊕I⊕-I⊕-I⊕-I⊕-I)S-1

B=S(I⊕I⊕-I⊕-I⊕I⊕I⊕-I⊕-I)S-1

C=S(I⊕-I⊕-I⊕I⊕I⊕-I⊕-I⊕I)S-1

那么可分别计算

A+A2=S(2I⊕2I⊕2I⊕2I⊕0⊕0⊕0⊕0)S-1

B+B2=S(2I⊕2I⊕0⊕0⊕2I⊕2I⊕0⊕0)S-1

C+C2=S(2I⊕0⊕0⊕2I⊕2I⊕0⊕0⊕2I)S-1

A-A2=S(0⊕0⊕0⊕0⊕-2I⊕-2I⊕-2I⊕-2I)S-1

B-B2=S(0⊕0⊕-2I⊕-2I⊕0⊕0⊕-2I⊕-2I)S-1

C-C2=S(0⊕-2I⊕0⊕-2I⊕0⊕-2I⊕0⊕-2I)S-1

从上面的表达式中,观察可得

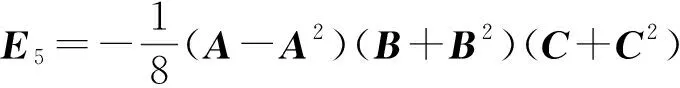
又因为

则可得

λ1=a+b+c+d+e+f+g+h,λ2=a+b+c-d+e-f-g-h

λ3=a+b-c-d-e+f-g+h,λ4=a+b-c+d-e-f+g-h

λ5=a-b+c+d-e+f-g-h,λ6=a-b+c-d-e-f+g+h

λ7=a-b-c-d+e+f+g-h,λ8=a-b-c+d+e-f-g+h

我们知道

参考文献：

[1]Fraybill FA.Introduction to matrices with applications in statistics[M].wadsworth publishing compang Inc.california,1969.

[2]Tian Yongge, Styan G P H.Rank equlities for idempotent and involutory matries[J]. Linear Algebra Appl,2001,335:101～117.

[3]Tian Yongge,Styan G P H.Rank equlitiesfor idempotent matries with applications[J].Journal of Computational and Applied Math, 2006,191:77～97.

[4]Zuo Kezheng.Nonsingularity of the difference and the sum of two idempotent matrices[J].Linear Algebra Appl,2010,433:476～482.

[5]Gro J,Trenkler G.Nonsingularity of the difference oftwo oblique projectors[J]. SIAM J Matrix Anal Appl,1999,21:390～395.

[6]Koliha J J, Rakocevic V.Invertibility of the difference of idempotents[J].Linear and Multilinear Algebra, 2003,51:97～110.

[7]Koliha J J, Rakocevic V, Straskraba I.The difference and sum of projectors[J]. Linear Algebra Appl,2004,388:279～288.

[8]Deng Chunyuan.The Drain inverses of products and difference of orthogonal projections[J].J Math Anal Appl,2007,335:64～71.

[9]Zhang S, Wu J.The Drain inverse of the linear combinations of two idempotents in the Banach algebra[J]．Linear Algebra Appl.2012,436:3132～3138.

[10]Deng Chunyuan.The Drain inverses of sum and difference of idempotents[J].Linear Algebra Appl,2009,430:1282～1291.

[11]Deng Chunyuan.Characterizations and representations of the group inverse involving idempotents[J]．Linear Algebra Appl,2011,434:1067～1079.

[12]Horn R A ,Johnson C R.矩阵分析[M]. 北京:人民邮电出版社，2005.

[13]Julio Benitez,Murat Sarduvan,Sedat Ulker,et al.On nonsingularity of combinations of three group invertible matrices and three tripotent [J].Linear Algebra Appl,2013,61:463～481.

[14]孙文瑜,何旭初.矩阵引论[M]. 南京:江苏科学技术出版社,1990.