Study on Kinetics of Iron Oxide Reduction by Hydrogen*

HOU Baolin (侯宝林), ZHANG Haiying (张海英), LI Hongzhong (李洪钟)** and ZHU Qingshan(朱庆山)**

State Key Laboratory of Multi-phase Complex Systems, Institute of Process Engineering, Chinese Academy of Sciences, Beijing 100190, China

1 INTRODUCTION

Although blast furnace is the main process equipment for the production of iron, sponge iron produced by direct reduction process becomes more and more popular because of the shortage of high-quality coking coals. In the process of producing sponge iron, hydrogen often is employed as the reductive gas. Better and full understanding of reduction mechanism and kinetics is extremely important for the technological development and process control. Although kinetic parameters have been extensively studied and widely used for scaling up and optimizing various reactors[1-6], reliable reaction kinetics have not yet been obtained up to date. Among the pending problems, the data of activation energy determined by difference authors [7, 8] for the reduction of iron oxide scattered over a wide range of at least one order of magnitude.The reasons are conceived as the following:

(1) The influence of diffusion was not completely eliminated. In the case of thermal gravitation (TG)study, the balance requires a quiescent atmosphere to get accurate measurement of mass, and gas velocity should not be higher than 0.01 m·s-1in most cases,which is too slow to eliminate the external diffusion resistance for fast reactions like iron oxide reduction.

(2) The mechanism of reaction for reduction of iron oxide varies with temperature. In-situ X-ray diffraction (XRD) [7, 8] investigations showed the dependence of reduction route on reduction temperature are as following:Obviously, it is difficult to distinguish each reaction separately using TPR (temperature program reduction)and TG techniques, especially when the heating-up speed is high [9-11] and the overlap of these reactions makes it difficult to obtain kinetic parameters.

Often, empirical equations, based on the assumption of different diffusion effects, were used to fit experimental data to determine the kinetic parameters.Over 20 semi-empirical equations [12] have been proposed for the reaction. However, it is often found that one set of experimental data could be well fit by many empirical equations [13], and then Arrhenius parameters obtained were of significant difference. It is therefore necessary to measure the kinetic parameter under conditions free of diffusion resistance.

In this work, the isothermal differential micropacked bed was employed to determine the intrinsic kinetics of iron oxide reduction by hydrogen. The internal and external diffusion resistances were eliminated through increasing the gas flow rate and decreasing the particle size to the critical levels. Reaction temperatures over 570 °C were selected to ensure that the reduction proceeds according to reactions (1) to (3),i.e., iron oxide (hematite) is reduced successively to magnetite, then wustite, and finally to iron sponge:

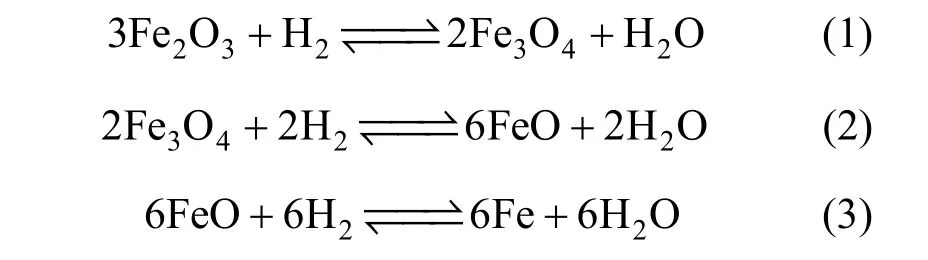
Under the condition that different reaction steps can be well distinguished, a process mass chromatograph was employed to measure the concentration of off-gas. The evaluation of the reaction order was not attempted in this paper since it has been fully understood [4, 7, 8, 14].

2 EXPERIMENTAL

Iron oxide powder with a mean diameter of 0.249 μm [purity＞99.9% (by mass), BET surface area 3.9 m2·g-1] were bought from Sinopharm Chemical Reagent Co., China; purity＞99.9% (by mass), BET surface area 3.9 m2·g-1. To obtain iron oxide with different particle sizes, the iron oxide powder was granulated by mixing with a small amount of water,followed by heating 600 °C for 4 h. The heated granulation was then crushed and sieved to obtain iron oxide particles of desire size ranges. The iron oxide particles were diluted with γ-Al2O3of 100 μm by 1∶1(mass ratio) to avoid the hot spot during the reduction process.

The experimental setup was illustrated by Fig. 1,where the diameter of bed was 8 mm. Quartz wool of 3 mm thickness was placed on the top of perorated plate to keep fine particulates of iron oxide from leaking out. The mixture of 0.1 g iron oxide and 0.1 g of γ-Al2O3was sandwiched between the top and bottom layers of 0.1 g of γ-Al2O3, which was place on the top of the quartz wool. A K-type thermal couple protected by quartz tube of 2 mm diameter was inserted into the iron oxide to measure the reaction temperature.

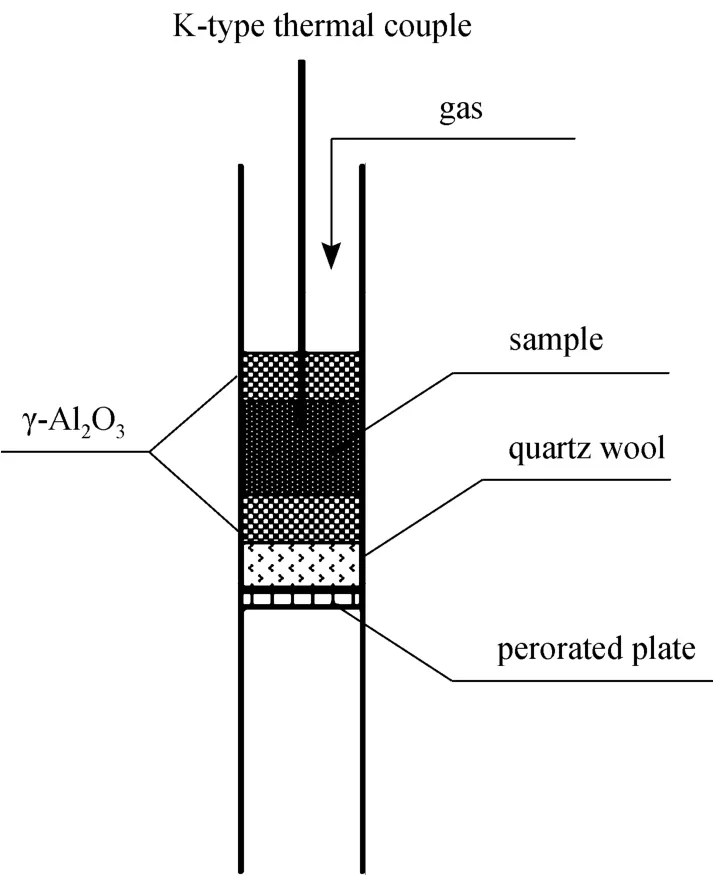
Figure 1 Scheme of packed bed

Gas flow rate was controlled by a mass flow meter (Seven Star Co., China). The quartz reactor and γ-Al2O3were treated by hydrogen at the 800 °C for 2 h at first to eliminate any measurement error. At the beginning of each experiment, the sample was purged by argon for half an hour for oxygen desorption, followed by heating the mixture of iron oxide and γ-Al2O3to specified temperatures in argon by the external electric heating coil. After the temperature was stabilized, the gas stream was switched to the mixture of hydrogen and argon, and in the meantime, the off-gas composition was measured by a process mass spectrograph (Proline, Ametek Process Instrument,USA) with the frequency of about 3 Hz.

3 KINETIC MODELS

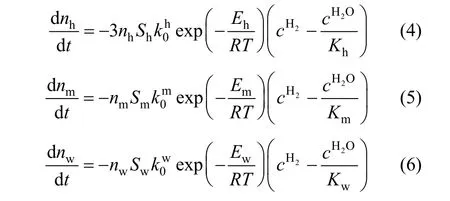
During the iron oxide reduction process by hydrogen, the reaction rates were often expressed by the following equations [1, 2, 6, 15-17]:are the pre-exponential factors for hematite reduced to magnetite, magnetite reduced to wustite and wustite reduced to metal iron by hydrogen respectively;Eh,EmandEware the corresponding activation energies.Sh,SmandSware the specific surface areas per mol for hematite, magnetite and wustite respectively;nh,nmandnware the mole of hematite,magnetite and wustite.cH2andcH2Oare the mole concentration of hydrogen and water vapor in the gas phase respectively;Kh,KmandKware the equilibrium constant for reactions (1) to (3) respectively. Because hematite is reduced to magnetite at first, then to wustite, it can be assumed thatSmandSwcan be expressed as the direct proportional function ofShas

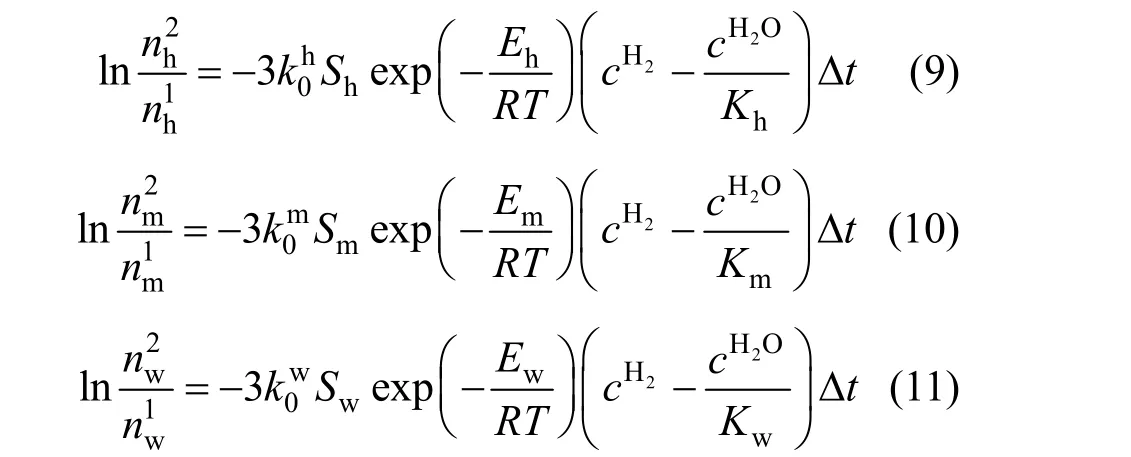
They apply when ∆tis small so that the gas composition can be regarded as constant.

Based on the total reduction degree of iron oxide,Eqs. (9), (10) and (11) can be rewritten as

which are valid when the three reaction steps can be well distinguished and ∆tis sufficiently small.

In the above equations, the reduction degreeα, or the conversion of iron oxide, is defined as:

whereugis the superficial gas ve2locity (m·s-1),Athe cross area of section of reactor (m),cinandcoutare the inlet and outlet gas concentration respectively(mol·m-3),is the mass of sample of Fe2O3(g),andthe molar mass of Fe2O3(g·mol-1).

Kinetic parameters for reduction of iron oxide can be evaluated only if three sequential steps are successfully distinguished under suitable conditions.Since reaction speed of hematite to magnetite is several orders of magnitude faster than the that of the two others, so it is easy to distinguish this step from the other two, which was clearly indicated by the TG experiments [7, 8]. In our experiment, this also has been confirmed, and the results were shown in Fig. 2. In this experiment, the mixture of hydrogen and argon(1∶20, volume ratio) (because the reaction order of hydrogen was not discussed in this paper, in the following experiment, the concentration of hydrogen kept unchanged) at the flow rate of 200 ml·min-1was employed to reduce iron oxide at the temperature 600 °C. When the conversionαreaches 11%, there is a complete peak of hydrogen which means the end of process from hematite to magnetite. When the total conversion reaches to 33%, there is significant change of slope of hydrogen concentration, indicating that the reaction transition from magnetite-wustite to wustite-iron.In this way, when the conversion of iron oxide reaches a certain value, three steps can be distinguished from each other under the condition of isothermal temperature.Eqs. (12), (13) and (14) can be rewritten as follows:

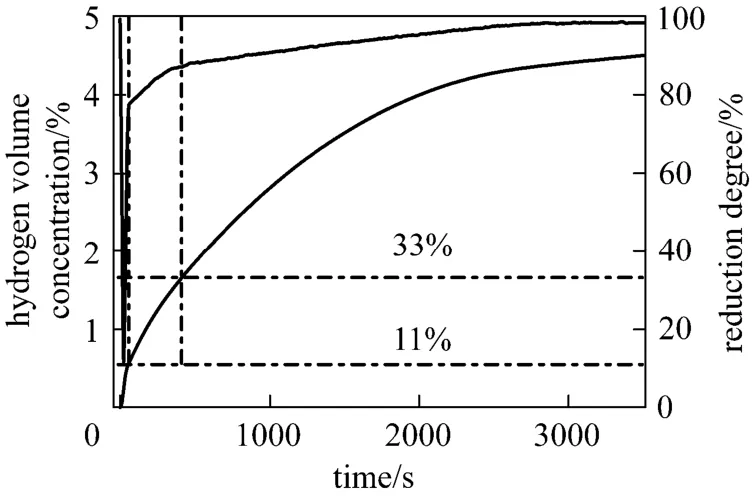
Figure 2 Concentration of hydrogen and degree of reduction with time during reduction of iron oxide by hydrogen (temperature 600 °C, hydrogen volume concentration 5%, sample mass 0.1 g, particle size 249 nm, velocity of gas 200 ml·min-1)

As shown in Eqs. (16), (17) and (18), if the three steps of this reduction can be very well distinguished the left side of Eqs. (16), (17) and (18) will keep constant during the iron oxide reduction process under the isothermal condition, which is going to be further referred to in the following section.

4 RESULTS AND DISCUSSION

4.1 Heat and mass transfer limitations

The influence of gas flow rate on overall reaction rates was first studied at 763 K to check the external diffusion effect. Illustrated in Fig. 3 is the dependence of the hydrogen concentration difference, ∆c, at inlet and outlet of the reactor on the reduction degree under constant residence time, which was realized by adding iron oxide particles into the reactor in proportion to the gas flow rate. It demonstrates that when the gas velocity is greater than 0.3 m·s-1, the dependence of ∆con the gas velocity is marginal, indicating that the external diffusion resistance can be ignored above 0.3 m·s-1.

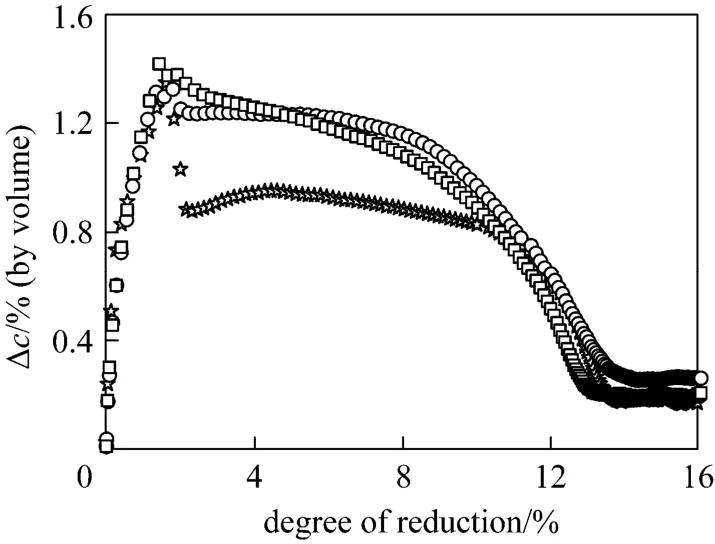
Figure 3 The influence of gas flow rate on the overall reaction rates (temperature 763K, average particle size 249 nm)☆ 0.2 m·s-1; ○ 0.3 m·s-1; □ 0.4 m·s-1

The influence of particle size on overall reaction rates was then investigated at the same temperature to check the internal diffusion effect at the gas velocity of 0.3 m·s-1. As shown in Fig. 4, ∆cincreases with decreasing particle size down to 0.045 mm, below which the dependence of ∆con the particle size is neglectable, demonstrating that the internal diffusion resistance can be eliminated when the particle size is less than 0.045 mm.

Figure 4 The influence of the particle size on the overall reaction rates (temperature 763K, gas velocity 0.3 m·s-1, sample mass 0.1 g, hydrogen volume concentration 5%)□ averaged diameter 249 nm; ○ 0.025-0.045 mm; △ 0.045-0.100 mm; ▽ 0.100-0.150 mm; ☆ 0.150-0.200 mm

The influence of heat transfer limitation was checked by the Mears criterion [18]:where ∆Hris the reaction heat,Ris the ideal gas constant,hgis the heat transfer coefficient between gas and solid which can be calculated based on the following expression [18]:

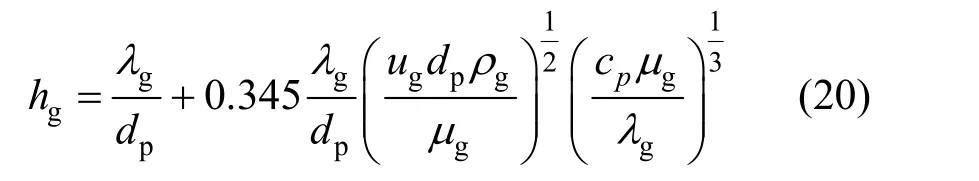
wherecpis the heat capacity of gas,λgis the heat conductivity of gas. The calculated ∆hM,heatis lower than 0.05 in this experiment, suggesting that the heat transfer does not play an important role in the kinetic study.

From the above investigations, the gas velocity of higher than 0.3 m·s-1and the average diameter of iron oxide of 249 nm were employed in the subsequent experiments to ensure that the kinetic experiments were performed under conditions free of internal and external diffusion resistance.

4.2 Kinetic parameters

In Fig. 5, the difference of hydrogen concentration between inlet and outlet are shown from 713 K to 763 K. In these experiments, 0.1 g of average diameter 249 nm iron oxide was used, the mixture of hydrogen and argon (1∶20, volume ratio) employed as the reducing gas, and the velocity of gas was set as 0.3 m·s-1. As shown in Fig. 5, the difference of hydrogen concentration between inlet and outlet first increases to a maximum value, and then decreases gradually.The main reason leading to this phenomenon may be the switch of gas stream from pure argon to the mixture of argon and hydrogen at the beginning of reaction, when the reactor was preheated to the specified temperature. Meanwhile, the mass spectrograph began to characterize the concentration of hydrogen of off-gas. Therefore, due to the gas axial diffusion and back mixing in the pipe and reactor, a certain time was need to reach the maximum difference of hydrogen concentration. In order to more accurately evaluate the intrinsic kinetic parameters, only those values after the blue dot line in Fig. 5 were employed to solve the reaction kinetic parameters.

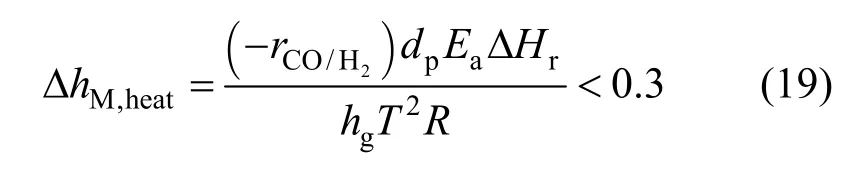
To evaluate the kinetic parameters,is plotted with the reduction degree in Fig. 6. When the reduction degree is lower than a certain value, the change of right side of Eq. (16) can be neglected at certain temperature and took as a constant. However,the values deviate significantly from a constant at higher reduction degree, which can be explained by that a little product of Fe3O4further reduced to FeO by hydrogen, and this kind of phenomena becomes more and more serious at the higher temperature.Therefore, in order to avoid this kind of inaccuracy,those values of right side of Eq. (16) were used to solve the intrinsic kinetics parameters with the reduction degree below 6%. As shown in Fig. 7, the apparent activation energy was calculated to be 105.4 kJ·mol-1, and the pre-exponential parameter was calculated to be 4.6×104m·s-1.

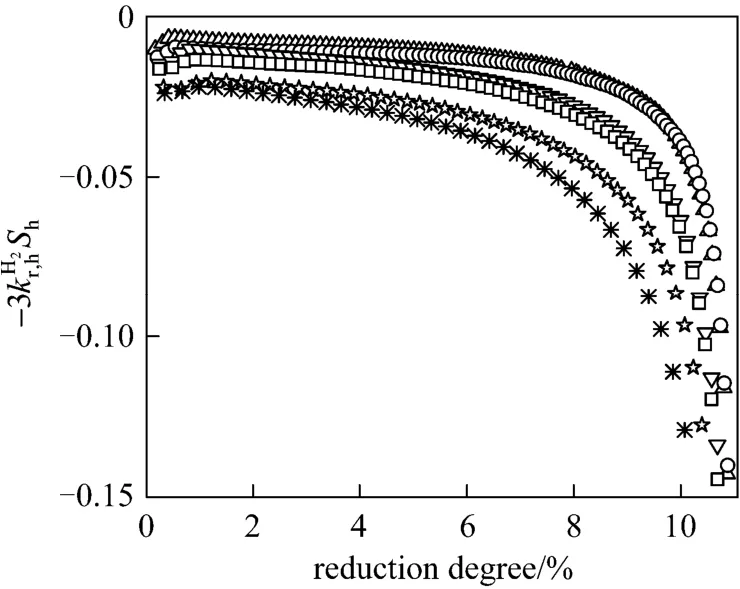
Figure 6 Plot of - against degree of reduction of iron oxide△ 713 K; ○ 723 K; ▽ 733 K; □ 743 K; ☆ 753 K;763 K

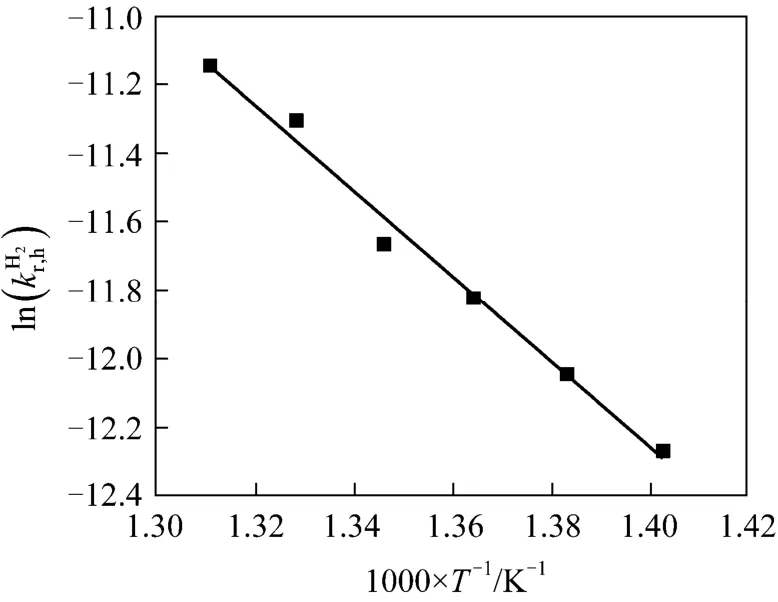
Figure 7 Kinetic parameters of reducing Fe2O3 to Fe3O4 by hydrogen

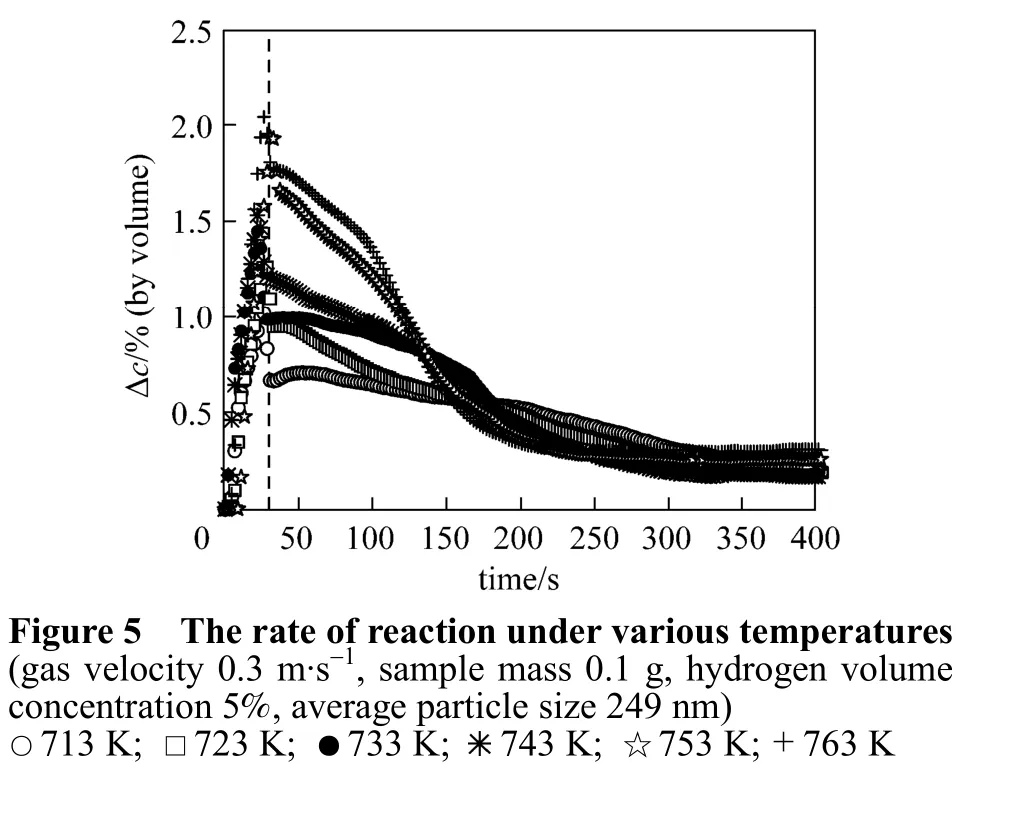
In Fig. 8, the difference of hydrogen concentration between inlet and outlet are shown at the temperatures from 863 K to 903 K. In these experiments, the conditions are the same as in Fig. 5. Due to the same reasons with Fig. 5, at the beginning of reaction, the difference of hydrogen concentration increased to reach the maximum value in a short time. When the temperature is higher than 863 K, the reaction of Fe2O3reduced to Fe3O4was very fast and seriously influenced by external mass transfer, so as shown in Fig. 8 the increase of the overall reaction rate is not significant with increasing the reaction temperature. However, when the reaction of Fe3O4to FeO started, the influence of temperature on the overall reaction rate became significant. With the sample further reduced,FeO was reduced to Fe. However, because the product of Fe tends to sinter, the reaction is controlled by the internal diffusion of reducing gas, the influence of temperature on the reaction rate becomes marginal at the final stage of reduction, and this also can be validated by the scanning electron microscope (SEM)picture of products. As shown in Fig. 9, the metallization ratio of all products is higher than 90%, the reaction times were 3 h.

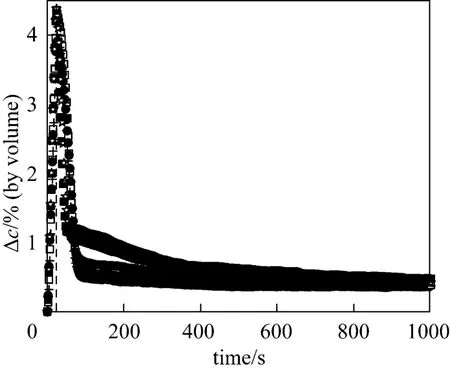
Figure 8 The rate of reaction under the various temperatures (gas velocity 0.3 m·s-1, sample mass 0.1 g, hydrogen volume concentration 5%, average particle size 249 nm)● 863 K; + 873 K; □ 883 K; ☆ 893 K; ■ 903 K

In Fig. 10, the change of right side of Eq. (17)with reduction degree is shown. Based on the same reason, the values of right side of Eq. (17) were employed but only the data with the reduction degree less than 24% were used. The activation energy of the reduction of Fe3O4to FeO by hydrogen can be evaluated with Fig. 11. The activation energy is 131.5 kJ·mol-1and the pre-exponential parameter is 1.3×105m·s-1.

Due to the sintering of newly formed metallic iron, the values of right side of Eq. (18) are shown only for the reduction degree is from 35% to 60% in Fig. 12, and these values are employed to solve the intrinsic kinetic parameters for the reaction of reducing FeO to Fe by hydrogen. As shown in Fig. 13, the activation energy for reduction of FeO to Fe by hydrogen is 76.0 kJ·mol-1, and the pre-exponential parameter is 9.5 m·s-1.

Thus, the reaction kinetics can be summarized as follows;

Figure 9 SEM image of iron oxide and as-reduced iron at various temperatures (a: iron oxide; b: iron from reduction at 590 °C;c: iron from reduction at 600 °C; d: iron from reduction at 610 °C; e: iron from reduction at 620 °C; f: iron from reduction at 630 °C)

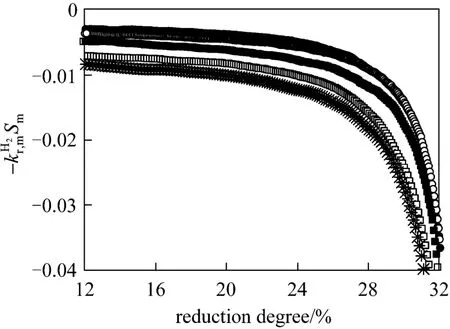
Figure 10 Plot of - against degree of reduction of iron oxide● 863 K; ○ 873 K; ■ 883 K; □ 893 K;903 K

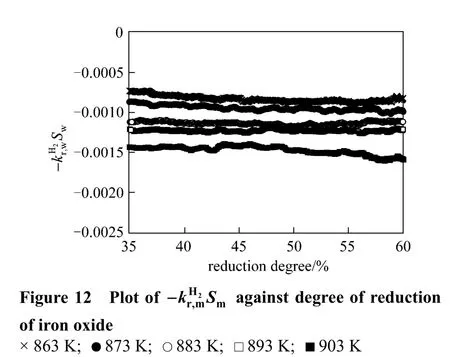
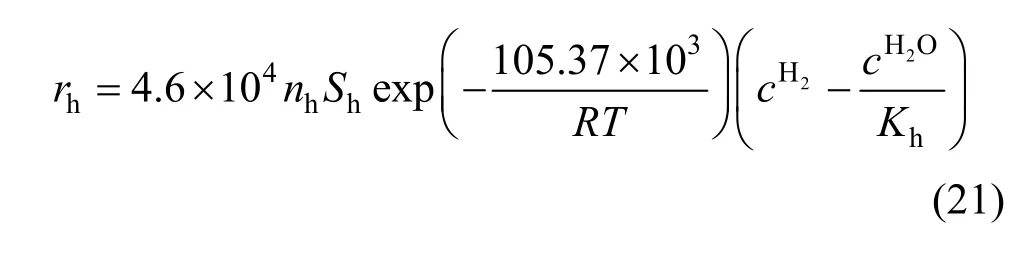
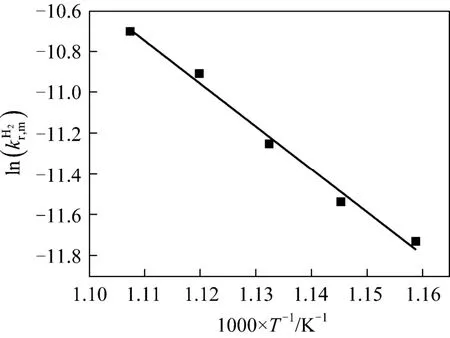
Figure 11 Activation energy plot for reducing Fe3O4 to FeO by hydrogen

Figure 13 Kinetics parameters of reducing FeO to Fe by hydrogen

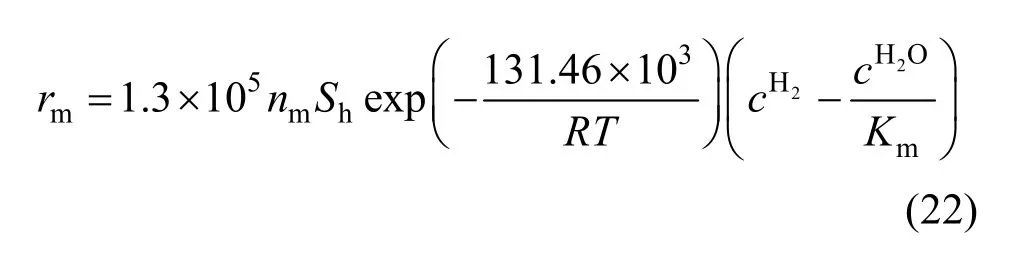
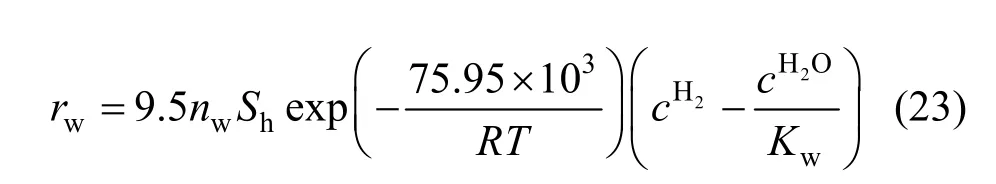
It should be noted that the influence of sintering of product iron on the reduction rate was not considered during evaluating the kinetic parameters. They are therefore not applicable when the newly-formed iron product has high tendency to sinter during the reduction process.

4.3 Validation experiments

Reduction experiments were performed to validate the intrinsic kinetics, where 1.5 g iron oxide powder having the average diameters of 249 nm with a surface area of 239 m2·mol-1was employed. To keep the hydrogen concentration nearly constant in the whole reduction process, the mixture of hydrogen and argon (1∶1, volume ratio) was used, and the velocity of gas was set to 2 m·s-1. The maximum difference of hydrogen concentration between inlet and outlet was characterized to be lower than 0.5% during the experiment by the on-line mass spectrograph. When the reaction was completed, the temperature of reactor was cooled to room temperature in argon to avoid the re- oxidation of the reduced sample. The reduction degree of sample was measured by the chemical titration method. The reduction process can be simulated based on the equations (24), (25) and (26), simplified from equations (4), (5) and (6) due to the reasons that the variation of hydrogen concentration was marginal(less than 0.5%) during the reduction process. The equations can be solved by the fourth order of Runge-Kutta method with suitable mesh grid, together with initial conditions expressed by Eq. (27).

The experimental results are shown in Fig. 14,together with the calculated results. It is clear that the prediction from the newly established kinetic model shows a good agreement with the experimental data.

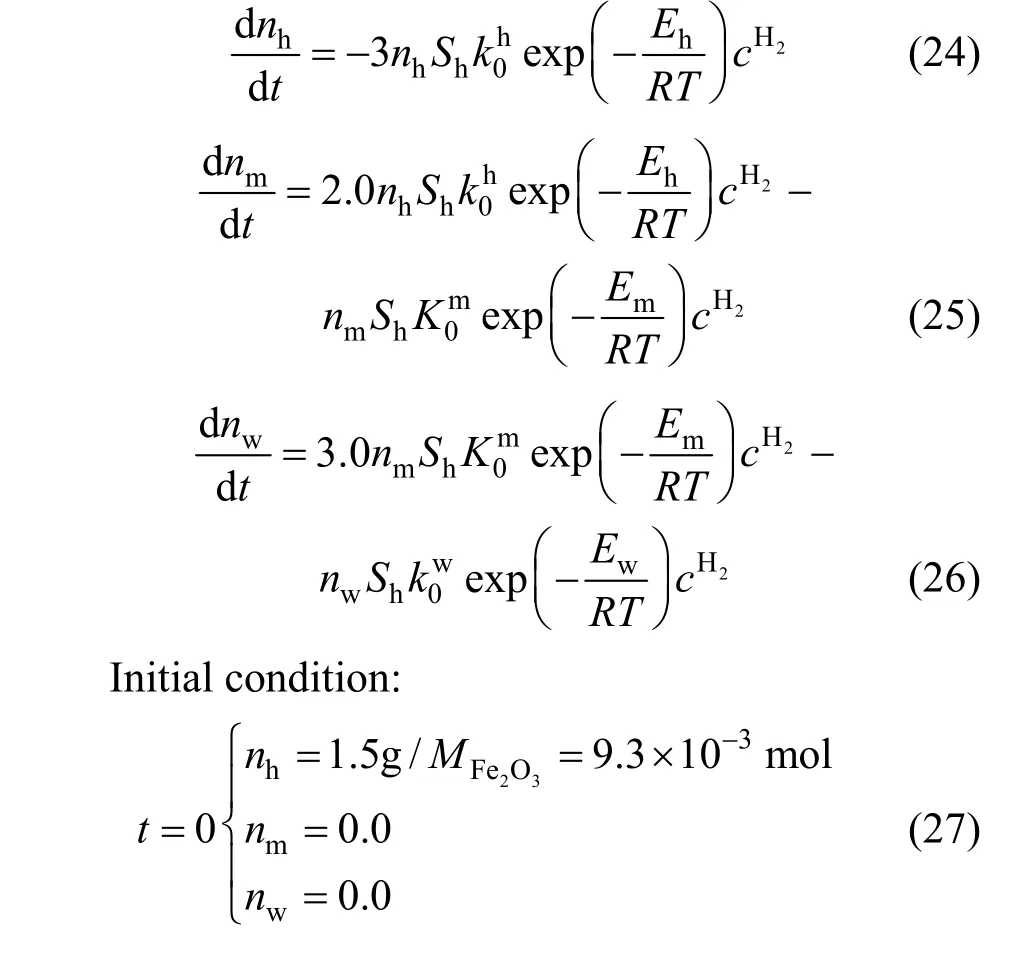
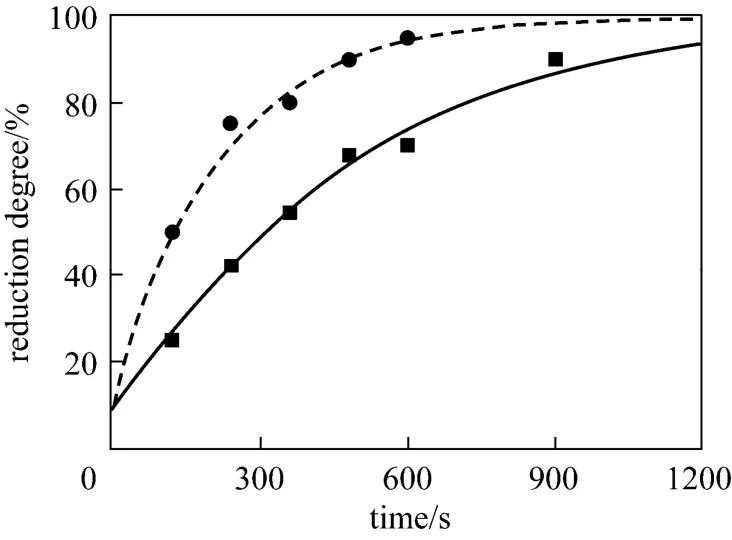
Figure 14 The comparison between simulation results and experimental data for reducing iron oxide (sample mass 1.5 g, hydrogen volume concentration 50%, gas velocity 2 m·s-1)■ 500 °C exp.; ● 550 °C exp.; 500 °C simulation;550 °C simulation

5 CONCLUSIONS

Isothermal method for evaluated kinetic parameters of iron oxide reduction by hydrogen is reasonable.Three steps of reaction above can be successfully distinguished from each other. The reaction kinetics parameters were measured under conditions free of the internal and external diffusion resistance. The activation energy was thus evaluated together with the pre-exponential parameter for reactions of Fe2O3to Fe3O4, Fe3O4to FeO, and FeO to Fe. The kinetic equations have been validated by a group of specially designed reduction experiments.

NOMENCLATURE

1 Tsay, Q.T., Ray, W.H., Szekely, J., “The modeling of hematite reduction with hydrogen plus carbon monoxide mixtures: Part II. The direct reduction process in a shaft furnace arrangement”, AIChE J., 22,1072-1079 (1976).

2 Tsay, Q.T., Ray, W.H., Szekely, J., “The modeling of hematite reduction with hydrogen plus carbon monoxide mixtures: Part I. The behavior of single pellets”, AIChE J., 22, 1064-1072 (1976).

3 Warner, N.A., “Reduction kinetics of hematite and the influence of gaseous diffusion”, Trans. Metall. Soc. AIME, 230, 1631-1676 (1964).4 Spitzer, R.H., Manning, F.S., Philbrook, W.O., “Generalized model for the gaseous, topochemical reduction of porous hematite spheres”,Trans. Metall. Soc. AIME, 236, 1715-1723 (1966).

5 Jozwiak, W.K., Kaczmarek, E., Maniecki, T.P., Ignaczak, W.,Maniukiewic, W.Z., “Reduction behavior of iron oxides in hydrogen and carbon monoxide atmospheres”, Appl. Catal. A, 326, 17-27 (2007).

6 Srinivasan, N.S., Staffansson, L., “A theoretical analysis of the fluidized-bed process for the reduction of iron ores”, Chem Eng Sci, 45,1253-1265 (1990).

7 Pineau, A., Kanari, N., Gaballah, I., “Kinetics of reduction of iron oxides by H2: Part I: Low temperature reduction of hematite”,Thermochim. Acta, 447, 89-100 (2006).

8 Pineau, A., Kanari, N., Gaballah, I., “Kinetics of reduction of iron oxides by H2: Part II. Low temperature reduction of magnetite”,Thermochim. Acta, 456, 75-88 (2007).

9 Piotrowski, K., Mondal, K., Lorethova, H., Stonawski, L.,Szymanski, T., Wiltowski, T., “Effect of gas composition on the kinetics of iron oxide reduction in a hydrogen production process”, Int.J. Hydrogen Energy, 30, 1543-1554 (2005).

10 Heidebrecht, P., Galvita, V., Sundmacher, K., “An alternative method for parameter identification from temperature programmed reduction(TPR) data”, Chem Eng Sci, 63, 4776-4788 (2008).

11 Lin, H.Y., Chen, Y.W., Li, C., “The mechanism of reduction of iron oxide by hydrogen”, Thermochim Acta, 400, 61-67 (2003).

12 Szekely, J., Evans, J.W., Sohn, H.Y., Gas-Solid Reactions, Academic Press, London, 100-110 (1976).

13 Ortega, A., “Some successes and failures of the methods based on several experiments”, Thermochim Acta, 284, 379-387 (1996).

14 Bohn, C.D., Cleeton, J.P., Müller, C.R., Davidson, J.F., Hayhurst,A.N., Scott, S.A., “The kinetics of the reduction of iron oxide by carbon monoxide mixed with carbon dioxide”, AIChE J, 56,1016-1029 (2010).

15 Srinivasan, N.S., “Reduction of iron oxides by carbon in a circulating fluidized bed reactor”, Powder Technol, 124, 28-29 (2002).

16 Zhao, Y., Shadman, F., “Reduction of ilmenite with hydrogen”, Ind.Eng. Chem. Res., 30, 2080-2087 (1991).

17 Sun, K., Lu, W.K., “Mathematical modeling of the kinetics of carbothermic reduction of iron oxides in ore-coal composite pellets”,Metall. Mater. Trans. B, 40, 91-103 (2009).

18 Fogler, H.S., Elements of Chemical Reaction Engineering, Prentice Hall Press, New York (2005).