Research on Complex Refraction Indices of Expanded Graphite
2011-07-25DOUZhengwei豆正伟LIXiaoxia李晓霞ZHAOJijin赵纪金
DOU Zheng-wei(豆正伟),LI Xiao-xia(李晓霞),ZHAO Ji-jin(赵纪金)
(State Key Laboratory of Pulsed Power Laser Technology,Key Laboratory of Infrared and Low Temperature Plasma of Anhui Province,Electronic Engineering Institute of PLA,Hefei 230037,Anhui,China)
Introduction
The expanded graphite(EG)is a kind of graphite compound with low density,and can be used as interfering material in IR and MMW bands[1-2].Its electromagnetic property can be changed by mixing different matters into it,which leads to potential military use.At present,there are two ways to estimate its extinction effect.One is the dynamic way based on a screening smoke box,and the other is the static way by spreading the EG on a transparent plank to test its transmissivity.The scattering theory of particles can be used to analyze the extinction effect of graphite from its refractive index,by which the experiment results can be approved.
Currently,there are many ways to test the refractive index of particles,such as dynamic-static light scattering test[3],forward complex scattering light field method[4],the inversion ways based on Mie theory and Kramers-Kronig(K-K)relations[5]or on the T-matrix theory and K-K relations[6],etc.Since the light scattering theory is needed in these methods,at least,the shape and grain distribution of particles have to be approximated,and it leads to inevitable experiment errors.
In this paper,we propose a method to measure the reflection spectrum of EG pellet and calculate its refractive indices by using K-K relations and Bruggeman effective medium approximation(EMA).The methods usually used to calculate the effective refractive index are Maxwell Garnett model and Bruggeman model[7],and the latter is suitable to study EG’s effective dielectric constant.
Huffman theoretically proved that the bulk optical constants,without modification,may not apply to small particles if the size of particles is much smaller than the wavelength of an incident light.Based on the results of strict extinction experiments and theoretical extinction calculation of SiO2particles less than 0.1 μm,it comes out that the extinction curve derived from bulk refractive index coincided with experiment result better[8].The size of EG particles in this paper is about tens to hundreds of microns,and its optical constant in 5-10 μm can be considered as no different from that of the bulk material.Thus,the calculated refractive index of the bulk material can be used for the particles in smoke screen.
1 Calculation of Refractive Indices
According to the K-K relations[8],if the reflectivityR(λ)of a kind of materials to an normal incident light(λ=0 - ∞)is given,its phase shiftΘ(λ)can be expressed as
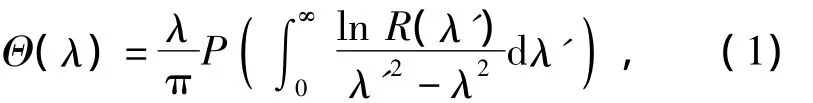
wherePis the principal value of the Cauchy’s integral.
In fact,only the reflectivityR(λ)in bandλaλbcan be tested owing to the limits of the test equipment,and reflectivitiesR1(λ)andR2(λ)outsideλa-λbcan be obtained by using empirical formula or constant extrapolation method.Thus,Θ(λ)can be expressed as

Suppose the complex refractive index of the material to bem=n+ik,the real partnand the imaginary partkcan be expressed as

For non-ferromagnetic EG,the relation between the refractive indexmand the dielectric constantεcan be written as

The Bruggeman effective medium approximation can be described as[9]
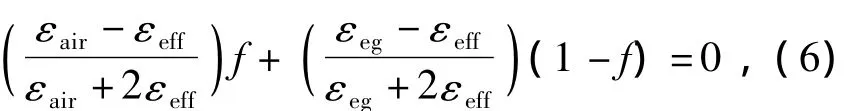
wherefis the volume ratio of air in material,εeg,εairandεeffare the dielectric constants of an EG pellet,air and EG powder,respectively,andεairis 1.
Θ(λ)can be obtained from K-K relations,then the refractive index of the EG pellet can be calculated by using Eq.(3 - 4),andεegby Eq.(5).The volume change of EG due to pelleting can be measured,and thenfwill be obtained.Ultimately,the refractive index of EG can be deduced afterεeffis known by using Eq.(6).
2 Experiments and Measurements
Take a certain volume of EG made in Qingdao Yingshida Graphite Co.Ltd.,EV=100 - 400,then crush gently and press into pellet,and measure the thicknesshand diameterdof the pellet,calculate its volume.
The specular reflection spectra in the 2.5 - 25 μm band can be measured by FT spectrometer typed Nicolet 8 700 made by American Thermo-electric,and the incident angle is 18 °.Those in the UV-VIS-IR band can be measured by the spectrophotometer typed U-4100 made by Hitachi,Japan.And the micro morphology of EG can be observed by using SEM typed JSM-6700 made by Japan Electronic.
3 Results and Discussions
The reflection spectrum of an EG pellet in IR band(2.5 - 25 μm)is shown in Fig.1,and that in 0.24 -2.6 μm band is shown in Fig.2.

Fig.1 Mid-far-IR reflection spectrum of EG pellet
For the measurement of reflection spectra,the reflection is specular in the FT-IR spectrometer,while those in the UV-VIS-NIR spectrophotometer are diffuse reflection integrated by integrating spheres.Therefore,the latter may be higher than the former at the same wavelength if the surface of EG pellet is not smooth enough.Comparing Fig.1 and Fig.2,it is found that there is a small difference between two reflectivity values at about 2.5 μm,which implies that the reflection of a smooth EG pellet in 5 -10 μm band could be supposed as a specular reflection.
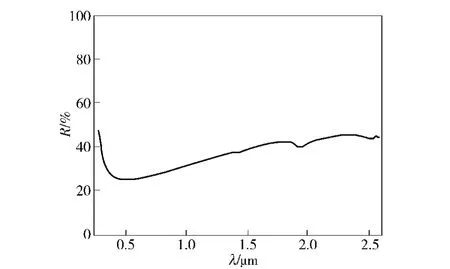
Fig.2 UV-VIS-NIR reflection spectrum of EG pellet
According to the classical oscillator model of light interaction with material,when the frequency of an incident wave is much higher than the oscillation frequencies of crystal lattices and electrons in a material,the relation between the normal reflectivityR1(λ)of the material and the wavelengthλcan be described as[8]

wherePis 3 -4,andAis determined by the reflectivity.The reflectivity at the high frequency outside the effective range of the spectrometer can be deduced by using Eq.(7),and that at the lower frequency,can be extrapolated by using a constant.
In the case of EG pellets,the value ofR2(λ)can be taken as 0.75 in the wavelengths beyond 25 μm,andp=3.5 in the high frequencies.R1(λ)can be expressed as 46.7λ3.5,based on the reflectivity at the wavelength of 0.24 μm.Therefore,the refractive index of EG pellet is calculated first,and then,that of EG can be calculated by using EMA,as shown in Fig.3 -4.
4 Error Analysis
4.1 Influence of Incident Angle
The normal reflectivityR(λ)in Eq.(2)can not be measured by FT-IR directly,which can only determine the reflectivity at the incident angle of 18°.Asnis 2-5 andkis 1-4,R(λ)can be calculated by using Eq.(8),andRi(λ)at the incident angle ofΘican be calculated by using Eq.(9).The differences betweenR(λ)andRi(λ)are shown in Tab.1,which are obviously very small.

Fig.3 Real part of refractive index of EG
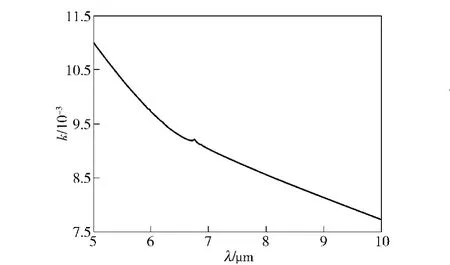
Fig.4 Imaginary part of refractive index of EG

Tab.1 Reflectivity differences between normal and oblique incidences
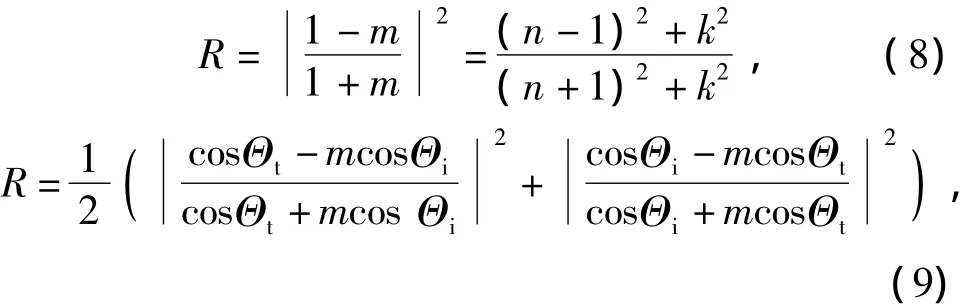
whereΘtis given by
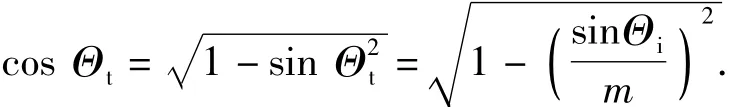
4.2 Effect of Extrapolation
In mid band,the minimum change of the phase shift of reflectivity occurs while reflectivity extrapola-tions vary in the long or short wavelength band[10].For the refractive index in 5 -10 μm band,R2(λ)can be supposed as 12.66λ2.5,24.3λ3,46.7λ3.5or 89.9λ4,respectively,andR1(λ)as 0.75.The refractive indices are extrapolated from the reflectivity in high frequency band,as shown in Fig.5 - 6.IfR1(λ)is taken as 0.65,0.75,0.85 or 0.95,andR2(λ)as 46.7λ3.5,the calculation results are shown in Fig.7 -8.
With reference of Fig.5 -8,bothnandkchange little with different extrapolations.Furthermore,for the wavelength below 0.24 μm,the longer the wavelength is,the smaller the differences between the refractive indices caused by different reflectivities are,while the same occurs in the wavelengths over 25 μm.

Fig.5 Real part of refractive index vs.reflectivity in high frequency range

Fig.6 Imaginary part of refractive index vs.reflectivity in high frequency range
4.3 Influence of Micro Structure of EG
Fig.9 shows SEM image of EG with layer thickness less than 0.1 μm. According to the volume change of EG before and after pelleting,we have
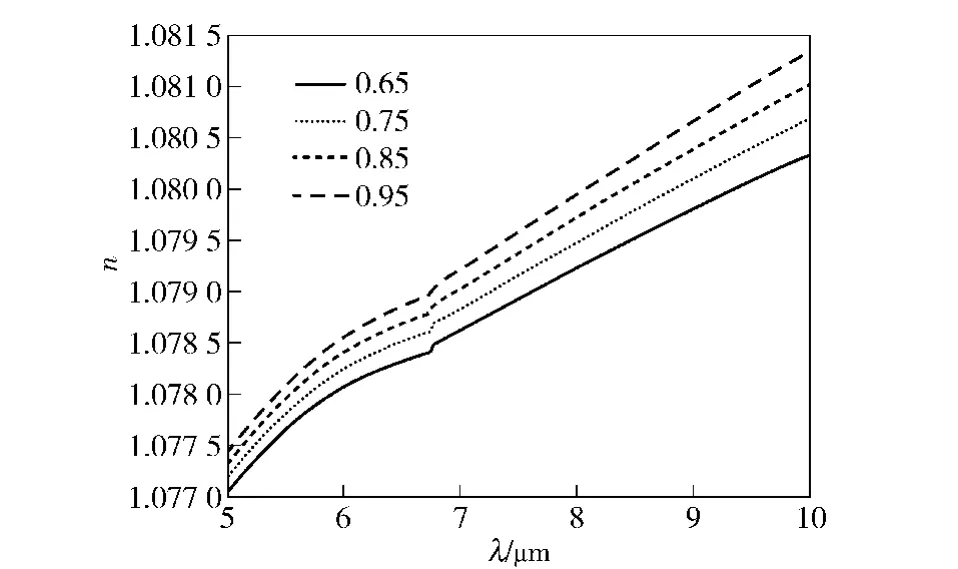
Fig.7 Real part of refractive index vs.reflectivity in low frequency range
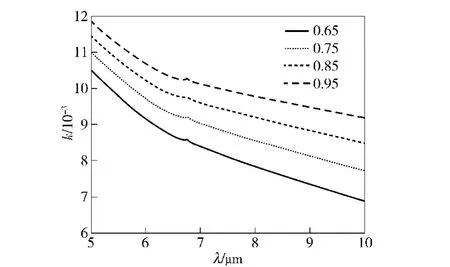
Fig.8 Imaginary part of refractive index vs.reflectivity in low frequency range
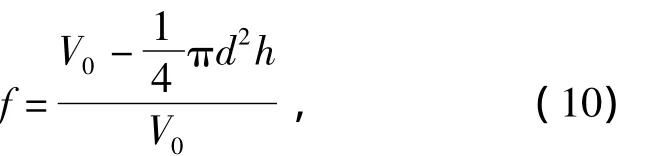
whereV0is the volume of EG before pelleting,handdare the thickness and diameter of the EG pellet.fis calculated to be about 0.95.

Fig.9 SEM image of EG (×50 000)
According to Kolokolova’s experiments[9],if the thickness of EG layer is less than the wavelength of an incident light,the calculated refractive index of powder can almost agree with the experiment results.
5 Conclusions
It is feasible to calculate the refractive index of EG from the reflection spectra of EG pellets based on EMA theory and K-K relation.The calculated refractive indices in IR band of 5 - 10 μm are valuable for the analysis of extinction property of EG.
The incident angles and different reflectivity extrapolations have little influence on the refractive index of EG.Up to now,there is no any public report about the refractive index of EG,thus,the results in this paper need to be proved experimentally.However,based on the calculated refractive index of EG by the presented method,combined with T-matrix theory,the extinction effects of different intercalated EG can be analyzed quantitatively,and it can offer theoretical basis for a smoke extinction experiment.
[1]CHEN Zuo-ru,WAN Chun,LI Yong-fu.Study on infrared attenuation characteristic of expanded graphite worm[J].Journal of Materials Science& Engineering,2005,23(1):42-44.(in Chinese)
[2]REN Hui,JIAO Qing-jie,CUI Qing-zhong.A research on the property of expanded graphite interfering 8 mm wave[J].Acta Armamentarii,2006,27(6):994 -997.(in Chinese)
[3]YUE Cheng-feng,YANG Guan-ling,HE Zhen-jiang,et al.A measurement method of particle’s refractive index by dynamic-static light scattering[J].Acta Photonica Sinica,2008,37(10):2009-2013.(in Chinese)
[4]Taubenblatt M A,Batchelde J S.Measurement of the size and refractive index of a small particle using the complex forward-scattering electromagnetic field[J].Applied Optics,1991,30(33):4972-4979.
[5]RUAN Li-ming,YU Qi-zheng,TAN He-ping.A transmission method for determination of optical constants of small particles in polydispersion[J].Journal of Infrared and Millimeter Waves,1996,15(1):43 - 49.(in Chinese)
[6]QI Hong,WANG Xi-ying,LIU Bing,et al.Determination of the optical constants of non-spherical particles[J].Journal of Engineering Thermophysics,2009,30(5):837-840.(in Chinese)
[7]Lucarini V,Saarinen J J,Peiponen K E,et al.Kramers-Kronig relations in optical materials research[M].Germany:Springer-Verlag Berlin Heidelberg,2005:19 -25.
[8]Bohren C F,Huffman D R.Absorption and scattering of light by small particles[M].New York:John Wiley &Sons,1983:33 -362.
[9]Ludmilla K,Gustafson Bo Å S.Scattering by inhomogeneous particles:microwave analog experiments and comparison to effective medium theories[J].Journal of Quantitative Spectroscopy& Radiative Transfer,2001,70(4-6):611-625.
[10]Rosenheimer M S,Linker R.Impact of the non-measured infrared spectral range of the imaginary refractive index on the derivation of the real refractive index using the Kramers-Kronig transform[J].Journal of Quantitative Spectroscopy & Radiative Transfer,2009,110(13):1147-1161.
杂志排行
Defence Technology的其它文章
- An Estimation Method for Kill Probability Based on Random Residence Time Series
- Numerical Simulation on New Perforator
- Structure and Dynamic Characters of New Radar Stabilized Platform
- An Effective Method of Threshold Selection for Small Object Image
- Modeling and Simulation of Aerial Dispersion on Piston Dispersal Mechanism
- Test and Analysis for Spraying Ammonia in Diesel Engine
