PROGRESS IN STUDIES ON ICE ACCUMULATION IN RIVER BENDS*
2011-05-08WANGJunCHENPangpang
WANG Jun, CHEN Pang-pang
School of Civil Engineering, Hefei University of Technology, Heifei 230029, China, E-mail: wangjunhfut@126.com
SUI Jue-yi
Environmental Engineering Program, University of Northern British Columbia Prince George, British Columbia, Canada, V2N 4V9
PROGRESS IN STUDIES ON ICE ACCUMULATION IN RIVER BENDS*
WANG Jun, CHEN Pang-pang
School of Civil Engineering, Hefei University of Technology, Heifei 230029, China, E-mail: wangjunhfut@126.com
SUI Jue-yi
Environmental Engineering Program, University of Northern British Columbia Prince George, British Columbia, Canada, V2N 4V9
(Received February 23, 2011, Revised September 16, 2011)
River ice is an important hydraulic element in temperate and polar environments and would affect hydrodynamic conditions of rivers through changes both in the boundary conditions and the thermal regime. The river bend has been reported as the common location for the initiation of ice jams because the water flow along a river bend is markedly affected by the channel curvature. In this article, the experimental studies about the ice accumulation in a river bend are reviewed. Based on experiments conducted so far, the criteria for the formation of ice jams in the river bend, the mechanisms of the ice accumulation in the river bend and the thickness profile of the ice accumulation in the river bend are discussed. The k−ε two-equation turbulence model is used to simulate the ice accumulation under an ice cover along a river bend. A formula is proposed for describing the deformation of the ice jam bottom. Our results indicate that all simulated thickness of the ice accumulation agrees reasonably well with the measured thickness of the ice accumulation in the laboratory.
experiment, ice jam/accumulation, bend channel, thickness profile
Introduction
Under an open flow condition, the flow in a river bend is under the influence of the centrifugal acceleration and the gravity, which makes the flow threedimensional with a spiraling motion in the flow field and a super-elevation on the water surface. The transverse circulation on the surface is directed toward the concave bank, and that on the bottom is directed toward the convex bank. The spiral motion of the fluid along a bend due to the longitudinal flow and the transverse circulations has a strong influence on the overall flow behavior and generally promotes mixing of mass.
In winter or early spring, an ice cover would affect the hydrodynamic conditions through changes both in boundary conditions of the rivers and in the thermal regime. Due to the increase of the flow resistance caused by the extra solid boundary, the ice cover raises the water level and reduces the flow velocity as compared with the open flow conditions[1]. Under the ice cover conditions, the flow in the upper portion of a cross-section is mainly affected by the ice cover, and the flow in the lower parts of a cross section is mainly influenced by the riverbed[2,3].
The river bend is known as one of the most common locations for the initiation of ice jams because the water flow along a river bend is markedly affected by the channel curvature[4-6]. The transverse current in a river bend is often related with the lodgment or the gorging process of ice floes. As a consequence, the ice jam or the ice dam often initiates in a river bend. The formation of an ice jam in a river involves a significant change of hydraulic parameters and the sediment transport and riverbed deformation will be dramatically affected[7,8]. Due to the channel curvature and the ice accumulation along the river bend, the flow structure under the ice cover or the ice jam is very complicated. In view of the difficulties and risks in carrying out field observations of the ice accumulationalong a river bend, laboratory studies are essential and they offer a common alternative for studying the mechanisms of the ice accumulation along a river bend.
The past 40 years saw many experimental studies in that respect. The first experimental study regarding the formation of the ice jam in a river bend was carried out by Burgi and Krogstad[9]. Since 1980’s, the ice accumulation along river bends has attracted much research attention[4,6,10,11]. Based on the experimental study in a bend flume, Sun et al.[6]pointed out that, under ice covered conditions, there is a double transverse circulation of flow (1 helical cell) along the river bend. This finding was confirmed by Ettema and Zabilansky[12]. Based on an experimental study in a curved flume using polyethylene floes and beads, Urroz and Ettema[13,14]studied the mechanisms of the ice jam formation in the bend flume. They found that the “lodgment” and the “gorging” are two principal processes of the ice jam initiation in a bend channel. They pointed out that the “lodgment” process occurred for relatively large ice fragments as compared to the channel width while the “gorging” process took place when ice floes were relatively small. It was suggested that the maximum ice concentration on the water surface before jamming increases linearly with the Froude number when the “gorging” process is dominant.
Based on experiments carried out in a bend flume at Hefei University of Technology, China, Sui et al.[5]and Wang et al.[15-17]studied the thickness profile of the ice accumulation along a river bend. Their experimental results were compared with those of Urroz and Ettema[13,14].
1. Mechanisms of ice accumulation in a river bend
1.1 Factors influencing the formation of ice jam
The formation of ice jams in a river bend is related with many factors. Using a straight flume, Calkins and Ashton[18]carried out experiments to see the impacts of dimensions of the ice floe on the formation of ice jam. It was found that the longer the ice floe, the easier the ice jam occurs. Tatinclaux and Lee[19]pointed out that the critical ice concentration on a water surface is a suitable variable to determine if an ice jam would occur in a channel and it is a function of the flow Froude number (Fr) and the dimension of the ice floe (l/B, where, l is the length of ice floe, and B is the width of the channel). Based on the experimental study, Johnson and Kotras[20]studied the ice jam formed in a river bend. It was suggested that the formation of the ice jam in a bend channel is related with the ice concentration on the water surface, the dimension of the ice floe, the channel width and the geometry of the channel.
The most important studies regarding the forma-
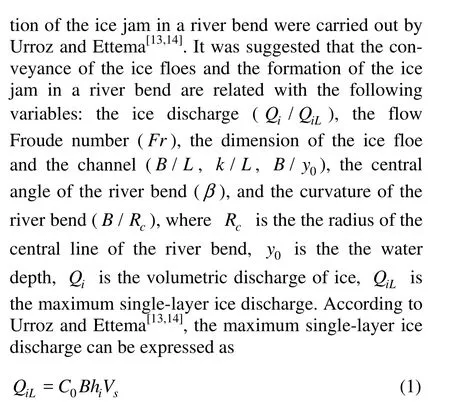
where C0is the the maximum ice discharge on the water surface, hiis the the thickness of the ice floe and Vsis the the approaching flow velocity of the ice floe.
In their experimental study, Urroz and Ettema[13,14]focused on the impacts of Qi/QiL, Fr, B /L and k/L on the formation of the ice jam in a river
Wbeanndg.[15]
proposed the following general function for describing the relationship between different variables during the formation of the ice jam in a river bend
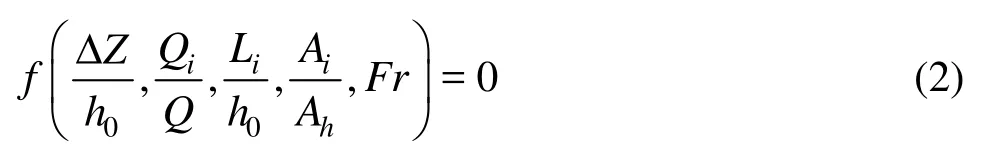
where ΔZ is the the increment of the water level at the trailing edge of the ice cover, h0is the the water depth of the open flow with the same discharge, Q is the flow discharge, Liis the longitudinal length of the ice jam,iA is the the cross section area of the ice jam, Ahis the the flow cross section area under the ice jam.
1.2 Formation of ice jam via mechanical process
Urroz and Ettema[14]carried out small-scale experiments to investigate the initiation process of ice jams in an S-curved flume with 13 bends. The rectangular cross section of the bend flume has a width of 0.254 m. A tailgate is used to maintain a water depth of 0.09 m in the flume. Polyethylene with mass density of 920 kg/m3is used for the ice floe model. In their experiments, 4 different ice floes with dimensions of B/D=3.5, 7.3, 20 and 53 (where D is the floe diameter) were used.
It was suggested that the “lodgement” process and the “gorging” process are two principal mechani-
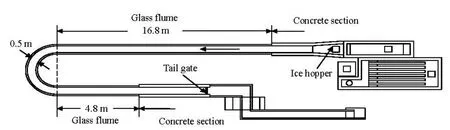
Fig.1 Experiment setup at Hefei University of Technology
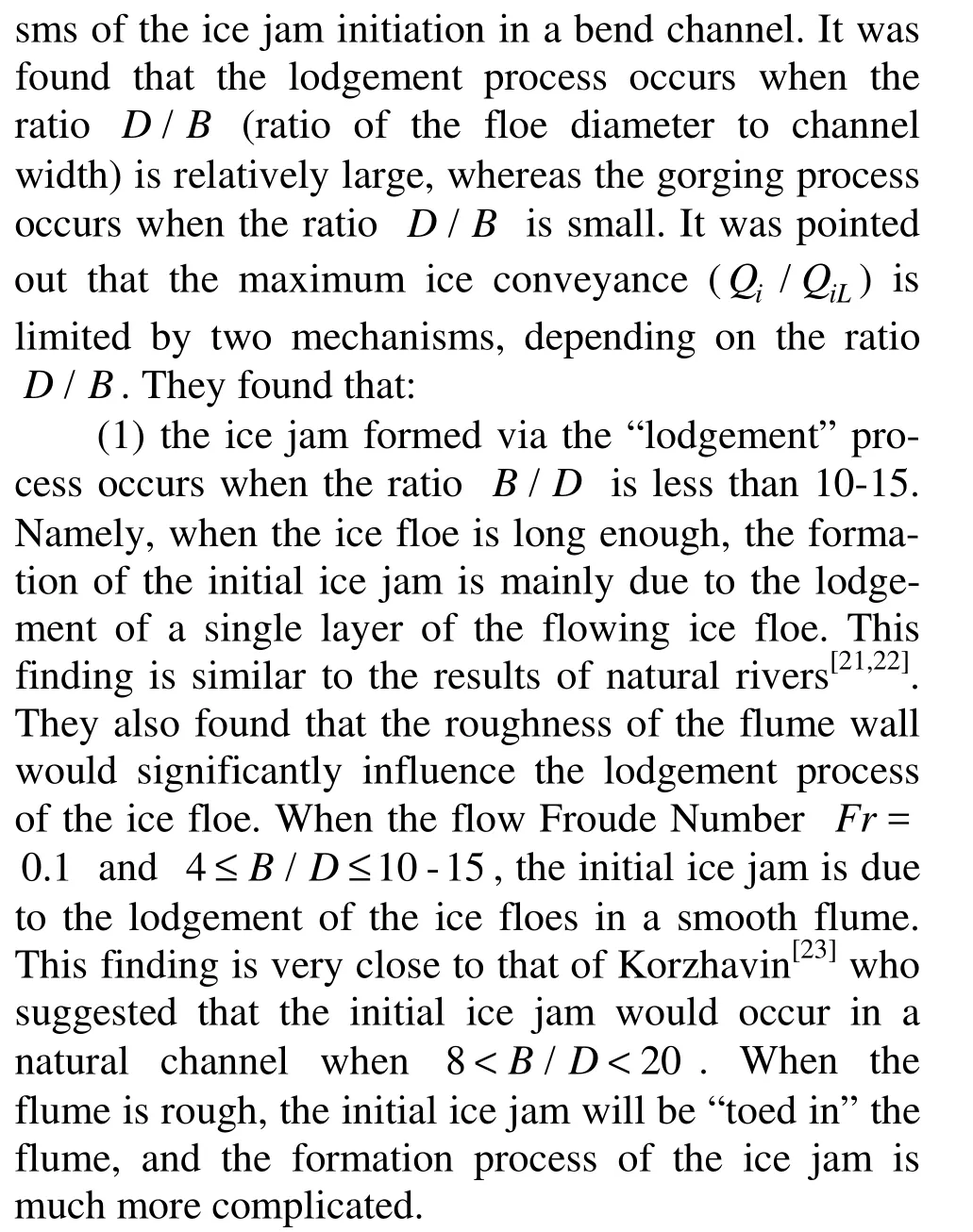
(2) when the ratio B/D is large, namely the diameter of the ice floe is small, the initial ice jam would be formed via the “gorging” process. The formation of the ice jam via the “gorging” process often occurs when the velocity of the flowing ice is small, and the ice concentration on the water surface is high This finding is similar to the results found in natural rivers[24,25]. It was also suggested that the maximum ice floe concentration before jamming increases linearly with the Froude number when the gorging process is dominant. Because of the channel curvature and the ice accumulation along the river bend, the flow structure under an ice cover/jam is very complicated.
As pointed out by Urroz and Ettema[14], the initial ice jam formed by the “lodgement” process is relatively more stable than that formed by the “gorging”process. The thickness of the initial ice jam formed by the “lodgement” process is smaller than that formed by the “gorging” process. In their experiments, the flow Froude number for the “lodgement” process is about 0.26, much higher than that for the “gorging”process.
1.3 Stability of ice jam
Urroz and Ettema[14]did not discuss any obvious relationship between the capacity of the ice conveyance and the flow Froude number in the “lodgement”process. Their focus was on the ice jam formed by the“gorging” process.
In the past, the critical flow Froude number in front of the trailing edge of the ice cover was used to determine whether or not an ice jam can be formed in a river. Kivisild[26]suggested that the critical flow Froude number for the submergence of the ice floe at the trailing edge of the ice cover is 0.08. Ashton[27]pointed out that the critical flow Froude number for the submergence of the ice floe at the trailing edge of the ice cover is between 0.1 and 0.12. It was found that the maximum critical flow Froude Number for the submergence of the ice floe at the trailing edge of the ice cover is 0.17. Based on field observations of the ice jams at the Hequ Reach of the Yellow River, China, Sui et al.[28,29]found that the critical flow Froude number for the submergence of the ice floe at the trailing edge of ice cover is 0.09.

2. Accumulation process of ice jam

jam, since the increased hydraulic slope leads to an increase of the flow velocity. When the flow Froude number is in the range 0.035 < Fr< 0.06, the ice accumulation could be considered as the transitional process. Interestingly, when 0.035 < Fr< 0.06, the bottom of the ice jam has a wavy shape, similar to the sand dune on a riverbed.
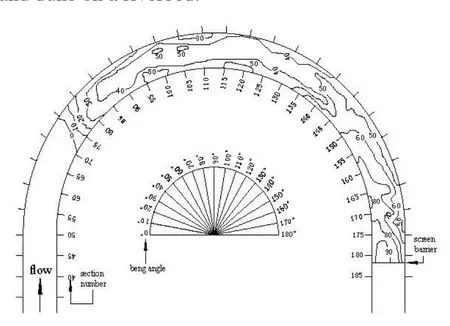
Fig.2(a) The thickness profile of ice accumulation along a bend channel[13]( =0.08 Fr , unit: mm)
3. Thickness of ice jam along the bend channel
3.1 Experimental studies
Under the condition of the fixed channel bed, Urroz and Ettema[13]considered only one case in their experiments in a 180° U-shape bend flume for the thickness profile of the ice jam in the bend channel. It is found that the ice jam along the bend channel is thicker in a downstream section than in an upstream section of the bend channel. Along the convex bank, the ice jam is thicker than along the concave bank of the bend channel, as shown in Fig.2(a). It is also found that an open segment appears in the section between 140° and 155°. The head of the ice jam is located at the cross section of 60o. Along the bend section between 60oand 80o, there exists a transitional section of ice accumulation. Along the bend section between 80oand 134o, the thickness profile of the ice jam changes little both in the longitudinal direction and the transverse direction. It is suggested that the ice jam along the section between 80oand 134ois the equilibrium ice jam, similar to the concept of the equilibrium ice jam proposed by Ashton[30]. Downstream of the cross section 120o, the ice jam is clearly thinner than in the upstream section. The topography of the ice jam along the bend is somehow similar to the scouring topography of a bend fluvial channel. Urroz and Ettema[13]also suggested that the thickness of the ice accumulation in the bend channel increases with the flow velocity.

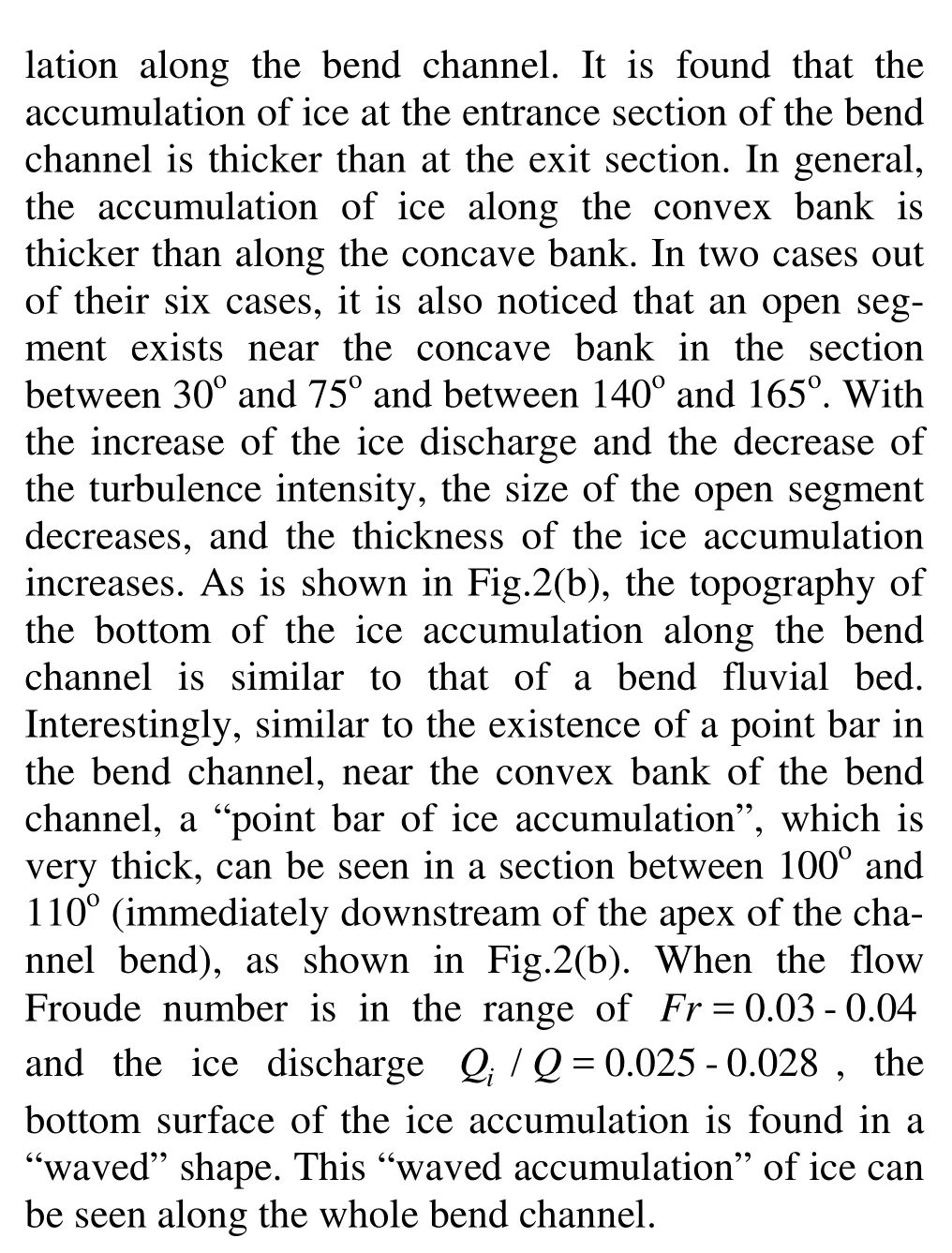
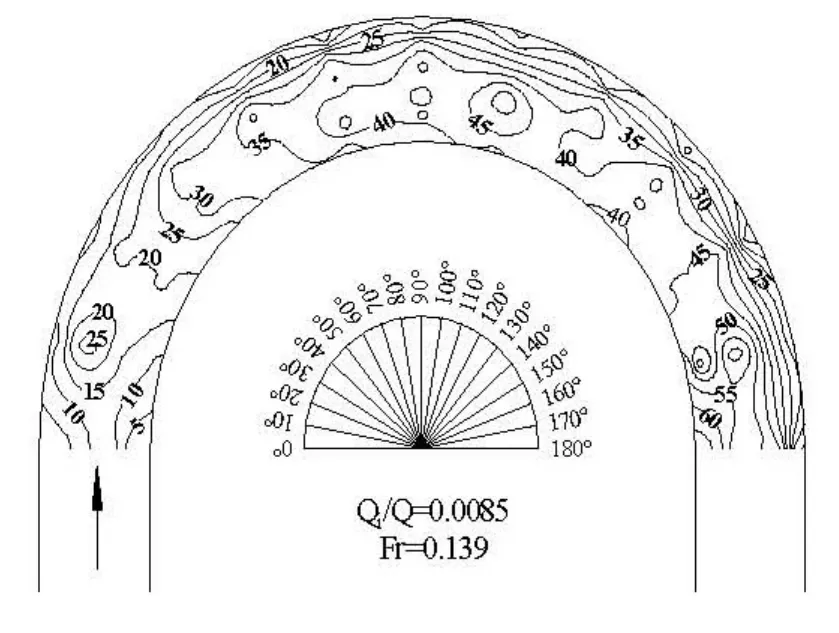
Fig.2(b) The thickness profile of ice accumulation along a bend channel[17]( =0.139 Fr , Unit: mm)
In the second experiment setup, Wang et al.[16,17]considered seven cases to study the thickness profile of the ice accumulation along the bend channel. Results indicate that the ice accumulation starts from upstream of the bend channel and progresses to downstream along the bend channel, when the flow Frooude num
ober Fr< 0.035. Along the section from 60 and 75, an open segment near the concave bank can be observed. Along theosection from the entrance of the bend channel to 45 cross section, the ice accumulation near the concave bank is thicker than near the convex bank. Alongothe section from the apex of the bend channel to 160 cross section, the ice accumulation near the concave bank isothicker than near the convex bank. Between the 160 cross section and the

In practice, a meandering channel (or S-shaped river bends) appears very often in the flat region. Due to its low hydraulic gradient and the transverse flow in the river bend, ice jams are often formed in the river bend, and open segments appear between river bends. Up to date, there are only experiments carried out by Urroz and Ettema[14]and Wang et al.[16,17]to study the formation of ice jams in the S-shaped bend flumes. Although Urroz and Ettema[14]studied the formation of ice jams in an S-shaped bend flume, they did not obtain the thickness profile of the ice accumulation along an S-shaped bend flume.
By using an S-shaped bend flume, Wang et al.[17]studied the ice accumulation process in this S-shaped bend flume. Their S-shaped bend flume consists of three 180°-bend sections. Their experiments were conducted under two different setups with different initial experimental conditions as mentioned above. In the first experiment setup, before discharging model ice cubes into the flume, the flowing water in the bend flume is covered by a model ice cover. Then, experiments will start. In their second experiment setup, one piece of the model ice cover is installed at the downstream of the S-shaped bend flume to form the initial ice cover at the downstream cross section of the S-shape bend channel. With each of these two experimental setups, three cases are considered. It is found that the ice accumulation is generally thicker near the convex bank than near the concave bank. It is also observed that when the flow Froude number is relatively small, namely, Fr <0.065, the thickest ice accumulation is closer to the concave bank rather than to the convex bank. With the increase in the flow Froude number (the maximum flow Froude number in their experiments is 0.15), the difference between the thickest and thinnest accumulation of ice in the bend channel diminishes.
3.2 Numerical simulation
To our knowledge, numerical simulations regarding the accumulation process of the frazil ice along a bend channel are few. To simulate the floating rates of the frazil ice particles in water under a covered condition, based on the theory of two-phase flow and laboratory data, Wang et al.[31]developed a 3-D model. The Lagrangian trajectory method is used in the 3-D simulation of the floating rates of frazil ice particles along a 180obend channel. Their results can be summarized as follows: Along the bend section between the entrance section (0o) and the 60ocross section, the maximum longitudinal flow velocity zone is close to the convex bank. Afterward, the maximum velocity zone is gradually shifted to the concave bank. At the outlet cross section (180o) of the bend, the maximum flow velocity is distributed along the concave bank. Along the bend section between the entrance section (0o) and the 60ocross section, the maximum residual pressure zone is close to the convex bank. Afterward, the maximum residual pressure zone is gradually shifted to the concave bank. At the outlet cross section (180o) of the bend, the maximum residual pressure is distributed along the concave bank. Additionally, for the bend section between the entrance section (0o) and the 60ocross section, the gradient of the residual pressure is larger along the convex bank than along the concave bank. For the bend section between 60ocross section and the outlet cross section (180o), however, the gradient of the residual pressure is larger along the concave bank than along the convex bank. With the same flow Froude number, the velocity profiles along the bend channel are similar to those in the prototype bend channels. The patterns of the secondary transverse circulations of flow in the model are similar to those in the prototype bend channels. However, due to the difference in the Reynolds number, along the sections from 90ocross section to 150ocross section, the variations of the velocity profiles, the turbulence intensity and the residual pressure are relatively great, with little similarities between the model bend channel and the prototype bend channels. The floating rate of the ice particles along the convex bank is also clearly higher than along the concave bank. In overall, both the calculated floating rate of the ice particles and the accumulated thickness of ice at each cross section are clearly higher along the convex bank than along the concave bank. The profiles of the floating rates for flow in the model channel are similar to those in the prototype bend channels. The simulated floating rates of ice particles are clearly higher along the convex bank than along the concave bank at each cross section.
Further more, Wang et al.[32]used the k−ε two-equation turbulence model to simulate the ice accumulation under an ice cover along a river bend. The 2-D depth-averaged numerical model is developed in a nonorthogonal coordinate system with nonstaggered curvilinear grids of 9 116 grid nodes. Thefinite volume method is used for numerical solutions. The irregular boundary is transformed using the elliptic differential equations.
In their model, the contravariant velocity is treated as an independent variable. To avoid the pressure oscillation in the non-staggered grids, the momentum interpolation method is used to interpolate the variables at the interface. The discretized equations are solved by using pressure correction algorithms. Formulas are proposed to describe the deformation of the ice jam bottom.
To assess their developed numerical model for the ice accumulation along the bend channel, experiments were carried out in eight cases to examine the effects of the flow rate, the water depth and the ice discharge on the thickness distribution of the ice accumulation along the bend flume at Hefei University of Technology. The thickness distribution of the ice accumulation along this bend is measured.
Using their developed numerical model, the elevations of the ice jam bottom along the bend flume are simulated in experiments. Results indicate that all simulated results agree reasonably well with the experimental results. It appears that the developed numerical model can be used successfully to simulate the thickness distribution of the ice accumulation along a river bend.
4. Conclusions

The process of the ice accumulation along a bend channel is very complicated due to the spiral flow. In a natural channel, since the channel bed is also movable, the ice accumulation under the ice jammed condition will be affected[7,8]. The interaction between the ice accumulations and the river bed deformation even in a straight channel is not well studied. So far, neither experimental studies nor numerical simulations have been reported regarding the interaction between the ice accumulations and the river bed deformation along a bend channel. Future research should be carried out for the impacts of the ice accumulation on the riverbed deformation along a bend channel.
Acknowledgement
This work was supported by the Scientific Research and Development fund of Hefei University of Technology (Grant No. 2010HGXJ0064).
[1] SUI J. Y., THRING R. and KARNEY B. W. et al. Effects of river ice on stage-discharge relationships–A case study of the Yellow River[J]. International Journal of Sediment Research, 2007, 22(4): 263-272.
[2] SUI J. Y., WANG J. and HE Y. et al. Velocity profiles and incipient motion of frazil particles under ice cover[J]. International Journal of Sediment Research, 2010, 25(1): 39-51.
[3] WANG Jun, SUI Jue-yi and KARNEY Bryan W. Incipient motion of non-cohesive sediment under ice cover–An experimental study[J]. Journal of Hydrodynamics, 2008, 20(1): 117-124.
[4] ZUFELT J. E., SUN Z. A laboratory study of transverse velocities and ice jaming in a river bend[C]. Proceedings of the IAHR Symposium on Ice Problems. Sapporo, Japan, 1988, 3: 189-198.
[5] SUI J. Y., WANG J. and BALACHANDAR R. et al. Accumulation of frazil ice along a river bend[J]. Canadian Journal of Civil Engineering, 2008, 35(2): 158-169.
[6] TSAI W. F., ETTEMA R. Ice cover influence on transverse bed slopes in a curved alluvical channel[J]. Journal of Hydraulic Research, 1994, 32(4): 561-581.
[7] SUI J. Y., WANG D. and KARNEY B. W. Sediment concentration and deformation of riverbed in a frazil jammed river reach[J]. Canadian Journal of Civil Engineering, 2000, 27(6): 1120-1129.
[8] SUI J. Y., HICKS F. and MENOUNOS B. Observations of riverbed scour under a developing hanging ice dam[J]. Canadian Journal of Civil Engineering, 2006, 33(2): 214 -218.
[9] BURGI P. H., KROGSTAD D. E. Ice management at dickinson dam spillway crest gate[C]. IAHR Symposium on Ice 1986–Proceedings. Iowa City, IA, USA, 1986, 2: 235-247.
[10] BELTAOS S., DEAN A. M. Field investigations of a hanging ice dam[C]. Proceedings of the 6th IAHR International Ice Symposium. Quebec, Canada, 1981, 2: 475-485.
[11] GARBRECHT J. Determination of the execution sequence of channel flow for cascade routing in a drainage network[J]. Hydrosoft, 1984, 1(3): 129-138.
[12] ETTEMA R., ZABILANSKY L. Ice influences on channel stability: Insights from Missouri’s fort peck reach[J]. Journal of Hydraulic Engineering, 2003, 130(4): 279-292.
[13] URROZ G. E., ETTEMA R. Bend ice jams: Laboratory observations[J]. Canadian Journal of Civil Engineering, 1992, 19(5): 855-864.
[14] URROZ G. E., ETTEMA R. Small-scale experiments on ice jam initiation in a curved channel[J]. Canadian Journal of Civil Engineering, 1994, 21(5): 719-727.
[15] WANG Jun. Research on mechanism of ice jam evolution and simulation of velocity and ice particle solidliquid two-phase flow under ice cover[D]. Ph. D. Thesis, Hefei: Hefei University of Technology, 2007(in Chinese).
[16] WANG Jun, FU Hui and YI Ming-kun. Analysis of stages under ice-covered in winter[J]. Advances in Water Science, 2007, 18(1): 102-107(in Chinese).
[17] WANG Jun, GAO Yue-xia and YI Yun-ji. An experimental study of ice jam formation and its thickness distribution in a curved channel[J]. Journal of Glaciology and Geocryology, 2007, 29(5): 764-769(in Chinese).
[18] CALKINS D. J., ASHTON G. D. Arching of fragmented ice cover[J]. Canadian Journal of Civil Engineering, 1975, 2(4): 392-399.
[19] TATINCLAUX J. C., LEE C. L. Initiation of ice jams-a laboratory study[J]. Canadian Journal of Civil Engineering, 1978, 5(2): 202-212.
[20] JOHNSON R. P., KOTRAS T. V. Physical hydraulic model study of an ice-covered river[C]. Proceedings of the ASCE Hydraulics Division Conference. Chicago, USA, 1980, 3: 431-440.
[21] YANG Kai-lin, LIU Zhi-ping and LI Gui-fen et al. Numerical simulation of river ice jams[J]. Water Resources and Hydropower Engineering, 2002, 33(10): 40-47(in Chinese).
[22] BOEHRER B., TIBKE M. and SUHR U. Frontal progression of a juxtaposed ice cover on the Elbe river[J]. Journal of Hydrology, 2004, 288(10): 258-263.
[23] KORZHAVIN K. N. Conditions of ice passage through bridge openings free of jams on Siberian rivers[C]. Proceeding of the IAHR Symposium on Rivers and Ice. Budapest, Hungary, 1974, 4: 57-65.
[24] CAI Lin. River ice jams in China[M]. Zhengzhou: The Yellow River Conservancy Publication, 2008(in Chinese).
[25] BELTAOS S. Progress in the study and management of river ice jams[J]. Cold Regions Science and Technology, 2008, 51(1): 2-19.
[26] KIVISILD H. R. Hanging ice dams[C]. Proceedings of the 8th Congress of the International Association for Hydraulic Research. Montreal, Canada, 1959, 3: 1-30.
[27] ASHTON G. D. River and lake ice engineering[M]. Littleton, Colorado, USA: Water Resources Publications, 1986.
[28] SUI J. Y., KARNEY B. W. and SUN Z. et al. Field investigation of frazil jam evolution–A case study[J]. Journal of Hydraulic Engineering, 2002, 128(8): 781-787.
[29] SUI J. Y., KARNEY B. W. and FANG D. Variation in water level under ice-jammed condition–Field investigation and experimental study[J]. Nordic Hydrology, 2005, 36(1): 65-84.
[30] HEALY D., HICKS F. E. Experimental study of ice jam formation dynamics[J]. Journal of Cold Regions Engineering, 2006, 20(4): 117-139.
[31] WANG Jun, Li Qing-gang and SUI Jue-yi. Floating rate of frazil ice particles in flowing water in bend channels–A three-dimensional numerical analysis[J]. Journal of Hydrodynamics, 2010, 22(1): 19-28.
[32] WANG J., SUI J. Y. and CHEN P. Numerical simulations of ice accumulation under ice cover along a river bend[J]. International Journal of Environmental Science and Technology, 2009, 6(1): 1-12.
[33] HEALY D., HICKS F. E. Experimental study of ice jam thickening under dynamic flow conditions[J]. Journal of Cold Regions Engineering, 2007, 21(3): 72-91.
10.1016/S1001-6058(10)60171-0
* Project supported by the National Natural Science Foundation of China (Grant No. 50979021).
Biography: WANG Jun (1962-), Male, Ph. D., Professor
SUI Jue-yi, E-mail: sui@unbc.ca
杂志排行
水动力学研究与进展 B辑的其它文章
- LATTICE BOLTZMANN METHOD SIMULATIONS FOR MULTIPHASE FLUIDS WITH REDICH-KWONG EQUATION OF STATE*
- DYNAMIC ANALYSIS OF FLUID–STRUCTURE INTERACTION OF ENDOLYMPH AND CUPULA IN THE LATERAL SEMICIRCULAR CANAL OF INNER EAR*
- SIMULATIONS OF FLOW INDUCED CORROSION IN API DRILLPIPE CONNECTOR*
- NUMERICAL SIMULATION OF FLOW OVER TWO SIDE-BY-SIDE CIRCULAR CYLINDERS*
- NUMERICAL STUDY OF HYDRODYNAMICS OF MULTIPLE TANDEM JETS IN CROSS FLOW*
- EXPERIMENTAL STUDY ON SEDIMENT RESUSPENSION IN TAIHU LAKE UNDER DIFFERENT HYDRODYNAMIC DISTURBANCES*
