在等距节点处的反周期函数的一类三角插值问题

李艳坡,何尚琴,高明晶,郑国萍,郭亚军

(河北科技师范学院数学与信息科技学院,河北 秦皇岛 066004)

在等距节点处的反周期函数的一类三角插值问题

李艳坡,何尚琴,高明晶,郑国萍,郭亚军

(河北科技师范学院数学与信息科技学院,河北 秦皇岛 066004)

研究了以π为周期的反周期函数的一类缺项三角插值,解决了在等距节点处的反周期函数的(0,P(D))三角插值问题,得到了解存在的条件、插值函数的显式表达式及其收敛阶.

反周期函数;插值;正则;收敛阶

1 几个引理

2 主要结果

3 主要结果的证明

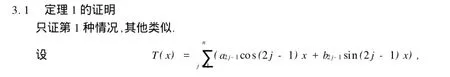
4 例题

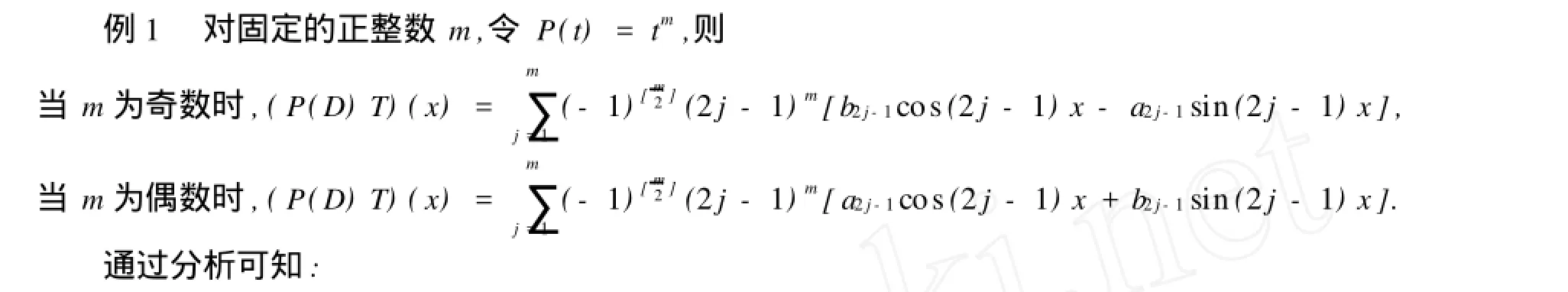
当m为奇数时,Po(i(2 j-1))+Po(i(2n-2 j+1))=(2 j-1)m+(2n-2 j+1)m≠0.

当m为偶数时,Pe(i(2 j-1))-Pe(i(2n-2 j+1))=(2 j-1)m-(2n-2 j+1)m≠0.由此可见,文献[7]的结论是本文的特殊情况.

例2 令P(t)=t+t3,即P(t)是奇多项式,则当

Po(i(2j-1))+Po(i(2n-2 j+1))=(2 j-1)-(2 j-1)3+(2n-2 j+1)-(2n-2 j+1)3≠0时,定理1-3总成立.

令P(t)=t2+t4,即P(t)是偶多项式,则当

Pe(i(2 j-1))-Pe(i(2n-2 j+1))=-(2j-1)2+(2 j-1)4-(-(2n-2j+1)2+(2n-2j+1)4)≠0时,定理1-3总成立.

[1]LOREN TZ G G.Birkhoff Interpo lation[M].London:A ddison-W esley Publishing Com pany,1983.

[2]SHARMA A,XU Y.Mean convergence of trigonometric interpolationson equidistant nodes:Birkhoff dada[J].Bull Polish Acard Sci,1991,39(3):199-206.

[3]SHARMA A,SZABADOSJ,VARGA R S.Some 2-periodic trigonometric interpolationsof equidistant nodes[J].Analysis,1991,11:165-190.

[4]L IU Yongping.The app roximation properties of certain trigonometric interpolation polynomia operato res[J].No rthestern Math,1988,4(3):289-308.

[5]DELVOS F J,KNOCHE L.Lacunary interpolation by antiperiodic trigonometric polynomials[J].B IT,1999,39(3):430-450.

[6]韩惠丽.Hermite半三角插值公式及其应用[D].武汉:武汉大学数学与统计学院,2004.

[7]何尚琴,侯象乾.反周期函数(0,m)三角插值的收敛性[J].宁夏大学学报:自然科学版,2006,27(3):218-224.

[8]谢庭藩,周颂平.实函数逼近论[M].杭州:杭州大学出版社,1998.

(责任编辑:王兰英)

Problem of a Kind Antiperiodic Trigonometric Interpolation on Equidistant Nodes

LIYan-po,HE Shang-qin,GAOMing-jing,ZHENG Guo-ping,GUO Ya-jun
(Schoo l of M athematics and Info rmation Techno logy,Hebei No rm al University of Science and Techno logy,Qinhuangdao 066004,China)

The Birkhoff trigonometric interpolation ofπ-antiperiodic function was studied,the p roblem of theπ-an tiperiodic function(0,P(D))in terpo lation on equidistant nodes w as solved,the existing conditionsof the so lution was obtained,the exp licit fo rm and the rate of convergence were go t in the corresponding condition.

antiperiodic function;interpolation;regular;the rate of convergence

O 174.41

A

1000-1565(2010)06-0617-05

2009-12-05

河北省自然科学基金数学研究专项(08M 004)

李艳坡(1973-),女,河北昌黎人,河北科技师范学院讲师,主要从事函数论方向研究.