Pore-pressure and stress-coupled creep behavior in deep coal: Insights from real-time NMR analysis

Wenho Ji ,Hongwei Zhou *,Senlin Xie ,Yimeng Wng ,Xinfeng Hu ,Lei Zhng

a School of Mechanics and Civil Engineering,China University of Mining and Technology-Beijing,Beijing 100083,China

b School of Energy and Mining Engineering,China University of Mining and Technology-Beijing,Beijing 100083,China

c State Key Laboratory of Coal Resources and Safe Mining,China University of Mining and Technology-Beijing,Beijing 100083,China

d College of Safety and Emergency Management Engineering,Taiyuan University of Technology,Taiyuan 030024,China

Keywords:Real-time monitoring Pore pressure-stress coupling Microscopic pore-fracture structure Variable-order fractional creep model Deep coal

ABSTRACT Understanding the variations in microscopic pore-fracture structures (MPFS) during coal creep under pore pressure and stress coupling is crucial for coal mining and effective gas treatment.In this manuscript,a triaxial creep test on deep coal at various pore pressures using a test system that combines in-situ mechanical loading with real-time nuclear magnetic resonance (NMR) detection was conducted.Full-scale quantitative characterization,online real-time detection,and visualization of MPFS during coal creep influenced by pore pressure and stress coupling were performed using NMR and NMR imaging(NMRI) techniques.The results revealed that seepage pores and microfractures (SPM) undergo the most significant changes during coal creep,with creep failure gradually expanding from dense primary pore fractures.Pore pressure presence promotes MPFS development primarily by inhibiting SPM compression and encouraging adsorption pores(AP)to evolve into SPM.Coal enters the accelerated creep stage earlier at lower stress levels,resulting in more pronounced creep deformation.The connection between the micro and macro values was established,demonstrating that increased porosity at different pore pressures leads to a negative exponential decay of the viscosity coefficient.The Newton dashpot in the ideal viscoplastic body and the Burgers model was improved using NMR experimental results,and a creep model that considers pore pressure and stress coupling using variable-order fractional operators was developed.The model’s reasonableness was confirmed using creep experimental data.The damagestate adjustment factors ω and β were identified through a parameter sensitivity analysis to characterize the effect of pore pressure and stress coupling on the creep damage characteristics (size and degree of difficulty) of coal.

1.Introduction

Creep is a phenomenon where a material slowly deforms under constant stress[1].Given the complex environment in which deep coal is found and the need for safe pretreatment,deep coal and underground structures will serve the coal resource mining process for an extended period [2].Therefore,understanding coal creep deformation is crucial for long-term stable coal extraction and effective gas treatment [3,4].Research has shown that creep deformation is a macroscopic result of the evolution of microscopic pore-fracture structures(MPFS)[5].Coal,as a porous medium with numerous MPFS[6],undergoes complex and variable pore-fracture evolution during creep,leading to noticeable nonlinear deformation of coal[7].Therefore,examining the microstructural evolution during coal creep provides valuable insights into the macroscopic creep deformation behavior of coal and supports safe mining practices.

To elucidate the mechanical properties of rock creep from a microscopic perspective,numerous scholars have analyzed and characterized creep deformations using various microscopic observations[8].Optical microscopy[9–12]and SEM techniques[13,14]have been employed to describe the type,distribution,development mode,density,and expansion degree of fractures in the rocks during creep deformation,as well as to determine the effects of different conditions on rock creep deformation.However,these methods involve 2D local observations and can only provide a limited view within a confined range [15–17].Moreover,these studies are black-box tests,meaning only the specimens’ initial and final states during loading can be tested,and the evolution trend throughout the loading process remains unknown.Some researchers have detected real-time damage during the entire rock creep process using acoustic emission technology[18–20].However,this technique can only locate the source and amount of damage within a sample using the elastic wave signals released when large fractures occur within the rock;it cannot quantify the size and distribution of MPFS [21,22].Therefore,there is an urgent need for a microscopic characterization method that enables quantitative characterization of the initiation,development,real-time propagation,evolution characteristics,and spatial distribution of pore fractures during rock creep to improve corresponding mechanical analyses.

Nuclear magnetic resonance (NMR) and NMR imaging (NMRI)are rapid,accurate,non-destructive,and comprehensive pore size characterization techniques for macro-scale samples(25 mm × 50 mm) [23,24].NMR can quantitatively characterize the identification,complexity,and distribution of coal MPFS based onT2spectra[25–27].NMRI can qualitatively characterize the spatial distribution of the seepage pores and microfractures (SPM)[28–30].In recent research,numerous researchers have described the MPFS evolution during the creep in shales and sandstones under various loading conditions using NMR techniques combined with other mechanical test machines [1,22,31,32].However,there has been limited work on deep coal creep studies based on NMR and the characterization of MPFS during creep combined with NMRI techniques.The above studies on rock creep behavior based on NMR are all off-line tests,which not only share the limitation of a ‘‘black box test”,but also overlook the influence of recoverable deformation caused by unloading [33].Importantly,these creep experimental studies from a microscopic perspective have rarely considered the effects of deep occurrence environments.

Considering the effects of groundwater and gas pressure in high-gas coal seams,pore pressure significantly impacts the development of MPFS during creep and thus influences the creep deformation mechanism [34].Taking the effect of pore pressure into account,numerous researchers have conducted triaxial creep tests on different rock species at various pore pressures [35].They analyzed the effects of pore pressure on creep strain [36,37],creep strain rate [38],creep failure time [39],elastic modulus [40,41],viscosity coefficient[13],and MPFS development using experimental results,and explored the deformation mechanism of rock creep under the influence of pore pressure.Since these mechanical analyses primarily emphasize the macroscopic perspective,establishing a distinct linkage between the microscopic and macroscopic facets becomes paramount for a more scientifically insightful elucidation of the creep deformation mechanism.

Creep modeling is an essential tool for establishing a connection between laboratory testing and engineering applications[42].Considering the advantages of clear physical meaning and a flexible combination of component models,various creep models that take the influence of pore pressure into account have been developed to predict creep deformation in practical engineering.These models generally fall into two categories: (1) creep models with effective stress principle,where pore pressure affects the change in effective stress,resulting in a change in creep loading stress [34,43];(2)creep models considering the effect of pore pressure,established by assuming that the variation in pore pressure leads to the deterioration of macroscopic mechanical parameters (including longterm strength and viscosity coefficient) during creep [44–46].However,the first model does not analyze the creep deformation mechanism by pore pressure from a microscopic perspective.The second model defines the deterioration index simply by assuming that the parameters obey a certain law or are influenced by parameters such as axial pressure,pore pressure,and confining pressure.Consequently,numerous scholars are engaged in formulating a creep model with straightforward engineering applicability and distinct physical significance.

Firstly,this study utilized a test system that combines in-situ mechanical loading and NMR real-time detection to achieve online,real-time testing and analysis of coal creep deformations under pore pressure and stress coupling.By integrating NMR and NMRI real-time online test systems,a comprehensive,accurate,and transparent characterization of MPFS evolution during coal creep was achieved.The results of the microscopic tests established the relationship between the real-time MPFS evolution and macroscopic deformation during creep.Therefore,a variable-order fractional creep model considering pore pressure and stress coupling was developed by combining the two creep modeling concepts of effective stress and parameter degradation.A comparative analysis of the NMR test results demonstrates that the model accurately describes the entire coal creep process,considering the effect of the pore pressure.This indicates the reliability of using microscopic parameters to construct creep models characterizing macroscopic creep deformations of coal.

2.Creep test procedure

2.1. Test preparation

Deep coal samples were meticulously chosen from the front of working face JI15-31040 of Pingdingshan Mine No.12,at a specific sampling depth of approximately 1000 m.The coal seam had an initial gas content of 17.45 m3/t with a gas pressure of 1.3 MPa.Coal blocks larger than 300 mm3were selected from the same coal seam location and wrapped with preservative film and shockproof foam to maintain the in-situ properties of the coal sample as much as possible.The samples of φ25 mm ×H50 mm were prepared according to the IRSM standards after transportation to the laboratory.The physical properties of the coal are listed in Table 1.The proximate analysis of the coal samples revealed moisture,ash,volatile matter,and fixed carbon contents of 2.71%,11.64%,24.56%,and 61.09%,respectively.Additionally,P-wave velocity and initialT2tests were conducted on the coal to ensure accurate results and minimize the effects of sample dispersion.Coal samples with similar initialT2spectra and wave velocities of 2.740–3.053 km/s were selected for testing.

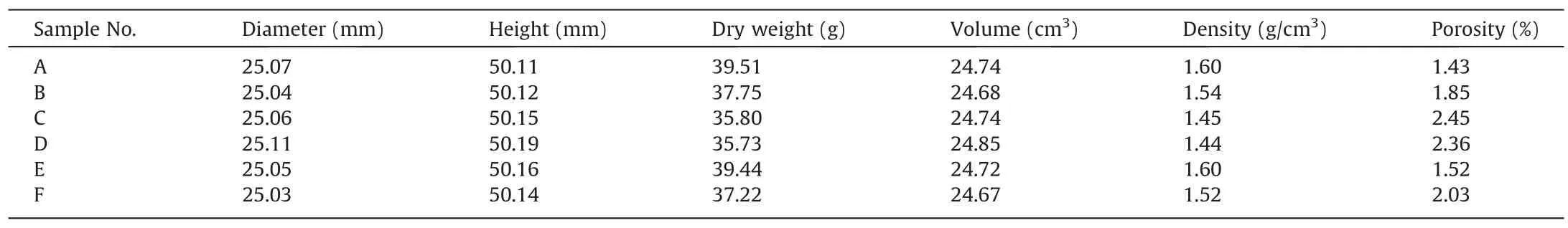
Table 1 Physical parameters of the coal samples.

2.2. Test equipment and process

A test system combining in-situ mechanical loading and NMR real-time detection was employed to achieve real-time detection of MPFS evolution in deep coal during creep and to better capture the mechanical response of coal during loading.The system primarily consists of a data acquisition,pressure loading,and NMR test system.Due to the influence of the NMR device,the basic functions and information of the loading system are as follows: (1)axial pressure loading can be accomplished with a ceramic loading head,with a maximum value of 100 MPa,and water as the loading medium;confining pressure σ3loading can reach up to 40 MPa with FC-40 as the loading medium;and pore pressurePloading can reach up to 20 MPa with water as the loading medium.The specific loading position is shown in Fig.1;(2)the NMR test system uses the MacroMR12-150H instrument from Niumag,which provides a magnetic field in three directions of(0.3±0.05)T for microscopic pore-structure observation of the sample;(3) the data acquisition system enables real-time capture and recording of microscopicT2spectra,sample imaging,and macroscopic displacements.The displacement is mainly collected by an axial displacement meter,and the strain variation of the sample can be calculated from the recorded displacement variation.

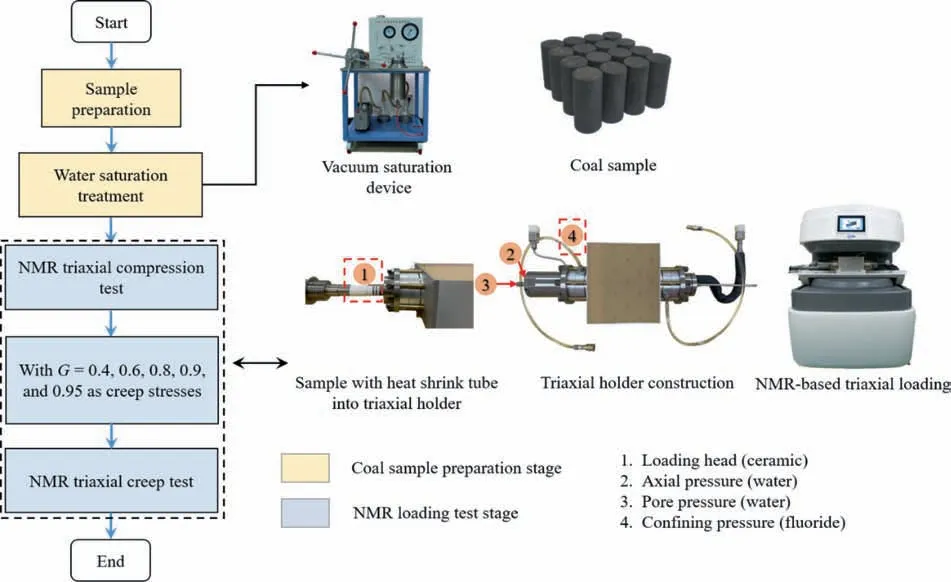
Fig.1.Creep test equipment and process.

Smooth mining of gas-containing coal necessitates long-term pre-extraction of gas,which leads to creep deformation,affecting the safe extraction of coal and effective treatment of gas.Because of the effects of gas extraction and complex deep environments,different pore pressures exist in coal seams at different locations.The coal in front of the working face is in a deviatoric stress state,where axial pressure increases and σ3decreases.Consequently,considering the stress environment of the coal in front of the working face and studying the creep deformation behavior of the coal under different deviatoric stresses,creep tests of the axial pressure for gradient loading at constant confining pressure (10 MPa) were conducted at pore pressures of 2,3,and 4 MPa.

The experiment was conducted with the following NMR parameters: a waiting time of 3000 ms,a cumulative number of 32,an echo time of 0.1 ms,and an echo number of 15000.Fig.1 illustrates the detailed test steps:(1)drying coals at 105°C for 24 h.The samples were subjected to vacuum for 2 h using a ZYB-II vacuum saturation device,followed by saturation with water at 15 MPa for at least 24 h;(2) the sample was wrapped in a heat-shrinkable tube and inserted into a holder.Considering the stress state of the coal in front of the working face after unloading,the axial pressure and confining pressure are simultaneously loaded to 10 MPa at a rate of 2 MPa/min,i.e.,the hydrostatic pressure state.Subsequently,the same water injection pressureP=2 MPa was set at both ends of the sample,and theT2spectrum was measured until it remained essentially unchanged,signifying constant pore pressure during loading.(3) axial pressure was applied (2 MPa/min),whereas thePand σ3remained constant until the sample was destroyed.TheT2spectrum and NMRI were measured at each increase in the axial pressure by 2 MPa.(4) using the triaxial compression strength σcpat the same pore and confining pressures as a reference,the ratio of creep stress σiat gradeito triaxial compression strength σcpwas defined as the loading gradeG,i.e.,G=σi/σcp.Creep experiments were performed withGequal to 0.4,0.6,0.8,0.9,and 0.95,with each grade having a loading time of 10 h.The displacement acquisition interval is 60 s.T2spectrum and NMRI were alternately tested at an interval of 1 h.Considering the discretization of the sample itself and ensuring the ultimate failure of the coal,the loading stress can be appropriately adjusted to the actual situation.(5)Substitute the sample,altering only the pore pressurePto 3 MPa and 4 MPa,respectively,then replicate steps (2)–(4).The specific creep experimental loading scheme is shown in Table 2.

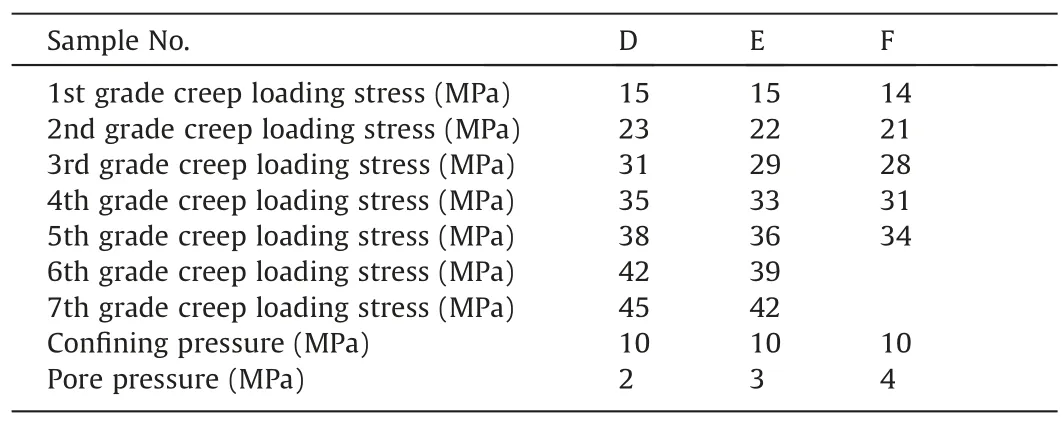
Table 2 Loading scheme of creep experiment.

2.3. Basic principles of NMR

NMR can be used to obtain the relaxation information of1H in water using a radiofrequency (RF) pulse and an external magnetic field [24,47].The coal porosity and the distribution of the pore sizes for the different pores can be determined from theT2spectra of the saturated samples.In conjunction with NMRI,the spatiallocation distribution of different pores can be determined,achieving a comprehensive characterization of coal MPFS [30].The Carr-Purcell-Meiboom-Gill (CPMG) sequence is widely employed to measure theT2and signal intensities of samples[48].TheT2spectrum and pore radius for coal are intuitively described as follows[49]:

whereT2iisT2at positioni;ρ2the transverse relaxation rate;rithe pore radius at positioni;Vthe pore volume;Sthe pore surface area;andCsa geometrically dependent pore parameter.

TheT2spectrum is proportional to the pore size of the coal.A smallerT2value indicated a faster relaxation rate and a smaller corresponding pore size,while a largerT2value indicated a larger pore size.The pore size and total volume of the coals were determined from the positions and magnitudes of theirT2spectral peaks,respectively.Indeed,the NMRT2spectrum represents the distribution of water content across pores of various sizes,while the integral of the curve corresponds to the total volume of the water-bearing pores.Consequently,pore volume can be ascertained from the NMR signal intensity.Subsequently,the porosity of the samples can be determined using the method of calculating porosity based on water saturation.The specific steps are as follows [50]: (1) establish the linear relationship between porosity and NMR signal intensity by testing the signal intensity of known porosity standard specimens;(2) determine the porosity of the samples by the NMR signal intensity of different saturated samples.

3.Test results

3.1. Creep deformation behavior of coal based on NMRI

NMRI can detect the water content in coal using a gradient magnetic field to reflect the evolution process and spatial distribution of macropores and microfractures in coal during loading[51].The image transitions from red to blue,representing the corresponding position of the coal from higher to lower water content.Fig.2 shows the graded creep curves for deep coal under 2,3,and 4 MPa pore pressure.The strains in this section are all total axial creep strains.

Fig.2.Total axial creep curves at various pore pressures.

Creep deformation influenced by pore pressure and stress coupling was analyzed by comparing the creep curves at various pore pressures.From Fig.2,at pore pressures of 2,3,and 4 MPa,the stresses at failure of the coal were 45,42,and 34 MPa,respectively.The final creep strains were 1.14%,1.61%,and 2.30%in the order ofThe failure times of the coals were 62.01,60.71,and 42.57 h,in the order oft2＞t3＞t4.Notably,the increased pore pressure significantly influences the creep deformation behavior of coal.With the increase in pore pressure,the coal enters the accelerated creep stage earlier under lower axial stress,resulting in more substantial deformation.Especially atP=4 MPa,the coal undergoes a creep strain of 1.45% within 2 h.

The influence of pore pressure on coal creep is a multifaceted phenomenon governed by several intricate mechanisms.Elevated pore pressure decreases effective stress on the coal matrix contact surface and weakens coal matrix bonds.Consequently,fluid pressure functions as a lubricant,reducing cohesion and friction among coal particles thereby enhancing their fluidity.Furthermore,fluid flow resulting from variations in pore pressure influences stress redistribution within the coal,consequently accelerating the overall creep rate,as illustrated in Fig.2.Increased pore pressure intensifies fluidity among coal particles,magnifies the temporal impact on pore fracture structure evolution,and facilitates coal’s overall creep deformation.

Based on NMRI technology,the creep failure process of coal was further analyzed.Fig.3 combines NMRI to illustrate the creep deformation of the last two grades of creep at different pore pressures (G=0.9 andG=0.95).The creep strain and steady-state creep rate of coal increased gradually with the increased axial stress.Taking the NMRI results atP=3 MPa as an example,it can be observed that during the initial creep stage,macropores and microfractures are more developed and distributed more homogeneously,which is primarily expected for the homogeneous distribution of the initial pore fractures.As time progressed,the MPFS were gradually compressed,and only the larger microfractures located in the coal sample’s lower and middle areas were clearly observed.Following the accelerated creep stage,the MPFS developed rapidly and gradually extended upward along the microfractures in the bottom and middle of the coal,forming macroscopic fractures throughout the coal.This also provides clear evidence that accelerated creep occurs owing to the rapid intersection and development of the MPFS and that the final damage gradually accumulates to form penetrating fractures.NMRI can only provide a qualitative description of MPFS evolution,and the quantitative description of MPFS evolution will be provided in the next section.

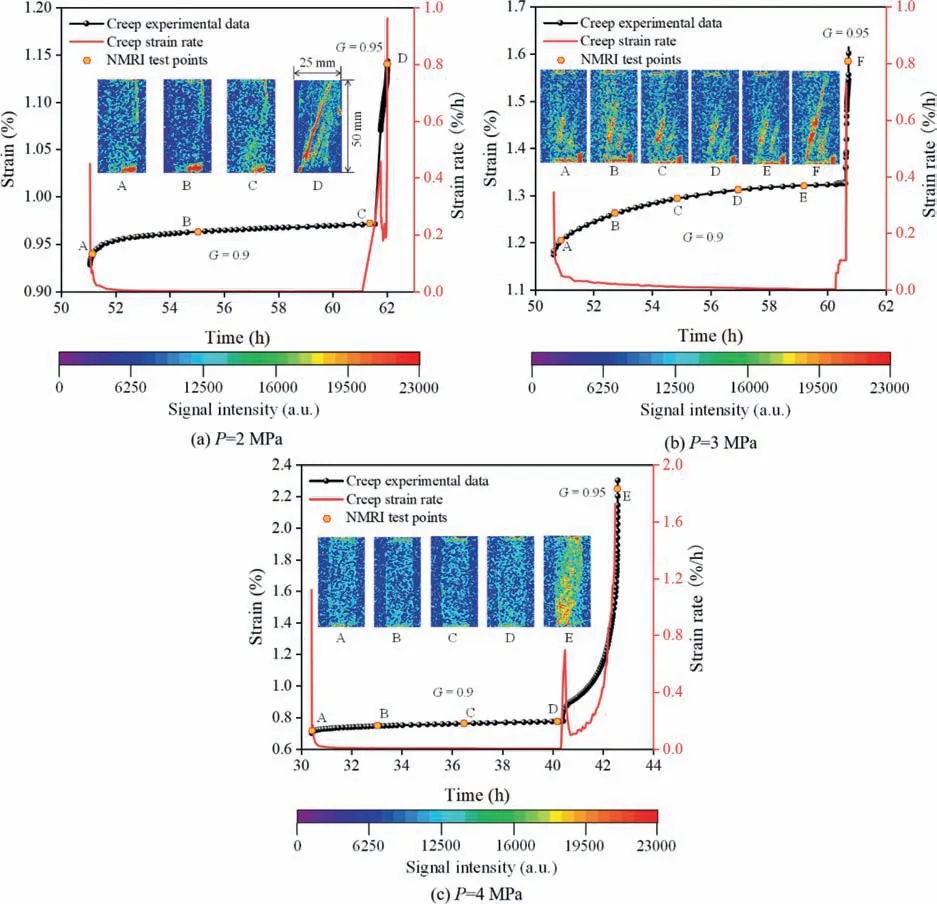
Fig.3.Total axial creep curves and NMRI of coal at G=0.9 and G=0.95.

3.2. Evolution of MPFS

The initialT2spectrum of the coal is depicted in Fig.4.According to the classical method of pore classification[23,52],total pore fractures (TP) can be divided into the left peak (T2＜2.5 ms) corresponding to micropores and transition pores (＜0.1 μm),which are defined as adsorption pores(AP)since they primarily influence the adsorption capacity of coal.The middle (2.5 ms＜T2＜100 ms)and right(T2＞100 ms)peaks correspond to mesopores,macropores,and microfractures,respectively.They are collectively known as SPM (＞0.1 μm) because of their effect on coal permeability.

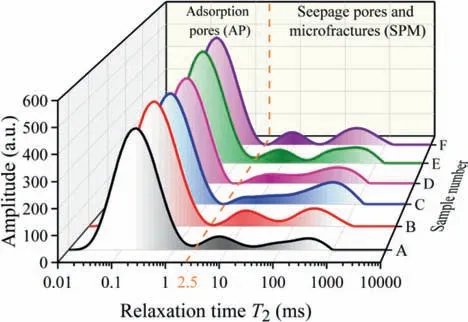
Fig.4.Initial T2 spectra of different coal samples at saturation.

A dimensionless parameterNis proposed to quantitatively analyze the evolution of various pores during creep,which considers the variation in theT2peak area.ParameterNfor different pores is defined as follows:

whereNA0,NS0,andNT0represent the peak areas of AP,SPM,and TP,respectively,at the beginning of the first grade of creep;NAi,NSi,andNTirepresent the peak areas of AP,SPM,and TP,respectively,at different creep times;andNA,NS,andNTrepresent the degrees of variation of AP,SPM,and TP,respectively,at different creep times.

To precisely depict the variation trends of different pores throughout the creep process,the experimental data were processed by the smoothing method [53],and theNA,NS,andNTvariability trends during the entire creep process were obtained,as shown in Fig.5.Regrettably,during creep atP=3 MPa,the heat-shrink tubing was destroyed before creep damage occurred,so the completeT2spectrum evolution was not monitored,but this did not affect the pre-failure results.

Fig.5.Evolution behavior of different pores during coal creep under different pore pressures.Note: Gi is the ith-grade creep.

At the beginning of creep (from first-grade to second-grade creep),the original SPM was gradually compressed,with maximum compression values ΔNSof the coal samples being 0.193,0.072,and 0.017 at pore pressures of 2,3,and 4 MPa,respectively,in the orderCorrespondingly,both AP and TP showed a gradual increase at the beginning of creep owing to SPM being gradually compressed into AP and producing new AP,which accounted for more than 70% of the overall pore structure.The maximum increase in AP during this process was 0.090,0.150,and 0.138 at pore pressures of 2,3,and 4 MPa,respectively.The SPM gradually increased as the stress level increased (from second-grade creep to pre-creep failure),showing a trend of gradual expansion and extension.The corresponding AP showed a decreasing trend,with the greatest reductions ΔNAof 0.022,0.040,and 0.069 at pore pressures of 2,3,and 4 MPa,respectively,in the orderHowever,the evolution of TP tended to be stable,indicating that the MPFS evolution of coal during this creep process was stable.During the final creep failure (from precreep failure to creep failure),AP,SPM,and TP rapidly increased at different pore pressures.Notably,only the AP showed a slow drop atP=4 MPa;the corresponding SPM exhibited a sudden and sharp increase,and the rate and magnitude of the increase were significantly greater than the change in TP.The primary reason is that the number of new AP was much smaller than the AP formed SPM at high pore pressures,and the extension of SPM dominated the creep process.

The microscopic deformation mechanism of coal creep at various pore pressures was revealed through the quantitative analysis of various pore structures during creep.The increase in pore pressure inhibited the compression closure of SPM during creep and promoted the development of AP into SPM.Coal is more likely to undergo extensional penetration at high pore pressures,resulting in large macroscopic deformation failures.Thus,accelerated creep occurred primarily because of the rapid penetration of the SPM.The high pore pressure indicates that the faster the penetration speed of the SPM is,the more likely the coal is to experience instability and destruction.

3.3. Porosity increment versus creep strain

To further determine the relationship between micro parameters and macro deformation during coal creep,the changes in coal porosity and creep strain during creep were compared and analyzed (Fig.6).As the loading gradeGincreased,both the porosity increment Δφ and creep strain ε inside the coal gradually increased,and the creep strain was linearly correlated with the porosity increment,a finding consistent with that of Zhou et al.[32].During graded creep,elevated stress levels induce the simultaneous compression,closure,and extension of coal containing numerous microporous fracture structures.Small pores are less susceptible to compression;only some large pores and microcracks will be compressed,as depicted in Fig.5.The interplay of creep stress and time effects triggers the evolution of the coal microstructure,involving mechanisms like grain rearrangement,dislocation motion,fracture healing,and partial pore reopening.Consequently,novel voids and microfractures emerge within the coal,contributing to an elevated coal porosity resulting from the gradual accumulation of damage during creep.

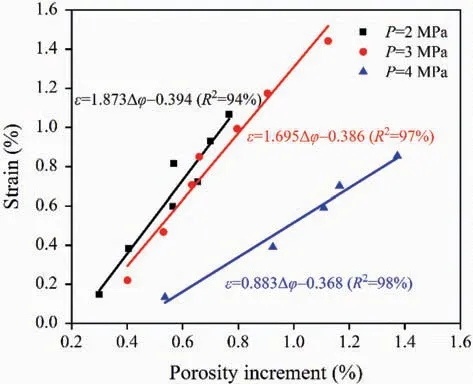
Fig.6.Porosity increment versus creep strain at various pore pressures.

Numerous studies have shown that increased stress levels during creep contribute to damage development [54].The microfractures within the coal gradually expand and extend over time,eventually intersecting to form macrofractures,resulting in increased porosity and creep strain in the coal.Therefore,the NMR-based evolution of coal MPFS can reflect the damage mechanism during creep from a microscopic perspective and indirectly describe the macroscopic creep deformation [1,32].

3.4.Characterization of macroscopic creep deformations of coal based on MPFS evolution

As demonstrated in Section 3.3,the MPFS evolution based on NMR could describe the macroscopic creep behavior of coal.The relationship between MPFS evolution and macroscopic creep deformation was investigated using Sun’s method [55] to determine the viscosity coefficient η of coal during triaxial creep,as shown in Eq.(3).

where σ1is the axial creep stress;andthe steady-state creep rate,is calculated using the slope of the creep curve during the steadystate creep stage [56].

Taking the two loading grades (G=0.8 andG=0.9) of coal before creep failure as examples,it could be observed that the viscosity coefficient in macroscopic creep deformation decays exponentially as pore pressure increases.The higher the loading grade,the more severe the attenuation of the viscosity coefficient (Fig.7a),a finding consistent with that of Yang et al.[13].Based on the findings in Section 3.2,it is clear that the decrease in the viscosity coefficient during coal creep is primarily owing to the increased pore pressure,which promotes the development of MPFS in coal,resulting in the deterioration of coal properties.Thus,the variation in the viscosity coefficient with increasing porosity at different pore pressures was established (Fig.7b).The exponential decay of the viscosity coefficient with increasing porosity increment further confirmed the reliability of the NMR-based microscopic parameters for characterizing the macroscopic creep deformations of coal.

Fig.7.Relationship between changes in pore structure and viscosity coefficient during coal creep at different pore pressures.

The variation in the porosity increment during accelerated creep was analyzed further to reveal the evolution trend of MPFS during accelerated creep and better establish the connection of MPFS evolution with macroscopic deformation.Fig.8 illustrates the evolution of the porosity increment over timetduring accelerated creep atP=4 MPa.The occurrence of accelerated creep could primarily be attributed to the exponential increase in porosity,leading to the gradual accumulation of damage that affects the mechanical performances of coal and eventually results in coal creep failure.Thus,a reasonable characterization of the macroscopic creep deformation behavior of coal with porosity changes provides a basis for subsequent creep modeling.

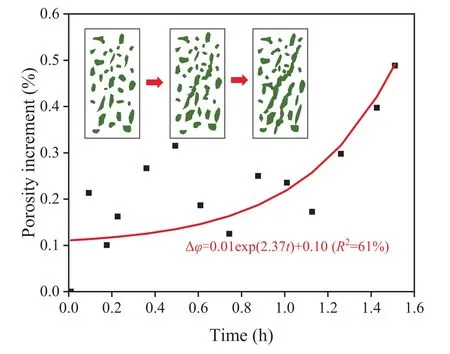
Fig.8.Evolution of porosity increment with time during accelerated creep stage(P=4 MPa).

4.Establishment of creep model

Certain mechanical components were refined based on the findings derived from online NMR creep experiments to investigate the creep behavior of deep coal under the concurrent influences of pore pressure and stress.The impacts of coupling pore pressure and stress on instantaneous and viscoelastic deformations during creep primarily entail enhancements to the Maxwell and Kelvin bodies.Meanwhile,modifying the ideal viscoplastic body addresses the influence of pore pressure and stress coupling on viscoplastic deformation during creep.Fig.9 illustrates the refined creep model and its corresponding creep deformations.

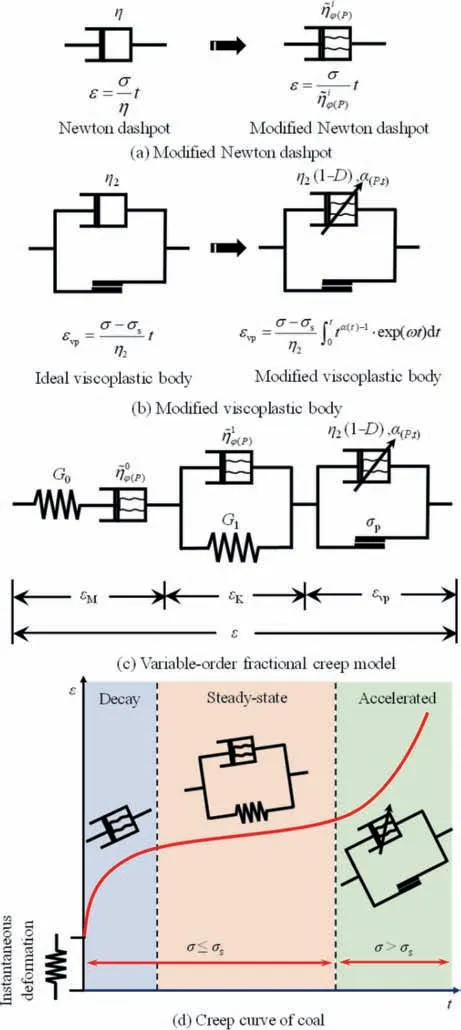
Fig.9.Mechanical elements and creep model taking the effect of pore pressure into account.

4.1. Modified mechanical elements

4.1.1.Modifiedviscoelasticelement

The Burgers model enables the characterization of the viscoelastic properties of rocks in deep underground engineering;however,it overlooks the influence of the deep occurrence environment.To study the impact of high pore pressure at depth on the safe mining of coal resources,we conducted an online creep test study on deep coal at different pore pressures using an NMR device with a mechanical loading function.The pore pressure significantly affected the MPFS evolution during creep,leading to an exponential decay of the coal viscosity coefficient (Fig.7a).Combined with related studies,it has been shown that the primary cause of creep damage in rocks at pore pressure and stress coupling is a sharp drop in the viscosity coefficient [46,57].

Therefore,the Newton dashpot in the Burgers model (Fig.9)was modified [58],considering the negative exponential relationship between the MPFS evolution and viscosity coefficient.

Considering the influence of pore pressure,the Maxwell body creep equation is

Considering the initial condition of ε(t)=0 att=0,the Kelvin body creep equation is

Considering the Boltzmann superposition principle,the expression for the modified Burgers model is

4.1.2.Modifiedviscoplasticelement

An ideal viscoplastic body is often used to characterize the viscoplastic deformation of materials;however,it cannot adequately characterize the accelerated creep process.To accurately depict the accelerated creep characteristics of coal under the combined influence of pore pressure and stress,refining the ideal viscoplastic body to align with the material’s accelerated creep behavior is essential.

As discussed in Section 3,we experimentally investigated the creep process at various pore pressures from a microscopic perspective based on NMR.It was observed that during the accelerated creep process,the MPFS of coal rapidly evolves and develops,resulting in the gradual accumulation of damage,which eventually leads to the accelerated deformation of the material and then creep failure.Furthermore,pore pressure promotes the evolution and development of MPFS,expedites coal damage accumulation,and induces continuous alterations in coal’s creep characteristics.Therefore,to describe the accelerated creep characteristics under the coupling effect of pore pressure and stress,it is necessary to consider the combined effects of loading stress,MPFS evolution,damage accumulation,pore pressure,and coal creep properties.

The fractional operator possesses a long-term historical dependence or memory effect,enabling the comprehensive characterization of stress loading history and deformation history within the material during the creep process.Hence,the inclusion of the Abel dashpot in the construction of the creep model enables the description of material properties across the continuum between the ideal solid(α=0)and the ideal fluid(α=1)during the creep process through order-variation[59].Considering the effect of damage accumulation on permanent viscous deformation during accelerated creep,Zhou et al.[60] introduced the damage variableDto the Abel dashpot and established the variable coefficient Abel dashpot based on the weakening of the viscosity coefficients,which is expressed as:

where α is the fractional order;η2the viscosity coefficient;and ω the material parameter,which is related to the properties of the coal.

Previous research revealed that fractional-order parameters can reflect the damage state and mechanical response of creep [61].Therefore,the variable coefficient Abel dashpots is enhanced based on microscopic experimental findings during coal creep deformation in Section 3.An improved variable-order Abel dashpot is formulated to capture the actual creep deformation characteristics in alignment with the evolving behavior of the MPFS.This allows for the representation of pore pressure influence and variations in creep properties through varying orders.Finally,the modified variable-order Abel dashpot is employed in lieu of the Newton dashpot in the ideal viscoplastic body to characterize the accelerated creep behavior of coal under the coupling of pore pressure and stress (Fig.9).

The modified variable-order Abel dashpot focuses on the effect of pore pressure and stress coupling on damage accumulation during accelerated creep.This is achieved by taking into account both the permanent viscous deformation of integer order and the fractional order contribution of pore pressure to the promotion of MPFS evolution.This requires the construction of a connection between integer and fractional order derivatives to realize the order crossover from integer to fractional order.We introduce conformable derivatives,which aligns with the classical definition of polynomials lifted to constant powers,proposed by Khalil et al.[62].The conformable derivative breaks the insurmountable gap between integer and fractional order derivatives and satisfies most of the properties of integer order derivatives.Hence,the conformable derivative can achieve a perfect description of the coupling effect by using an integer order derivative to characterize the decay of the permanent viscosity and a fractional order derivative to characterize the pore pressure promoting effect in the modified Abel dashpot.Considering the change of order in the modified Abel dashpot,the variable-order conformable derivative(VOCD)of a functionf:(0,∞)of order α(τ):(0,∞)→(0,1]is introduced as follows [63]:

where α(τ) is the varying-order function,which may be related to loading stress,strain,time,MPFS evolution,and damage accumulation in this study.

Importantly,it has the following relationship with the first derivative [63]:

VOCD is a local operator,and when the order α(τ)=1,it is reduced to the first derivative.Therefore,it has a similar algorithm to the first derivative,which can compensate for the complex disadvantage of fractional derivative operation.

Based on VOCD,the modified Abel dashpot can be expressed as:

In the modified Abel dashpot,a crucial consideration lies in determining an appropriate order function to reflect the pore pressure and the effect of MPFS evolution on the creep properties during the creep process.Referring to the evolution law of MPFS,the porosity increment is observed to increase exponentially with time during accelerated creep (Fig.8).An increase in pore pressure caused changes in the pore structure during creep,which affected the mechanical response of coal.Therefore,with the exponential growth in porosity increment over time due to pore pressure impact,the creep properties influenced by pore pressure are postulated to follow a negative exponential trend over time,i.e.,α（τ）=exp（-βt）,where β is the adjustment parameter of the coupling effect of pore pressure and stress,which is dependent on the properties and stress state of the coal.When β=0,α(τ)=1,the model degenerates into an ordinary Newton dashpot without considering the influence of pore pressure and the time-varying alterations in creep properties.When β varies,the magnitude of β can be utilized to portray the promoting effect of pore pressure on MPFS,consequently facilitating the depiction of the evolving characteristics of coal’s creep properties during the creep process.Interestingly,fort＞0,the range of α(τ)precisely falls within 0＜α(τ)≤1,indirectly affirming the reliability of the order function.

Accelerated creep is considered the process of MPFS evolution leading to damage accumulation when σ (creep stress) exceeds σs(long-term strength) [33,54,64].When σ＞σs,the viscoplastic body comes into play,where σpcan be denoted as

where σsis the yield stress;and σpthe stress in the friction element.

Considering the parallel relationship of the elements,the stress of the modified viscoplastic body is

where σdis the stress of the modified variable-order Abel dashpot.

Combining with Eq.(11),the expression for the modified viscoplastic body is as follows:

where η2is the viscosity coefficient of the viscoplastic body.

Substituting Eq.(10) into Eq.(14) yields the following relationship:

For VOCD,when α:[0,+∞)→(0,1),f:[0,+∞)→R are continuous functions,μ ∈R,then [63]:

The corresponding solution ofy(x) is

Considering the initial condition εvp=0 att=0 as well as Eq.(17),the analytical solution for the viscoplastic body is

The modified viscoplastic body can describe the macroscopic accelerated creep deformation behavior under the influence of pore pressure and stress coupling based on the MPFS evolution.Through the variable-order function determined based on the MPFS evolution law,it effectively encompasses the combined effects of various factors,including the loading stress history,MPFS evolution,damage accumulation,the impact of pore pressure,and alterations in coal creep properties throughout the creep process.Consequently,this offers a microscopic perspective for elucidating the variation in mechanical properties during coal creep.

4.2.Establishment of the creep model considering the influence of pore pressure

A fractional creep model (PFCM) that considers the effect of pore pressure was introduced by connecting a modified Burgers model in series with a modified viscoplastic body (Fig.9).This model can explain the creep deformation mechanism under the influence of pore pressure from a microscopic perspective.Since the coal seam is in a triaxial stress state,the PFCM is derived as a 3D stress state.

The pore pressure changes the stress environment of coal.Considering the effective stress principle [65],the actual stress during creep is

where σijis the nominal stress tensor;the effective stress tensor during creep;δijthe Kronecker delta function;Pthe pore pressure;andBthe Biot’s coefficient,generally approximated as 1.

Considering the Boltzmann superposition principle,the PFCM satisfies the following relationship:

The volume creep can be negligible,assuming coal is an isotropic material.The 3D expression for the modified Maxwell body is:

For the modified Kelvin body,the 3D expression is

whereG1is the shear modulus of the modified Kelvin body.

The 3D constitutive relation for the modified viscoplastic body was obtained using the Perzyna model[66]and the associated flow rule as follows:

whereFis the yield function of coal;F0the reference value of the initial yield function of coal;and φ(·)a power function with a power index of 1.

Considering that the stress-deviatoric tensor dominates the creep process,the followingFcan be used [58]

For the conventional triaxial compression testand consideringF0=1,the total expression for the 3D creep of the viscoplastic body in the axial direction is

Combined with Eqs.(21),(22),and (27),the 3D creep equation considering the influence of pore pressure is

5.Discussion

5.1. Validation of PFCM

The effectiveness of PFCM depends on how well it can fit experimental data on creep at different pore pressures.In this study,creep experimental data atP=2 MPa andP=4 MPa were selected to validate the model’s reliability in describing steady-and unsteady-state creeps,respectively.The selection of PFCM model parameters is primarily determined by creep experiment data and the physical significance of related parameters to establish their approximate ranges.Subsequently,the parameters are precisely determined through nonlinear least-squares fitting.The specific model parameters are listed in Table 3,and the fitting results for the creep model at different stages are shown in Fig.10.

Fig.10.Comparison analysis of creep experimental data and creep models at different pore pressures.

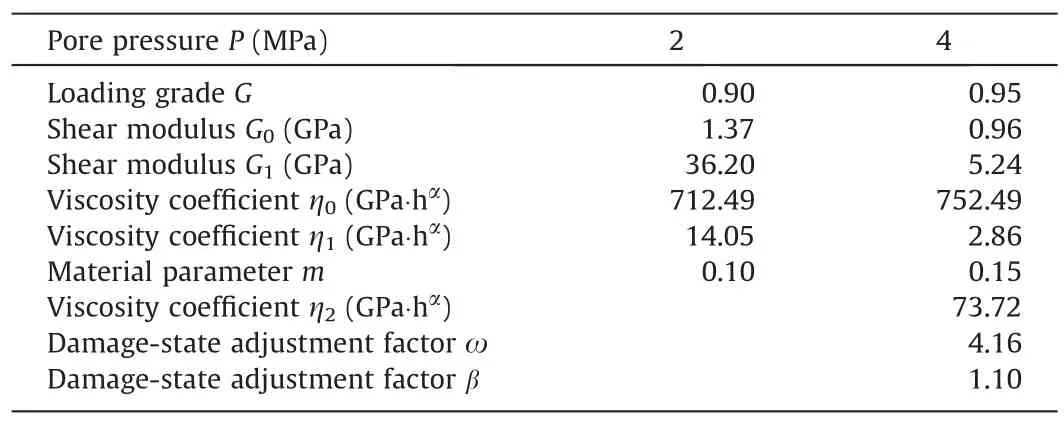
Table 3 PFCM parameters fitting results.

From Fig.10,PFCM describes the creep behavior of deep coal during pore pressure well.The correlation coefficientR2was greater than 96% for both steady-and unsteady-state creep with a rapid damage accumulation.The values of residual sum of squares(RSS)are small.PFCM can accurately characterize the nonlinear increase in creep strain caused by the rapid development of pore-fracture structures during the pore pressure increase,validating the model’s reliability in describing macroscopic creep deformations based on variations in microscopic pore-fracture parameters.

5.2. Parameter sensitivity analysis

Accelerated creep is vital for the safe extraction of deep underground resources.In this paper,a sensitivity analysis of the key parameters σ,ω,and β that affect the occurrence of acceleratedcreep at various pore pressures is performed.The effect of these parameters on the creep behavior and its mechanism is described.

Referring to the model parameters atP=4 MPa in Table 3,maintaining σ3=10 MPa and varying only the creep axial stress σ at 32,34,and 36 MPa,the creep curves are shown in Fig.11.Increased σ affects all three stages of creep deformation.The higher the σ,the greater the creep strain in all three stages,the greater the corresponding steady-state creep rate,and the faster the coal entered the accelerated creep stage,followed by large deformations,leading to coal failure.This agrees with the experimental results of this study and the actual deformation law of creep [67],which further validates the effectiveness and rationality of PFCM.

Fig.11.Influence of deviatoric stress on creep deformation.

There are two essential parameters ω and β,in the modified viscoplastic body that affect damage accumulation during accelerated creep.While maintaining the other parameters constant,the influence of pore pressures and stress coupling on creep deformation was investigated,and the action mechanism of each parameter was analyzed by varying the amounts of ω and β,respectively.Fig.12a shows the creep curves at ω=3.66,4.16,and 4.66.Fig.12b illustrates the creep curves for parameters β=0.1,0.6,and 1.1.From Fig.12,it is clear that both ω and β significantly affected the accelerated creep stage.The larger ω or the smaller β,the earlier the coal undergoes the accelerated creep stage,and the faster the creep deformation rate,the more likely it is to be damaged,leading to structural instability.

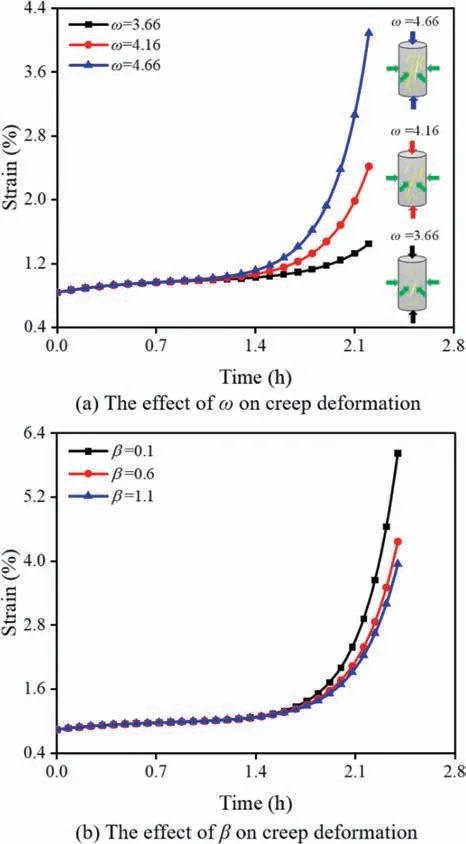
Fig.12.Effect of variation of ω and β on creep behavior.

Considering the MPFS evolution in NMR creep experiments,it becomes evident that the parameter β primarily signifies the promotional effect of pore pressure on the MPFS.Smaller β values correspond to a more pronounced enhancement of coal creep deformation induced by pore pressure.The MPFS evolves more smoothly and rapidly during the creep process,leading to changes in the mechanical properties of coal.Consequently,coal enters the accelerated creep stage earlier,making it more vulnerable to instability and failure during the creep process.

A smaller β corresponds to a more significant fractional order evolution when assessed in terms of the corresponding variableorder function.This effectively highlights that the accelerated creep process leads to substantial viscoplastic deformation,primarily driven by the progressive development of MPFS.As a result,the fractional order evolution approaches 1,indicative of a gradual increase in coal viscosity.Evidently,the time-varying order function α(τ) effectively captures variations in creep properties due to damage accumulation during accelerated creep,reflecting realtime changes in the MPFS and aligning more closely with coal’s actual deformation.Therefore,it is consistent to reflect the damage characteristics during coal creep through the MPFS evolution and the mechanical response of materials by employing the variableorder fractional derivative [68,69].

By conducting a sensitivity analysis of parameters ω and β,it becomes apparent that parameter ω primarily characterizes the difficulty level associated with accelerated creep and the extent of creep-induced damage in the current material.In contrast,parameter β primarily signifies the facilitating effect of pore pressure on the evolution of MPFS during creep.To further determine the physical meaning of parameters ω and β,the mechanism of parameters ω and β is explained and analyzed from the perspective of theoretical modeling in Section 4.The parameter ω primarily influences the magnitude of the damage variable;the larger the ω,the greater the damage variable,corresponding to more severe decay of the viscosity coefficient of the Abel dashpot.The parameter β mainly reflects the size of the coal’s plastic deformation under the influence of pore pressure by affecting the magnitude of the fractional order.The smaller the β,the slower the decay of the fractional order,resulting in greater plastic deformation produced by the coal.

Consequently,the fundamental influence mechanism of parameters ω and β is essentially identical.In this study,parameters ω and β are defined as damage-state adjustment factors that jointly influence damage characteristics,encompassing both size and degree of difficulty,during coal creep.Larger values of ω or smaller values of β correspond to a more pronounced intersection and penetration of the MPFS during coal creep,resulting in the rapid accumulation of damage.This,in turn,heightens the likelihood of instability and failure in underground structures.

6.Conclusions

Using an NMR real-time online test system with an in-situ mechanical loading test function,real-time detection and transparent description of the entire creep process in deep coal affected by different pore pressures were achieved.Combined with the evolution law of the MPFS during coal creep,a PFCM that considers the influence of pore pressure was proposed.The major conclusions are summarized below:

(1) The most evident change during creep was the evolution of the SPM.The increase in pore pressure inhibited the compression closure of SPM during creep and promoted the development of AP into SPM.Coal is more likely to expand and penetrate at high pore pressures.Combined with NMRI,it was observed that the evolution of the MPFS during creep generally started from dense primary pore fractures and then gradually expanded and penetrated to form macroscopic fractures.

(2) The relationship between microscopic parameters and macroscopic deformation during creep was established.The viscosity coefficient had a negative exponential decay with increasing porosity at different pore pressures.During accelerated creep,the exponential increase in porosity with time led to the gradual accumulation of coal damage and,thus,the eventual failure of creep.

(3) Considering the effect of pore pressure on MPFS evolution during creep,the Burgers model and ideal viscoplastic body were improved.PFCM,which considers the influence of pore pressure,was developed using the VOCD.The rationality of PFCM was verified using NMR-based experimental data.Through sensitivity analysis,damage-state adjustment factors ω and β were defined,and their capacity to characterize the role of damage state in the process of pore pressure’s influence on coal creep through MPFS evolution was established.

Acknowledgements

The present work is supported by the National Natural Science Foundation of China (Nos.52121003,51827901 and 52204110),China Postdoctoral Science Foundation (No.2022M722346),the 111 Project (No.B14006),and the Yueqi Outstanding Scholar Program of CUMTB (No.2017A03).