Fuel Consumption Analysis of Single and Twin-Screw Propulsion Systems of a Bulk Carrier

M.Tadros, M.Ventura and C.Guedes Soares

Abstract This paper presents a comparative analysis between single and twin-screw propulsion systems of a bulk carrier to evaluate the ship and propeller performance in terms of fuel consumption as well as to discuss the cavitation and noise criteria.An optimization model is developed to select the optimum propeller geometry and operational point along the engine load diagram for the selected engines of each case.The engines are selected from the same series due to the same behaviour along the engine load diagram.The propellers are selected from the B-series as fixed-pitch propellers.It has been concluded that while the components of the single-screw propulsion system are larger than the twin-screw,the single-screw propulsion system shows a reduction in fuel consumption than the twin screw by around 19%, thus affecting the amount of exhaust emissions from the ship.This model helps the ship designers to select a suitable propeller to improve the energy efficiency of the ships.

Keywords Propeller performance; Exhaust emissions; Energy efficiency; IMO; Decarbonization; Fuel consumption; Single and twin-screw

1 Introduction

According to the new stringent regulations issued by the International Maritime Organization (IMO), several tech‐nologies have been suggested and developed to reduce the amount of power required to operate the ships (Mallouppas and Yfantis, 2021; Tadros et al., 2023) for instance, opti‐mizing hull shape (Nazemian and Ghadimi, 2021), integra‐tion of air lubrication technologies (Hao, 2019), benefiting from wind energy (Seddiek and Ammar, 2021) and reduc‐tion of ship speed (Taskar et al., 2023).The selection of a suitable propulsion system during the preliminary stages of ship design is another concept and becomes an essential process (El-Gohary, 2013) to ensure the safety of the ship during the trip as well as the operation of the ship with the lowest amount of fuel onboard (Vettor et al., 2016; Zaccone et al., 2018; Moreira et al., 2021).This procedure directly affects the operation cost of the ship along the life span,which decreases the amount of fuel consumed and thus in‐creases the percentage of the profit of the ship owner (Du et al., 2022).This procedure is not specific to marine diesel oil, which shows an increment in the fuel price nowadays,as shown in Figure 1, but also for the other new type of fu‐els (El Gohary and Ammar, 2016; Karatuğ et al., 2022),including those that will be available in the market shortly,such as hydrogen, biofuel, ammonia and methanol (El-Go‐hary et al., 2014; Chiong et al., 2021; Tadros et al., 2021b;Vedachalam et al., 2022).

The typical propulsion system of large ships is com‐posed of the marine diesel engine coupled to a propeller through the propeller shaft and gearbox in the case of a four-stroke engine, and without a gearbox in the case of a two-stroke engine, thus various models are developed to be available to simulate their performance (Nahim et al.,2015; Altosole et al., 2017; Tadros et al., 2020a).

In addition to the engine, the different types of propel‐lers (Zainol and Yaakob, 2016; Najafi and Pourmostafa,2022) have been well designed due to the fast rapid tech‐nology that allows high-accuracy models during produc‐tion and provides more control during different operational conditions.

Figure 1 Average price at top 20 bunker ports (Miller, 2022)

Regarding the main source of power, the diesel engine,engines with high performance are already available in the market from different manufacturers to produce the re‐quired power supporting the ship’s needs to achieve a cer‐tain speed.The efficiency of the new engines has been in‐creased due to the control procedures applied on the differ‐ent parts of the engine and thus achieving more than 50%,showing a good increment compared to the old engines(Wartsila, 2023).The new engines have been optimized to reduce the amount of brake-specific fuel consumption(BSFC) for each operating point (Tadros et al., 2019; Tad‐ros et al., 2020b; Stoumpos and Theotokatos, 2020; Figari et al., 2022; Xiang et al., 2023).Thus, these types of proce‐dures allow the engines to comply with the stringent regu‐lations of international organizations towards mitigating climate change (Latarche, 2020).

These types of control are implemented in developing high-performance turbocharger(s) and intercoolers and pro‐viding control to the intake and exhaust valves (Wei et al.,2019; Wei et al., 2022), injection systems (Raeie et al.,2014; Tadros et al., 2020a) and exhaust gas recirculation(EGR) (Wang et al., 2021).The recuperation of the ex‐haust heat is seen in several solutions (Cherednichenko and Mitienkova, 2020; Cherednichenko and Serbin, 2018;Tadros et al., 2021c).

The main concept of implementing these types of con‐trols is to increase the amount of intake air inside the cyl‐inders accompanied by the perfect injection timing,which will reduce the amount of fuel consumption and the level of exhaust emissions (Zetterdahl et al., 2017;Tadros et al., 2020b; Fridell et al., 2021; Mocerino et al.,2021).Therefore, the development of several numerical models easily assists in the computation procedures to take the optimal decision and achieve highly accurate models, ensuring the sustainability of the propulsion sys‐tems (Benvenuto et al., 2021; Benvenuto and Campora,2022; Trivyza et al., 2022).These models can vary from the look-up table to the complex computational fluid dy‐namics (CFD) depending on the required level of detail of the computed results.

Regarding the propulsor, several profiles of propellers have been developed to be used according to the applica‐tion.The selection of an appropriate propeller(s) from the given series is important to ensure the generation of the re‐quired thrust to the ship.Also, it is crucial to take into con‐sideration the criteria of cavitation, noise and strength (Carl‐ton, 2012).For instance, Wageningen B-Series (Van Lam‐meren et al., 1969) has been widely used in commercial ships equipped with fixed-pitch propellers (FPP).However,by the increased demand for controllable-pitch propellers(CPP) to provide more control to the ship, Wageningen Pro‐peller C- and D-Series have been developed and taking place to serve the market demands (Dang et al., 2013).

Recently, the Wageningen F-series (Huisman et al., 2021)has been designed to provide the highest efficiency and compliance with cavitation limits for the merchant’s ves‐sels.The propeller is usually designed at the maximum propeller efficiency (Hong et al., 2014), while the selec‐tion of a propeller at the engine operating point with mini‐mum fuel consumption and power management can achieve a higher reduction in fuel consumption, either with a fixed pitch (Vesting and Bensow, 2018; Arapako‐poulos et al., 2019; Tadros et al., 2021a), a controllable pitch propeller (Jaurola et al., 2020; Bacciaglia et al.,2021; Tadros et al., 2022b) or contra-rotating propeller(Nouri et al., 2018; Tadros et al., 2022c).In addition, ap‐plying several modifications to the propeller, such as the cupping percentage (Hwang et al., 1995; Tadros et al.,2022e), boss cap fins (Xiong et al., 2013) or installing en‐ergy devices before or after the propeller (Stark et al.,2022) will help to improve the wake flow and thus in‐crease the propeller performance.

From the concept of design, designers need to select the optimal number of propulsion systems, including the num‐ber of engines and propellers.The single-screw propulsion has an advantage over twin-screw propulsion as it is simpler and consists of only one engine and one propeller.However,the engine and propeller can be bigger to produce the re‐quired power and thrust for the ship, therefore the loads on this system are larger than on the twin screw propulsion sys‐tem.On the other hand, the twin screw is more complex,where the number of each part of the propulsion system is doubled, as well as the initial cost, in addition to the hull modification by adding a twin-skeg to support the propul‐sion system (Williams, 1975; Kim et al., 2014).However,the engine and propeller size are smaller than the single screw propulsion, and the loads are distributed along the two propulsion systems.Also, it shows an advantage in emergen‐cies as the ship can operate with one of the two lines of the propulsion system until reaching the nearest safe port.

Usually, small ships with higher speeds require more than a single propulsion system due to the limitations of the stern part and stability computations to install a large engine with only one propeller and achieve the required speed.Therefore, the twin-screw propulsion system will be more effective than the single one.On the other hand,selecting the number of propulsion systems in large ships becomes more difficult as the ship can operate effectively using both systems (single and twin-screw).

However, due to international regulations and incre‐ments in fuel prices, it is beneficial to select the optimum propulsion system to ensure the lowest fuel consumption.To the authors’ knowledge, the research works already published and mentioned in the literature review mainly focus on determining the amount of power required and the reduction of the installed engine loading ratio without comparing the amount of fuel consumed between the dif‐ferent configurations.This is due to the lack of fuel con‐sumption information along the engine load diagram.

Therefore, this paper used the data of engine perfor‐mance previously calculated using engine simulation soft‐ware to present a comparative analysis between the single and twin-screw propulsion systems of a bulk carrier to evaluate the ship and propeller performance in terms of fuel consumption as well as discuss the cavitation and noise criteria.The computations are performed based on the propeller geometry of each case that is designed using optimization procedures and has been selected to mini‐mize fuel consumption.

The remainder of this paper is organized as follows.The main characteristics of the bulk carrier are presented in Section 2.Next, the numerical model used to perform the simulation is described in section 3.The discussion of the computed results is presented in section 4.Finally, a sum‐mary of the main findings and future recommendations are presented in Section 5.

2 Main characteristics of the bulk carrier

This study considers the selection of a bulk carrier’s sin‐gle and twin screw propellers from B-series as an FPP de‐pending on the number and rated power of the engine(s).The ship is 154 m in length and can sail at 14.5 kn.The ship is operated by a four-stroke marine diesel engine(s) from the series of MAN 32/44CR, where each cylinder’s power is 510 kW, and the engine’s total power differs according to the number of cylinders.The main characteristics of the ship and the engine are presented in Tables 1 and 2,respectively.

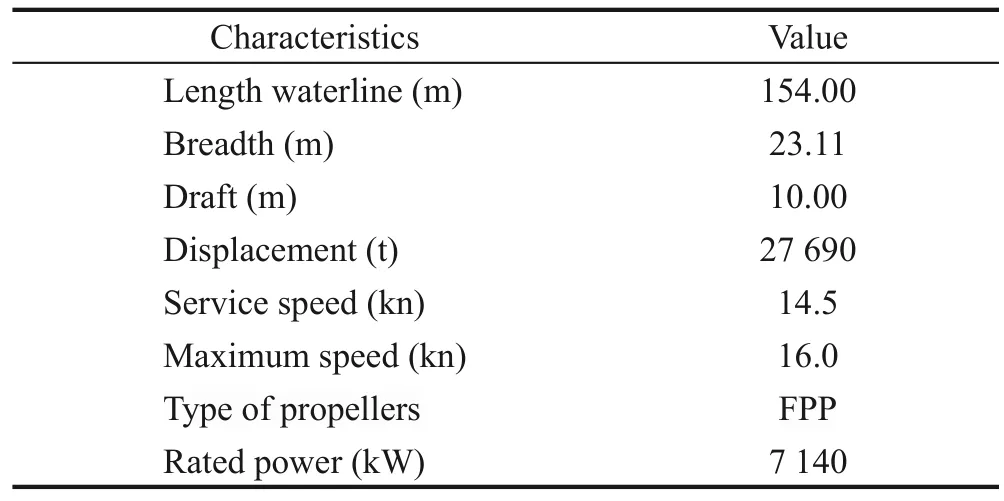
Table 1 Main characteristics of the bulk carrier

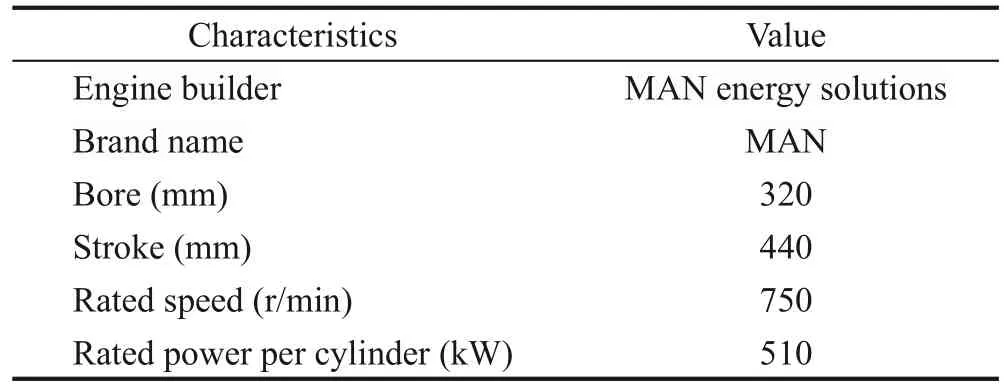
Table 2 Main characteristics of the diesel engine

3 Numerical model

The computation of each case is performed using a de‐veloped optimization model coupling NavCad (Hydro‐Comp, 2018) and an optimizer implemented in Matlab to select the optimum propeller geometry and the operational point of the propeller inside the engine load diagram for a given ship speed (Vs) and the number of propeller blades(Z).This model allows operational research techniques to find the optimal values of the design variables of the pro‐peller while minimizing the fuel consumption as the main objective of the model and complying with the limitations of several criteria as constraints, such as cavitation and noise.The design variables include propeller diameter (D),expanded area ratio (EAR), pitch diameter ratio (P/D) and gearbox ratio (GBR).Figure 2 shows a schematic diagram of the developed optimization model that is used in this study, coupling the different software components and showing the different variables and the output results of the optimization model.

In general, the optimization model based on the fmincon function can find the minimum of the problem specified by:

wheref(x) is the objective of the optimization model,xis the optimization variables,cis the inequality constraints,ceqis the equality constraints,Aas a matrix andbas a vec‐tor are the linear inequality constraints,Aeqas a matrix andbeqas a vector are the linear equality constraints, andlbandubare the lower and upper bounds, respectively.

This model is slightly converted, where the objective and constraints are defined into one single function called the fitness function.This conversion is performed to re‐duce the time of simulation combining all the computed re‐sults into one equation and evaluated by the optimizer as in the following equation.

whereRis a constant,gis the penalty function presenting the constraints, andjis the number of constraints.

The fuel consumption, FC, is computed using the fol‐lowing expression:

wherePBis the brake power.

The first step of the model is to compute the ship resis‐tance by defining all the required variables into the soft‐ware to be implemented into the numerical equations pre‐sented by Holtrop (1984) and Holtrop (1988) as the sug‐gested method by NavCad, which comply with the ship type and dimensions.This method shows good computa‐tional accuracy in the estimation of the ship resistance for several ship speeds (Gaafary et al., 2011; Elkafas et al.,2019; Islam et al., 2022) and ship conditions (Islam and Guedes Soares, 2019).After defining all the ship parame‐ters and operational speed, the total ship resistance is com‐puted, as shown in Figure 3, as a summation of the barehull resistance and marginal resistance taking into account the roughness of a clean hull (Tadros et al., 2022d).The marginal resistance is used to predict an appropriate value of the resistance as a percentage of the bare-hull resis‐tance.The value used in this study is 10% and corresponds to the feasibility estimation.

Once the resistance is computed, the simulation is then moved to compute the propulsion system as the second step.The method by Holtrop and Mennen (1982) is consid‐ered to compute the propulsive coefficient to evaluate the wake fraction (w), thrust deduction fraction (t) and relative rotative efficiency (ηRR), which differs from single and twin-screw.The main important parameters required in this step are the selection of propeller series, maximum propeller diameter, rated engine power and speed and the gearbox and propeller shaft efficiencies.

The value of these parameters differs for each case sug‐gested in this study, and they will be presented in detail in section 4.After defining all the variables, the ship power,the propulsive coefficients, the operation of the engine(s)and propeller(s), as well as the cavitation and noise crite‐ria are computed.The main characteristics of the propel‐ler, such as the advance coefficient (JA), thrust coefficient(KT), torque coefficient (KQ) and open water efficiency(ηo) are computed.The cavitation is evaluated by the method of Keller (1973) to compute the minimum EAR,Burrill and Emerson (1963) to compute the average load‐ing pressure, Blount and Fox (1978) to calculate the aver‐age predicted back cavitation percentage and the mini‐mum pitch as suggested by MacPherson (1991) to avoid face cavitation.The noise is computed based on the tip speed and the limits are provided in the manual of Hydro‐Comp (2018).More information about the methods and the limits of each criterion is presented in detail by Tad‐ros et al.(2022a).

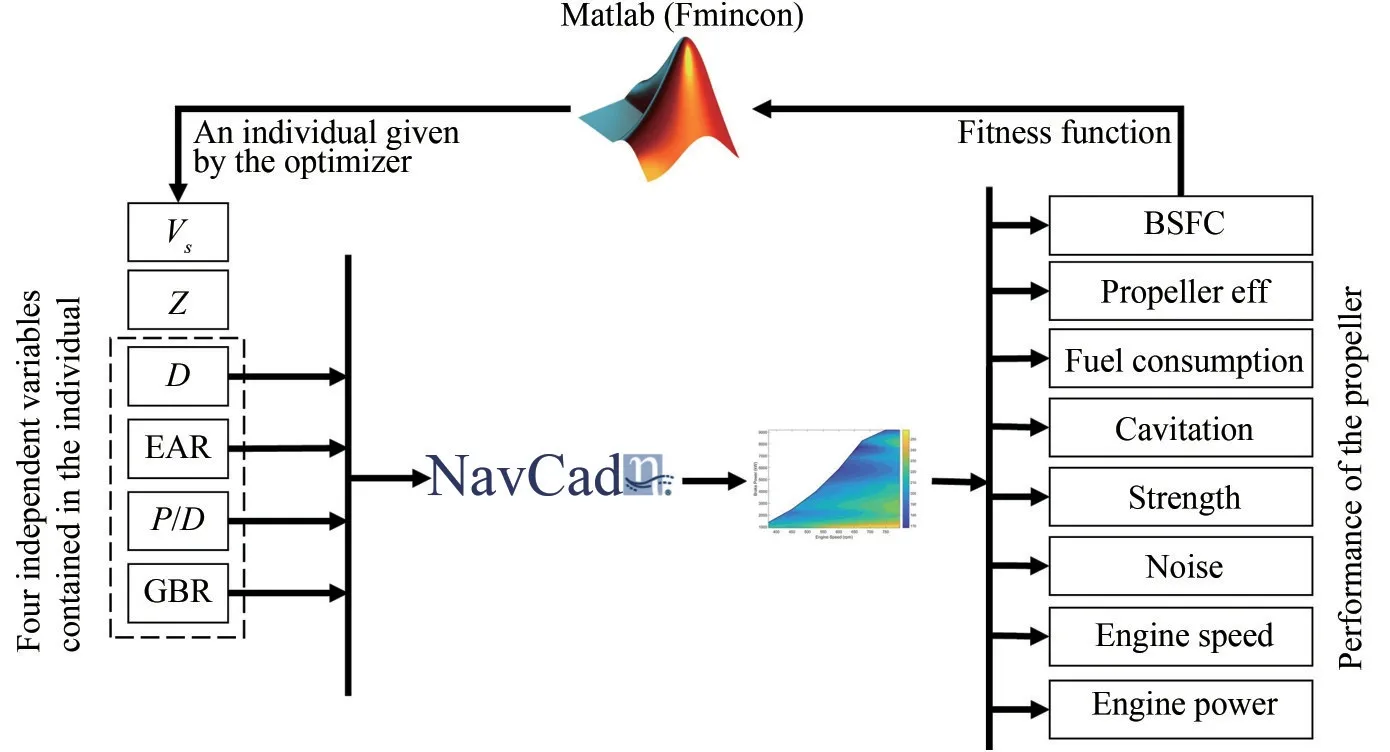
Figure 2 Schematic diagram of the developed optimization model (Tadros et al., 2021d)

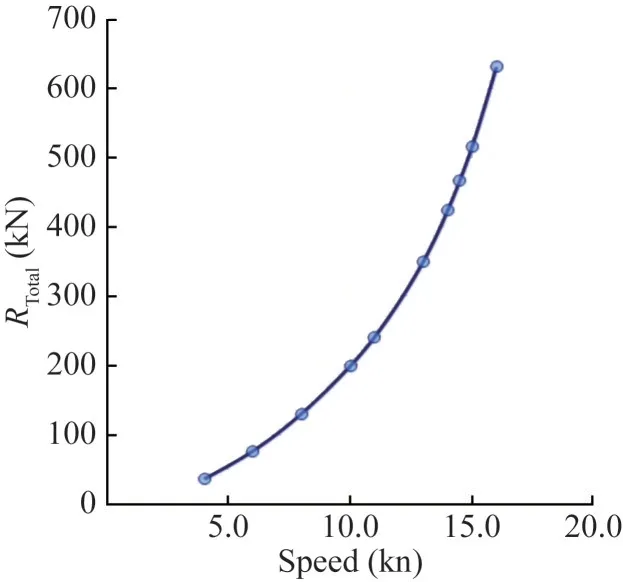
Figure 3 Total ship resistance for different ship speeds

4 Results and discussion

In order to perform the comparison between the two cas‐es, the simulation is performed twice, and the results are exported for each case.The first case concerns the simula‐tion of the performance of a single-screw propulsion sys‐tem, and in the second one, the performance of a twinscrew propulsion system is simulated.In both cases, the hull is assumed to be the same, and the total resistance is kept the same, as the difference between the two cases is the modification of the stern by adding another skeg in the second case, which can increase the wetted surface by a small amount than the first case.Therefore, it is preferable to keep the use of the same hull with the same resistance for comparison.

The engines from the same series MAN 32/44 CR are se‐lected as they have the same performance along the engine load diagram, even if the number of cylinders is changed.

In the first case, the engine with fourteen cylinders is se‐lected, providing 7 140 kW brake power and coupled with an FPP.In the second case, two engines with seven cylinders each are selected, each one able to produce 3 570 kW, and coupled as well to an FPP.The maximum diameter in each case is selected according to the shape of the stern.The max‐imum diameter in the first case is 60% of the ship draft, and the maximum diameter of each FPP in the second case is 50% of the ship draft.This maximum value is used to define the upper boundary of the optimization model, while the lower boundary is defined by 80% of the upper boundary.

Once all the parameters are defined, the input data are pro‐cessed into the optimization model and thus going through NavCad using the premium feature; then the results are com‐puted and exported into Excel spreadsheet files, which have been collected to evaluate the fitness function to reduce the fuel consumption as in equation (3) and comply with all the limit values of the constraints defined.The numerical opti‐mized results are presented in Table A1 for more informa‐tion, while the results are normalized in Figures 4 to 6 to provide a clear comparison between the two cases.

Regarding the ship characteristics, as shown in Figure 4,it has been shown that the two propulsion systems can pro‐vide the required thrust to operate the ship smoothly at 14.5 kn as service speeds.However, the single screw can allow the ship to reach 16 kn with more than 5% in the case of the twin screw.This is due to the larger diameter that is installed in the first case compared to the second one.Oth‐erwise, larger engines in the second case can replace the suggested ones to allow the ship to reach the same maxi‐mum speed as in the first case.Engines with eight cylinders can be selected to produce 4 080 kW to achieve the top speed, and thus the propeller can be designed to operate at lower loads and thus reduce fuel consumption.

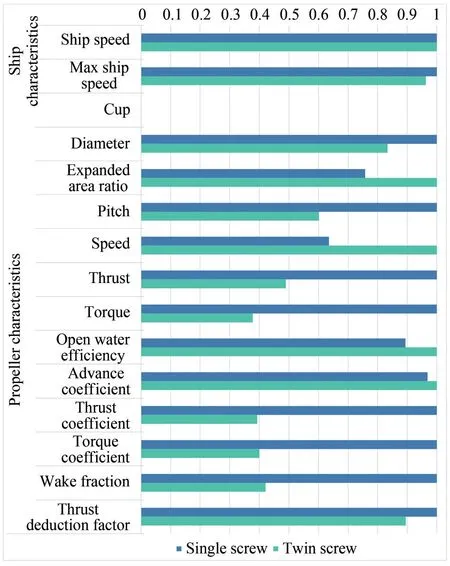
Figure 4 Ship and propeller characteristics of each calculated case

Regarding the propeller characteristics, the propeller di‐ameter is always selected at the upper boundary limits (6 m for the single screw and 5 m for the twin screw).This pa‐rameter shows its importance in increasing the propeller efficiency compared to the same propeller with lower di‐ameter, and the propeller can produce the required thrust at lower engine loads.It has been shown that the propeller thrust in the twin screw is a little bit lower than half of the single screw to achieve the same ship speed due to the pro‐pulsive coefficient calculations, in particular, the thrust de‐duction factor, which shows a reduction in the twin screw case by almost 10% compared to the single screw case.

As the propeller diameter decreases in the second case,the EAR is higher than in the first case to provide the re‐quired thrust to propel the ship at the same speed.The EAR in the twin screw case is higher by 25% than in the single screw case.This increment leads to an increase in the blade area and thus reduces the amount of pressure on the blades, which affects the cavitation criteria as de‐scribed in the next paragraphs.From the calculation, it has been shown that the pitch in the first case is higher by 40%than in the second case, this leads to achieve higher ship speed in the single screw than in the twin screw, also re‐ducing the engine loading conditions and reducing the fuel consumption, and thus affects the gear between the engine and propeller.Furthermore, it has been affected by the minimum pitch value in the cavitation section to avoid face cavitation, where the single screw has a higher mini‐mum pitch than the twin screw.Based on the pitch value,the propeller speed is reduced in the case of a higher pitch and increases in the case of a lower pitch.

Based on the overall propeller design, the open water ef‐ficiency is lower by 10% in the single screw case com‐pared to the twin screw case.A slight difference in advance coefficient between the two cases is noticed and not ex‐ceeding 2%.However, theKTandKQare in the twin screw are less by 60% compared to the single screw.Therefore,the thrust and torque are less in the second case than the first case by almost 50% to 60%.Based on the empirical formulas, the wake fraction in the twin screw case is lower by around 60% compared to the single screw case.

Regarding the cavitation criteria and due to the empirical equations to compute the propulsive coefficients, a change in thrust deduction factor is noticed between the two cases and, thus, a slight change in the total thrust of the two pro‐pellers by around 2% to achieve the design speed.

From Figure 5 and based on the propeller diameter and speed, the tip speed is reduced in the first case than in the second one, while both comply with the maximum tip speed to reduce the noise.The minimum EAR using the Keller method is higher in the first case than the second one and is equal to the selected EAR; however, the mini‐mum EAR in the twin-screw propeller is reduced due to the split of the required thrust by the two propellers.The same behaviour is followed by the other cavitation criteria,where both the average loading pressure and the back cavi‐tation are lower in the case of a twin screw than the single screw.As the propeller speed is lower in the first case than in the second one, the GBR is higher in the first case as the rated engine speed is the keeping same (750 r/min).

Regarding the engine performance in Figure 6, the singlescrew propeller is selected at a lower loading ratio due to the large propeller diameter than the twin screw as it plays effectively to provide higher thrust at lower engine power.A big difference in the loading ratio between the two cases has been noticed, where the difference in brake power reaches 19% higher in the twin screw propeller for the two lines of the propulsion system compared to the single screw.

Figure 5 Cavitation and noise criteria of each calculated case

Figure 6 Gearbox and engine characteristics as well as exhaust emissions of each calculated case

This increment in the brake power for each engine in the second case will increase the total amount of fuel con‐sumed in the first case by around 19% to achieve the same speed.Thus, affects the total energy efficiency of the ship based on the values of the different exhaust emissions, in‐cluding carbon dioxide (CO2), nitrogen oxides (NOx) and sulphur oxides (SOx) that will increase according to the in‐crement in fuel consumption.

5 Conclusions

This paper presents a comparative study between two types of propulsion systems for the concept of ship design of a bulk carrier, helping ship designers towards improv‐ing the energy efficiency of the ships.The first case is a single-screw propulsion system, and the second one is a twin-screw propulsion system.The engines in each case are selected from the same series due to the same behav‐iour along the engine load diagram.Based on the devel‐oped optimization model coupling NavCad and Matlab,the geometry of the propellers is optimized based on the selected B-series as an FPP as well as the gearbox ratio for each case.The hull of the ship is assumed to be the same,as the difference in the total resistance in both cases is small and can be negligible.From the computed results, it has been concluded that:

1) It is important to select an optimum propulsion sys‐tem that is not complex and can operate at lower fuel con‐sumption due to the higher fuel prices.

2) The computed results can be extended to reach any kind of engine operated with any type of fuel.

3) The optimization model can successfully find the op‐timum value of propeller geometry and operation for dif‐ferent configurations.

4) While the components of the single-screw propulsion system are larger than the twin-screw, the single-screw propulsion system shows advantages over the twin screw in terms of fuel consumption by around 19% and thus re‐ducing the level of exhaust emissions.

5) The twin-screw propulsion system performs better in cavitation while evaluating the different cavitation criteria due to the pressure distribution over the two propellers.

The model can be extended to implement the prices of each part to provide an economic study of the capital ex‐penditure (CAPEX) besides the operating expenses(OPEX) along a specific route.Also, hybrid propulsion systems combining dual-fuel engines and electrical compo‐nents can be considered for further research.

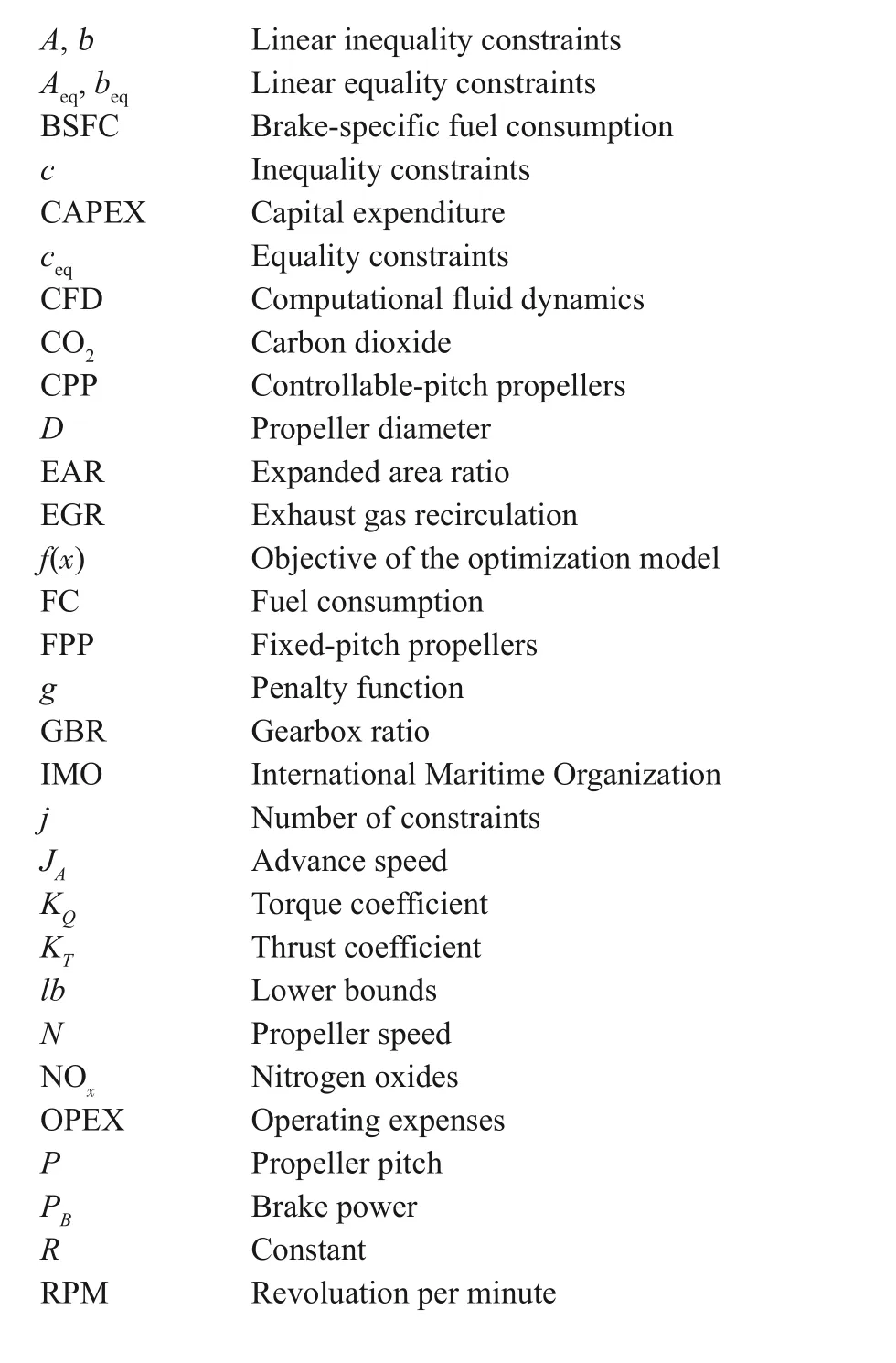
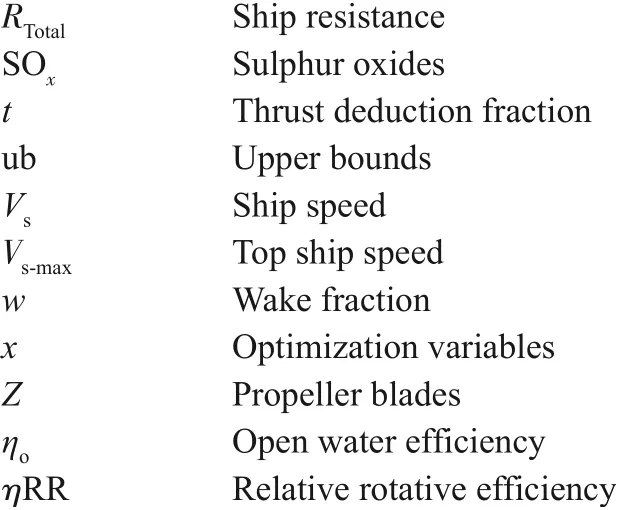
Nomenclature

Appendix

Table A1 Characteristics of different propulsion configurations

Competing interestC.Guedes Soares is one of Editors for the Journal of Marine Science and Application and was not involved in the editorial review, or the decision to publish this article.All authors declare that there are no other competing interests.

ReferencesAltosole M, Benvenuto G, Campora U, Laviola M, Zaccone R(2017) Simulation and performance comparison between diesel and natural gas engines for marine applications.Proceedings of the Institution of Mechanical Engineers, Part M: Journal of Engineering for the Maritime Environment 231(2): 690-704.https://doi.org/10.1177/1475090217690964

Arapakopoulos A, Polichshuk R, Segizbayev Z, Ospanov S, Ginnis AI, Kostas KV (2019) Parametric models for marine propellers.Ocean Engineering 192: 106595.https://doi.org/10.1016/j.oceaneng.2019.106595

Bacciaglia A, Ceruti A, Liverani A (2021) Controllable pitch propeller optimization through meta-heuristic algorithm.Engineering with Computers 37: 2257-2271.https://www.doi.org/10.1007/s00366-020-00938-8

Benvenuto G, Campora U (2022) Influence of the marine engine load diagram characteristics on the ship propulsion system performance.International Journal of Frontiers in Engineering and Technology Research 3(2): 59-67.https://doi.org/10.53294/ijfetr.2022.3.2.0059

Benvenuto G, Campora U, Altosole M, Balsamo F (2021) Numerical modelling and analysis of the ambient conditions influence on the performance of a marine diesel engine.In: Guedes Soares C,Santos TA Eds.Developments in Maritime Technology and Engineering.Taylor and Francis, London, 463-474

Blount DL, Fox DL (1978) Design considerations for propellers in a cavitating environment.Marine Technology 15(2): 144-178.https://www.doi.org/10.5957/mt1.1978.15.2.144

Burrill LC, Emerson A (1963) Propeller cavitation: Further tests on 16in propeller models in the King’s College cavitation tunnel.International Shipbuilding Progress 10(104): 119-131.https://www.doi.org/10.3233/isp-1963-1010402

Carlton J (2012) Marine propellers and propulsion.Butterworth-Heinemann, Oxford

Cherednichenko O, Mitienkova V (2020) Analysis of the impact of thermochemical recuperation of waste heat on the energy efficiency of gas carriers.Journal of Marine Science and Application 19(1):72-82.https://doi.org/10.1007/s11804-020-00127-5

Cherednichenko O, Serbin S (2018) Analysis of efficiency of the ship propulsion system with thermochemical recuperation of waste heat.Journal of Marine Science and Application 17(1):122-130.https://doi.org/10.1007/s11804-018-0012-x

Chiong MC, Kang HS, Shaharuddin NMR, Mat S, Quen LK, Ten KH,Ong MC (2021) Challenges and opportunities of marine propulsion with alternative fuels.Renewable and Sustainable Energy Reviews 149: 111397.https://doi.org/10.1016/j.rser.2021.111397

Dang J, Van den Boom HJJ, Ligtelijn JT (2013) The Wageningen Cand D-series propellers.12th International Conference on Fast Sea Transportation (FAST)

Du W, Li Y, Zhang G, Wang C, Zhu B, Qiao J (2022) Energy saving method for ship weather routing optimization.Ocean Engineering 258: 111771.https://doi.org/10.1016/j.oceaneng.2022.111771

El-Gohary MM (2013) Overview of past, present and future marine power plants.Journal of Marine Science and Application 12(2):219-227.https://doi.org/10.1007/s11804-013-1188-8

El-Gohary MM, Welaya YMA, Saad AA (2014) The use of hydrogen as a fuel for inland waterway units.Journal of Marine Science and Application 13(2): 212-217.https://doi.org/10.1007/s11804-014-1243-0

El Gohary MM, Ammar NR (2016) Thermodynamic analysis of alternative marine fuels for marine gas turbine power plants.Journal of Marine Science and Application 15(1): 95-103.https://doi.org/10.1007/s11804-016-1346-x

Elkafas AG, Elgohary MM, Zeid AE (2019) Numerical study on the hydrodynamic drag force of a container ship model.Alexandria Engineering Journal 58(3): 849-859.https://doi.org/10.1016/j.aej.2019.07.004

Figari M, Theotokatos G, Coraddu A, Stoumpos S, Mondella T(2022) Parametric investigation and optimal selection of the hybrid turbocharger system for a large marine four-stroke dualfuel engine.Applied Thermal Engineering 208, 117991.https://doi.org/10.1016/j.applthermaleng.2021.117991

Fridell E, Salberg H, Salo K (2021) Measurements of emissions to air from a marine engine fueled by methanol.Journal of Marine Science and Application 20(1): 138-143.https://doi.org/10.1007/s11804-020-00150-6

Gaafary MM, El-Kilani HS, Moustafa MM (2011) Optimum design of B-series marine propellers.Alexandria Engineering Journal 50(1): 13-18.https://doi.org/10.1016/j.aej.2011.01.001

Hao W (2019) Numerical study of the effect of ship attitude on the perform of ship with air injection in bottom cavity.Ocean Engineering 186, 106119.https://doi.org/10.1016/j.oceaneng.2019.106119

Holtrop J (1984) A statistical re-analysis of resistance and propulsion data.International Shipbuilding Progress 31(363): 272-276

Holtrop J (1988) A statistical resistance prediction method with a speed dependent form factor.Proceedings of Scientific and Methodological Seminar on Ship Hydrodynamics (SMSSH ‘88).Bulgarian Ship Hydrodynamics Centre, Varna, Bulgaria, 1-7

Holtrop J, Mennen GGJ (1982) An approximate power prediction method.International Shipbuilding Progress 29(335): 166-170

Hong Y, Hao LF, Wang PC, Liu WB, Zhang HM, Wang RG (2014)Structural design and multi-objective evaluation of composite bladed propeller.Polymers and Polymer Composites 22(3): 275-282.https://doi.org/10.1177/096739111402200308

Huisman J, Foeth E-J, Slot J, Lampe A, Moulijn J, Dang J (2021)Design of the Wageningen F-series.MARIN, Wageningen,Netherlands

Hwang JL, Tsai JF, Li, CY (1995) Cupped propeller test and analysis.Ship Technology Research 42(4): 186-192

HydroComp (2018) NavCad: Reliable and confident performance prediction.NH, USA: HydroComp Inc.Available from https://www.hydrocompinc.com/solutions/navcad/.[Accessed on 30 January 2019]

Islam H, Guedes Soares C (2019) Effect of trim on container ship resistance at different ship speeds and drafts.Ocean Engineering 183: 106-115.https://doi.org/10.1016/j.oceaneng.2019.03.058

Islam H, Ventura M, Guedes Soares C, Tadros M, Abdelwahab HS(2022) Comparison between empirical and CFD based methods for ship resistance and power prediction.In: Guedes Soares C,Santos TA Eds.Trends in Maritime Technology and Engineering.Taylor & Francis Group, London, 347-357

Jaurola M, Hedin A, Tikkanen S, Huhtala K (2020) A TOpti simulation for finding fuel saving by optimising propulsion control and power management.Journal of Marine Science and Technology 25(2): 411-425.https://www.doi.org/10.1007/s00773-019-00651-2

Karatuğ Ç, Arslanoğlu Y, Guedes Soares C (2022) Evaluation of decarbonization strategies for existing ships.In: Guedes Soares C, Santos TA, Eds.Trends in Maritime Technology and Engineering.Taylor & Francis Group, London, 45-54

Keller WH (1973) Extended diagrams for determining the resistance and required power for single-screw ships.International Shipbuilding Progress 20: 133-142

Kim K, Tillig F, Bathfield N, Liljenberg H (2014) Hydrodynamic optimization of twin-skeg LNG ships by CFD and model testing.International Journal of Naval Architecture and Ocean Engineering 6(2): 392-405.https://doi.org/10.2478/IJNAOE-2013-0187

Latarche M (2020) Pounder's marine diesel engines and gas turbines.Elsevier Ltd., Oxford

MacPherson DM (1991) Reliable propeller selection for work boats and pleasure craft: techniques using a personal computer.SNAME Fourth Biennial Power Boat Symposium, New Jersey,USA: SNAME

Mallouppas G, Yfantis EA (2021) Decarbonization in shipping industry: a review of research, technology development, and innovation proposals.Journal of Marine Science and Engineering 9(4): 415.https://doi.org/10.3390/jmse9040415

Miller G (2022) Ship fuel enters uncharted territory as prices hit new wartime peak.Available from https://www.freightwaves.com/news/ship-fuel-enters-uncharted-territory-as-prices-hit-newwartime-peak.[Accessed on 10 September 2022]

Mocerino L, Guedes Soares C, Rizzuto E, Balsamo F, Quaranta F(2021) Validation of an emission model for a marine diesel engine with data from sea operations.Journal of Marine Science and Application 20(3): 534-545.https://doi.org/10.1007/s11804-021-00227-w

Moreira L, Vettor R, Guedes Soares C (2021) Neural network approach for predicting ship speed and fuel consumption.Journal of Marine Science and Engineering 9(2): 119.https://doi.org/10.3390/jmse9020119

Nahim HM, Younes R, Nohra C, Ouladsine M (2015) Complete modeling for systems of a marine diesel engine.Journal of Marine Science and Application 14(1): 93-104.https://doi.org/10.1007/s11804-015-1285-y

Najafi S, Pourmostafa M (2022) Investigating the performance of twin marine propellers in different ship wake fields using an unsteady viscous and inviscid solver.Journal of Marine Science and Application 21(2): 92-105.https://doi.org/10.1007/s11804-022-00279-6

Nazemian A, Ghadimi P (2021) Multi-objective optimization of ship hull modification based on resistance and wake field improvement: combination of adjoint solver and CAD-CFDbased approach.Journal of the Brazilian Society of Mechanical Sciences and Engineering 44(1): 27.https://doi.org/10.1007/s40430-021-03335-4

Nouri NM, Mohammadi S, Zarezadeh M (2018) Optimization of a marine contra-rotating propellers set.Ocean Engineering 167:397-404.https://doi.org/10.1016/j.oceaneng.2018.05.067

Raeie N, Emami S, Karimi Sadaghiyani O (2014) Effects of injection timing, before and after top dead center on the propulsion and power in a diesel engine.Propulsion and Power Research 3(2):59-67.http://dx.doi.org/10.1016/j.jppr.2014.06.001

Seddiek IS, Ammar NR (2021) Harnessing wind energy on merchant ships: case study Flettner rotors onboard bulk carriers.Environmental Science and Pollution Research 28(25): 32695-32707.https://www.doi.org/10.1007/s11356-021-12791-3

Stark C, Xu Y, Zhang M, Yuan Z, Tao L, Shi W (2022) Study on applicability of energy-saving devices to hydrogen fuel cellpowered ships.Journal of Marine Science and Engineering 10(3):388.https://doi.org/10.3390/jmse10030388

Stoumpos S, Theotokatos G (2020) Multiobjective optimisation of a marine dual fuel engine equipped with exhaust gas recirculation and air bypass systems.Energies 13(19): 5021.https://doi.org/10.3390/en13195021

Tadros M, Ventura M, Guedes Soares C (2019) Optimization procedure to minimize fuel consumption of a four-stroke marine turbocharged diesel engine.Energy 168: 897-908.https://doi.org/10.1016/j.energy.2018.11.146

Tadros M, Ventura M, Guedes Soares C (2020a) A nonlinear optimization tool to simulate a marine propulsion system for ship conceptual design.Ocean Engineering 210: 107417.https://doi.org/10.1016/j.oceaneng.2020.107417

Tadros M, Ventura M, Guedes Soares C (2020b) Optimization of the performance of marine diesel engines to minimize the formation of SOxemissions.Journal of Marine Science and Application 19(3): 473–484.https://www.doi.org/10.1007/s11804-020-00156-0

Tadros M, Ventura M, Guedes Soares C (2021a) Design of propeller series optimizing fuel consumption and propeller efficiency.Journal of Marine Science and Engineering 9(11): 1226.https://doi.org/10.3390/jmse9111226

Tadros M, Ventura M, Guedes Soares C (2021b) A review of the use of Biodiesel as a green fuel for diesel engines.In: Guedes Soares C, Santos T Eds.Developments in Maritime Technology and Engineering.Taylor & Francis Group, London, 481-490

Tadros M, Ventura M, Guedes Soares C (2021c) Sensitivity analysis of the steam Rankine cycle in marine applications.In: Guedes Soares C, Santos T Eds.Developments in Maritime Technology and Engineering.Taylor & Francis Group, London, 491-500

Tadros M, Ventura M, Guedes Soares C (2022a) An optimisation procedure for propeller selection for different shaft inclinations.International Journal of Maritime Engineering 164(A3): 295-315.https://doi.org/10.5750/ijme.v164iA3.809

Tadros M, Ventura M, Guedes Soares C (2022b) Optimization procedures for a twin controllable pitch propeller of a ROPAX ship at minimum fuel consumption.Journal of Marine Engineering and Technology.https://doi.org/10.1080/20464177.2022.2106623

Tadros M, Ventura M, Guedes Soares C (2022c) Towards fuel consumption reduction based on the optimum contra-rotating propeller.Journal of Marine Science and Engineering 10(11):1657.https://doi.org/10.3390/jmse10111657

Tadros M, Ventura M, Guedes Soares C (2023) Review of current regulations, available technologies, and future trends in the green shipping industry.Ocean Engineering 280, 114670.https://doi.org/10.1016/j.oceaneng.2023.114670

Tadros M, Vettor R, Ventura M, Guedes Soares C (2021d) Coupled engine-propeller selection procedure to minimize fuel consumption at a specified speed.Journal of Marine Science and Engineering 9(1): 59.https://doi.org/10.3390/jmse9010059

Tadros M, Vettor R, Ventura M, Guedes Soares C (2022d)Assessment of ship fuel consumption for different hull roughness in realistic weather conditions.Journal of Marine Science and Engineering 10(12): 1891.https://doi.org/10.3390/jmse10121891

Tadros M, Vettor R, Ventura M, Guedes Soares C (2022e) Effect of propeller cup on the reduction of fuel consumption in realistic weather conditions.Journal of Marine Science and Engineering 10(8): 1039.https://doi.org/10.3390/jmse10081039

Taskar B, Sasmal K, Yiew LJ (2023) A case study for the assessment of fuel savings using speed optimization.Ocean Engineering 274: 113990.https://doi.org/10.1016/j.oceaneng.2023.113990

Trivyza NL, Rentizelas A, Theotokatos G, Boulougouris E (2022)Decision support methods for sustainable ship energy systems: A state-of-the-art review.Energy 239: 122288.https://doi.org/10.1016/j.energy.2021.122288

Van Lammeren WPA, van Manen JD, Oosterveld MWC (1969) The Wageningen B-screw series.Trans.SNAME 77(8): 269-317

Vedachalam S, Baquerizo N, Dalai AK (2022) Review on impacts of low sulfur regulations on marine fuels and compliance options.Fuel 310: 122243.https://doi.org/10.1016/j.fuel.2021.122243

Vesting F, Bensow RE (2018) Particle swarm optimization: an alternative in marine propeller optimization? Engineering Optimization 50(1): 70-88.https://www.doi.org/10.1080/0305215X.2017.1302438

Vettor R, Tadros M, Ventura M, Guedes Soares C (2016) Route planning of a fishing vessel in coastal waters with fuel consumption restraint.In: Guedes Soares C, Santos TA Eds.Maritime Technology and Engineering 3.Taylor & Francis Group, London, 167-173

Wang P, Tang X, Shi L, Ni X, Hu Z, Deng K (2021) Experimental investigation of the influences of Miller cycle combined with EGR on performance, energy and exergy characteristics of a fourstroke marine regulated two-stage turbocharged diesel engine.Fuel 300, 120940.https://doi.org/10.1016/j.fuel.2021.120940.

Wartsila (2023) Improving efficiency.Available from https://www.wartsila.com/sustainability/climate-and-environment/innovatingfor-sustainability/improving-efficiency.[Accessed on 05 May 2023]

Wei S, Wu C, Yan S, Ding T, Chen J (2022) Miller cycle with early intake valve closing in marine medium-speed diesel engines.Journal of Marine Science and Application 21(1): 151-160.https://doi.org/10.1007/s11804-022-00250-5

Wei S, Zhao X, Liu X, Qu X, He C, Leng X (2019) Research on effects of early intake valve closure (EIVC) miller cycle on combustion and emissions of marine diesel engines at medium and low loads.Energy 173: 48-58.https://doi.org/10.1016/j.energy.2019.01.110

Williams A (1975) Single- and twin-screw propulsion of tankers and bulk carriers.First Ship Technology and Research (STAR)Symposium, 26-29 August 1975, Washington, D.C.: Society of Naval Architects and Marine Engineers

Xiang L, Theotokatos G, Ding Y (2023) Parametric investigation on the performance-emissions trade-off and knocking occurrence of dual fuel engines using CFD.Fuel 340, 127535.https://doi.org/10.1016/j.fuel.2023.127535

Xiong Y, Wang Z, Qi W (2013) Numerical study on the influence of boss cap fins on efficiency of controllable-pitch propeller.Journal of Marine Science and Application 12(1): 13-20.https://doi.org/10.1007/s11804-013-1166-9

Zaccone R, Ottaviani E, Figari M, Altosole M (2018) Ship voyage optimization for safe and energy-efficient navigation: A dynamic programming approach.Ocean Engineering 153: 215-224.https://doi.org/10.1016/j.oceaneng.2018.01.100

Zainol I, Yaakob O (2016) Use of diesel engine and surface-piercing propeller to achieve fuel savings for inshore fishing boats.Journal of Marine Science and Application 15(2): 214-221.https://doi.org/10.1007/s11804-016-1336-z

Zetterdahl M, Salo K, Fridell E, Sjöblom J (2017) Impact of aromatic concentration in marine fuels on particle emissions.Journal of Marine Science and Application 16(3): 352-361.https://doi.org/10.1007/s11804-017-1417-7