Magnetic properties and magnetocaloric effects of Tm1−xErxCuAl(x=0.25,0.5,and 0.75)compounds

Hao Sun(孙浩) Junfeng Wang(王俊峰) Lu Tian(田路) Jianjian Gong(巩建建)Zhaojun Mo(莫兆军) Jun Shen(沈俊) and Baogen Shen(沈保根)

1School of Rare Earths,University of Science and Technology of China,Hefei 230026,China

2Ganjiang Innovation Academy,Chinese Academy of Sciences,Ganzhou 341000,China

3Key Laboratory of Cryogenics,Technical Institute of Physics and Chemistry,Chinese Academy of Sciences,Beijing 100190,China

Keywords: TmCuAl-based alloy,magnetic property,magnetocaloric effect,magnetic refrigeration

1. Introduction

2. Experimental details

The polycrystalline Tm1−xErxCuAl (x=0.25, 0.5, and 0.75)alloys were fabricated by arc-melting the constituent elements (Tm and Er: purity≥99.9 wt%; Cu and Al: purity≥99.99 wt%) in an appropriate stoichiometric ratio under a high-purity argon atmosphere. To ensure homogeneity of components,the ingots were flipped and re-melted three times in the course of the melting process. The resulting ingots were sealed in evacuated quartz tubes and heat-treated at 1173 K for seven days. Subsequently, a rapid cooling process was performed by quenching in liquid nitrogen.The structure of these samples at room temperature was characterized by a Bruker D8A A25 x-ray diffractometer (XRD) with the Bragg angles 2θin the range from 2◦to 90◦. Magnetic measurement was carried out by a commercial physical properties measurement system(PPMS).

3. Results and discussion

The x-ray powder diffraction patterns and Rietveld refinements for the Tm1−xErxCuAl (x=0.25, 0.5, and 0.75) compounds at room temperature are shown in Figs.1(a)–1(c). All compounds crystallize in a hexagonal ZrNiAl-type structure(space groupP¯62m) with no impurity phase. The lattice parametersa(c)are 6.951(3.995) ˚A,6.957(3.998) ˚A,and 6.963(4.002) ˚A for the compounds ofx=0.25, 0.5, and 0.75, exhibiting a monotonous increase with the increasing Er concentration,which is attributed to the larger atom radium of Er than Tm. The crystal structure ofReCuAl(Re=Er or Tm)is plotted in Fig. 1(d). The crystal structure ofReCuAl consists of two types of layers,which are alternately stacked along thecaxis direction. One consists of all rare-earth atoms distributed in triangle and one third of Cu atoms. The other contains all Al atoms and the other Cu atoms. It can be inferred from the structure that this series of compounds may have strong magnetocrystalline anisotropy.

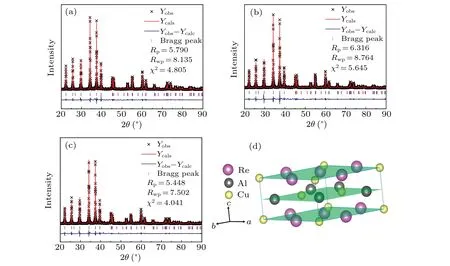
Fig.1. The x-ray powder diffraction patterns and Rietveld refinements at room temperature for(a)Tm0.75Er0.25CuAl,(b)Tm0.5Er0.5CuAl,and(c)Tm0.25Er0.75CuAl,and(d)the crystal structure of ReCuAl(Re=Er or Tm)compound.

Figures 2(a)–2(c) displays the temperature (T) dependence of magnetization(M)under the condition of zero-fieldcooling(ZFC)and field-cooling(FC)for Tm1−xErxCuAl(x=0.25, 0.5, and 0.75) compounds forµ0H= 0.01 T. These compounds show a typical ferromagnetic (FM) characteristic. A significant splitting between ZFC and FC curves is observed for these compounds at low temperature, which may be attributed to the domain-wall pining effect as usually observed in materials with low ordering temperature and high anisotropy.[24,25]Based on the ZFC curves, the dM/dT–Tcurves are obtained as shown in the insets. TheTCvalues of the compounds withx=0.25,0.5,and 0.75 are determined to be 3.2 K,5 K,and 6 K,respectively,which correspond to the minimum values of the dM/dT–Tcurves.

Fig. 2. Temperature dependence of magnetization measured in zero-field-cooling (ZFC) and field-cooling (FC) together with (inset) their dM/dT versus T for (a) Tm0.75Er0.25CuAl, (b) Tm0.5Er0.5CuAl, and (c) Tm0.25Er0.75CuAl under µ0H of 0.01 T. (d) The inverse magnetic susceptibility versus temperature(symbols)and linear fitting according to the Curie–Weiss law(line)for Tm1−xErxCuAl(x=0.25, 0.5, and 0.75)underµ0H of 0.01 T.

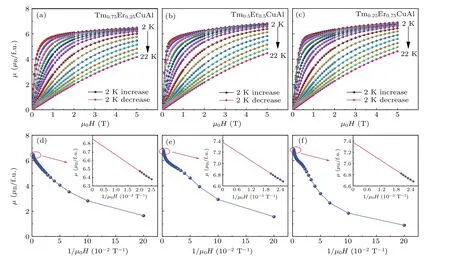
Fig.3. (a)–(c)Isothermal magnetization curves and(d)–(f)the 1/H dependence of magnetization of Tm1−xErxCuAl(x=0.25,0.5,and 0.75).The insets in(d)–(f)show theµ–1/H curves above the field of 4 T and their linear fitting curves.

Fig.4. The temperature dependences of −∆SM for(a)Tm0.75Er0.25CuAl, (b)Tm0.5Er0.5CuAl, and(c)Tm0.25Er0.75CuAl, under µ0∆H from 0–1 T to 0–5 T,and(d)the −∆SmaxM and RC for x=0.25,0.5,and 0.75 underµ0∆H of 0–2 T.

Figures 3(a)–3(c) present the isothermal magnetization curves of Tm1−xErxCuAl (x=0.25, 0.5, and 0.75) measured in a temperature region from 2 to 26 K under the applied field up to 5 T. AtT=2 K, the magnetization curves measured in increasing and decreasing field modes overlap completely,indicating the absence of magnetic hysteresis in these compounds. Moreover,it is observed that the magnetization of the three compounds increases rapidly with increasing magnetic field at low fields belowTC,which is a typical FM feature. As the magnetic field increases further, the magnetization starts to increase slowly and does not reach saturation until 5 T.The saturation magnetic moment(µs)can be evaluated by converting theµ–Hcurve measured at 2 K to theµ–1/Hcurve and then extrapolating linearly theµ–1/Hcurve in high field region toy-axis.[28]Figures 3(d)–3(f)display theµ–1/Hcurves for compounds withx=0.25,0.5,and 0.75,where part of theµ–1/Hcurves above the field of 4 T are selected(blue dot and line)and linearly fitted(red line)as shown in the insets. The values ofµsare 6.85µB/f.u., 7.37µB/f.u., and 7.47µB/f.u.,forx= 0.25, 0.5, and 0.75, respectively, which are significantly lower than theirµeffvalues obtained by the Curie–Weiss law.Their differences are 0.75µB/f.u.,0.63µB/f.u.,and 0.93µB/f.u.,respectively.This phenomenon may be attributed to the different angles between each magnetic moment ofReelements and thec-axis. Although the vector sum of the magnetic moments of Tm and Er in parent-alloy is aligned parallel along thec-axis, every magnetic moment of Tm and Er has an angle with thec-axis and the angle of Tm is smaller than Er. Meanwhile, the distortion of lattice is severest forx=0.5, which causes greater internal stress that may bring about a larger magnetic entropy change. It is similar to the mechanism of regulating magnetic interaction through hydrostatic pressure.[29]

The rebuilt ∆S′–θcurves of Tm1−xErxCuAl (x= 0.25,0.5,and 0.75)are given in Fig.5.For compounds withx=0.5,and 0.75, the ∆S′–θcurves atµ0∆Hof 0–1 T do not completely overlap with the other curves forθ<0,which is caused by taking too few points atθ<0.The rest of the ∆S′–θcurves almost collapse on the same universal curve at different magnetic fields,proving their second-order phase transition nature.

Fig.5. Normalized magnetic entropy change as a function of the rescaled temperature for(a)Tm0.75Er0.25CuAl,(b)Tm0.5Er0.5CuAl,and(c)Tm0.25Er0.75CuAl,underµ0∆H from 0–1 T to 0–5 T.

4. Conclusion

Acknowledgements

Project supported by the National Natural Science Foundation of China (Grant Nos. 52171195, and 52171054), the National Science Foundation for Distinguished Young Scholars (Grant No. 51925605), and the Scientific Instrument Developing Project of Chinese Academy of Sciences (Grant No.YJKYYQ20200042).