Simulation analysis of significance and interaction of influencing factors on mixing uniformity of double-drum recycling mixing plant

Ma Dengcheng Gui Xue Li Xuan Ma Chenglin

(1 National Engineering Laboratory for Road Maintenance Equipment, Chang’an University, Xi’an,710064, China)(2 Shaanxi Zhonglin Asphalt Pavement Maintenance Technology Co., Ltd., Xi’an 710000, China)

Abstract：To examine the influence of the structural parameters and working parameters of a double-drum regeneration mixing station on its mixing uniformity, the influence of the discrete element method and response surface method on the uniformity of the aggregate mixing when the interaction between two different factors was analyzed.A mathematical model of the influence of various factors and interactions on the coefficient of variation of the aggregates was established.The matching of each parameter was optimized with the goal of minimizing the coefficient of variation.The results show that when the aggregate particle size is different, the significance of each parameter affecting its mixing uniformity is also different.Moreover, increasing the speed and reducing the axial installation angle of the blade can reduce the coefficient of variation of the three aggregates.To obtain a good mixing uniformity, the mixing-arm phase angle when the drum inclination angle is large should be smaller than the phase angle when the drum inclination angle is small, and the mixing of large particles should not be arranged with a large mixing-arm phase angle.With a blade radial installation angle of 38°, a blade axial installation angle of 35°, a drum inclination angle of 6°, a drum rotation speed of 10 r/min, and a mixing-arm phase angle of 32°, the aggregate as a whole can exhibit the best mixing uniformity.

Key words：double-drum recycling mixing station; mixing uniformity; response surface method; discrete element method; significance and interaction analysis

The structural and working parameters of a double drum have an important impact on the mixing uniformity of a recycled asphalt mixture.Accordingly, many scholars have conducted considerable research on this topic.Ma et al.[1]analyzed the influence of the single factor of structure parameter and working parameter on the mixing uniformity.Liu et al.[2]analyzed the inclination installation range of double-drum blades.Zhao et al.[3]matched the blade parameters of an asphalt mixer based on the discrete element method(DEM).Simons et al.[4]studied the characterization of granular mixing in a helical ribbon blade blender.Sakai et al.[5]evaluated the uniformity of solid mixing in an industrial blender based on discrete element simulation.Milstead[6]improved the shape of agitation blades on the outer wall of an inner cylinder to improve the uniformity of a mixture.Larry et al.[7]proposed a mixing chain device that can reduce the adhesion of an asphalt mixture on the outer surface of an inner cylinder to prevent the adhered aging materials from cracking and falling into a mixing chamber and hence contaminating the mixture.Wallevik et al.[8]analyzed shear rates within a concrete truck mixer.In addition, scholars have conducted relevant research on the mechanical properties, production processes, and structural characteristics of double-drum regeneration equipment[9-13].Other studies on the structural parameters of mixed equipment using the DEM have also been conducted[14-17].

The above-mentioned studies focused on the optimization and improvement of the performance of double-drum mixing plants.The limited literature on the effect of structural parameters and working parameters on the mixing uniformity only covered the influence of a single parameter.In the actual mixing process, the uniformity of mixing is affected by the interaction of parameters, such as drum rotation speed, drum inclination angle, blade axial installation angle, blade radial installation angle, and mixing-arm phase angle.

Therefore, based on the DEM, a simulation model with actual working conditions was established in this study.By analyzing the influence of every single factor on the uniformity of aggregate mixing, the response surface method(RSM)was used to establish a mathematical model between the discrete coefficient of aggregates and the factors.Then, the significant relationship between each factor and its interaction on the uniformity of the aggregate was analyzed.This study provides a reference for the further understanding of the mixing mechanism and reveals the movement law of particles on mixing blades.

1 Main Influencing Parameters and Value Range

A double drum, as shown in Fig.1, is the core component of a double-drum recycling mixing plant.

Fig.1 Structure diagram of a double drum

It consists of an inner cylinder and a number of outer cylinders, and the outer wall of the inner cylinder is equipped with mixing blades.New aggregates enter the inner cylinder from the feed port after proportioning and move toward the burner with the rotation of the inner cylinder.Then, the heated aggregate falls into the outer cylinder.Recycled materials, new asphalt, and powder were heated and stirred in the outer cylinder of the recycled mixture, which realizes the recycling of old recycled materials.The mixing uniformity and mixing efficiency of the asphalt mixture have many influencing factors.In addition to the characteristics of the mixture itself, the other main factors are the axial installation angle of the blade(β), blade radial installation angle(α), mixing-arm phase angle(φ), drum inclination angle(γ), drum rotation speed(n), and their influence on each other.

According to the normal production requirements of the double-drum recycling mixing plant and Ref.[1], the parameters’ value ranges are as follows:α=31.54°～39.36°,β=33.54°～45.36°,φ=20°～60°,γ=2°～6°, andn=6～10 r/min.

2 Simulation Analysis

2.1 DEM contact model

The simulation model was established based on the DEM.The contact relationship of the DEM generally includes particle to particle and particle to geometry, as shown in Fig.2.The displacement change of particles in contact is shown in Fig.3.For example, particleiis in contact with particlejat pointC.The dotted line is the initial contact position;ais the tangent overlap; andδis the normal overlap.The vibration equation can be used to express the contact problem of the particle model in the DEM.The contact relationship of particles is represented by the vibration model, as shown in Fig.4.

Fig.2 Particle contact diagram

Fig.3 Particle contact displacement

(a)

The vibration motions of particles are disintegrated into the normal and tangential directions in the process of contact.The vibration equation is

(1)

wherem1,2is the equivalent mass of particles;I1,2is the equivalent moment of inertia;unis the normal relative displacement;usis the tangential one;θdenotes the rotational angle;Fnis the normal components of particles acted on by the outside force;Fsis the tangential one;Mis the external moment of particles;Knis the normal elastic coefficient in the contact model;Ksis the tangential one;cnis the normal damping coefficient;csis the tangential one.

The friction force between contacting particles has an effect on the tangential sliding and rolling of particles.Through the sliding model, the limiting conditions of tangential sliding and rolling can be obtained by

(2)

whereμis the friction coefficient.

2.2 Simulation parameter settings

The simulation model of the double-drum regeneration mixing equipment was established by the EDEM software.To improve the simulation speed, the aggregate in the mixture was simplified as a single spherical particle model.Three kinds of aggregate with different particle size ranges were used to simulate the mixture.The particle size ranges of the aggregates A1, A2, and A3 were 2.36 to 4.75, 4.75 to 9.50, and 9.50 to 19.00 mm, respectively.Based on the production efficiency and gradation, the production rates of the particle factory, aggregate A1, and aggregate A2 are 1.51, 14.73, and 20.21 kg/s, respectively.

The complex contact forces between the asphalt and mineral aggregate, the mineral aggregate and mineral aggregate covered with asphalt, and the mineral aggregate and mixing plant include the intermolecular van der Waals force, acid-base force based on the chemical reaction, and meshing friction force.To simulate the adhesion of asphalt, Hertz Mindlin with Johnson-Kendall-Roberts cohesion contact model in the EDEM software was selected.The intrinsic parameters and contact parameters of the mixing device and material particles are set according to the guidelines in the literature[1].

3 Influencing Factor Analysis

3.1 Effect of n on the mixing uniformity

In this study,β=40°,α=36°,φ=40°,γ=4°, andn=6～10 r/min.After the material flow became stable, the distribution data of the three kinds of aggregate at the outlet were extracted.The relationship betweenCv(the coefficient of variation of the aggregate)andnis shown in Fig.5.

Fig.5 Relationship between Cv and n

As shown in Fig.5,nhas a great influence on the mixing uniformity of the mixture.Whennincreases from 6 to 9 r/min,Cvof the three kinds of aggregate decreases, andCvof A1 and A3 decrease.Because A1 and A3 were relatively fine and coarse particles and the number of particles was relatively small, segregation could be easily produced.Whennis low, the distribution of particles is less disturbed.Withnincreases, the aggregate throwing increases correspondingly, the large-scale cross movement among particles becomes more intense, and the renewal frequency of the particle position increases.Because the number of particles in A2 is relatively large and the particle size is moderate, its particle distribution has a strong anti-interference ability and a small fluctuation range of the dispersion coefficient.Whennchanges from 9 to 10 r/min, the decrease range of theCvof A1 and A3 becomes smaller, and theCvof A2 slightly increases; that is, the influence ofnon the uniformity of the aggregate is nonlinear.Whennreaches a certain value, the mixture distribution tends to be stable, and the further increase ofnwill not have a great positive effect on the improvement of the mixing uniformity.

3.2 Effect of γ on the mixing uniformity

In this study,β=40°,α=36°,φ=40°,n=8 r/min, andγ=2°～6°.The relationship betweenCvandγis shown in Fig.6.

Fig.6 Relationship between Cv and γ

As shown in Fig.6, with the change inγ, theCvof A1 and A2 fluctuates less, and theCvof A3 relatively fluctuates more, but it is also limited within the range of 0.07.This result indicates that the change inγhas less influence on the uniformity of the mixture.Theoretically, increasingγcan prolong the retention time of the mixture in the mixing drum and increase the mixing times.However, as shown in Fig.6, the greater theγ, the greater the segregation of the mixture.

The feed rate of the particle factory was reduced by 30%, but other parameters remained unchanged.The uniformity of the mixture was analyzed when the inclination of the roller was 6°.TheCvof A1, A2, and A3 were 0.427, 0.184, and 0.683, respectively, which are lower than those before the feed rate was reduced.This finding shows that the filling ratio of the mixing chamber is high whenγis 6° without the decrease in the feeding rate.Therefore, increasingγcan prolong the mixing time of the mixture.However, the retained mixture can easily result in a large filling ratio in the mixing drum, which is not conducive to mixing.

Whenγis 4° andnis 9 r/min, theCvof the three aggregates is smaller than that whenγis 2°andnis 8 r/min.Therefore, to address the increase in the filling ratio of the mixing drum caused by the increase inγ,ncan be properly increased without reducing the productivity.

3.3 Effect of β on the mixing uniformity

In this study,α=36°,φ=40°,γ=4°,n=8 r/min, andβ=31°, 34°, 37°, 40°, 43°, 46°.The relationship between theCvof the aggregate andβis shown in Fig.7.

Fig.7 Relationship between Cv and β

As shown in Fig.7,βhas a great influence on the mixing uniformity.Whenβincreases from 34° to 46°, theCvof the three aggregates all increase.Whenβdecreases from 34° to 31°, theCvof A1 and A3 increase slightly.Overall, whenβ=34°, the uniformity of the mixture is the best.

βaffects the intensity of the axial and circumferential movements of the mixture, which in turn affects the mixing uniformity.The axial average velocity(Va)and circumferential average velocity(Vc)of all particles were collected at different values ofβto analyze the effect ofβon the motion speed of the aggregate, as shown in Fig.8.

Fig.8 Average velocity of particles with different β

As shown in Fig.8, whenβincreases from 31° to 46°, the average axial velocity of the particles increases first and then decreases, and the maximum value is obtained whenβ=40°.The average circumferential velocity of the particles in the mixture reaches the maximum value whenβ=34° and decreases whenβincreases.As shown in Figs.7 and 8, whenβ=34°, the circumferential movement of the mixture is sufficient, which is conducive to the mixing of aggregates; the axial movement speed of the mixture is slow, which is conducive to the extension of the mixing time.

The feed rate of the particle plant was reduced by 30%, and other parameters were unchanged.The uniformity of the mixture was analyzed whenβ=31°.The dispersion coefficients of A1, A2, and A3 were 0.363, 0.145, and 0.626, respectively, which are lower than those before the feed rate was reduced.This finding shows that the filling ratio of the mixing drum is high whenβ=31° without a decrease in the feeding rate.As shown in Fig.8, compared withβ=34°, whenβ=31°, the axial movement speed of the mixture is slower, and the filling ratio of the mixing drum is higher, which is not conducive to the cross movement between particles.The circumferential velocity of the mixture was decreased, and the particle throwing was weakened.Therefore, the axial installation angle should take into account the axial and circumferential movements of the mixture.

3.4 Effect of φ on the mixing uniformity

In this study,β=40°,α=36°,γ=4°, andn=8 r/min,φ=20°, 30°, 40°, 50°, 60°.The relationship between theCvof the aggregate andφis shown in Fig.9.

Fig.9 Relationship between Cv and φ

As shown in Fig.9, theCvof A1 changes slightly, and theCvof A2 and A3 vary greatly.Whenφ=30°,Cvof A2 and A3 are at the minimum.Whenφincreases from 30° to 60°,Cvof A2 and A3 increases gradually, butCvof A3 increases exponentially.This finding shows thatφhas a significant impact on the uniformity of large particles in the mixture.An excessively largeφis unfavorable to the mixing of the coarse aggregate.

The feed rate of the particle plant was reduced by 30%, whereas other parameters were unchanged.The uniformity of the mixture was analyzed whenφ=60°.TheCvof A1, A2, and A3 were 0.451, 0.316, and 1.038, respectively, which are lower than those before the feed rate was reduced.Hence, when the feed rate is constant andφis 60°, the filling ratio of the mixing drum is on the high side.

3.5 Effect of α on the mixing uniformity

β=40°,φ=40°,γ=4°,n=8 r/min, andα=32°, 34°, 36°, 38°, 40°.The relationship between theCvof the aggregate andαis shown in Fig.10.

Fig.10 Relationship between Cv and α

As shown in Fig.10, theCvof A1 and A2 has little change.TheCvof A3 is largely influenced byα, but its fluctuation range is also less than 0.11.Hence,αhas little influence on the uniformity of the mixture.

αcan separate the mixture from the inner wall of the outer cylinder, shorten the rolling distance, and reduce the segregation of the coarse particles in the rolling process.Therefore, the uniformity of A3 is greatly affected byα.Whenαis 36° and the other parameters remain unchanged, the uniformity of the aggregate is compared whenφis 60° and 40°.The results show that theCvof A1, A2, and A3 are 0.464, 0.363, and 1.298, respectively, whenφis 60°, which are higher than that whenφis 40°.

In addition,αwill affect the movement speed of the mixture particles.To study the influence ofαon the mixture moving speed, we collected the average speed of all particles and determined the average moving speed of particles with differentα, as shown in Fig.11.

Fig.11 Change in the average particle velocity with different α

As shown in Fig.11, the average velocity of particles has little change; that is,αhas little influence on the velocity of the mixture.Whenαis 36°, the average veloci-ty of particles in the mixture is relatively large, and the homogeneity of the mixture is relatively good.Whenαis 40°, the average velocity of particles is slow, which is not conducive to the mixing of the mixture.

4 RSM Analysis

To analyze the interaction between various factors and find the best parameter match, the RSM was used for further research.

4.1 Simulation scheme design

Referring to the design of the structural parameters and motion parameters of the double-drum mixing equipment in the previous paper, the value ranges of five factors are set as follows: 34°≤α≤46°,32°≤β≤40°,20°≤φ≤60°,2°≤γ≤6°, and 6 r/min≤n≤10 r/min.

Takingα,β,φ,γ, andnas the influencing factors andCvas the response value, according to the design method of the response surface experiment, the simulation scheme of the five factors and three levels was designed, as shown in Tab.1.

Tab.1 Influencing factors and level value

4.2 Simulation results and analysis

According to the range of the influencing factors in Tab.1, the simulation scheme and results were obtained by the Box-Behnken design test method.The simulation results are analyzed by RSM.Each factor of aggregates is

(3)

whereCv1，Cv2，Cv3are the coefficients of variation of A1, A2, and A3, respectively.The significant factors and the significant relationship ofCv1aren>β>φ.In the interaction term,φandγ,φandβhave a significant influence.The significant factors and the significant relationship ofCv2aren>β>γ>φ, and among the interaction terms,φandγ,φandα,φandβhave significant influences.The significant factors and significant relationship ofCv3areφ>n>β>γ, and among the interaction terms,φandγ,φandn,φandαhave significant effects.

4.3 Interaction factor analysis

Among the interaction factors, the interactions ofγandφ,βandφhave a significant effect onCv1.The response surface relationship betweenCv1and the significant interaction factor of A1 is shown in Fig.12.

The response surface relationship between the discrete coefficient and interaction factor is shown in Fig.12(a).When the phase angle of the mixing arm is 20°,Cv1decreases whenγincreases.Whenφis 60°,Cv1increases whenγincreases.As shown in Fig.12(b), ifφis any value,Cv1will decrease whenβdecreases.Among them, whenφis 60°,Cv1decreases faster.

(a)

Among the interaction factors, the interactions ofφandβ,αandφ,γandφhave a significant effect onCv2.The response surface relationship betweenCv2and the significant interaction factor of A2 is shown in Fig.13.

As shown in Fig.13, the interaction betweenφandα，βhas a similar effect on the uniformity of A2.Whenφis constant, the change inα，βhas little influence onCv1.In particular, whenφis 60°, the effect is relatively obvious.With changes inφ,γhas a great influence on the uniformity of A2.Whenφis 20°,Cv2decreases whenγincreases.Whenφis 60°,Cv2increases whenγincreases.

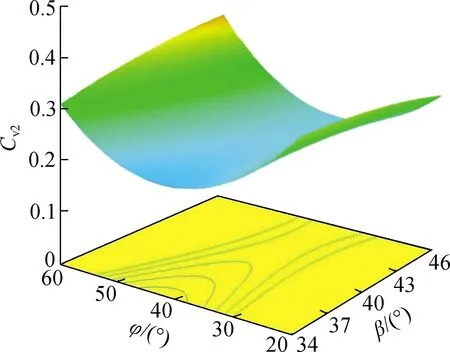
(a)

Among the interaction factors, the interactions ofβandφ,γandφ,nandφhave a significant effect onCv3.The response surface relationship betweenCv3and the significant interaction factors of A3 is shown in Fig.14.

As shown in Fig.14, whenφis 20°,β,γ, andnhave little influence onCv3.Whenφis 60°,Cv3increases whenβincreases, decreases whenγdecreases, and increases whenndecreases.Therefore, whenφis large,n,γ, andβhave an obvious influence on the uniformity of A3.As shown in Fig.14(c), whennis 10 r/min,Cv3decreases first and then increases whenφincreases, and the change range is small.Whennis 6 r/min,Cv3greatly increases whenφincreases.The results show that whenφis large andnis reduced, the segregation of A3 easily increases.

(a)

As shown in Figs.12(a), 13(c), and 14(b), the interaction betweenγandφhas a significant effect on theCvof the three kinds of aggregates.To improve the mixing uniformity, whenγis large,φshould be smaller thanγ.Whenγis constant,φcorresponding to the low point of the response surface of theCvof the aggregate decreases in turn; that is, a small value ofφshould be arranged for the mixing of large particles.

Taking the minimum value ofCvas the optimization objective, the following values of each parameter are obtained:β=35°,α=38°,φ=32°,γ=6°, andn=10 r/min.

5 Conclusions

1)Increasing the speed and reducing the axial installation angle of the blade within the design range can reduce the discrete coefficient of the aggregates.

2)In the case of a certain feeding rate, increasing the phase angle alone can easily cause the mixing lag of the mixture, so the filling rate of the mixing chamber is increased.

3)To obtain a good mixing uniformity, whenγis large,φshould be a small value, and conversely, a large value should be taken.φshould be set to a large value when mixing large particles.

4)Through the RSM, the significant relationship between the influence of every single factor and its interaction on theCvof the aggregates is obtained.Taking the minimum value ofCvas the goal, the best parameter matching is as follows:α=38°,β=35°,γ=6°,n=10 r/min, andφ=32°.