Numerical Simulation of Reiner-Rivlin Nanofluid Flow under the Influence of Thermal Radiation and Activation Energy over a Rotating Disk

Arfan Shahzad,Muhammad Imran,Muhammad Nawaz Naeem and Mohsan Raza

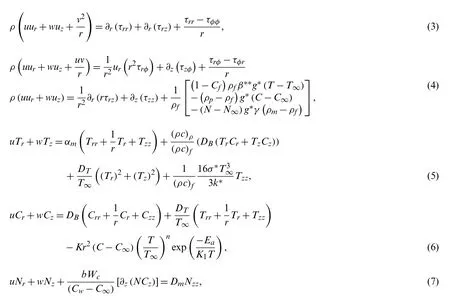
1Department of Mathematics,Government College University Faisalabad,Faisalabad,38000,Pakistan

2Department of Sciences &Humanities,National University of Computer and Emerging Sciences,Chiniot-Faisalabad Campus,Faisalabad,38000,Pakistan

ABSTRACT In current study,the numerical computations of Reiner–Rivlin nanofluid flow through a rotational disk under the influence of thermal radiation and Arrhenius activation energy is considered.For innovative physical situations,the motile microorganisms are incorporated too.The multiple slip effects are considered in the boundary conditions.The bioconvection of motile microorganism is utilized alongside nanofluids to provide stability to enhanced thermal transportation.The Bioconvection pattern in various nanoparticles accredits novel applications of biotechnology like the synthesis of biological polymers,biosensors,fuel cells,petroleum engineering,and the natural environment.By deploying some suitable similarity transformation functions,the governing partial differential equations(PDEs)of the flow problem are rehabilitated into dimensionless forms.The accomplished ordinary differential equations(ODEs)are solved numerically through the bvp4c scheme via a built-in function in computational MATLAB software.The upshots of some prominent physical and bioconvection parameters including wall slip parameters,thermophoresis parameter,Brownian motion parameter,Reiner–Revlin nanofluid parameter,Prandtl number,Peclet number,Lewis number,bioconvection Lewis number,and the mixed convection parameter against velocity,temperature,nanoparticles concentration,and density of motile microorganism profiles are dichotomized and pondered through graphs and tables.The presented computations show that the velocity profiles are de-escalated by the wall slip parameters while the thermal and solutal fields are upgraded with augmentation in thermophoresis number and wall slip parameters.The presence of thermal radiation enhances the temperature profile of nanofluid.The concentration profile of nanoparticles is boosted by intensification in activation energy.Furthermore,the increasing values of bioconvection Lewis number and Peclet number decay the motile microorganisms’field.

KEYWORDS Reiner–Rivlin nanofluid;bioconvection;motile swimming microorganisms;rotational disk;multiple slip conditions;bvp4c

Nomenclature

B*Mixed convection parameter

bChemotaxis constant

CConcentration of nanomaterials,molL－1

CfSkin friction coefficient

CwConcentration of nanomaterials at the surface

C∞Concentration of nanomaterials away from the surface

DMicroorganisms’diffusion coefficient,m2s－1

DBBrownian diffusion coefficient,m2s－1

DmMicroorganisms’diffusivity,m2s－1

DTThermophoresis diffusion coefficient,m2s－1

EActivation energy parameter

EaActivation energy,J

g*gravity

jmMotile microorganism flux

jwLocal mass flux,Kgm－2s－1

KrChemical reaction rate

kThermal conductivity,Wm－1K－1

k*Mean absorption coefficient

LbBioconvection Lewis number

LeLewis number

NDensity of microorganisms,m－3

NbBrownian diffusion parameter

NtThermophoresis number

NuLocal Nusselt number

NwMicroorganism concentration at the surface

N∞Microorganism concentration away from the surface

PePeclet number

PrPrandtl number

qwLocal heat flux,W

nPower Law index

RbBioconvection Rayleigh number

RcBuoyancy ratio parameter

RdThermal radiation parameter

rRadius of disk,m

ShLocal Sherwood number

SnMicroorganisms’density number

TTemperature of fluid,K

T0Reference temperature of fluid

TwTemperature of fluid at the surface

T∞Temperature of fluid away from the surface

u,v,wVelocity components,ms－1

WcMaximum cell swimming speed

Greek Symbols

λReiner–Rivlin fluid parameter

αmThermal diffusivity,m2s－1

β**Coefficient of thermal expansion

β1,β2,β3,β4,β5Wall slip parameters

γ1Thermal slip coefficient

γ2Concentration slip coefficient

γ3Microorganisms slip coefficient

δTemperature difference parameter

δ1Microorganism’s difference parameter

σ*Stefan–Boltzman constant

θDimensionless temperature

φDimensionless volume fraction of nanoparticles

χDimensionless microorganisms’density function

ζSimilarity variable

ρfDensity of fluid,kgm－3

ρmMicroorganism particles density

(ρc)pHeat capacity of nanoparticles,Jm－3K－1

(ρc)fHeat capacity of base fluid,Jm－3K－1

μDynamic viscosity of nanoliquid,Nsm－2

νKinematic viscosity of nanoliquid,m2s－1

ωAngular velocity

Subscript

w Condition at the wall

0 Ambient condition

f Fluid

∞ Condition at the free stream

1 Introduction

The flow of spinning disks is critical in both theoretical and practical considerations.In principle,such flows are among the fluid mechanics’problems that the Navier–Stokes equations may effectively solve.These flows are realistically based on the cases such as geophysics,microbiology,and other industrial implementations such as turbomachinery.Whether the vortex formed,or small eddy motion created automatically leads to the talk of rotating fluids.The flow of spinning disks is typically employed in many tracts viz.,nutriment processing,chemical reactors,designing turbines,engine gearing and brake components,disk cleaners,viscometry,medical gadgetry,typical rotating geometry of internal cooling,compressors,rotors,and cyclone separator.The study of the heat transfer phenomenon in the presence of thermal radiation has also achieved great attention by scientists in recent decades.The impact of thermal radiation at high temperatures cannot be denied as it encountered a variety of interesting applications in power generation,solar energy systems,nuclear industries,missiles technology,semiconductor wafers,etc.

Non-Newtonian fluid flows are found more in research and technology than Newtonian fluids.Scientists,biologists,and mathematicians worked tirelessly to explain and grasp the rheological characteristics of non-Newtonian fluids.These fluids have difficult nature of the operation and are complicated to apply in industries owing to their changeable composition.Therefore,this work discusses the bioconvective,steady,and incompressible flow of the Reiner–Rivlin nanofluid over a rotating disk.An empirical approach using a bvp4c method is proposed for the current study.Nanofluid is classified as nanometer-sized particles suspended in normal fluids with a diameter of less than 100 nm.Nanofluids have properties that make them potentially useful in many applications of heat transfer.They exhibit enhanced thermal conductivity and the convective heat transfer coefficient compared to the base fluid.Nanofluids are useful in a variety of science and industrial applications,including biomedicine,automotive cooling,transformer cooling,electrical device cooling,heat exchanger,the development of liquid displays,and nuclear power plants[1–5].Hafeez et al.[6] analyzed the stagnation point flow of Oldroyd-B fluid over a rotatory disk.Hayat et al.[7] studied the influence of magnetohydrodynamics (MHD) 3-dimensional flow of nanoliquids and thermal transport of convective boundary conditions.Ellahi et al.[8] investigated slip’s role in two-phase nanofluid flow.Alsaedi et al.[9] examined entropy production in MHD Eyring–Powell nanofluid flow because of viscous dissipation,nonlinear mixed convection,and Joule heating.Khan et al.[10] studied the effect of thermal and solutal transport behavior in the Maxwell flow of nanofluid on the solar radiative expansion surface.Gireesha et al.[11] created a numerical model of nonlinearly thermal radiation as well as chemical reactions on a Maxwell nanofluid layer.Heat and mass diffusion of Maxwell nanoparticles were combinedly studied by Khan et al.[12] as they traveled through a linearly stretching surface.Nadeem et al.[13]analyze numerically the heat transformation process for Maxwell nanofluids.Khan et al.[14]investigate the impact of nonlinear heat radiation with nanomaterials and Arrhenius activation energy on the flowing of a generalized 2nd-grade nanofluid.Alghamdi [15] investigated the erratic flow of carbon nanotubes-type fluids between two moveable discs.Ullah et al.[16] proposed a computational model of the Darcy–Forchheimer nanoliquids motion by a rotating disc with partial slip.Tabassum et al.[17] investigated the numerical behavior of non-Newtonian Reiner–Rivlin nanofluid partial slip and heat transportation basis on a spinning disc.Attempts [18–20]offer more detail on the Reiner–Rivlin fluid.

Bioconvection models (swimming of motile microorganisms) have a crucial role in various real life-fields including model construction,pharmaceutical manufacturing,hydrodynamics system,sedimentary waterways,micro-fluidics machines,biofuels,food processing,gas-bearing,hydrodynamics fabrication,microbial increased oil recovery,lubrication materials,polymer construction,etc.Microorganisms are used in medicines as antibiotics and help to create vaccines.Furthermore,there has been reported to be a great combination of nanoliquids and bioconvective processes.The bioconvection of motile microorganisms is utilized alongside nanofluids to provide stability to enhanced thermal transportation.Microorganism particles are used in a wide range of commercial and industrial product categories,such as ethanol,biodiesel,biofuels,microsystems,and bio-fertilizers.Ansari et al.[21] scrutinized the effects of motile swimming microorganisms and nanomaterials over the bioconvection magnetohydrodynamics (MHD) movement of Casson-type liquid at the nonlinearly stretched boundary.Rashad et al.[22] addressed the bio-convection process in nanoliquid flow by the circular cylinder.The mass movement of steady incompressible MHD liquid immediately the stagnation level with the deferral of microscopic particles under the stretchable surface is evaluated by Mamatha et al.[23].Iqbal et al.[24] discussed the effect of motile gyrotactic microorganisms absorbed in the nanoliquid is often by control of mass flux,bio-convection,as well as energy convection.Kasaragadda et al.[25] inspected the consequence of highly hydrophobic surfaces on the acceptance of nanoparticle-reinforced biomaterial structures.Waqas et al.[26] used both cylinder and plate to observe the bioconvective flow by considering Wu’s slip conditions.Amirsom et al.[27] focused on a bi-axial stretched layer for the 3-dimensional motion of gyrotactic motile microorganisms in bioconvection nanoliquids.Uddin et al.[28] studied the mathematical model of bio-nano-convection transition with blowing and multiple slips through the horizontal layer.Ferdows et al.[29] discovered the conceptual boundary layer flow of cylinder heat and mass transportation in the motile microorganism living in a viscous liquid.Khan et al.[30] examine bioconvection in two stretchable rotating disks utilizing entropy imitation.Li et al.[31] examined the behavior of a transformed second grade nanofluid with bioconvection properties in the presence of Wu’s slip.Muhammad et al.[32] used slip results from a wedge to discover the cause of bioconvection in Carreau nanofluid.More research on bioconvection is being conducted [33–35].Zhang et al.[36] examined the influence of thermal radiation on the stretching/shrinking of a disc in a bioconvective rate type nanofluid with Arrhenius activation energy.Waqas et al.[37] examined the passage of an Oldroyd-B fluid around a spinning disk containing swimming motile microorganisms.Naqvi et al.[38] scrutinized the bioconvection flow of a few stress fluids involving nanomaterials,magnetic fields,and gyrotactic microorganisms in rotating disks.

1.1 Scope of the Study

The heat transfer in electronic devices is the main challenge in the world due to shorter sizing.To fulfill this gap nanofluids with bioconvection are more useful due to the higher thermal conductivity of nanoparticles.Therefore,in this article,we explore the bioconvection analysis of Reiner–Rivlin nanofluid flow over a rotating disk.For innovative physical situations,the consequences of motile microorganisms along with multiple slip effects are also part of this study.The bioconvection of motile microorganisms is utilized alongside nanofluids to provide stability to enhanced thermal transportation.In literature,the structure of the proposed problem under the impacts of multiple slip constraints is not discussed yet so pertinent results are given importance to fill this gap.The effects of some asymmetrical controlling parameters including wall slip parameters,thermophoresis parameter,Brownian motion parameter,Reiner–Revlin fluid parameter,Prandtl number,Peclet number,Lewis number,bioconvection Lewis number,and the mixed convection parameter on the flow,concentration,density of motile microorganism,and thermal field are examined thoroughly.The numerical outcomes are obtained by using the bvp4c method in MATLAB software.The graphical trend of different controlling parameters besides subjective flow fields is discussed and elaborated.

2 Mathematical Modeling

Consider the three-dimensional,steady,and incompressible flow of Reiner–Rivlin nanofluid containing gyrotactic motile microorganisms over a rotating disk.The rotation of nanofluid is taken about thez—axis normal to the surface with angular velocityω,(u,v,w)the velocity components alongr,φ,z(see Fig.1).The flow formulation is developed by addressing the effects of thermal radiation to the energy Eq.(5) and Arrhenius activation energy to the nanoparticle’s concentration Eq.(6).It is presumed thatTw,Cw,NwandT∞,C∞,N∞are the temperature,concentration of nanoparticles,the density of motile microorganisms at the surface and far away from the surface respectively.The highly nonlinear equations of the flow problem are transformed into ordinary ones with suitable similarities.For this purpose,we employed the bvp4c scheme.The tensor of Reiner–Rivlin fluid is given by [17]:

Hereτij,p,μc,δijanddesignate the deformation rate tensor,pressure,and stress tensor and cross-viscosity coefficient,respectively.The governing flow equations are proposed by [17,37]:

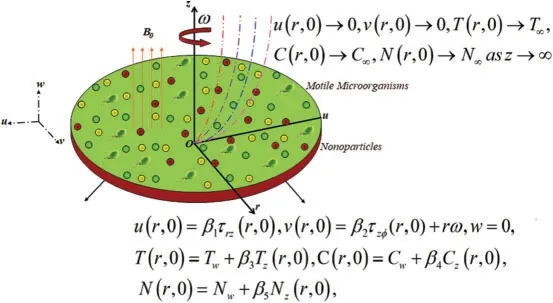
Figure 1:The geometrical shape of the problem

Furthermore,distortion rate tensor’s components are [17]:

Stress tensor components are assumed to be defined as [17]:

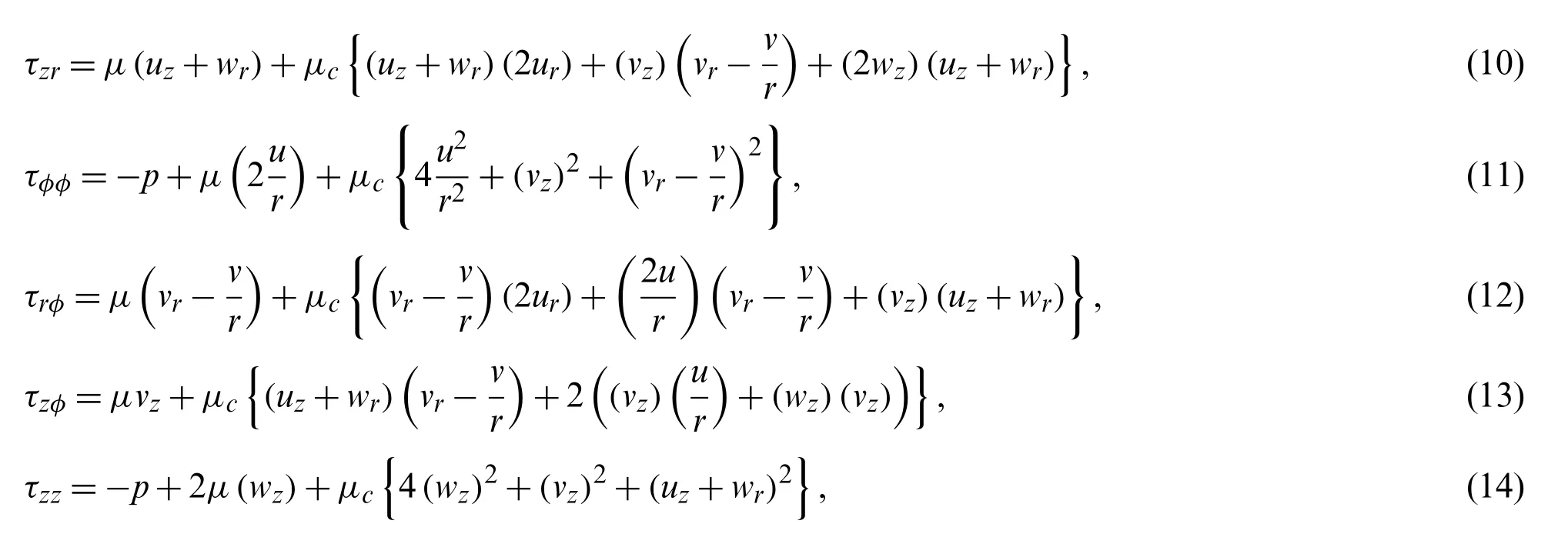
The physical boundary conditions are [17,37]:

whereTstands for the temperature of nanofluid,Cstands for the concentration of nanomaterials,Nstands for the concentration of motile microorganisms,αm=k/(ρc)ffor thermal diffusivity,(ρc)pfor the heat capacity of nanomaterials,(ρc)ffor base fluid heat capacity,ρsignifies the fluid density,kfor thermal conductivity,DTfor the coefficient of thermophoresis diffusion,DBfor Brownian diffusion coefficient,the term 16σ*T3∞/3k*in Eq.(5) depicts the thermal radiative heat transfer.The termin Eq.(6) represents the modified Arrhenius function whereEadenotes the activation energy andKris the chemical reaction rate,npower-law index,Dmthe mass diffusion coefficient,Wcthe maximum swimming speed of microorganism cell andβ1,β2are wall slip parameters.

The similarity transformations are [17,37]:

wheref (ζ)andg(ζ)are the non-dimensional stream functions,θ(ζ)is the non-dimensional temperature of nanofluid,φ(ζ)is the non-dimensional nanoparticles concentration function,χ(ζ)is the non-dimensional microorganisms density field,andζis the similarity transformation variable.

The following dimensionless ordinary differential equations are obtained by applying suitable similarity transformations [17,37]:

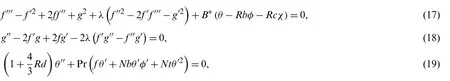
where the Reiner–Rivlin fluid parameter is andRc=for bioconvection Rayleigh number as well as buoyancy ratio parameter,respectively,Rd=4σT3∞/kk*thermal radiation parameter,/ωrfor mixed convection parameter,activation energy parameter read as E=Ea/kT∞,Le=(α1/DB)Lewis number,Prandtl number denoted bydenotes Brownian diffusion parameter,Nt=be the thermophoresis parameter,Lb=for bioconvection Lewis number,Peclet number and microorganisms differences parameter,respectively.

TorqueT0is specified by definite integral such as:

The engineering quantities of interest are local skin friction coefficientCf(explain the shear stress on the surface),local Nusselt numberNu(explain the rate of heat transfer),Sherwood numberSh(explain the rate of mass transfer),as well as the local density number of motile microorganismsSn(explain the motile microorganisms flux) are presented as:

whereqw,jwandjmdenotes shear stress,local heat flux,local mass flux,and motile microorganisms’flux on the surface,respectively.

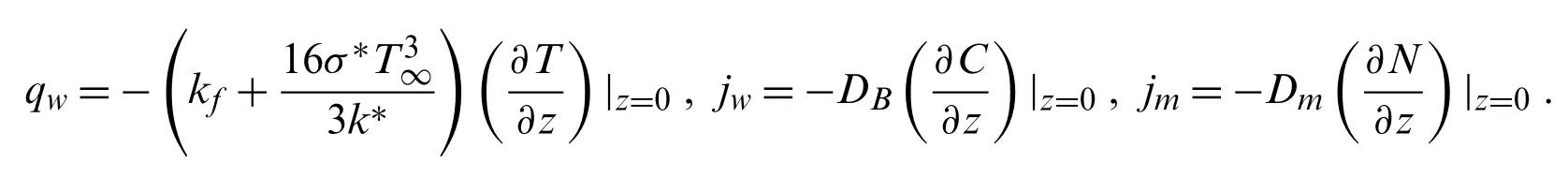
Thus from (24),we have dimensionless quantities as:

3 Numerical Scheme

The ordinary differential Eqs.(17)–(21) under the boundary conditions (22) are tackled by bvp4c method with the comfort of computing software MATLAB.The arrangement of nonlinear governing ordinary differential equations (ODEs) is restructured to the initial-order ordinary differential equation (ODEs).Let

with

4 Results and Discussion

The numerical solution of non-linear dimensionless ordinary differentiated structures(17)–(21) with boundary constraints given in expression (22) has been attained by the bvp4c function on MATLAB using a mathematical shooting scheme.The major focus of the current work is to observe the effect of several developing specific parameters against axial velocityf (ζ),radial velocityf′(ζ),azimuthal velocityg(ζ),the thermal fieldθ(ζ)of nanomaterials,the concentration fieldφ(ζ)of nanomaterials,and the motile microorganisms fieldχ(ζ).The outcomes of dimensionless profiles are analyzed briefly with the aid of graphs and tabular data.Figs.2–27 are delineated the effects of prominently involved parametersvs.flow fields.

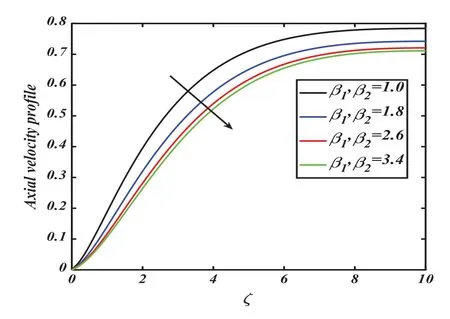
Figure 2:Axial velocity profile f for distinct values of wall slip parameters β1,β2

Figure 3:Radial velocity profile f′ for distinct values of wall slip parameters β1,β2

Figure 4:Azimuthal velocity profile g for distinct values of wall slip parameters β1,β2

Figure 5:Axial velocity profile f for distinct values of bioconvection Rayleigh number Rb

Fig.2 is depicted to see the impact of distinct estimations of wall slip parametersβ1,β2on the axial velocity profilefof Reiner–Rivlin nanofluid.It is manifest from the figure that increasing values of wall slip parameters diminishes the axial velocity profiles.Fig.3 is designed to analyze the inspiration of wall slip parametersβ1,β2vs.radial fluid velocityf′.From the figure,it is also witnessed that radial fluid velocity decreases by enhancing the estimation of wall slip parameters.The significance of wall slips parametersβ1,β2on azimuthal velocitygis elaborated through Fig.4.It is depicted that azimuthal velocity is depressed by enlarging wall slips parameters.Physically,wall slip occurs due to liquid-to-solid transitions under shear stress so crucially dependent on liquid-to-wall molecular interactions.

Figure 6:Radial velocity profile f′ for distinct values of bioconvection Rayleigh number Rb

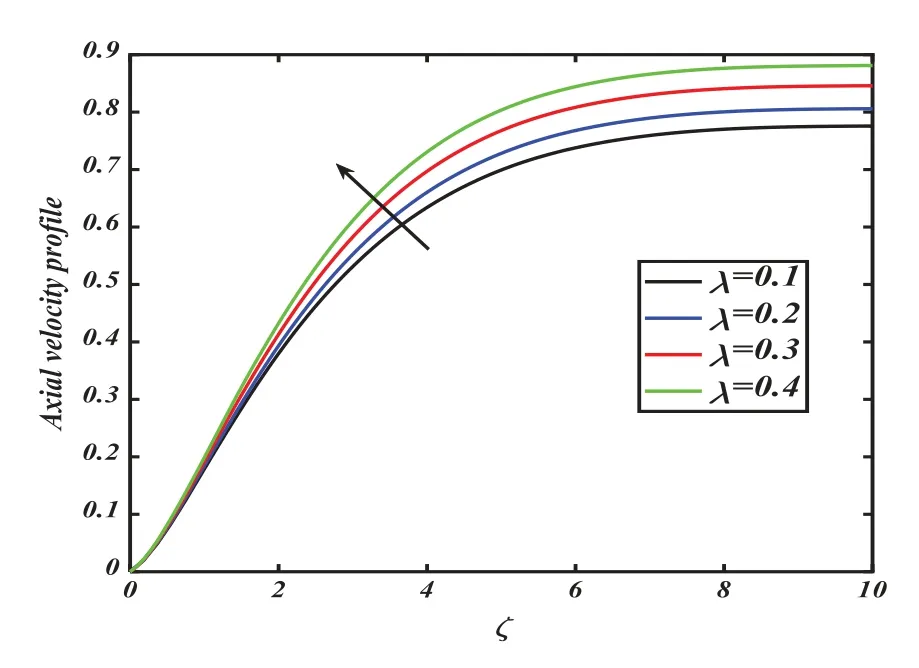
Figure 7:Axial velocity profile f for distinct values of Reiner–Revlin parameter λ

Figure 8:Radial velocity profile f′ for distinct values of Reiner–Revlin parameter λ

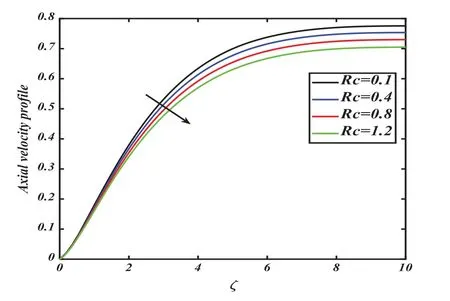
Figure 9:Axial velocity profile f for distinct values of buoyancy ratio parameter Rc

Figs.5 and 6 demonstrate the inspiration of bioconvection Rayleigh numberRbagainst the axial and radial velocitiesf,f′of the fluid.It is observed that declination in velocities occurs with augmenting magnitudes of bioconvection Rayleigh number.Physically,the bioconvection Rayleigh numberRbmeasures the instability between layers of fluid due to the variation of temperature and density from top to bottom.The significance of the Reiner–Rivlin fluid parameterλ vs.axial and radial velocitiesf,f′are provided in Figs.7 and 8.The rise in magnitudes of the Reiner–Rivlin fluid parameterλdiminishes both velocity fields of the fluid.Furthermore,radial velocity boosts up away from the disk.The variations of axial and radial velocitiesf,f′for distinct values of buoyancy ratio parameterRcand mixed convection parameterB*are presented in Figs.9–12.The velocities escalate with higher magnitudes of mixed convection parameter while an opposing trend has been detected forbuoyancy ratio parameter.As mixed convection parameter exemplifies ratio among buoyancy to viscous force and large value of this parameter conveys higher buoyancy force,while buoyancy ratio develops further progressive for largerRcwhich reduce velocities.

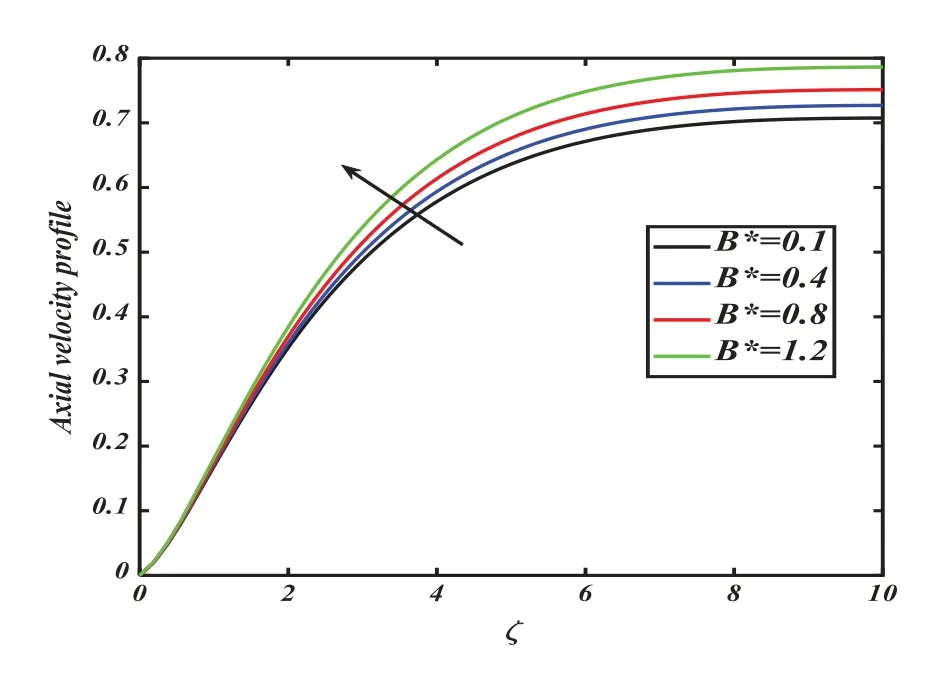
Figure 10:Axial velocity profile f for distinct values of mixed convection parameter B*

Figure 11:Radial velocity profile f′ for distinct values of mixed convection parameter B*

Figure 12:Radial velocity f′for distinct values of buoyancy ratio parameter Rc

Figure 13:Temperature profile θ for distinct values of Prandtl number Pr

The dependence of temperature profileθon relevant parameters is explained in Figs.13–17.Fig.13 demonstrates the outcomes of dimensionless temperature fieldθagainst a varying range of Prandtl numbers Pr.Here,the temperature of nanofluid and its thermal boundary layer thickness declines for enlarging magnitudes of Prandtl number.Physically,when the Prandtl number boosted,the thermal diffusivity de-escalated and consequently led to the decrement in the ability of energy,so the thermal boundary layer declined.Fig.14 discloses the stimulus of thermal slip coefficientγ1against a thermal field of species.The temperature field diminishes with rising numbers of thermal slip parameters.Fig.15 is interpreted as the influence of distinct estimations of wall slip parametersβ1,β2on the temperature profile of Reiner–Rivlin nanofluid.It is apparent from the figure that emerging values of wall slip parametersβ1,β2boosted the concentration of the temperature field.The upshots of thermophoresis parameterNt vs.temperature distributionθare demonstrated in Fig.16.Temperature distribution is increased by enlarging variation of thermophoresis parameter.Physically,this phenomenon occurs because the thermophoresis parameter raises the density of the thermal boundary layer,and suspended particles are transported from warmer to a cooler region which raises fluid temperature significantly.Therefore,temperature increases with an increase inNt.Fig.17 explaines the effects of thermal radiationRdon the thermal profileθof nanofluids.The thermal field goes up due to augmentation in the values of the thermal radiation parameter.Physically,larger radiation parameterRdleads to enhancement in the kinetic energy of fluid particles and hence increases the temperature profile of fluid.

Figure 14:Temperature profile θ for distinct values of thermal slip coefficient γ1

Figure 15:Temperature profile θ for distinct values of wall slip parameters β1,β2

Figure 16:Temperature profile θ for distinct values of thermophoresis parameter Nt

Figure 17:Temperature profile θ for distinct values of thermal radiation parameter Rd

Figs.18–23 reveal a dependence of concentration distributionφon the relevant parameters.Fig.18 decelerates the consequence of the Brownian motion parameterNbvia the concentration profileφof nanoparticles.It is inferred that the concentration of nanomaterials retarded down for larger estimations of the Brownian motion parameter.Physically,the greater Brownian motion results in an arbitrary movement of nanoparticles so the extra heat is produced.Due to this extra heat,the temperature of nanoparticles is also increased causes reduced nanoparticles concentration profile.Fig.19 delineates the characteristics of Lewis numberLeover concentration profile of species.Diminution in the concentration of Reiner–Rivlin nanofluid can be noticed for enlarging magnitudes of Lewis number.Physically,the Lewis number decreases the mass diffusivity and hence reduces the permeation depth of the boundary layer.Fig.20 discloses the consequences of activation energyEagainst the volumetric concentration of nanoparticles.The volumetric concentration of nanoparticles is boosted by the intensification in the values of activation energy.Activation energy,the minimum energy required to start a chemical reaction.So,the volumetric concentration profile increases asE.The properties of the wall slip parameter on the solutal field of species are demonstrated in Fig.21.Solutal profile decreases for larger magnitudes of concentration slip coefficientγ2.Fig.22 is deliberated to investigate the inspiration of wall slip parametersβ1,β2against the volumetric distribution of nanofluid.It is viewed that volumetric nanoparticle concentration enhancing parallel to the enlarging estimations of wall slip parametersβ1,β2.

Figure 18:Nanoparticle concentration profile φ for distinct values of Brownian diffusion parameter Nb

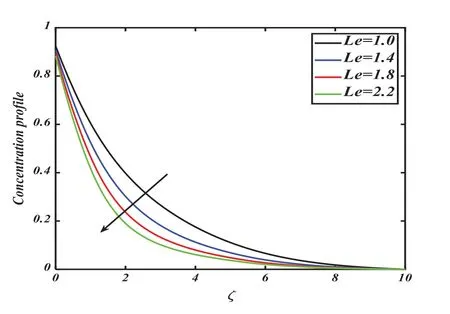
Figure 19:Nanoparticle concentration profile φ for distinct values of Lewis number Le

Figure 20:Nanoparticle concentration profile φ for distinct values of activation energy E

Figure 21:Nanoparticle concentration profile φ or distinct values of concentration slip coefficient γ2

Fig.23 is considered to study the upshot of thermophoresis parameterNton the solutal fieldφof nanomaterials.It is observed that the thermophoresis parameter strongly enhanced the concentration field of species.Physically,in the thermophoresis phenomenon,the tiny particles of fluid are pulled back from the warm to a cold area.Then the particles of the nanofluid move back from the heated surface and consequently nanoparticles’volume profile is enhanced.

Figure 22:Nanoparticle concentration profile φ for distinct values of wall slip parameters β1,β2

Figure 23:Nanoparticle concentration profile φ for distinct values of thermophoresis number Nt

Figure 24:Motile microorganism density profile χ for distinct values of bioconvection Lewis number Lb

Figure 25:Motile microorganism density profile χ for distinct values of Peclet number Pe

Figs.24–27 revealed the effectiveness of bioconvection Lewis numberLb,Peclet numberPe,microorganism slip coefficientγ3,and wall slip parametersβ1,β2over the swimming motile microorganism’s concentration fieldχ.Fig.24 is plotted to allocate the influence of bioconvection Lewis numberLbover the swimming motile microorganism’s concentration fieldχ.It is conceivable to note that the motile microorganisms’profileχdiminishes by positive variations of bioconvection Lewis numberLb.This happens,as the increasing valuesLbincrease the rate of viscous diffusion,and the density profile of motile microorganisms decreases in the boundary layer.Fig.25 is drawn to outline the significance of bioconvection Peclet numberPeof motile gyrotactic microorganism’s distributionχ.From the curves,it is promising to observe that the motile microorganisms’fieldχreduces when the Peclet number boosts.As bioconvection Peclet numberPe=increases,the cell swimming speedWcalso increases,and hence density profile of motile microorganisms decreases.Fig.26 exhibits the variation of microorganism slip coefficientγ3against motile microorganism’s distribution profileχ.From the graphs,it is perceived that the concentration of motile microorganism’s profile curve declined for distinct estimations of microorganism slip coefficient.Fig.27 reports the assortment of motile microorganisms’fieldsχfor evident estimations of wall slip parametersβ1,β2.Enhancement in the wall slip parameters boosted the density of the motile microorganism’s field.

Figs.28a–28c show the streamlines at different values of theβ1,β2.The contour of Nusselt number and Sherwood number against subjective parameters is shown in Figs.29a and 29b.From the figures we observed that the Shewood number and Nusselt number are enhanced via larger subjective parameters.The 3-D plot of Nusslet number viaRbandRcdrawn in Fig.30.Furthermore,the 3D plot of microorganism’s density number for different estimations of thePeandLbgiven in Fig.31.

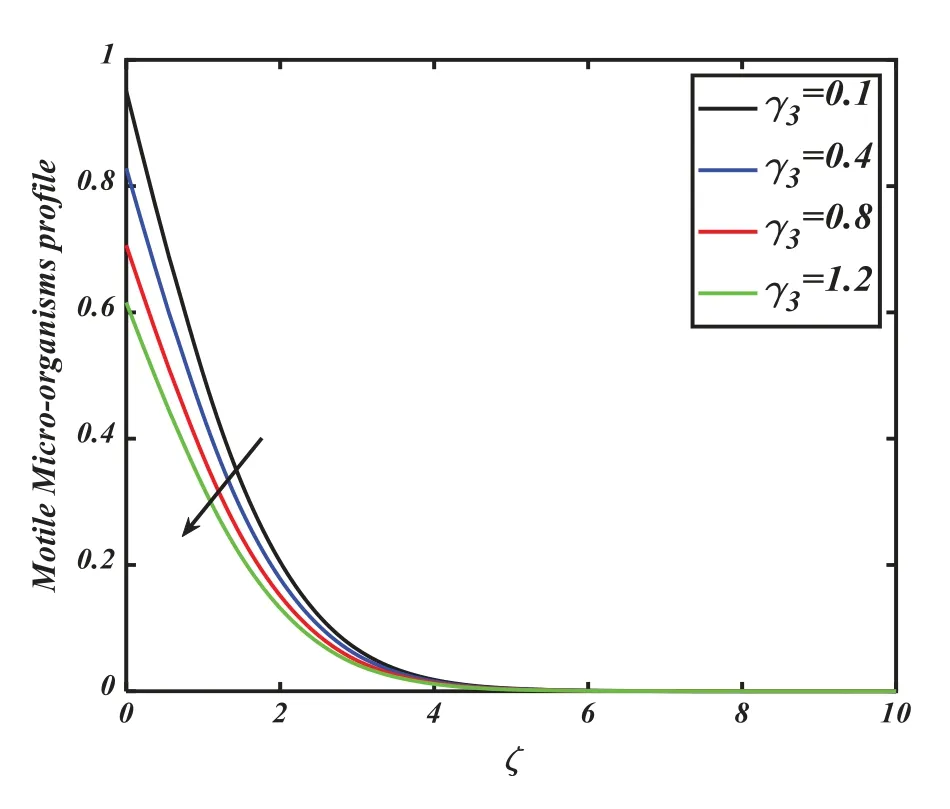
Figure 26:Motile microorganism density profile χ for distinct values of microorganisms slip coefficient γ3

Figure 27:Motile microorganism density profile χ for distinct values of wall slip parameters β1,β2

Tables 1–3 are pinched to estimate the variations of the local Nusselt number,the local Sherwood number,as well as the local density number of swimming microorganisms against various mentioned parameters.From Table 1,it can be noted that the local Nusselt number (heat transfer rate) decreases throughλand Pr.Furthermore,the local Sherwood number (mass transfer rate) declined for larger magnitudes of the parametersRb&Rc,and increases asγ2shown in Table 2.From Table 3,it is concluded that the local density number of microorganisms (motile microorganisms’flux) rises withPeandLb.Table 4 gives the comariosion of resuts between current study and results by Naqvi et al.[38].

Table 1:Tabulation of －θ′(0) with variations of Pr,λ,Nb,Nt,B*,Rc,Rb and γ1

Table 2:Tabulation of －φ′(0) with variations of Pr,Nb,Nt,λ,B*,Rc,Rb,Le and γ2

Table 2:(continued)Pr Nb Nt λ B* Rc Rb Le γ2 －φ′(0)0.1 0.2 0.2 2.0 1.5 0.1924 0.1910 0.1894 1.2 0.3 0.5 0.2 0.3 0.4 0.2 0.3 0.1 0.2 0.3 0.2 0.2 2.0 1.5 1.5 0.3503 0.3536 0.3567 0.2 1.5 0.3473 0.3404 0.3359 1.2 0.2 0.3 0.5 0.1 0.2 0.2 0.6 1.2 1.2 0.2 0.3 0.5 0.1 0.2 0.6 1.2 2.0 1.5 0.2445 0.2425 0.2287 1.2 0.2 0.3 0.5 0.1 0.2 0.2 1.0 1.6 2.2 1.5 0.3462 0.4464 0.5193 1.2 0.2 0.3 0.5 0.1 0.2 0.2 2.0 0.2 1.0 3.0 0.3461 0.3463 0.3466

Table 3:Tabulation of －χ′(0) with variations of λ,B*,Rc,Rb,Pe,Lb and γ3

Table 4:Validations of β1,β2 and K

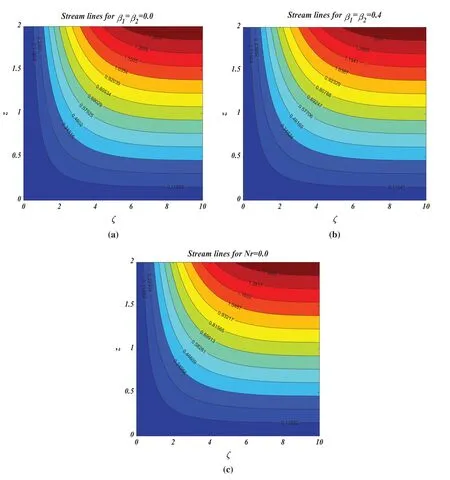
Figure 28:(a–c) Aspects of streamline Nr and β1=β2

Figure 29:(a and b) Aspects of Contour line Sherwood and Nusselt number

Figure 30:3D plot of Rb & Rc on Nusselt number

Figure 31:3D plot of Lb&Pe on Microorganism’s profile

5 Conclusions

In this manuscript,we have discussed the steady and incompressible bioconvection flow of Reiner–Rivlin nanofluid over a rough rotational disk containing motile microorganisms with thermal radiation and Arrhenius activation energy.The effects of some asymmetrical controlling parameters including wall slip parameters,thermophoresis parameter,Brownian motion parameter,Reiner–Revlin nanofluid parameter,Prandtl number,Peclet number,Lewis number,bioconvection Lewis number,and the mixed convection parameter on the flow,the concentration of nanoparticles,thermal field,and density of motile microorganisms are examined thoroughly.

· The velocity profiles of nanofluid escalate for higher magnitudes of mixed convection parameterB*while an opposing trend has been detected for buoyancy ratio parameterRc,Reiner–Rivlin fluid parameterλ,wall slip parametersβ1&β2,and bioconvection Rayleigh numberRb.

· The temperature profile of nanofluid goes up due to augmentation in the values of thermal radiation parameterRd,thermophoresis numberNt,and wall slip parametersβ1&β2while an opposing trend has been noticed for enlarging magnitudes of Prandtl number Pr.

· The concentration profile is boosted up by the thermophoresis numberNtand wall slip parametersβ1&β2.

· The concentration profile of nanoparticles grows by intensification in activation energyE.

· Brownian motionNband Lewis numberLeconsequences show a decrement in the concentration profile of nanoparticles.

· The motile microorganisms’profile diminishes by positive variations of bioconvection Lewis numberLband Peclet numberPewhile escalating for wall slip parametersβ1&β2.

· The temperature,concentration,and microorganisms’density profiles decrease for enlarging values of thermal,concentration,and microorganisms slip coefficientsγ1,γ2&γ3,respectively.

Funding Statement: This work is financially supported by the Government College University,Faisalabad,and Higher Education Commission,Pakistan.

Conflicts of Interest: The authors declare that they have no conflicts of interest to report regarding the present study.