Properties of Certain Subclasses of Analytic Functions Involving q-Poisson Distribution

Bilal Khan,Zhi-Guo Liu,Nazar Khan,Aftab Hussain,Nasir Khan and Muhammad Tahir

1School of Mathematical Sciences and Shanghai Key Laboratory of PMMP,East China Normal University,Shanghai,200241,China

2Department of Mathematics,Abbottabad University of Science and Technology,Abbottabad,22010,Pakistan

3Department of Mathematics,King Abdulaziz University,Jeddah,21589,Saudi Arabia

4Department of Mathematics,FATA University,Akhorwal(Darra Adam Khel),FR Kohat,26000,Pakistan

ABSTRACT By using the basic (or q)-Calculus many subclasses of analytic and univalent functions have been generalized and studied from different viewpoints and perspectives.In this paper,we aim to define certain new subclasses of an analytic function.We then give necessary and sufficient conditions for each of the defined function classes.We also study necessary and sufficient conditions for a function whose coefficients are probabilities of q-Poisson distribution.To validate our results,some known consequences are also given in the form of Remarks and Corollaries.

KEYWORDS Analytic function; q-difference operator;sufficient condition; q-Poisson distribution

1 Introduction,Basic Definitions and Motivation

A functionfis said to be in the classAif the following normalization condition:

f (0)=0 andf′(0)=1

in the open unit disk:

U={z:z∈C and |z|＜1}

is satisfied and having series representation:

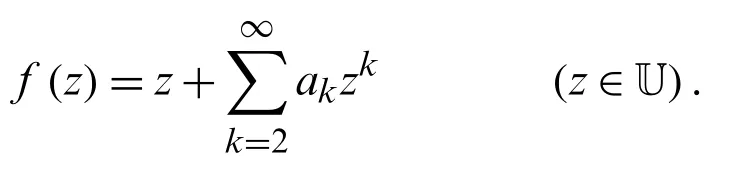
Note that the notation C denote the set of all complex numbers.Also,let all those functions which are univalent inSare placed in functions classA.

Furthermore,let us consider a subclassTof the function classS,having the following series representation:

Definition 1.1.[1] A functionfis said to be in the classF(μ,ρ),if it satisfy the following inequality:

Definition 1.2.[1] A functionfis said to be in the classH (μ,ρ),if it satisfy the following inequality:

It should be noted that forμ=0,we have

F(0,ρ)=F(ρ)andH (0,ρ)=H (ρ)(0 ≤ρ＜1),

whereF(ρ)andH (ρ)are the functions classes which were systematically studied by Silverman [2].

For a parameterψ,if the values 0,1,2,3,··· with probabilitiesare taken by a variable sayN,then this variableNis said to have Poisson distribution.Thus

The Poisson distribution is a statistical distribution that calculates the probability of a certain number of events occurring in a particular time period.The Poisson distribution is commonly used to model rate of random events that occur (arrive) in some fixed time interval.The Poisson distribution models packet arrival times as an independent identically distributed process with an exponential distribution.However,it has been demonstrated in reality that packet interarrival durations do not follow an exponential distribution,resulting in a considerable increase in the error caused by modeling them as a Poisson distribution.User-initiated TCP (Transmission Control Protocol) session arrivals,such as remote login and file transfer,are well-modeled as Poisson processes with fixed hourly rates,but other connection arrivals deviate significantly from Poisson;modeling TELNET (Teletype Network Protocol) packet inter arrivals as exponential greatly underestimates the burstiness of TELNET traffic,according to studies.

The power seriesϒ(ψ,z)given by

with its coefficients as probabilities of Poisson distribution which was introduced by Porwal [3](see also [4–6]).The radius of convergence ofϒ(ψ,z)is obviously infinity which can use proved by ratio test.Porwal [3] also defined and introduced the following series:

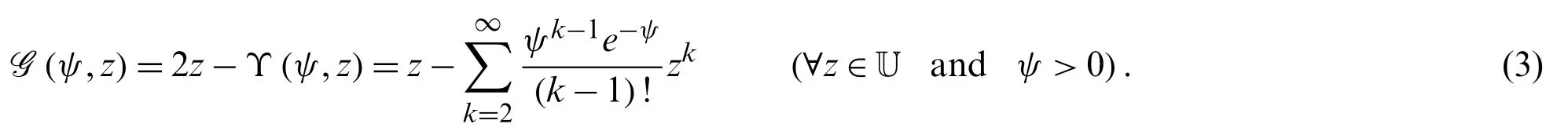
More about special functions and related topics,we may refer to [7–11].

The Basic (orq-) series and basic (orq-) polynomials,particularly basic (orq-) hypergeometric functions and basic (orq-) hypergeometric polynomials,are useful in a wide range of fields,including,for example,Non-Linear Electric Circuit Theory,Finite Vector Spaces,Combinatorial Analysis,Quantum Mechanics,Particle Physics,Mechanical Engineering,Lie Theory,Theory of Heat Conduction,Cosmology and Statistics (see for detail [12]).

In 1748 Euler studied a generating function forpn,the number of partitions of a positive integerninto positive integers by considering the infinite product.

which laid foundation of the study of basic hypergeometric series (also calledq-hypergeometric series orq-series).Nevertheless it took a century to get the status of independent subject after the Heine’s conversion of a straightforward observation that

into a systematic theory of2Φ1basic hypergeometric series which is nothing but parallel to the theory of Gauss’2F1hypergeometric series.

Beside from the influential study of Rogers and Thomae the subject stayed moderately comatose throughout the latter part of the nineteenth century up till Jackson embarked on a long lasting program of developing the theory of basic hypergeometric series in an organized mode(see [13] and [14]).

We next recollect certain elementary and useful concept details of theq-difference calculus,we let throughout the paper that

(0＜q＜1)and(0 ≤μ,ρ＜1).

Definition 1.3.Aq-generalization,q-extension,q-analogue or aq-deformation of an arbitrary numberλis given by

Definition 1.4.Let theq-factorial [n]q! withq∈(0,1)is define by

Definition 1.5.In a given subset of the set C of complex numbers,for functionf∈A,theq-derivative (orq-difference) operatorDqis defined by (see [13] and [14])

provided thatf′(0)exists.

In a given subset of C we can easily see from (4) that

whereasfis differentiable function.Furthermore,from (1) and (4) we obtain

In Geometric Function Theory of Complex Analysis,the role of theq-difference (or theqderivative) operatorDqis remarkably significant.In his article published by Ismail et al.(see[15]),presented theq-deformation of the class ofS*of starlike functions.However,historically speaking,in 1989,in the context of Geometric Function Theory,the usage of theq-difference (or theq-derivative) operatorDqwere systematically given by Srivastava [16].

More recently,Srivastava published a review article [12],in which the applications of theDqoperator in Geometric function Theory were survived.In the development of Geometric Function Theory,the works of Srivastava [16] and Ismail et al.[15] further motivate the researchers to give their finding to this field.For example,it were Wongsaijai et al.[17],who significantly studied certain subclasses ofq-starlike functions.In particular,they studied the inclusion results,radius problems and certain sufficient conditions for their defined functions classes.More recently,the works of Wongsaijai et al.[17] have been generalized by Srivastava et al.(see [18,19]) in a systematic way.In fact Srivastava et al.(see [18,19]) make use of theq-Calculus and certain Janwoski functions in order to devolved their results,which essentially are the generalizations of[17].Some more recent works related toq-calculus can be found in [20–28].

Motivated by the above-mentioned works of Srivastava [12,16] and Ismail et al.[15],in this article we shall mainly generalize the works presented in [1] and [3].We shall study a number of sufficient conditions here.A necessary and sufficient condition shall be encounter for a certain functionGq(ψ,z)∈Fq(μ,ρ)whose coefficients are nothing but merely the probabilities ofq-Poisson distribution.In order to validate our results many new and known consequences are also included.

2 The Subclasses Fq(μ,ρ)and Hq(μ,ρ)of Analytic Functions

In this section,making use of the concept ofq-calculus and the aforementioned works,we first define certain new subclassesFq(μ,ρ)andHq(μ,ρ)of analytic functions which involve theq-calculus.We then give certain sufficient conditions and closure results for these defined function classes.We have also highlighted some known and new consequences of our main results.The classFq(μ,ρ)can be define as:

Definition 2.1.A functionfwith series representation (1) is to be placed in the classFq(μ,ρ)if

Remark 1.First of all,it is easy to see that,if we putμ=0,we have

Fq(0,ρ)=Fq(ρ)=T S*q (ρ),

whereT S*q (ρ)is the function class,introduced and studied in [17].Secondly,we have

whereF(μ,ρ)is defined and studied in [1].Thirdly,we see that

whereT S*(ρ)function class is defined and studied by Silverman [2].

Definition 2.2.A functionfwith series representation (1) is to be placed in the classHq(μ,ρ)if

2.1 Sufficient Conditions for the Class Fq(μ,ρ)and Hq(μ,ρ)

Theorem 2.1.A functionfof the form (1) is in the classFq(μ,ρ)if and only if

For the following functionf1(z)the result is best possible

Proof.If we assume thatf∈Fq(μ,ρ),then we have

If the complex numberzlie on the real axis side,then

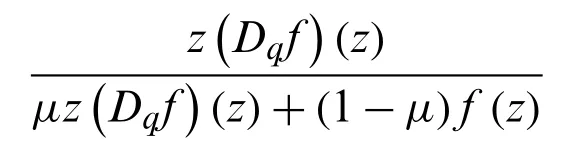
is real.And so in limiting case if we takez→1－,along the real line,then,we have

We see that the last inequality is equivalent to (6).

Conversely,suppose that (6) hold true.Then adding

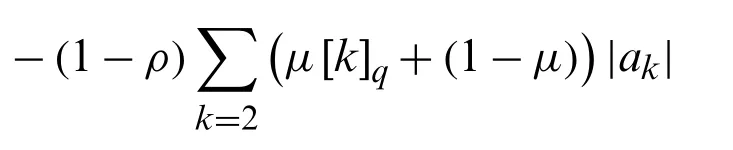
to both sides of (6),we get

On the other hand,we see that

It is now easy to see from (7) that the last expression in (8) is bounded above by(1－ρ).Which evidently showing thatf∈Fq(μ,ρ).

Corollary 1.Iff∈Fq(μ,ρ)then,we have

Remark 2.First of all,we see that if we putμ=0,in Theorem 2.1,we get the known result due to Wongsaijai et al.(see [17]).Secondly if we putμ=0 and letq→1－,Theorem 2.1,we have the sufficient condition for the classS*(ρ),which was studied by Silverman [2].Thirdly,if we letq→1－,Theorem 2.1 coincide with a known result given in [1].

Theorem 2.2.A functionfof the form (1) is in the classHq(μ,ρ)if and only if

The equality holds for the functionf1(z)which is given by

Proof.This theorem can be proved by using the arguments similar which we used in Theorem 2.1.

2.2 Closure Theorems for the Class Fq(μ,ρ)

We define the functionfκ(z) (κ=1,2,3,...,l)as

Furthermore,we now give certain closure results for the function involved in (10).

Theorem 2.3Let the functionsfκ(z) (κ=1,2,3,...,l)defined by (10) be in the classf∈Fq(μ,ρ).Then the functionT∈Fq(μ,ρ),where

Proof.From (10),we have

Now making use of Theorem 2.1,we have

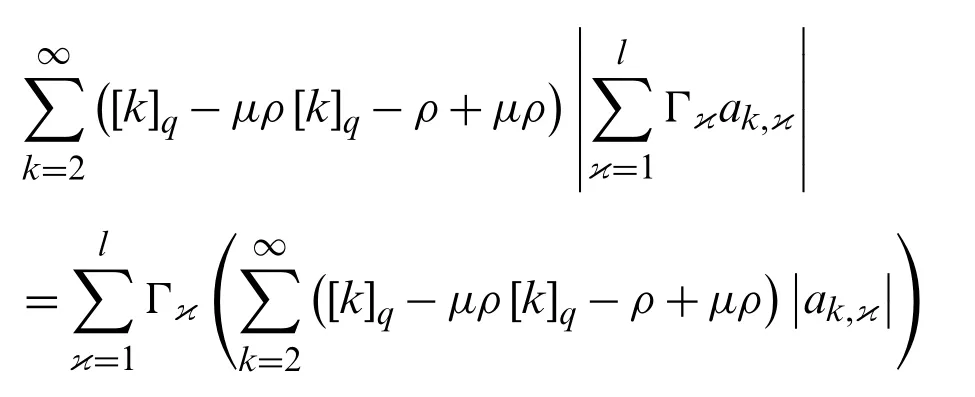
Now by Theorem 2.1,the proof of Theorem 2.3 is completed.

Theorem 2.4.The classFq(μ,ρ)defined in Definition 6 is closed under the convex combination.

Proof.To prove our result,we let that the given functionsfκ(z) (κ=1,2)which are defined by (10) be in the classFq(μ,ρ).It is enough to show that

g(z)=ρf1(z)+(1－ρ)f2(z)(0 ≤ρ≤1)

is in the classFq(μ,ρ).Since

By Theorem 2.1,we have

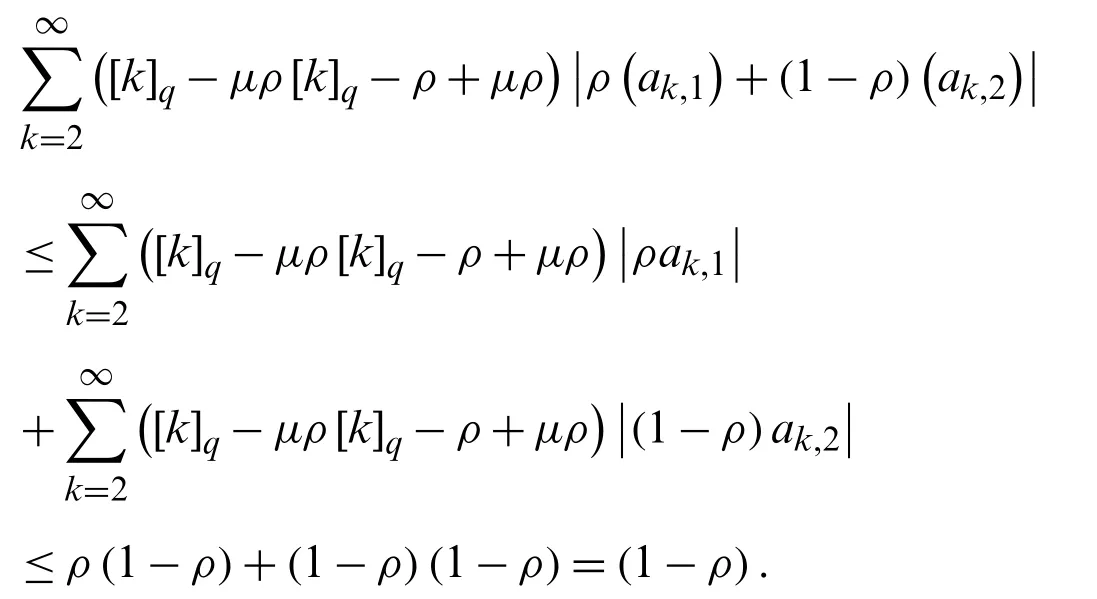
which evidently complete the proof of Theorem 2.4.

3 The Classes Fq(μ,ρ)and Hq(μ,ρ)Involving q-Poisson Distribution

The Euler distribution or Heine distribution,as shown in [29] is aq-deformed Poisson distribution that is known in the literature.All of these,of course,are natural deformations in some sense,but they are all discrete.Kemp [30] demonstrated that the Euler and Heine distributions are,respectively,the limiting forms of aq-analogue of the negative binomial distribution and one of the binomial distribution.Jing [29] proposed a new type ofq-analogue of the binomial distribution that uses the Euler distribution as its limiting form.

Furthermore,the theory ofq-discrete distributions is quite significant because of theoretical probabilistic and statistical interest.In quantum probability it appears at Brownian motion,further from fascinating Markov chains with discrete finite or infinite state spaces it arises as a steady state distributions (see for example [29–31]).Our aim in this section is to consider a necessary and sufficient condition for a functionGq(ψ,z)∈Fq(μ,ρ)such that its coefficients are probabilities ofq-Poisson distribution.Some known consequences are also highlighted.Theq-Poisson distributionNqcan be define as follows (see [32]):

where

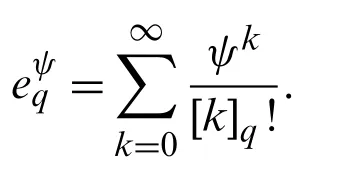
The graph ofeψqis given in Fig.1.

In other words,ifNqtakes the values 0,1,2,3,··· with probabilitiesfor the parameterψrespectively,thenNqis calledq-Poisson distributed.

Figure 1:Graph of eψq with q=0.5 and －1＜ψ＜1

In the following equations we settled a power series so that its coefficients are probabilities the ofq-Poisson distribution as

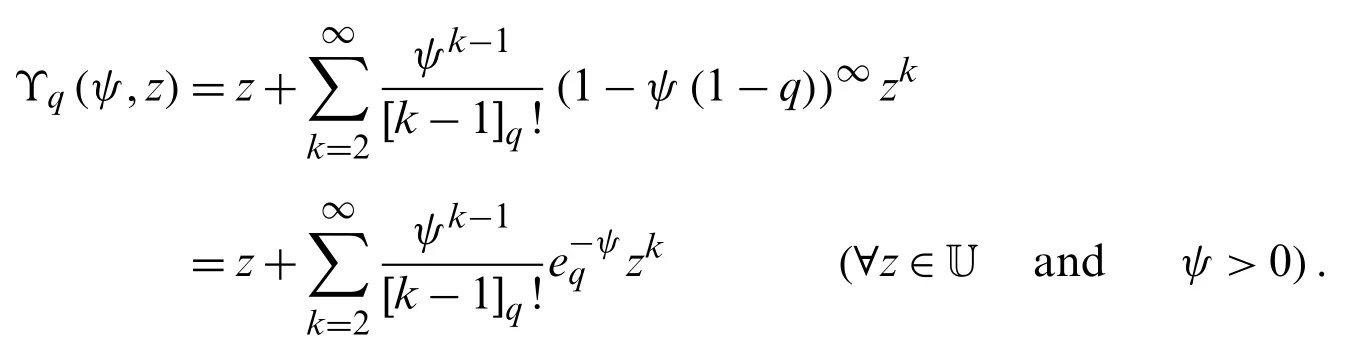
It could be seen that by ratio test the radius of convergence ofϒq(ψ,z)is infinity.Therefore we define the following series:

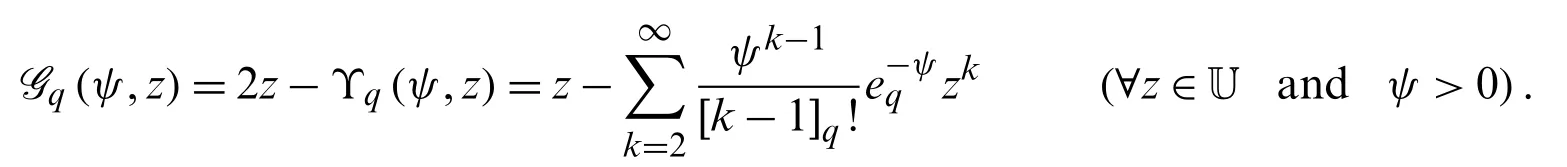
Theorem 3.1.A functionGq(ψ,z)is to be placed in the functions classFq(μ,ρ)if and only if

Proof.Since

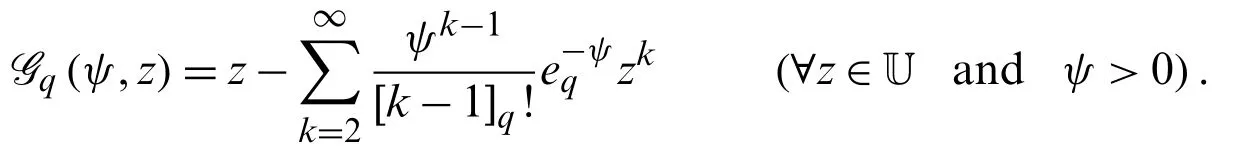
According to Theorem 2.1,we must show that

Therefore,we now consider

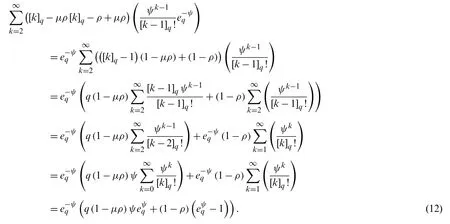
The last expression in (12) is evidently bounded by functional(1－ρ)in the case if and only if relation given in (11) holds true.

Thus we complete the required proof of our Theorem.

Corollary 2.A functionGq(ψ,z)is to be placed in the functions classFq(0,ρ)=T S*q (ρ)if and only if

Remark 3.If we setq→1－ in Theorem 3.1,it will lead us to the result given by Porwal [3].

Theorem 3.2.A functionGq(ψ,z)is to be placed in the classFq(μ,ρ)if and only if

Proof.Since

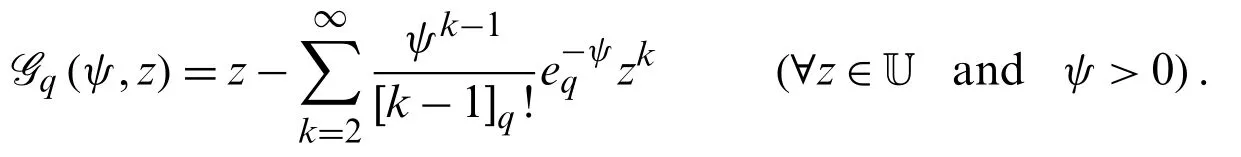
According to Theorem 2.2,we must show that

Therefore,we now consider

We in last expression see that the(1－ρ)is bound of (15) if and only if (11) holds true.

Thus we have completed the proof of our Theorem.

Remark 4.If in Theorem 3.2,we letq→1－,we will arrive at the result given by Porwal [3].

4 Concluding Remarks and Observations

In our present work,by using theq-calculus,we have first defined certain new subclasses of an analytic function.We then gave necessary and sufficient conditions for each of the defined function classes.We have also studied necessary and sufficient conditions for a function whose coefficients are probabilities ofq-Poisson distribution.We have also given particular known and new consequences in the form of Remarks and Corollaries.

The usage of basic (orq-) series in many diverse areas of Mathematics and Physics makes it very important.By using the basic (orq-) series,some wonderful works have been done.As we described in Section 1,the Srivastava’s observation [12] about the so-called(p,q)-calculus,we arrived at the point that indeed the result presented in this paper can be produced for the rather straightforward(p,q)-variations.

Authors’Contributions: All authors contributed equally to this work.All authors have read and agreed to the published version of the manuscript.

Availability of Data and Materials: Not applicable.

Funding Statement: The authors received no specific funding for this study.

Conflicts of Interest: The authors declare that they have no conflicts of interest to report regarding the present study.