Effects of magnetic field on the evolution of the wave function of a charged particle with an angular momentum

Pei-Song He and Yao-Hui Zhu

Physics department,Beijing Technology and Business University,Beijing 100048,China

Abstract We have calculated the effect of a magnetic field on the evolution of angular momentum eigenfunctions of a charged particle.An additional harmonic potential is supplemented to trap the wave packet.We find the probability density of the wave function is oscillating in the radial direction with a time period determined by the strength of the effective harmonic potential.When the magnetic field is along the z direction,if the initial wave function is an eigenfunction of,the probability density of the particle remains axis-symmetric.While for the case of an eigenfunction of,it is anisotropic in the x−y plane and rotates with a time period inverse proportional to the strength of the external magnetic field.We also extend the results in a phenomenological way to the case with an external magnetic field that varies harmonically in time.

Keywords:angular momentum,uncertainty,magnetic field

1.Introduction

Dynamics play fundamental roles in quantum physics.When an external field is applied to a quantum system,the wave function of the system evolves in time.The properties of the system may then be modified.The field can then be served as a probe to investigate the properties of the system.The coupling of magnetic field and charged particles leads to many interesting phenomena,such as Landau levels,the Aharonov–Bohm effect[1],the integral and fractional quantum Hall effect[2,3],the Messiner effect[4],etc.

Angular momentum is one of the elementary properties of particles.Recently,much work has been done on angular momentum-related quantum phenomena.It has been found that a magnetic field can modify the angular momentum of an electron[5,6].The magnetic field can affect the chemical reaction when the molecules have angular momentum[7].Despite these processes,a detailed wave function of a particle with angular momentum evolving in a magnetic field is still lacking.In this paper,we have obtained the evolution of eigenfunctions of various angular momenta,and some interesting features have been found.The group velocity of the particle is set to zero.It will experience no Lorentz force in a magnetic field according to classical theory[8].But in reality,due to the wave quality,there also exists dynamics.Furthermore,for a charged particle with orbital angular momentum,it has the corresponding magnetic momentum[9].The coupling of the latter with the magnetic field also leads to the dynamics of the particle.

Our main findings are that the uniform magnetic field plays effective roles in two aspects:one is that it serves as a two-dimensional harmonic potential,which makes the wave function oscillate with a time period related to the strength of the potential;the other is that it serves to rotate the wave function with respect to an axis along the direction of the magnetic field,with a time period inverse proportional to the strength of the magnetic field.We also extend the results phenomenanically to the case with an external magnetic field that varies harmonically in time.We argue that the probability density of the eigenfunctions ofoscillates between clockwise and anticlockwise with the magnitude of the angular velocity varying harmonically in time.

2.Evolution of eigenfunctions of orbital angular momentum

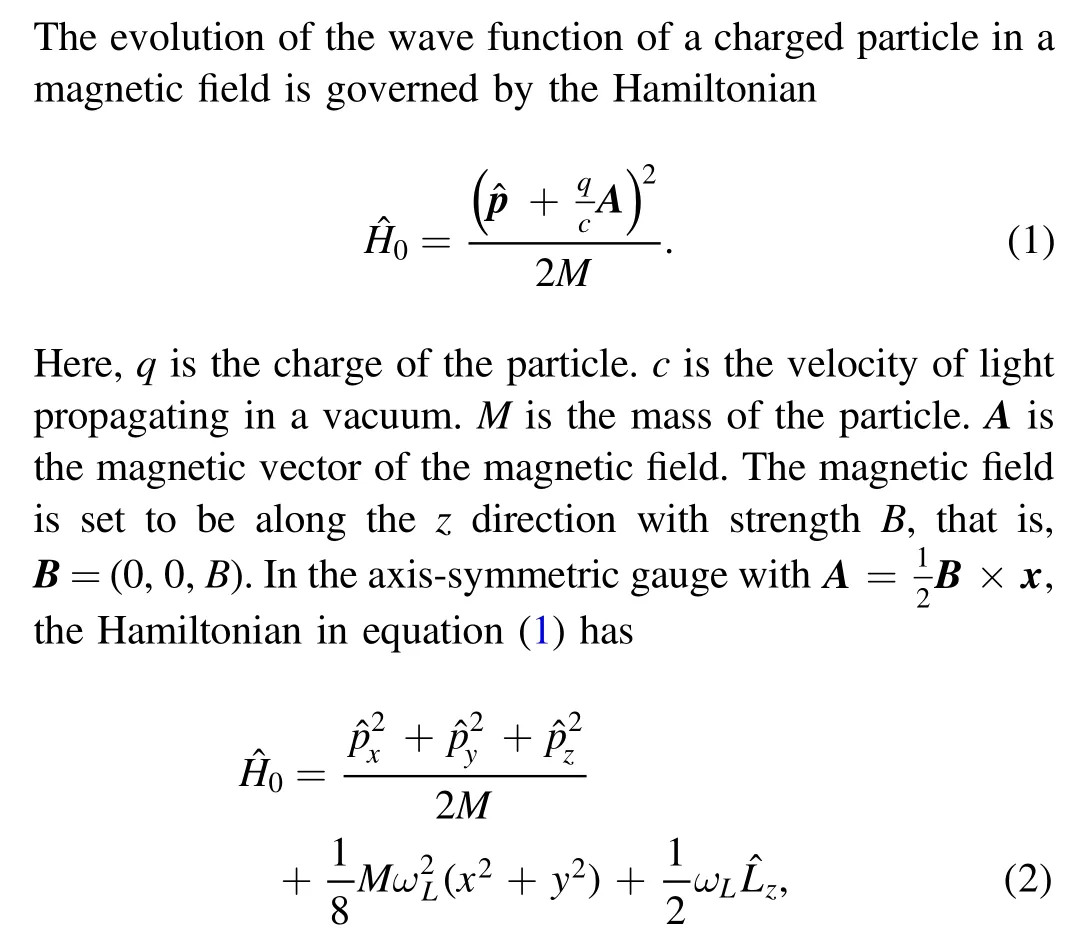
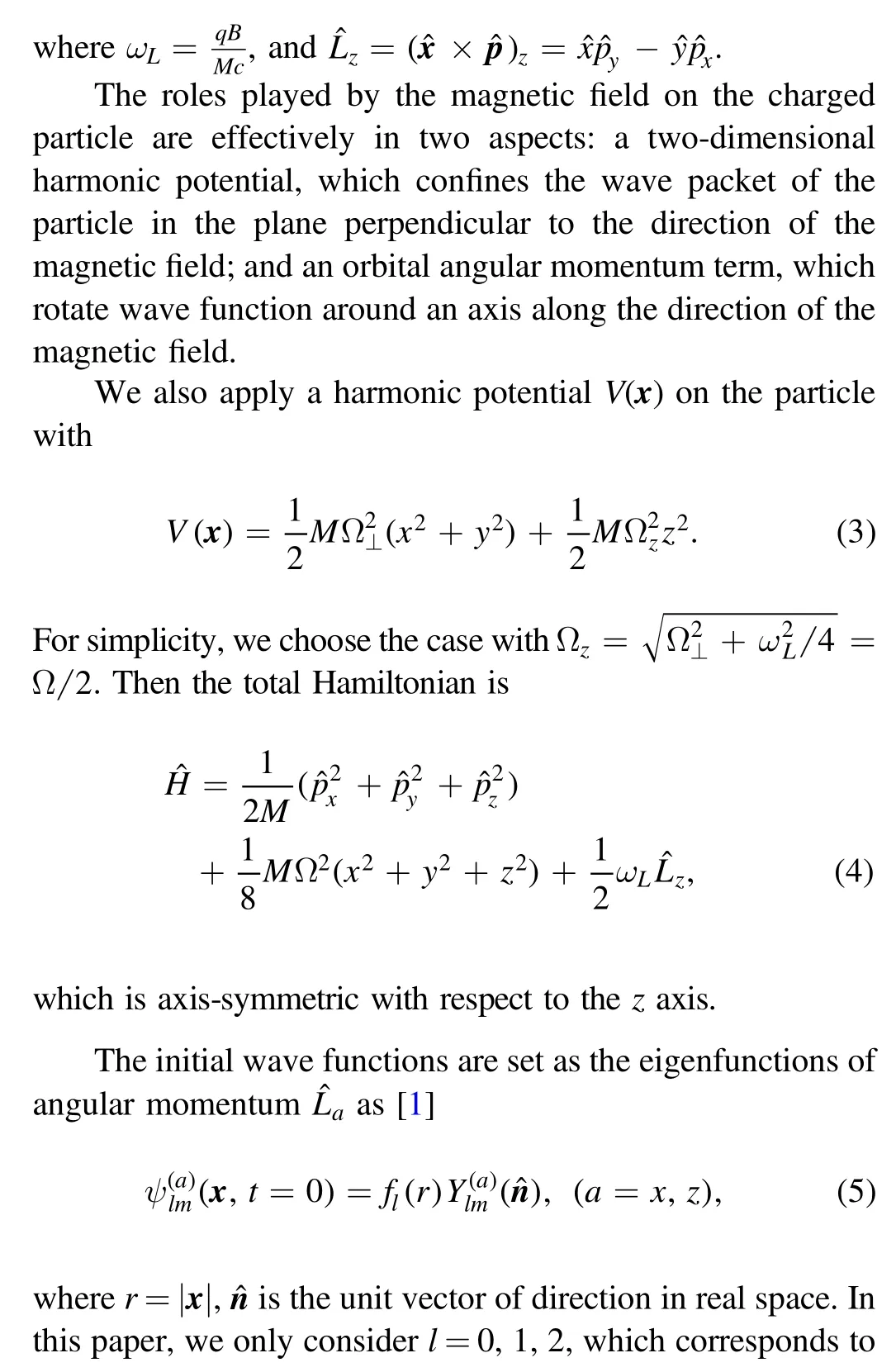
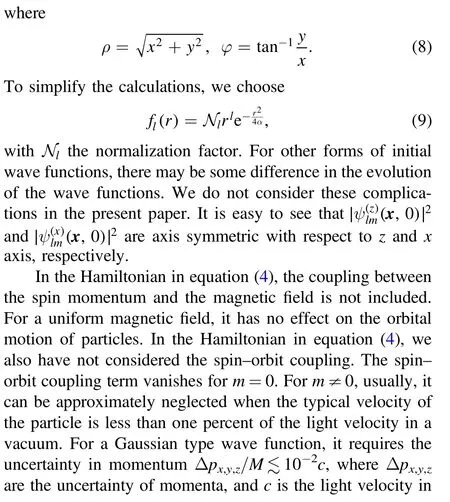
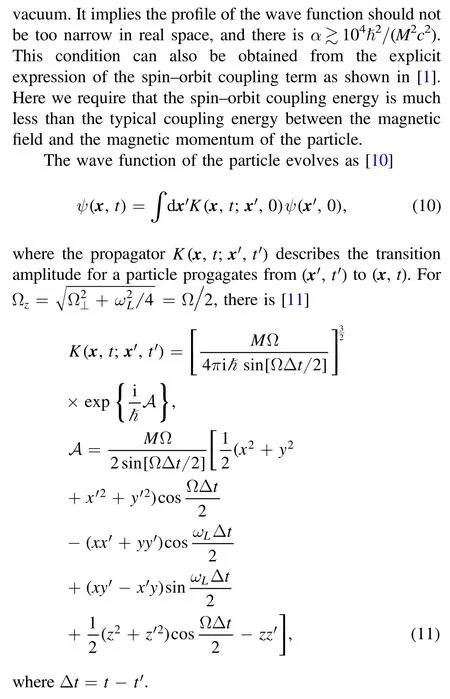
3.Results and discussions

Since ωLis inverse proportional to the mass of the particle,the particle will probably rotate slowly under a magnetic field.It can be a quasiparticle with a heavy mass,such as the heavy fermions in Kondo lattices[12],fermions in flat bands[13],etc.It can also be a charged atom or molecule.This rotation may be detectable experimentally.The rotation of the anisotropic wave packet probably affects the interactions between atoms or molecules[7,14].

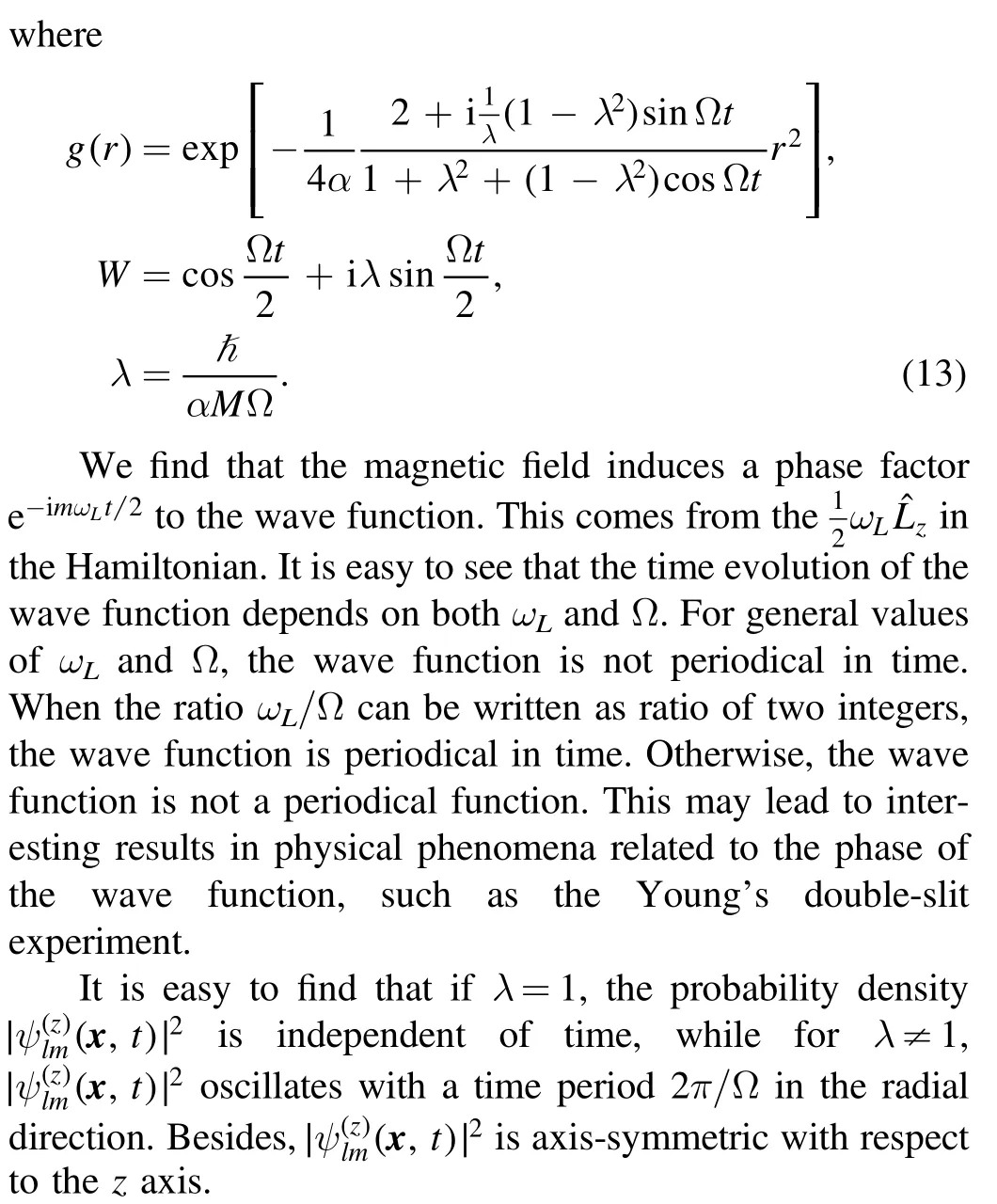
It is also of interest to consider the case when the rotation is extremely fast,corresponding to a large external magnetic field.In such a case,the profile of the wave packet may be unstable and split into parts,similar to what happened to quantum vortices with quantum numbers too large[15,16].Recently,ultracold atom experiments found that when a columnar neutral atomic cluster will be split into smaller parts when it rotates too fast[17].

4.Harmonic oscillating magnetic field

The above results that the probability density of wave functions rotates in a time-independent magnetic field can also be obtained in a phenomenological way using the interaction of the angular momentum of the quantum particle with the magnetic field.There is

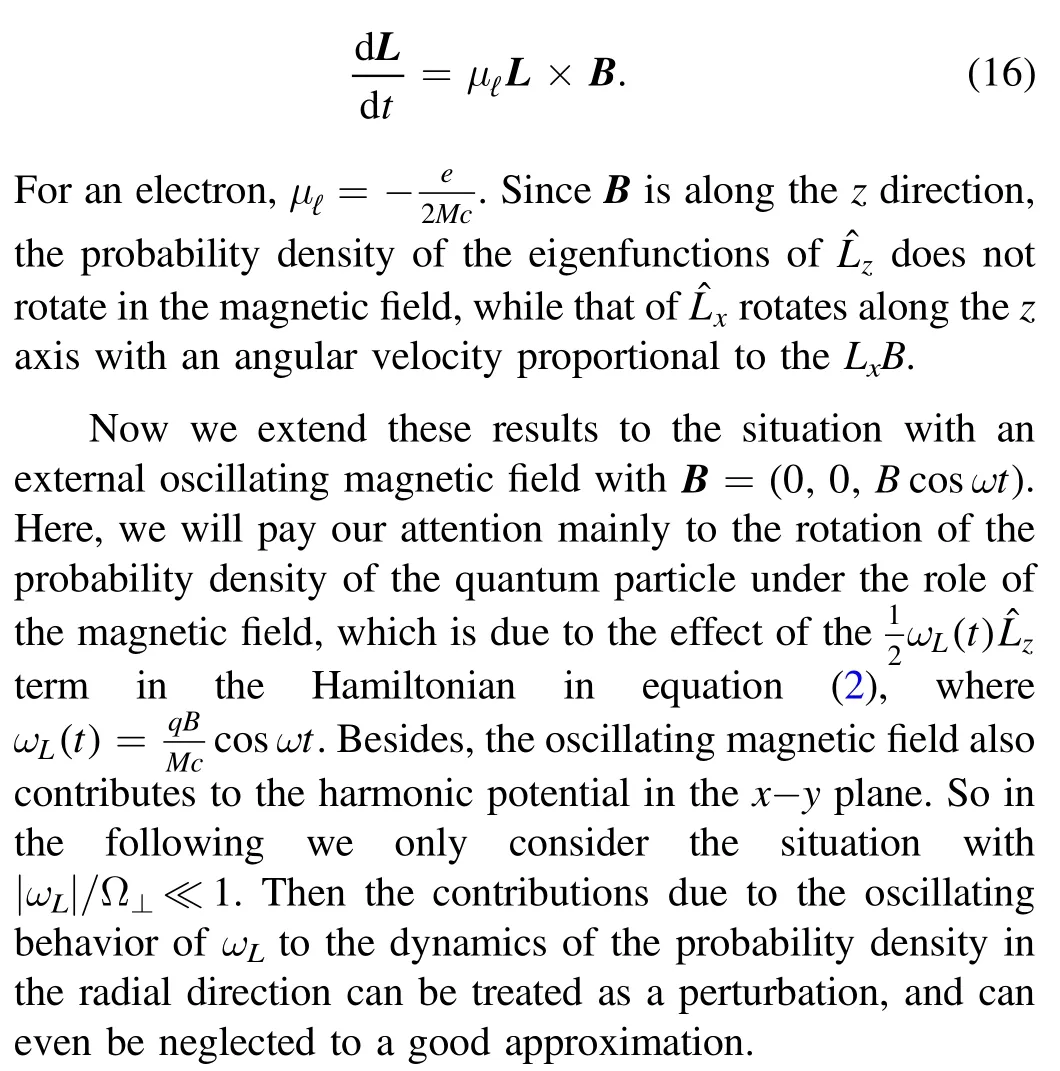
From the Maxwell equations,there coexists oscillating electric fields.In the simplest case with propagating electromagnetic fields in which the wavelength is much longer than the typical size of the wave packet of the quantum particle,the vector electric field is perpendicular to the magnetic fields.So to study the rotation of the probability density of the quantum particle,we can omit the effects of electric fields.We just consider the effects of the magnetic fields on the probability density of the quantum particle,which can be described phenomenanically by the interactions between the magnetic fields and the angular momentum.

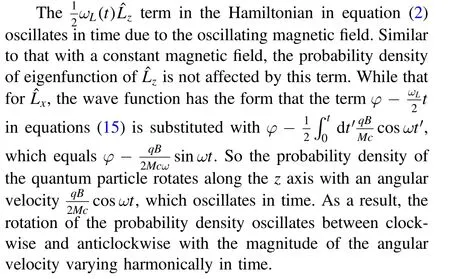
5.Conclusions

In this paper,we use a path integral method to calculate the effect of the magnetic field on the evolution of the eigenfunctions of angular momentum.The roles played by the magnetic field on the charged particle are effectively two aspects:a two-dimensional harmonic potential in the plane perpendicular to the magnetic field,which confines the wave packet of the particle;and a term proportional to the orbital angular momentum operator,which rotates wave function around an axis along the direction of the magnetic field.To confine the wave packet in the direction along the magnetic field,an extra harmonic potential is also applied.

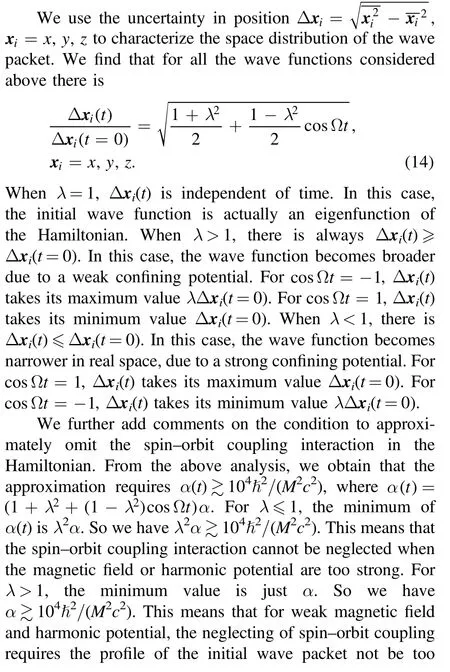
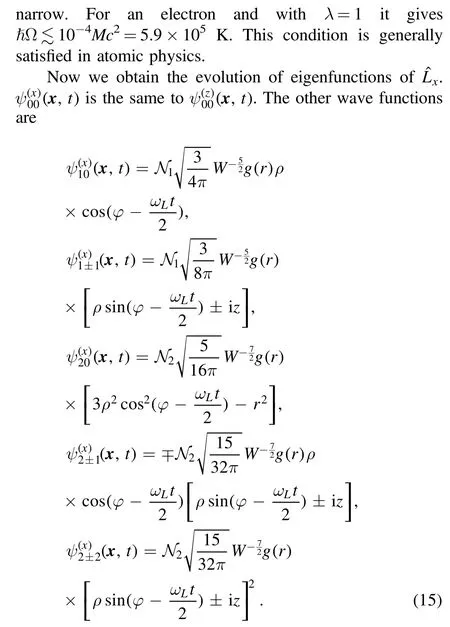
In coordinates with the magnetic field along thezdirection,we find that whether the initial wave functions are eigenfunctions ofor,the probability density of the particle oscillates radially with a time period determined by the strength of the effective harmonic potential.We also find that in the case of the initial wave function of,the probability density of the particle remains axis-symmetric with respect to thezaxis,while the counterpart ofis anisotropic in the perpendicular plane,and rotates with a time period inverse proportional to the strength of the magnetic field.

We also extend the results in a phenomenological way to the case with an external magnetic field that varies harmonically in time.We argue that when the effective harmonic potential induced by the magnetic field is much weaker than the external harmonic potential,that is,the probability density of the eigenfunctions ofoscillates between clockwise and anticlockwise with the magnitude of the angular velocity varying harmonically in time.