Generalization of the theory of three-dimensional quantum Hall effect of Fermi arcs in Weyl semimetal

Mingqi Chang(苌名起) Yunfeng Ge(葛云凤) and Li Sheng(盛利)

1National Laboratory of Solid State Microstructures and Department of Physics,Nanjing University,Nanjing 210093,China

2Collaborative Innovation Center of Advanced Microstructures,Nanjing University,Nanjing 210093,China

Keywords: Weyl semimetal,three-dimensional quantum Hall effect,Fermi arcs

1. Introduction

Quantum Hall effect (QHE), which is discovered in two-dimensional (2D) electron gases, opens a new era of condensed matter physics.[1–5]To generalize the QHE to three-dimensional (3D) systems, tremendous effort has been made.[6–15]Recently,the 3D QHE has been theoretically predicted to occur in Weyl semimetals.[16–18]Weyl semimetals are new topological states of matter of which low-energy bulk excitations can be explicited by Weyl equations.[19–28]The energy spectrums of Weyl equations touch at discrete points,called Weyl nodes. The topologically protected surface states between the Weyl nodes form Fermi arcs,which are the landmark feature of Weyl semimetals.[19]In a Weyl semimetal,the Fermi arcs at two opposite surfaces can be connected by the Weyl nodes via a “wormhole” tunneling, forming a complete Fermi loop producing the QHE.[16]Following the prediction,the quantized Hall plateaus have been experimentally observed in the 3D topological semimetal Cd3As2.[29–35]In Ref. [16], the Fermi arcs can support the QHE only when the Fermi energy is at Weyl nodes and the band anisotropy terms exist,which is hardly achievable. Meanwhile,the thickness dependence of Fermi-arc 3D QHE is unclear. However,Ref.[30]showed that the quantum Hall resistance is sensitive to the sample thickness. These contradictions between theory and experiments suggest that the present theory of the 3D QHE of Fermi arcs is imperfect.

In this paper, we generalize the theory of the 3D QHE of Fermi arcs in Weyl semimetal. Through calculating the sheet Hall conductivity of a Weyl semimetal slab, we show that the 3D QHE of Fermi arcs can occur in a large energy range and the thickness dependences of the QHE in different Fermi energies are distinct. When the Fermi energy is near the Weyl nodes,the Fermi arcs from two surfaces form a complete Fermi loop needed by QHE. The Fermi arcs give rise to the QHE which is independent of the thickness of the slab. In this case, the anisotropic terms are necessary, or the QHE cannot occur. When the Fermi energy is not at the Weyl nodes and is not out of the energy range of Fermi arc states, the Fermi arcs at two surfaces form a complete Fermi loop with the assistance of bulk states.The Fermi arcs and bulk states give rise to the QHE together which is dependent on the sample thickness. The anisotropic terms are not necessary in this case. The Fermi arc surface states are essential in both two cases. When the Fermi energy is out of the energy range of Fermi arcs,only the bulk states contribute to the Hall conductivity destroying the quantized plateaus of QHE. Our theory complements the imperfections of the present theory of 3D QHE of Fermi arcs.

The rest of the paper is organized as follows. In Section 2,we introduce the model Hamiltonian. In Section 3,we plot the energy spectrum for bulk states and Fermi arc states,and make a brief analysis according to the energy spectrum.In Section 4,the sheet Hall conductivities in different Fermi energies are calculated numerically when aydirection magnetic fieldB=(0,B,0)is applied.The final section contains a brief summary.

2. Model

We consider a 3D model of Weyl semimetal with two Weyl nodes,[16,36–40]

3. Energy spectrum

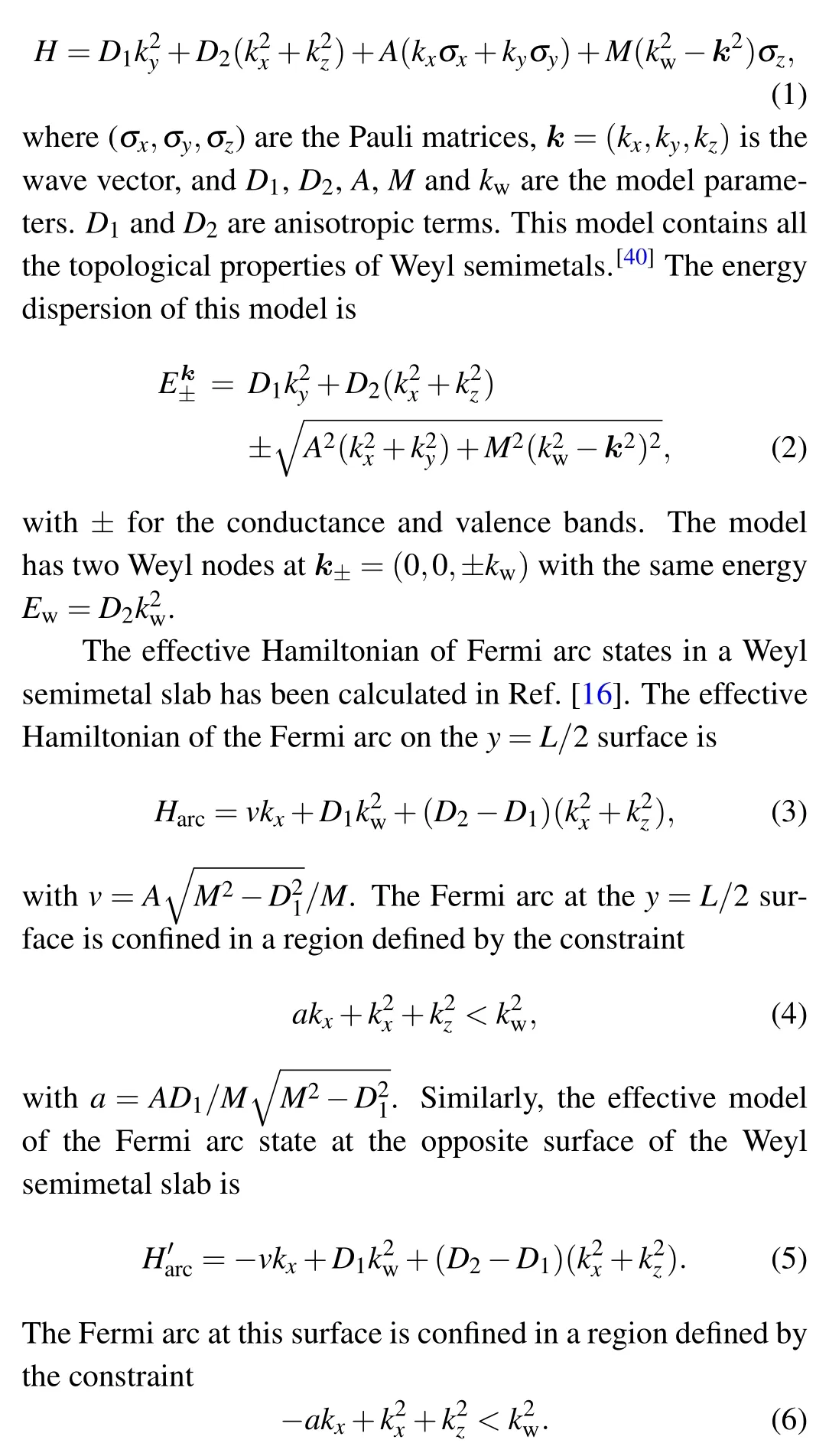
In Figs. 1(a) and 1(b), we plot the energy spectra for bulk states and Fermi arc surface states of the Weyl semimetal as functions ofkxandkzwith different anisotropicDterms.The Fermi arc states are plotted as blue and pink curves while the bulk states are plotted as red curves. In Fig. 1(a),when the anisotropic terms areD1=D2=0, the Fermi arc states disperse linearly withkxwhile the Fermi arc is not in Fig.1(b),when the anisotropic terms areD1=2 eV·nm2andD2=3 eV·nm2.

In Figs. 1(c) and 1(e), we plot the Fermi arc states and bulk states on the plane ofkxandkzforD1=D2=0 eV·nm2.When the Fermi energy is precisely at the Weyl nodes, the Fermi arc states of the two surfaces on the plane ofkxandkzform a straight line as shown in Fig.1(c). However,when the Fermi energy is not at Weyl nodes,the Fermi arcs at opposite surfaces can form a complete Fermi loop with the assistance of bulk states in Fig.1(e).

Fig.1. (a)and(b)Energy spectra for bulk states and Fermi arc states with(a) D1 =D2 =0 eV·nm2, (b) D1 =2 eV·nm2, D2 =3 eV·nm2. (c)–(f)Fermi arc states and bulk states on the plane of kx and kz for different Fermi energy. In (c) EF =0 eV and (e) EF =0.02 eV, the anisotropic terms are D1 = D2 = 0 eV·nm2. In (d) EF = 0.27 eV and (f) EF = 0.29 eV, the anisotropic terms are D1 =2 eV·nm2, D2 =3 eV·nm2. The blue curves and pink curves are the Fermi arc states plotted using Eqs.(3)and(5)while the bulk states are plotted as red curves using Eq.(2).

In Figs. 1(d) and 1(f), the situation is different when the anisotropic terms areD1=2 eV·nm2andD2=3 eV·nm2. In Fig.1(d),the Fermi arcs at two surfaces can form a complete Fermi loop themselves when the Fermi energy is precisely at Weyl nodes. When the Fermi energy is not at Weyl nodes,the Fermi arcs at two surfaces form a complete Fermi loop with the assistance of bulk states as shown in Fig.1(f).

We can make a brief analysis according to Fig. 1. First,when the Fermi energy is at Weyl nodes, the Fermi arcs at opposite surfaces can form a complete Fermi loop needed by QHE only when the anisotropic terms exist. Second,when the Fermi energy is not at Weyl nodes,the Fermi arcs at two surfaces form a complete Fermi loop with the assistance of bulk states no matter the anisotropic terms exist or not. Therefore,the Fermi arcs in Weyl semimetal can give rise to the QHE in a large energy range.On the one hand,when the Fermi energy is at Weyl nodes and the anisotropic terms exist,the Fermi arcs at two opposite surfaces form a complete Fermi loop giving rise to the QHE. Because the Fermi arcs are independent of the sample size, the QHE is independent of the thickness of the sample in this case. On the other hand,when the Fermi energy is not at Weyl nodes,the Fermi loop needed by QHE is formed by the cooperation of Fermi arc states and bulk states. In this situation,the QHE is dependent on the thickness of the sample because the bulk states are size dependent. In the following,we calculate the Hall conductivity of a Weyl semimetal slab to verify our analysis.

4. Hall conductivity

We numerically calculate the Hall conductivity of a Weyl semimetal slab in a magnetic field using the Kubo formula[14–16]

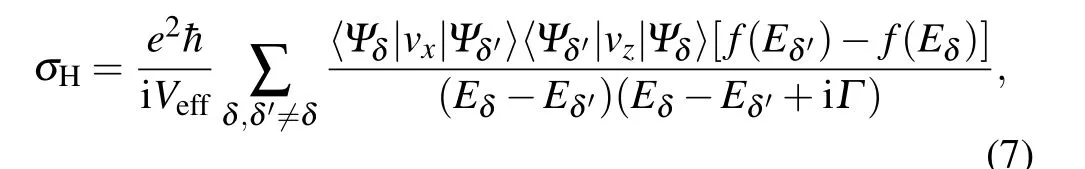
where|Ψδ〉is the eigenstate of energyEδforHin ay-direction magnetic field with open boundaries aty=±L/2,Veffis the volume of the slab,vxandvzare the velocity operators, andf(x)is the Fermi distribution.

The sheet Hall conductivity for the Weyl semimetal slab is defined byσsH=σHL. We numerically calculate the sheet Hall conductivity at zero temperature using the same method as in Refs.[16,41].

In Figs.2(a)and 2(b),we show the sheet Hall conductivity as a function of Fermi energyEFfor different anisotropic terms and sample sizes. The sheet Hall conductivity is quantized in units ofe2/hno matter the anisotropic terms exist or not. However, the plateaus of sheet Hall conductivity are obviously different in Figs. 2(a) and 2(b). When the Fermi energy is near Weyl nodes,σHsis zero forD1=D2=0 eV·nm2in Fig. 2(a) whileσHsis nonzero forD1=2 eV·nm2,D2=3 eV·nm2in Fig.2(b). It means that the anisotropic terms are necessary for the QHE in Weyl semimetal when the Fermi energy is near the Weyl nodes.Moreover,when the Fermi energy is near Weyl nodes,σHsis independent of the thickness of the slab as shown in Fig.2. When the Fermi energy is not near the Weyl nodes,the plateaus of sheet Hall conductivity depend on the sample size. TheσHsis symmetric about the energy of Weyl nodes in Fig.2(a)while is not in Fig.2(b)because of the anisotropic terms.

In Fig. 3(a), we plot the sheet Hall conductivity in units ofe2/has a function of 1/Bwhen the Fermi energy is at Weyl nodes for different anisotropic terms. WhenD1=D2=0 eV·nm2, the top and bottom Fermi arcs coincide forming a straight line between two Weyl nodes as shown in Fig. 3(b),which cannot give rise to the QHE.Therefore, theσsHis zero as the red line in Fig.3(a). However,the Fermi arcs at the two surface connected by Weyl nodes can form a complete Fermi loop needed by QHE whenD1=2 eV·nm2,D2=3 eV·nm2as shown in Fig.3(b). TheσsHis quantized in units ofe2/has the green line shown in Fig.3(a),which is the case in Ref.[16].In this situation,the anisotropic terms are necessary for the QHE in Weyl semimetal.

Fig. 2. In a Weyl semimetal slab, the sheet Hall conductivity in units of e2/h as a function of Fermi energy with different thicknesses for B=5 T.The anisotropic terms are (a) D1 =D2 =0 eV·nm2, (b) D1 =2 eV·nm2,D2 =3 eV·nm2. The parameters are M=5 eV·nm2, A=0.5 eV·nm, and kw=0.3 nm-1.

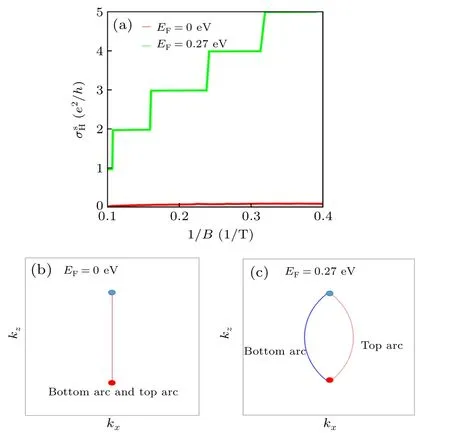
Fig. 3. When the Fermi energy is at Weyl nodes. (a) The sheet Hall conductivity σHs as a function of 1/B for D1 = D2 = 0 eV·nm2 (red line),D1 =2 eV·nm2, D2 =3 eV·nm2 (green line). The thickness of the slab is L=100 nm. Fermi arc states and bulk states on the plane of kx and kz for(b)D1=D2=0 eV·nm2,(c)D1=2 eV·nm2,D2=3 eV·nm2. The red point and blue point are Weyl nodes. The blue curves and pink curves are the Fermi arc states plotted using Eqs.(3)and(5). The other parameters can be found in Fig.2.

Fig. 4. (a) The sheet Hall conductivity σHs as a function of 1/B for D1 =D2 =0 eV·nm2 (red line), D1 =2 eV·nm2, D2 =3 eV·nm2 (green line) and L=100 nm. Fermi arc states and bulk states on the plane of kx and kz for (b) D1 =D2 =0 eV·nm2 at EF =0.02 eV, (c) D1 =2 eV·nm2,D2=3 eV·nm2 at EF=0.29 eV.The other parameters can be found in Fig.2.

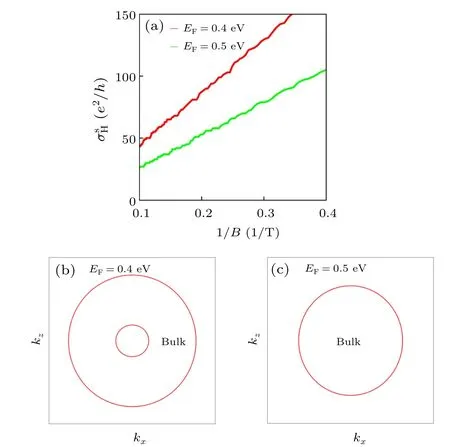
Fig. 5. (a) The sheet Hall conductivity σHs as a function of 1/B for D1 =D2 =0 eV·nm2 (red line), D1 =2 eV·nm2, D2 =3 eV·nm2 (green line)and L=100 nm. Fermi arc states and bulk states on the plane of kx and kz for(b)D1=D2=0 eV·nm2 at EF=0.4 eV,(c)D1=2 eV·nm2,D2=3 at EF=0.5 eV.The other parameters can be found in Fig.2.

We plot the sheet Hall conductivity in units ofe2/has a function of 1/Bwhen the Fermi energy is not at Weyl nodes in Fig. 4(a). In this case, theσsHshows plateaus no matter the anisotropic terms exist or not. This can be seen from the Fermi arc states and bulk states on the plane ofkxandkz. In Figs.4(b)and 4(c),the Fermi arcs form a complete Fermi loop needed by QHE with the assistant of bulk states no matter the anisotropic terms exist or not. The QHE is generated under the combined action of bulk states and Fermi arc states. The Fermi loop in Fig.4(c)is bigger than that in Fig.4(b). This is why the green line is above the red line in Fig.4(a).

In Fig. 5(a), when the Fermi energy is out of the energy range of the Fermi arc states, we plot the sheet Hall conductivity in units ofe2/has a function of 1/Bfor different anisotropic terms. When the Fermi energy is out of the energy range of the Fermi arc states,the bulk states themselves form a complete Fermi loop as shown in Figs.5(b)and 5(c). In this situation,only the bulk states contribute to the Hall conductivity.As like a normal metal,the sheet Hall conductivity follows the usual 1/Bdependence in Fig.5(a). Meanwhile,the Fermi loop in Fig. 5(b) is bigger than that in Fig. 5(c) causing the value ofσsHof red line above the green line in Fig.5(a).

The above results of Hall conductivity are consistent with our analysis in Section 3.

5. Summary

In summary,we generalize the theory of the 3D QHE of Fermi arcs in Weyl semimetal. Through calculating the sheet Hall conductivity of a Weyl semimetal slab,we show that the 3D QHE of Fermi arcs can occur in a large energy range and the thickness dependences of the QHE in different Fermi energies are distinct. The position of Fermi energy plays an important role. When the Fermi energy is near the Weyl nodes,the Fermi arcs at two surfaces form a complete Fermi loop when the anisotropic terms exist giving rise to the QHE.The anisotropic terms are necessary for the QHE in this situation.In this situation, the QHE is independent of the sample size because the Fermi arc states are independent of the sample thickness. When the Fermi energy is not near the Weyl nodes and is not out of the energy range of Fermi arc states,the Fermi arcs form a complete Fermi loop with assistance of bulk states no matter the anisotropic terms exist or not. The QHE is generated under the combined action of bulk states and Fermi arc surface states.Because the bulk states depend on the thickness of the slab,the QHE is dependent on the sample size. Finally,when the Fermi energy is out of the energy range of Fermi arc states,only the bulk states contribute to the Hall conductivity.The plateaus ofσsHcollapse and theσsHfollows the usual 1/Bdependence as like a normal metal. In experiment, it is difficult to distinguish if it is the Fermi arcs producing the QHE in Weyl semimetal. Using our theory, this can be estimated through the different thickness dependence in different Fermi energies.

Acknowledgment

This work was supported by the National Natural Science Foundation of China(Grant No.11974168)(L.S.).