Expressions of Two Classes of Infinite Series in Terms of Bernoulli Numbers

(1.School of Mathematics and Statistics,Zhoukou Normal University,Zhoukou 466001,China;2.School of Mathematics and Statistics,Wuhan University,Wuhan 430061,China)

Abstract:In this paper,the expressions of two classes of infinite series in terms of finite series involving Bernoulli numbers are obtained.As applications,we derive some special series including Dirichlet beta functionβ(s)with argument 2n+1 and Dirichlet lambda functionλ(s)with argument 2n.In addition,we solve the problem proposed recently by Zhou(2021).

Keywords:Bernoulli numbers;Bernoulli polynomials;Polygamma function;Generalized Zeta function

§1.Introduction

The Psi functionψ(z)is defined by

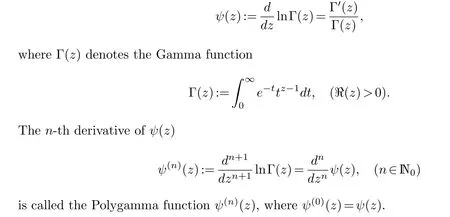
can be expressed by finite series involving Bernoulli numbers,wherea,b∈N.

In the next section,we shall give the finite sum expression of the infinite seriesSn(a,b)defined by(1.6)and provide a proof of Problem 1 mentioned above,as well as a particular caseβ(2n+1).In the third section,the finite series expression of the infinite seriesTn(a,b)defined by(1.7)will be established.And also,as special case,we derive the expression ofλ(2n)in terms of Bernoulli numbers.Then the paper will end in Section 4 with concluding remarks.

§2.Finite series expression of Sn(a,b)

In this section,we first prove the following theorem,and then provide a proof of the Problem 1 mentioned in the last section.

2.1.Main theorem

Theorem 2.1.For the series Sn(a,b)defined by(1.6),we have

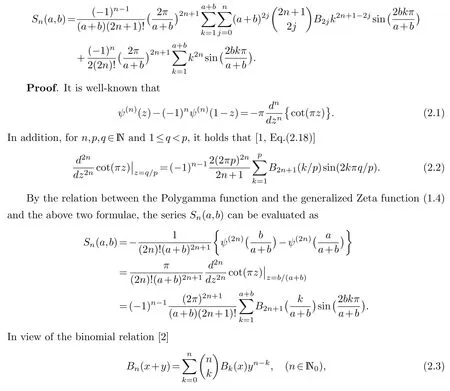
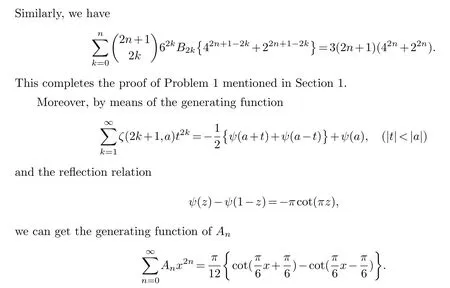
2.2.Proof of Problem 1

Firstly,forA0,we can evaluate it directly by Theorem 2.1.Of course,it can be calculated by other methods.Here,we use the integral approach to compute it.In fact,if let

§3.Finite series expression of Tn(a,b)

In this section,we mainly derive the finite series expression of the infinite seriesTn(a,b)defined by(1.7)and display some particular cases.

Theorem 3.1.For the series Tn(a,b)defined by(1.7)and n∈N,we have

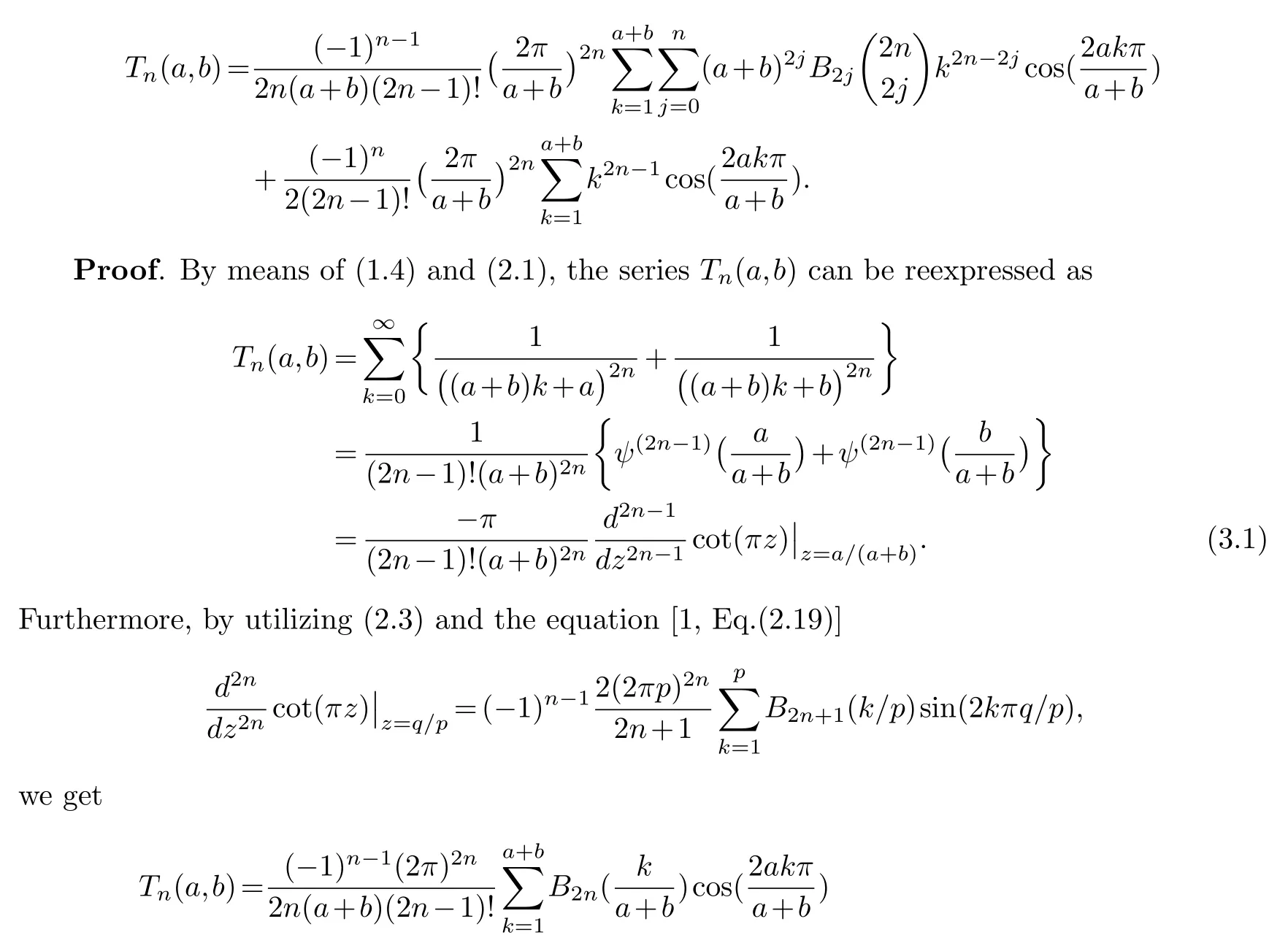
By specifying particular values foraandb,we can obtain many particular cases.For example,by lettinga=b=1,we derive

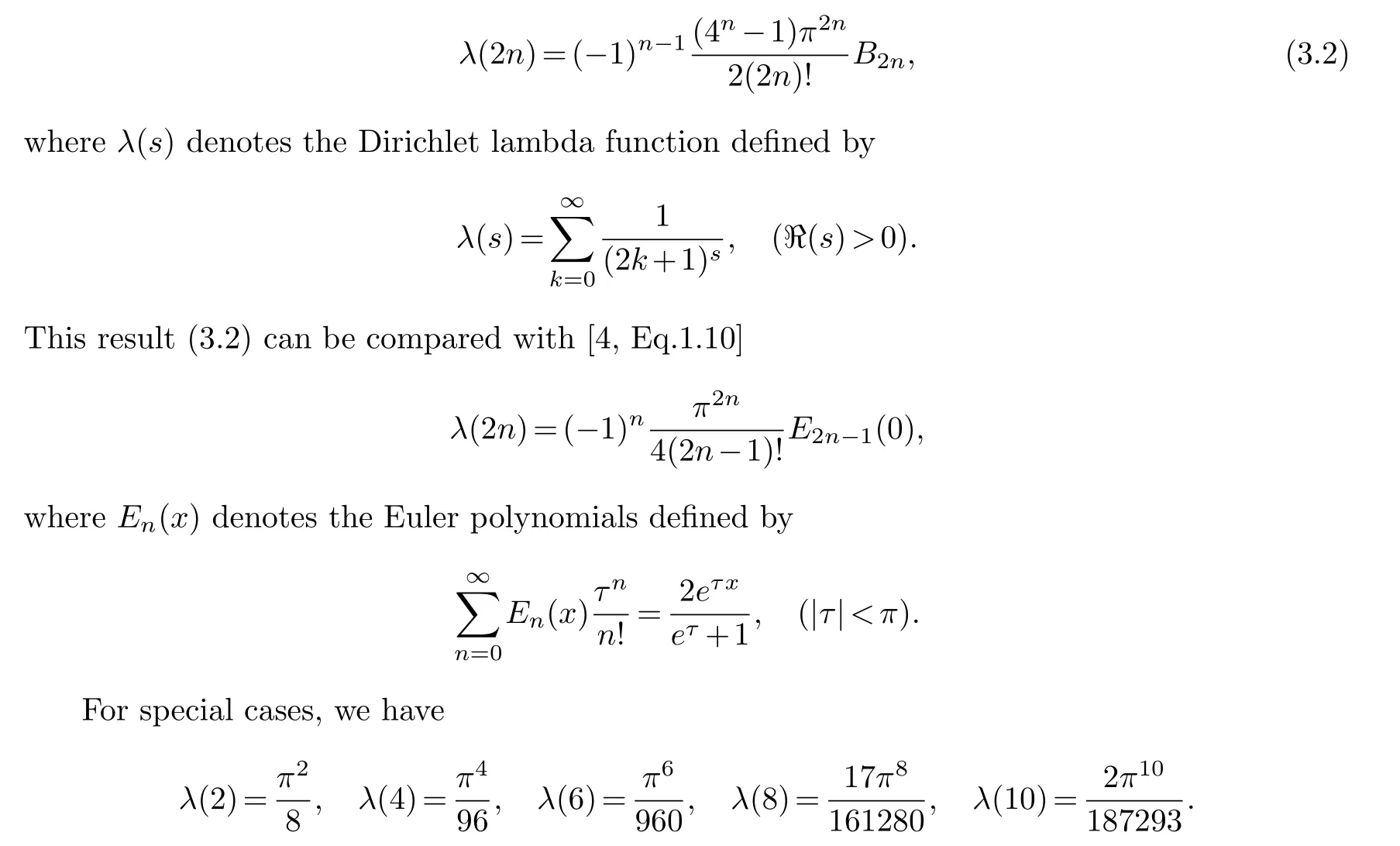
§4.Concluding remarks

The expressions of two families of infinite series in terms of Bernoulli numbers are established.As applications,we prove a monthly problem proposed recently by Zhou,and derive some special cases including Dirichlet beta functionβ(2n+1)and Dirichlet lambda functionλ(2n).The reader can obtain other special cases by specifying specific values of parametersn,aandb.

Acknowledgements

The authors are sincerely grateful to the referees for their careful reading and valuable suggestions.